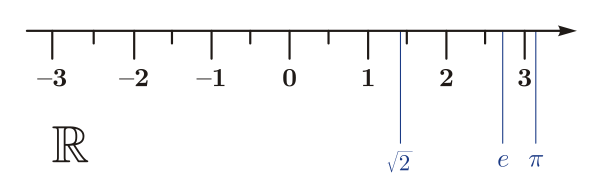
Realusis skaičius

Geometrinė realiųjų skaičių interpretacija skaičių tiesėje.

Realieji skaičiai – visi racionalieji ir iracionalieji skaičiai. Begalinėje skaičių tiesėje kiekvienas taškas atitinka realųjį skaičių. Realiųjų skaičių pavyzdžiai: 0, 2, −1/3, √2, ln 2, π ir skaičius e.

Realiųjų skaičių aibė žymima . žymi n-matę realiųjų skaičių erdvę.

Realusis skaičius vadinamas suskaičiuojamu, jei yra algoritmas, pagal kurį galima suskaičiuoti po kablelio esančius skaitmenis. Kadangi algoritmų aibė yra skaiti, o realiųjų skaičių aibė – neskaiti, dauguma realiųjų skaičių nėra suskaičiuojami.

Istorija

Apie 500 m. pr. m. e. pitagorininkai pastebėjo, kad kvadrato kraštinė ir įstrižainė yra nesuderinami, t. y., nėra tokios atkarpos, kuriai kraštinės ir įstrižainės ilgiai būtų natūralieji kartotiniai. Šiandieniniais terminais tai reiškia, kad nėra tokio racionalaus skaičiaus, kuris būtų kvadrato įstrižainės ir jo kraštinės ilgio santykis. Taip buvo parodytas skaičiaus iracionalumas.

Nuo seniausių laikų žinomas skaičius pi, kuris apibrėžiamas kaip apskritimo ilgio ir jo skersmens santykis taip pat pasirodė esąs iracionalusis skaičius, tai 1767 m. įrodė Johanas Lambertas. Kiekvienas atrastas iracionalumas kūrė spragą arba tarpą racionaliųjų skaičių aibėje. Realiųjų skaičių aibės įvedimas šiuos tarpus užpildo. Pirmoji sėkminga realiųjų skaičių konstrukcija laikoma Eudokso proporcijų teorija, aprašyta Euklido Pradmenyse. Nors pati pirmoji formalų realiųjų skaičių formuluotė buvo pasiūlyta vokiečių matematiko Ričardo Dedekindo, naudojant racionaliuosius skaičius ir Dedekindo pjūvį, aksiominį iracionaliųjų skaičių apibrėžimo metodą.[1]

Savybės

Realiųjų skaičių savybės:[2]

  • (sumos perstatymo dėsnis)
  • (sumos jungimo dėsnis)
  • (daugybos perstatymo dėsnis)
  • (daugybos jungimo dėsnis)
  • (daugybos skirstymo dėsnis)
  • su bet kokiu skaičiumi teisingos lygybės

Šaltiniai

  1. ↑ Dedekindo pjūvis. Visuotinė lietuvių enciklopedija (tikrinta 2023-02-25).
  2. ↑ Autorių kolektyvas. Matematika 11. I dalis. – Vilnius: TEV, 2002. – 29 p. ISBN 9955-491-22-1


Vikižodynas

Read other articles:

FIRS Inline Hockey World Championships

Annual roller inline hockey tournament Not to be confused with IIHF Inline Hockey World Championship. This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: FIRS Inline Hockey World Championships – news · newspapers · books · scholar · JSTOR (January 2017) (Learn how and when to remove this template message) FIRS Inline Hockey World ChampionshipsCurrent season...

 

Susano Oh

Japanese manga series This article is about the manga of Go Nagai. For the Shinto god, see Susanoo. This article includes a list of general references, but it lacks sufficient corresponding inline citations. Please help to improve this article by introducing more precise citations. (March 2020) (Learn how and when to remove this template message) Susano OhCover of Susano Oh #2 (2000 edition)凄ノ王(Susano Ō) MangaWritten byGo NagaiPublished byKodanshaMagazineWeekly Shōnen Magazi...

 

Administrative divisions of the Sasanian Empire

The administrative divisions of the Sasanian Empire were administrative divisions of the state organisation of the Sasanian Empire. Administration Coin of Ardashir I, the founder of the Sasanian Empire On his coinage, the first Sasanian King of Kings (shahanshah) Ardashir I (r. 224–242), introduces himself as King of Kings of a realm that he identified as Eran. His son and successor Shapur I (r. 240–270) calls himself King of Kings of Eran and Aneran in his SKZ inscription. Ac...

Raymond Massey

Raymond Massey nel trailer del film Uragano (1937) Raymond Massey (Toronto, 30 agosto 1896 – Los Angeles, 29 luglio 1983) è stato un attore canadese. Indice 1 Biografia 1.1 La prima guerra 1.2 Il cinema 1.3 Vita privata 2 Filmografia 2.1 Cinema 2.2 Televisione 3 Doppiatori italiani 4 Riconoscimenti 5 Altri progetti 6 Collegamenti esterni Biografia Nato a Toronto (Ontario), figlio di Chester D. Massey, ricco proprietario della Massey-Ferguson Tractor Company, studiò all'Appleby College di ...

 

Template talk:Catholic Church sidebar

Please place new discussions at the bottom of the talk page. This is the talk page for discussing improvements to the Catholic Church sidebar template. Put new text under old text. Click here to start a new topic. New to Wikipedia? Welcome! Learn to edit; get help. Assume good faith Be polite and avoid personal attacks Be welcoming to newcomers Seek dispute resolution if needed Archives: 1Auto-archiving period: 60 days  The subject of this article is controversial and content may be in disp...

 

Psilophyta

Come leggere il tassoboxPsilophyta Psilotum nudum Classificazione scientifica Dominio Eukaryota Regno Plantae Sottoregno Viridiplantae Clade Embryophyta Superdivisione Tracheobionta Divisione PsilophytaHeintze, 1927 Classe PsilopsidaD.H.Scott, 1909 Ordine PsilotalesEngl., 1892 Famiglia PsilotaceaeKanitz, 1887 Generi Psilotum Tmesipteris Le Psilofite (Psilophyta Heintze, 1927) sono una divisione di piante vascolari che comprende le più semplici piante vascolari viventi. Il loro nome deriva d...

Escapade in Florence

2nd and 3rd episodes of the 9th season of The Magical World of Disney Escapade in FlorenceThe Magical World of Disney episodesEpisode nos.Season 9Episodes 2-3Directed bySteve PrevinWritten by Maurice Tombragel Bob Wehling Based onThe Golden Doorsby Edward FentonProduced byBill AndersonFeatured musicBuddy BakerCinematography byKurt GrigoleitOriginal air date1962 (1962)Running time81 minutes Escapade in Florence is a two-part episode of the The Magical World of Disney television show...

 

コルク

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。（このテンプレートの使い方）出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL（2017年4月） コルクを打ち抜いて作った瓶の栓 コルク（木栓、�...

 

2020–21 Scottish Championship

Football league seasonScottish ChampionshipSeason2020–21Dates16 October 2020 – 30 April 2021ChampionsHeart of MidlothianPromotedHeart of MidlothianDundeeRelegatedAlloa AthleticMatches played135Goals scored380 (2.81 per match)Top goalscorerLiam Boyce (14 goals)[1]Biggest home winHeart of Midlothian 6–0 Alloa Athletic (9 April 2021)Biggest away winRaith Rovers 0–4 Heart of Midlothian (26 January 2021)Highest scoringHeart of Midlothian 6–2 Dundee (16 October 2020)Heart of...

Warrior (album Kesha)

WarriorAlbum studio karya KeshaDirilis30 November 2012 (2012-11-30)DirekamJanuari–Agustus 2012Genre Electropop[1] rock[2] Durasi44:2758:43 (Edisi Deluxe)Label Kemosabe RCA Produser Ammo Benny Blanco Billboard Cirkut Dr. Luke Greg Kurstin Kool Kojak Matt Squire Max Martin Shellback Stardeath and White Dwarfs Steven Wolf The Flaming Lips Kronologi Kesha I Am the Dance Commander + I Command You to Dance: The Remix Album(2011) Warrior(2012) Deconstructed(2012) Singel da...

 

Chinese cooking techniques

Chinese stir-frying cooking technique at a street food joint in Kerala, India. Chinese cooking techniques (Chinese: 中餐烹調法) are a set of methods and techniques traditionally used in Chinese cuisine.[1][page needed] The cooking techniques can either be grouped into ones that use a single cooking method or a combination of wet and dry cooking methods. Part of a series onChinese cuisine Regional cuisines Four Great Traditions Chuan (Sichuan) Lu (Shandong) Yue (G...

 

York Park

Sports stadium in Tasmania University of Tasmania StadiumFormer namesAurora StadiumLocationLaunceston, TasmaniaCoordinates41°25′33″S 147°08′20″E / 41.42583°S 147.13889°E / -41.42583; 147.13889OwnerLaunceston City CouncilOperatorAFL TasmaniaCapacity19,000[2]Field size170 m × 140 m (560 ft × 460 ft)[1]SurfaceGrassConstructionBroke ground1919Opened1921Construction costA$23.6M (redevelopment)TenantsTasmania Devils...

Hergé's Adventures of Tintin

Animated television series Not to be confused with The Adventures of Tintin (TV series) or The Adventures of Tintin. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Hergé's Adventures of Tintin – news · newspapers · books · scholar · JSTOR (January 2016) (Learn how and when to remove this message) Hergé's ...

 

Cycling at the 2024 Summer Olympics – Men's team sprint

Men's team sprintat the Games of the XXXIII OlympiadOlympic track cyclingVenuesVélodrome National de Saint-Quentin-en-YvelinesDates5–6 August 2024Competitors25 from 8 nationsTeams8Winning time40.949Medalists Jeffrey HooglandHarrie LavreysenRoy van den Berg  Netherlands Jack CarlinEd LoweHamish Turnbull  Great Britain Matthew GlaetzerLeigh HoffmanMatthew Richardson  Australia← 20202028 → Cycling at the2024 Summer OlympicsList of cyclistsQualif...

 

2012年のアメリカンリーグワイルドカードゲーム

2012年のアメリカンリーグワイルドカードゲーム チーム スコア ボルチモア・オリオールズ 5 テキサス・レンジャーズ 1 試合情報 開催日 10月5日（金） 開催球場 レンジャーズ・ボールパーク・イン・アーリントン 観客動員 4万6931人 責任審判 ゲイリー・ダーリング[1] 殿堂表彰者 ジム・トーミ（BAL内野手）エイドリアン・ベルトレ（TEX内野手） チーム情報 ボルチ�...

Die Olsenbande stellt die Weichen

Film Titel Die Olsenbande stellt die Weichen Originaltitel Olsen-banden på sporet Produktionsland Dänemark Originalsprache Dänisch Erscheinungsjahr 1975 Länge 105 Minuten Altersfreigabe FSK 12 Stab Regie Erik Balling Drehbuch Henning Bahs,Erik Balling Produktion Bo Christensen Musik Bent Fabricius-Bjerre Kamera Claus Loof Schnitt Ole Steen Nielsen Besetzung Ove Sprogøe: Egon Olsen Morten Grunwald: Benny Frandsen Poul Bundgaard: Kjeld Jensen Kirsten Walther: Yvonne Jensen Jes Holts�...

 

庭木

日本の栗林公園の庭木の様子 庭木（にわき）とは、庭に植える樹木の総称である。庭木はまた、「彫刻の木」を表す言葉でもある[1]。 概要 庭木は植えるときもさることながら、その後の水やり、雑草抜き、剪定、移植などを通して人との関わりが見て取れる貴重な緑である。他にもクリスマスなどの時期になると庭木にイルミネーションを設置する住人もいる�...

 

Elliptic curve

Algebraic curve Not to be confused with Ellipse. This article is about the mathematical curve. For the cryptography technique, see Elliptic-curve cryptography. Elliptic Equation redirects here. For the type of partial differential equation, see Elliptic partial differential equation. A catalog of elliptic curves. The region shown is x, y ∈ [−3,3].(For (a, b) = (0, 0) the function is not smooth and therefore not an elliptic curve.) Algebraic structure → Group theoryGroup theory Basic not...

Szymon Marcin Kozłowski

Szymon Marcin KozłowskiFonctionsArchevêque de MoguilevArchidiocèse de Mohilev14 décembre 1891 - 26 novembre 1899Aleksander Gintowt-Dziewałtowski (d)Bolesław Hieronim Kłopotowski (d)Évêque de LoutskDiocèse de Loutsk15 mars 1883 - 14 décembre 1891Kaspar Borowski (d)Cyril Lubowidzki (en)BiographieNaissance 5 novembre 1819AlytusDécès 14 novembre 1899 (à 80 ans)Saint-PétersbourgSépulture Église de la VisitationNationalité russeDomicile Saint-PétersbourgFormation Vilnius St....

 

Kaeson Youth Park

Amusement park in Pyongyang, North Korea Kaeson Youth ParkLocationNorth KoreaCoordinates39°2′52″N 125°45′29″E / 39.04778°N 125.75806°E / 39.04778; 125.75806Opened1984[1]Operating seasonYear-roundArea40 ha[1]AttractionsRoller coasters1 The Kaeson Youth Park (Korean: 개선청년공원) is an amusement park located in Pyongyang, North Korea. The park, located near the Kim Il Sung Stadium and in the west foot of Moran Hill was opened in 1984, t...