南京放送テレビ局

曖昧さ回避 「江蘇省放送テレビ総局」とは異なります。
南京広播電視台
南京广播电视台
Nanjing Broadcasting System
種類 国営企業
略称 NBC/NJTV
本社所在地 中華人民共和国の旗 中国
江蘇省南京市竜蟠中路338号
設立 2003年9月29日
業種 情報・通信業
事業内容 放送業
外部リンク 公式サイト
テンプレートを表示
南京放送テレビ局
各種表記
繁体字： 南京廣播電視臺
簡体字： 南京广播电视台
英文： Nanjing Broadcasting System (NBS)
テンプレートを表示

南京放送テレビ局[1]、南京テレビ局[2]、あるいは南京広播電視台[3]（簡体字中国語: 南京广播电视台）は、中華人民共和国江蘇省南京市の国営の公共放送テレビ局。

沿革

1980年1月17日、南京電視台が発足。

1981年2月4日、仮放送が開始。

2003年9月29日、南京電視台、南京人民広播電台、南京広播電視報社、南京音像出版社が統合され、南京放送テレビ局が発足。

本社所在地

チャンネル

テレビ

  • 新聞総合チャンネル
  • 影視チャンネル
  • 教科チャンネル
  • 文旅紀録チャンネル
  • 十八・生活チャンネル
  • 少児チャンネル

ラジオ

  • 新聞総合チャンネル
  • 城市管理チャンネル
  • 交通チャンネル
  • 経済チャンネル
  • 音楽チャンネル
  • 体育チャンネル
  • Cool Radio

脚注

[脚注の使い方]
  1. ^ “周 業欣 (YEXIN ZHOU) - マイポータル”. researchmap. 国立研究開発法人科学技術振興機構. 2024年12月4日閲覧。
  2. ^ “周業欣「中国伝統的な手漉き紙シリーズ」リニモテラス”. 長久手アートフェスティバル2021. 2024年12月4日閲覧。
  3. ^ “オオアラセイトウを植えて平和の花壇作るイベント　南京市”. 人民網日本語版. 人民日報社 (2018年11月19日). 2024年12月4日閲覧。
  4. ^ http://www.nbs.cn

関連項目

外部リンク

ウィキメディア・コモンズには、南京放送テレビ局に関連するカテゴリがあります。
全国放送
市・省級
  • 北京
  • 天津
  • 河北
  • 山西
  • 内モンゴル
  • 遼寧
  • 吉林
  • 黒竜江
  • 上海
  • 江蘇
  • 浙江
  • 安徽
  • 福建
  • 江西
  • 山東
  • 河南
  • 湖北
  • 湖南
  • 広東
  • 広西
  • 海南
  • 重慶
  • 四川
  • 貴州
  • 雲南
  • チベット
  • 陝西
  • 甘粛
  • 青海
  • 寧夏
  • 新疆
副省級
  • 石家荘
  • 太原
  • フフホト
  • 瀋陽
  • 大連
  • 長春
  • 延辺
  • ハルビン
  • 南京
  • 杭州
  • 寧波
  • 合肥
  • 福州
  • 廈門
  • 南昌
  • 済南
  • 青島
  • 鄭州
  • 武漢
  • 長沙
  • 広州
  • 深圳
  • 南寧
  • 海口
  • 成都
  • 貴陽
  • 昆明
  • ラサ
  • 西安
  • 蘭州
  • 西寧
  • 銀川
  • ウルムチ
省級衛星
  • 北京衛視
  • 天津衛視
  • 河北衛視
  • 山西衛視
  • 内モンゴル衛視
  • 遼寧衛視
  • 吉林衛視
  • 延辺衛視
  • 黒竜江衛視
  • 東方衛視
  • 江蘇衛視
  • 浙江衛視
  • 安徽衛視
  • 東南衛視
  • 海峡衛視
  • 江西衛視
  • 山東衛視
  • 山東教育電視台
  • 河南衛視
  • 湖北衛視
  • 湖南衛視
  • 広東衛視
  • 広東南方衛視
  • 広西衛視
  • 海南衛視
  • 重慶衛視
  • 四川衛視
  • 四川カムバチベット語衛視
  • 貴州衛視
  • 雲南衛視
  • チベット衛視
  • 陝西衛視
  • 農林衛視
  • 甘粛衛視
  • 青海衛視
  • アムド衛視
  • 寧夏衛視
  • 新疆衛視
  • 兵団衛視

香港・マカオ・台湾は除外。
「衛視」は、「衛星放送」を意味する。

スタブアイコン

この項目は、放送局に関連した書きかけの項目です。この項目を加筆・訂正などしてくださる協力者を求めています（プロジェクト:放送局／Portal:メディア）。

スタブアイコン

この項目は、中国に関連した書きかけの項目です。この項目を加筆・訂正などしてくださる協力者を求めています（P:中国/PJ:中国）。

Read other articles:

Deicing boot

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

 

 

Gempa bumi Vrancea 1977

Gempa bumi Vrancea 1977Hotel hancur di Bukares, RumaniaTampilkan peta EropaTampilkan peta RumaniaWaktu UTC1977-03-04 19:21:57ISC700695USGS-ANSSComCatTanggal setempat4 Maret 1977 (1977-03-04)Waktu setempat21:21:57Lama55 detikKekuatan7.5 Mw[1]Kedalaman94 km (58 mi)[2]Episentrum45°46′N 26°46′E / 45.77°N 26.76°E / 45.77; 26.76Koordinat: 45°46′N 26°46′E / 45.77°N 26.76°E / 45.77; 26.76Wila...

 

 

Artyom Zhmakin

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Artyom ZhmakinInformasi pribadiNama lengkap Artyom Ivanovich ZhmakinTanggal lahir 10 September 1995 (umur 28)Tinggi 1,74 m (5 ft 8+1⁄2 in)Posisi bermain GelandangInformasi klubKlub saat ini FC Avangard KurskKarier senior*Tahu...

United Overseas Bank

United Overseas Bank LimitedKantor pusat di UOB PlazaJenisPublikKode emitenSGX: U11IndustriJasa keuanganDidirikan6 Agustus 1935; 88 tahun lalu (1935-08-06)PendiriWee Kheng ChiangKantorpusatUOB Plaza, 80 Raffles Place, Singapore 048624Wilayah operasiAsia Tenggara, TiongkokTokohkunci Wong Kan Seng (Ketua) Wee Ee Cheong (wakil ketua & CEO) PendapatanS$8.061 miliar (2016)[1]Laba bersihS$3.096 miliar (2016)[1]Total asetUS$334.09 miliar (2021)[2]Karya...

 

 

Kuremaa

Borough in Estonia Place in Jõgeva County, EstoniaKuremaaKuremaa manor houseKuremaaCoordinates: 58°44′12″N 26°31′21″E / 58.73667°N 26.52250°E / 58.73667; 26.52250CountryEstoniaCountyJõgeva CountyMunicipalityJõgeva ParishPopulation (2020)[1] • Total280Time zoneUTC+2 (EET) Kuremaa (German: Jensel) is a small borough (alevik) in Jõgeva Parish, Jõgeva County in eastern Estonia. It lies 10 km from Jõgeva, near the northern sho...

 

 

Jimmy Santiago Baca

American poet and educator (born 1952) Jimmy Santiago BacaBaca during the videotaping of Add-Verse, 2004Born (1952-01-02) January 2, 1952 (age 72)Santa Fe, New Mexico, U.S.OccupationPoetNotable worksMartin and Meditations on the South ValleyNotable awardsAmerican Book Award, Pushcart Prize, International Hispanic Heritage Award, International Award.SpouseMarriedWebsitewww.jimmysantiagobaca.com Jimmy Santiago Baca (born January 2, 1952) is an American poet, memoirist, and screenwriter fro...

Saripidem

Chemical compound SaripidemClinical dataATC codenoneIdentifiers IUPAC name N-[[2-(4-chlorophenyl)imidazo[1,2-a]pyridin-3-yl]methyl]-N-methylbutanamide CAS Number103844-86-6 NPubChem CID3058746ChemSpider2319846 YUNII0J6174G60NChEMBLChEMBL73416 YCompTox Dashboard (EPA)DTXSID60146110 Chemical and physical dataFormulaC19H20ClN3OMolar mass341.84 g·mol−13D model (JSmol)Interactive image SMILES O=C(N(C)Cc1c(nc2ccccn12)c3ccc(Cl)cc3)CCC InChI InChI=1S/C19H20ClN3O/c1-3-6-18(24)22...

 

 

Catholic Church in England and Wales

Overview of the role of the Catholic Church in England and Wales Not to be confused with Catholic Church in the United Kingdom. Catholic Church in England and WalesWestminster Cathedral, the mother church for Catholics in EnglandClassificationCatholicOrientationLatinScriptureBibleTheologyCatholic theologyPolityEpiscopalGovernanceCBCEWPopeFrancisPresidentVincent Cardinal NicholsApostolic NuncioMiguel Maury BuendíaRegionEngland and WalesLanguageEnglish, Welsh, LatinHeadquartersLondon, EnglandF...

 

 

The Racer's Group

Racing team This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: The Racer's Group – news · newspapers · books · scholar · JSTOR (November 2009) (Learn how and when to remove this message) TRGOwner(s)Kevin BucklerBasePetaluma, CaliforniaSeriesTUDOR United SportsCar ChampionshipGT4 Challenge of North AmericaPirelli World Challenge ChampionshipManufacturerAsto...

Caroline Lamb

Cet article est une ébauche concernant une écrivaine britannique. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Pour les articles homonymes, voir Lamb. Caroline LambPortrait de Caroline Lamb vers 1805.BiographieNaissance 13 novembre 1785DorsetDécès 26 janvier 1828 (à 42 ans)LondresSépulture HertfordshireNationalité britanniqueActivités Poétesse, écrivaine, romancièrePère Frederick PonsonbyMèr...

 

 

Takalar pada Pekan Olahraga Provinsi Sulawesi Selatan 2022

Takalar pada Pekan Olahraga Provinsi Sulawesi Selatan 2022 Jumlah atlet TBD di TBD cabang olahraga Pembawa bendera TBD Total medali Emas4 Perak8 Perunggu7 19 (Urutan ke-19 ) Kontingen Takalar berkompetisi pada Pekan Olahraga Provinsi Sulawesi Selatan 2022 di Sinjai dan Bulukumba, Sulawesi Selatan pada 22 sampai 30 Oktober 2022. Kontingen ini menempati posisi ke-19 pada tabel klasemen perolehan medali Porprov Sulsel XVII/2022 setelah meraih total medali 19 dengan rincian 4 medali emas, 8...

 

 

غزل (شعر)

هذه المقالة بحاجة لصندوق معلومات. فضلًا ساعد في تحسين هذه المقالة بإضافة صندوق معلومات مخصص إليها.   لمعانٍ أخرى، طالع غزل (توضيح). جزء من سلسلة مقالات عنالثقافة العربية العمارة الأنماط يمني قديم نبطية أموية عباسية فاطمية مغربية مملوكية المميزات حجر أبلق بهو معمد مشرب...

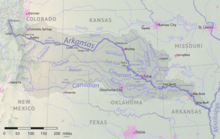
Arkansas River

Major tributary of the Mississippi River, United States Arkansas RiverArkansas River headwaters in ColoradoThe Arkansas River flows through Colorado, Kansas, Oklahoma, and Arkansas, and its watershed also drains parts of Texas, New Mexico and Missouri.LocationCountryUnited StatesStateColorado, Kansas, Oklahoma, ArkansasRegionGreat PlainsCitiesPueblo, CO, Wichita, KS, Tulsa, OK, Muskogee, OK, Fort Smith, AR, Little Rock, AR, Pine Bluff, ARPhysical characteristicsSourceConfluence of East F...

 

 

Państwowe Zjednoczenie na Kresach

Politics of Poland Government Constitution of Poland Law Human rights Legislature Parliament of Poland Current Parliament Sejm Marshal Szymon Hołownia (PL2050) Deputy Marshals Senate Marshal Małgorzata Maria Kidawa- Błońska (PO) Deputy Marshals Executive President of Poland Andrzej Duda (I) Prime Minister of Poland Donald Tusk (PO) Cabinet Ministries Judiciary Supreme Court of Poland First President Małgorzata Manowska Constitutional Tribunal President Julia Przyłębska Supreme Administ...

 

 

List of Danish monarchs

Royal Banner of the Kings of Denmark (circa 1300s). Politics of Denmark Constitution Fundamental laws Act of Succession Freedom of Speech Freedom of the Press The Crown Monarch Frederik X Crown Prince Christian Royal family Privy Council Purveyors to the Royal Court The unity of the Realm Denmark proper Faroe Islands Greenland Executive Government Frederiksen II Prime Minister (list) Mette Frederiksen Government Offices Ministries High Commissioners Faroe Islands Greenland Legislature Folket...

Water polo at the 2024 Summer Olympics – Women's qualification

This section tabulates the heads of qualification in a form suitable to be filled in as events progress. The full qualification rules[1] for water polo published by AQUA contain intricate conditions too lengthy for inclusion in Wikipedia. Water polo at the2024 Summer OlympicsQualificationmenwomenTournamentmenwomenRostersmenwomenvte The women's qualification for the Olympic water polo tournament occurred between July 2023 and February 2024, allocating ten teams for the final tournamen...

 

 

Postal codes in Vietnam

Postal codes in Vietnam have five digits.[1] The exact postal code designated for local government areas, local post offices, government offices or embassies and consulates can be searched on National Postal Code Website.Regional and provincial postal codes of Viet Nam Structure The postal code system of Vietnam has officially been changed from 6 digits to 5 digits. Each country has its own separate postal code or zip code system. The postal code of Vietnam is composed of 5 digits, w...

 

 

Alessandra Ferri

Michail Baryšnikov e Alessandra Ferri Alessandra Maria Ferri (Milano, 6 maggio 1963) è una ballerina italiana. Alessandra Ferri è stata prima ballerina del Royal Ballet (1983-1985) e dell'American Ballet Theatre (1985-2007) e prima ballerina assoluta del Teatro alla Scala.[1] Nel corso della sua lunga carriera ha vinto due Premi Laurence Olivier, un Prix Benois de la Danse, ha ballato come ospite con molte delle maggiori compagnie al mondo e ha danzato accanto ad alcuni dei maggior...

Gauss's law

Foundational law of electromagnetism relating electric field and charge distributions This article is about Gauss's law concerning the electric field. For analogous laws concerning different fields, see Gauss's law for magnetism and Gauss's law for gravity. For the Ostrogradsky–Gauss theorem, a mathematical theorem relevant to all of these laws, see Divergence theorem. Not to be confused with Gause's law. Gauss's law in its integral form is particularly useful when, by symmetry reasons, a c...

 

 

K-vertex-connected graph

Graph which remains connected when k or fewer nodes removed A graph with connectivity 4. In graph theory, a connected graph G is said to be k-vertex-connected (or k-connected) if it has more than k vertices and remains connected whenever fewer than k vertices are removed. The vertex-connectivity, or just connectivity, of a graph is the largest k for which the graph is k-vertex-connected. Definitions A graph (other than a complete graph) has connectivity k if k is the size of the smallest subs...