Differenciális csigasor

A differenciális csigasor vagy differenciál-csigasor az álló- és mozgócsigákból álló csigasorok azon változata, amely két különböző átmérőjű, egymáshoz erősített állócsigából, egy különálló mozgócsigából, valamint az ezeken végigfutó egyetlen, végtelenített láncból áll.

Működése

Differenciális csigasor működésének szemléltető ábrája

A mellékelt ábra szemlélteti a differenciális csigasor működési elvét. A felső állású, rögzített és együtt futó állócsigák peremén a visszacsúszást megakadályozó fogazatot képeznek ki, ebbe illeszkedik a lánc. A terhet a mozgócsigához erősítik, s az ennek – gyakran szintén fogazott – peremén is végigvezetett lánc teremti meg a mechanikai kapcsolatot az állócsigákkal. A nagyobb sugarú, rögzített állócsigáról szabadon lógó láncág (1) húzásával párhuzamosan a láncnak a csiga túloldaláról lelógó szakasza (4) megemeli a terhet. Ugyanakkor a kisebbik állócsigára felcsévélődő láncrész (3) visszaereszti a kis állócsiga és a mozgócsiga közötti, terhelt láncágat (2). Tekintve azonban, hogy e láncág (2) süllyedésének mértékét a kisebbik állócsiga sugara határozza meg, az elmarad a nagy sugarú állócsiga által kifejtett emelőhatástól (4), így a teher lassan emelkedik.

A teher megemeléséhez kifejtett kézi erő mértékét a két állócsiga sugarának aránya szabja meg, a következő képlet alapján:


Másképpen:


Más szóval minél nagyobb mértékben tér el egymástól a nagyobbik (R) és a kisebbik állócsiga (r) sugara, annál kisebb erőt kell kifejteni a teher felemeléséhez. Ugyanakkor a kifejtett erővel fordítottan arányos a megtett út hossza. Azaz ha egy 100 newtonos tömeg (FL) megmozdításához 25 newton erőt (FZ) fejtünk ki, a teher 10 centiméteres magasságba (h) emeléséhez 40 centiméter (s) utat kell megtennünk, azaz ennyit kell húznunk a láncon. Az állócsigák egymáshoz közelítő sugármérete esetén tehát az erőkifejtés kicsi, ám az út hosszabb lesz, a munkavégzés tovább tart, hiszen a kisebbik állócsigáról függő láncág (2) süllyedésének mértéke megközelíti a terhet emelő láncrész (4) emelkedését. Az emelőmagasság gyakorlatilag nem lehet hosszabb a lánc egy ágánál, azaz a lánchossz negyedrészénél.

A differenciális csigasor gyakorlati alkalmazási lehetőségei napjainkban szűkre szabottak, hiszen csak kézi erővel – erőátvitel segítségével – mozgatható terhek kis magasságra történő emelésére használható.

Története

A differenciál-csigasor egy angol feltaláló, Thomas A. Weston nevéhez fűződik, akinek szabadalma révén 1859-ben Birminghamben indult meg a Weston-féle differenciál-csigasorok (Weston’s differential pulley blocks) gyártása. Az 1862-es londoni világkiállításon is sikerrel szerepelt, s a 19. században nagy népszerűségnek örvendett a találmány. Széles körben alkalmazták bányákban, üzemekben, áruraktárakban, vasúti közlekedésben, vágóhídakon stb., de a 20. század korszerűbb, a kézi erőkifejtést motoros hajtással kiváltó emelőgépei jószerével kiszorították a használatból. Weston találmánya azonban nem volt előzmény nélküli. Hasonló elven működik a hagyományos kínai csörlő és bányacsörlő is (ábrázolásuk), amely két, egymással párhuzamosan futó, eltérő átmérőjű kötéldobra csévéli fel a terhet húzó vagy emelő, horogban végződő kötél egy-egy szárát.

Források

  • Erik Oberg et al., Machinery's handbook, New York, Industrial Press, 1959, 163.
  • Samuel Timmins, Birmingham and the Midland Hardware District, London, Cass, 1967, 648.
  • Magyar nagylexikon VI. (Csen–Ec). Főszerk. Berényi Gábor. Budapest: Magyar Nagylexikon. 1998. 576. o. ISBN 963-85773-2-0  

Read other articles:

Twisted Souls

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Twisted Souls – news · newspapers · books · scholar · JSTOR (September 2019) (Learn how and when to remove this template message) For the clothing brand, see Twisted Soul. Twisted Souls AuthorShaun HutsonCountryUnited KingdomLanguageEnglishGenreHorror novelPublisherTime Warner ...

 

 

Karl von Piloty

Karl von Piloty; foto karya Franz Hanfstaengl Karl Theodor von Piloty (1 Oktober 1826 – 21 Juli 1886) adalah seorang pelukis asal Jerman.[1] Kehidupan dan karya Von Piloty lahir di Munich. Ayahnya, Ferdinand Piloty (wafat 1844), dikenal sebagai litografer. Pada 1840, Karl menjadi murid Akademi Munich, di bawah naungan seniman Karl Schorn dan Julius Schnorr von Karolsfeld. Galeri Henry VIII dan Anne Boleyn Kematian Wallenstein. Thusnelda diPawai Kemenangan Germanicus. K...

 

 

Hadis Jibril

Hadis Jibril (Arab: حديث جبرائيلcode: ar is deprecated , Hadīts Jibraīl) adalah sebuah hadis yang memuat definisi tentang Islam, Iman, Ihsan, dan tanda-tanda hari kiamat menurut akidah umat Islam. Hadis ini diriwayatkan dari sahabat Umar bin Al-Khaththab dan Abu Hurairah. Hadis ini dapat ditemukan di kedua kitab Shahihain, Sahih Bukhari dan Sahih Muslim, juga Arbain Nawawi hadits ke-2. Matan & sanad Matan Dari Umar bin Khattab berkata:[1] “Suatu ketika, kami (para s...

Pilatus PC-9

Pilatus PC-9 adalah sebuah pesawat latih bermesin turboprop satu, sayap rendah (low wing) kursi tandem yang diproduksi oleh Pilatus Aircraft Swiss. PC-9 merupakan evolusi yang lebih kuat dari PC-7. Pesawat bermesin turboprop ini mempertahankan tata letak keseluruhan dari pendahulunya, tetapi memiliki sangat sedikit kesamaan struktural dengan itu. Di antara perbaikan lain, PC-9 memiliki kokpit lebih besar dengan kursi ejeksi melangkah dan juga memiliki Airbrake ventral. Referensi Harding, Ste...

 

 

List of acts of the Parliament of the United Kingdom from 1955

Part of a series onBritish law Acts of Parliament of the United Kingdom Year      1801 1802 1803 1804 1805 1806 1807 1808 1809 1810 1811 1812 1813 1814 1815 1816 1817 1818 1819 1820 1821 1822 1823 1824 1825 1826 1827 1828 1829 1830 1831 1832 1833 1834 1835 1836 1837 1838 1839 1840 1841 1842 1843 1844 1845 1846 1847 1848 1849 1850 1851 1852 1853 1854 1855 1856 1857 1858 1859 1860 1861 1862 1863 1864 1865 1866 1867 1868 1869 1870 1871 1872 1873 1874 1875 1876 1877 1878 ...

 

 

Emperor Wen of Chen

Emperor of the Chen dynasty Emperor Wen of Chen陳文帝Tang dynasty portrait of Emperor Wen by Yan LibenEmperor of the Chen dynastyReign559–566PredecessorEmperor WuSuccessorEmperor FeiBorn522Died566 (aged 43–44)BurialYongning Mausoleum (永寧陵, in present-day Qixia District, Nanjing)ConsortsEmpress Ande Han ZigaoIssueChen BozongChen BomaoChen BoshanChen BoguChen BogongChen BoxinChen BorenChen BoyiChen BoliChen BozhiChen BomouPrincess Feng'anPrincess FuyangNamesFamily name: Ch...

Full Circle (magazine)

Pour les articles homonymes, voir Full Circle. Cet article est une ébauche concernant l’informatique et Internet. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Logo du Full Circle Magazine Le Full Circle Magazine est un magazine en ligne anglophone amateur gratuit ayant pour sujet la distribution GNU/Linux Ubuntu et ses dérivés Kubuntu, Xubuntu et Edubuntu. Présentation Le magazine se définit lui-même ...

 

 

Cartulario (registro)

Volumen e Rotulus Un cartulario, cartolario o cartario, [dal latino medievale chartularius, cartularium o chartularium, derivazione del latino chartŭla, diminutivo di charta « carta »], è un manoscritto medievale, in volume o più raramente in rotolo (rotulus), contenente la trascrizione di documenti originali relativi alla fondazione, privilegi e diritti legali di istituzioni ecclesiastiche, corporazioni municipali, associazioni industriali, istituzioni di insegnamento, o famig...

 

 

Taiwan Open 2017

Taiwan Open 2017 Sport Tennis Data 30 gennaio – 5 febbraio Edizione 2ª Categoria International Superficie Cemento indoor Montepremi 250 000$ Impianto Taipei Arena Campioni Singolare Elina Svitolina Doppio Chan Hao-ching / Latisha Chan 2016 2018 Il Taiwan Open 2017 è stato un torneo di tennis giocato sui campi in cemento indoor. È stata la seconda edizione del Taiwan Open, che fa parte della categoria International nell'ambito del WTA Tour 2017. Si è giocato alla Taipei Arena di Ta...

The Hangover Part II

The Hangover Part IITheatrical release posterSutradaraTodd PhillipsProduserDaniel GoldbergTodd PhillipsDitulis olehScot ArmstrongCraig MazinTodd PhillipsPemeranBradley CooperEd HelmsZach GalifianakisJustin BarthaPenata musikChristophe BeckSinematograferLawrence SherPenyuntingDebra Neil-FisherMike SalePerusahaanproduksiLegendary PicturesGreen Hat FilmsDistributorWarner Bros. PicturesTanggal rilis 26 Mei 2011 (2011-05-26) Durasi102 menitNegaraAmerika SerikatBahasaInggrisAnggaran$80.0...

 

 

Frank Cady

American actor (1915–2012) This article is about the actor. For the Wisconsin legislator, see Frank A. Cady. Frank CadyCady in The Great Rupert (1950)BornFrank Randolph Cady(1915-09-08)September 8, 1915Susanville, California, U.S.DiedJune 8, 2012(2012-06-08) (aged 96)Wilsonville, Oregon, U.S.Resting placeLos Osos Valley Memorial Park, Los Osos, California[1]Other namesFrankie CadyFrank R. CadyAlma materStanford UniversityOccupationActorYears active1947–1990...

 

 

The Chang'an Youth

Chinese television program Some of this article's listed sources may not be reliable. Please help improve this article by looking for better, more reliable sources. Unreliable citations may be challenged and removed. (November 2021) (Learn how and when to remove this message) The Chang'an YouthAlso known asThe Chang An Youth[1]Traditional Chinese長安少年行Simplified Chinese长安少年行Hanyu PinyinCháng'ān Shàonián Xíng GenreHistorical fictionCostumeRom-ComYouthSchool...

Бєлінський Віссаріон Григорович

Бєлінський Віссаріон ГригоровичБелинский Виссарион Григорьевич Ім'я при народженні Бѣлинскій Виссаріон ГригорьевичъНародився 30 травня (11 червня) 1811[1][2][…]Свеаборг, Велике князівство Фінляндське, Російська імперія[4][1][…]Помер 26 травня (7 червня) 1848[1]...

 

 

Mike Read

English radio presenter and musician For the swimmer, see Michael Read. For those of a similar name, see Michael Reed (disambiguation) and Michael Reid (disambiguation). Mike ReadRead in 2013BornMichael David Kenneth Read (1947-03-01) 1 March 1947 (age 77)Bury, Lancashire, EnglandNationalityBritishOccupationsRadio DJwriterjournalisttelevision presenterYears active1976 – presentKnown forSaturday Superstore; BBC Radio 1; Top of the Pops; Pop Quiz Michael David Kenneth Read (bor...

 

 

Charles-Adolphe Wurtz

Questa voce sull'argomento chimici francesi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Charles-Adolphe Wurtz Charles-Adolphe Wurtz (Wolfisheim, 26 novembre 1817 – Parigi, 10 maggio 1884) è stato un chimico francese. Indice 1 Biografia 2 Opere 3 Note 4 Altri progetti 5 Collegamenti esterni Biografia È ricordato soprattutto per la sua pluridecennale difesa della teoria atomica e per le sue idee sulle strutture dei composti chimici, che andavano...

Chief Justice of Belize

Head of the Supreme Court of Belize Chief Justice of BelizeIncumbentLouise Blenmansince 2 September 2022Supreme Court of BelizeOffice of the Chief JusticeStyleThe Honourable Madam Chief JusticeStatusChief Justice, head of the Supreme Court of BelizeMember ofSupreme Court of BelizeSeatSupreme Court Building,Belize City,Belize,Central AmericaNominatorPrime MinisterAppointerGovernor GeneralTerm lengthNo set term, though retirement is mandatory at age 65.Constituting instrumentConstitution o...

 

 

Tarentaise, Loire

Commune in Auvergne-Rhône-Alpes, FranceTarentaiseCommune Coat of armsLocation of Tarentaise TarentaiseShow map of FranceTarentaiseShow map of Auvergne-Rhône-AlpesCoordinates: 45°22′26″N 4°29′20″E / 45.3739°N 4.4889°E / 45.3739; 4.4889CountryFranceRegionAuvergne-Rhône-AlpesDepartmentLoireArrondissementSaint-ÉtienneCantonLe PilatIntercommunalityMonts du PilatGovernment • Mayor (2020–2026) Mireille Tardy[1]Area112.57 km2 (4....

 

 

Arienzo

ArienzoKomuneComune di ArienzoLokasi Arienzo di Provinsi CasertaNegaraItaliaWilayah   CampaniaProvinsiCaserta (CE)Luas[1] • Total14,01 km2 (5,41 sq mi)Ketinggian[2]70 m (230 ft)Populasi (2016)[3] • Total5.333 • Kepadatan380/km2 (990/sq mi)Zona waktuUTC+1 (CET) • Musim panas (DST)UTC+2 (CEST)Kode pos81021Kode area telepon0823Situs webhttp://www.comune.arienzo.ce.it Arienzo adala...

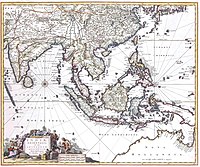
Peristiwa Andi Azis

Halaman ini berisi artikel tentang the 1950 conflict in Indonesia. Untuk the 1686 conflict in Siam, lihat Makassar revolt of 1686. pemberontakan makassarBagian dari Revolusi Nasional IndonesiaBatalyon Worang pemerintah pusat, menduduki Pelabuhan Makassar, 20–21 April 1950Tanggal5–21 April 1950(2 minggu dan 2 hari)LokasiMakassar; JakartaHasil Pemerintah Indonesia menang Pemberontak telah ditekan dan Andi aziz kemudian dipenjaraPihak terlibat Angkatan Darat Indonesia Pembe...

 

 

Kastellet ferry

KastelletledenThe ferry underway with Vaxholm Castle behindTransit typeCable ferryCarries130 passengersOperatorTrafikverketTravel time2 minutesFrequency2-4 return trips per hourNo. of vessels1 (Vaxholmen) The ferry at its town terminal, showing the large drum that carries the electrical power cable, which can also been seen attached at the land end. The Kastellet ferry (Swedish: Kastelletleden), also known as the Vaxholmen ferry, is a passenger cable ferry in Sweden's Stockholm archipelago. I...