Empilement de carrĂŠs dans un carrĂŠ
|
Read other articles:

اŮŘšŮاŮات اŮ٠اŮŮزŮŘŠ اŮ٠ؾعŮŘŠ ٠اŮŮزŮا ٠ؾع ٠اŮŮزŮا ٠ؾع تؚدŮ٠٠ؾدع٠- تؚدŮ٠اŮŘšŮاŮات اŮ٠اŮŮزŮŘŠ اŮ٠ؾعŮŘŠ Ů٠اŮŘšŮاŮات اŮŘŤŮا،ŮŘŠ اŮŘŞŮ ŘŞŘŹŮ Řš بŮ٠٠اŮŮزŮا Ů٠ؾع.[1][2][3][4][5] Ů ŮاعŮŘŠ بŮ٠اŮبŮŘŻŮŮ ŮŘ°Ů Ů ŮاعŮŘŠ ؚا٠؊ Ů٠ع؏ؚŮŘŠ ŮŮŘŻŮŮŘŞŮŮ: Ů؏٠اŮŮ ŮاعŮŘŠ ٠اŮŮزŮا Ů Řľ...

Ekspedisi Belanda ke ValdiviaBagian dari Perang Delapan Puluh TahunHendrik Brouwer mengatur dan memimpin ekspedisi ini hingga ia meninggal di ChiloĂŠTanggal6 November 1642 â 28 December 1643(1 tahun, 1 bulan, 3 minggu dan 1 hari)LokasiPantai Pasifik dari koloni Spanyol di Amerika SelatanPihak terlibat Republik Belanda Kerajaan SpanyolTokoh dan pemimpin Hendrik Brouwer Elias Herckmans Pasukan ChiloĂŠ: AndrĂŠs Herrera â Fernando de Alvarado Respons Spanyol (1644â1...

Tony GoldwynLahirAnthony Howard GoldwynPekerjaanAktor, direkturTahun aktif1986âsekarangSuami/istriJane Michelle Musky (1987âsekarang) Tony Goldwyn terlahir dengan nama Anthony Howard Goldwyn (lahir 20 Mei 1960 adalah pemeran pria Amerika Serikat. Dia digambarkan penjahat Carl Bruner dalam Ghost, Kolonel Bagley dalam The Last Samurai, dan suara Tarzan dari Disney film animasi Tarzan. Dia bintang di ABC dalam drama Scandal, sebagai Fitzgerald Hibah III, Presiden Amerika Serikat. Filmo...

Premier of British Columbia from 2017 to 2022 For other people named John Horgan, see John Horgan (disambiguation). His ExcellencyJohn HorganHorgan in 2017Canadian Ambassador to GermanyIncumbentAssumed office December 8, 2023Prime MinisterJustin TrudeauPreceded byIsabelle Poupart (acting)36th Premier of British ColumbiaIn officeJuly 18, 2017 â November 18, 2022MonarchsElizabeth IICharles IIILieutenant GovernorJudith GuichonJanet AustinDeputyCarole JamesMike FarnworthPrecede...

South Korean actress (born 1985) For the actress born 1993, see Yoon So-hee. In this Korean name, the family name is Moon. In the stage name or pen-name, the surname is Yoon. Yoon So-yiYoon So-yiBornMoon So-yi (1985-01-05) January 5, 1985 (age 39)Seongdong-gu, Seoul, South Korea[1]EducationDongguk UniversityOccupationActressYears active2001âpresentAgentEL Park[2]Spouse Jo Sung-yoon (m. 2017)Children1 Korean nameHangulě¤ěě´Hanjaĺ°š...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article adopte un point de vue rĂŠgional ou culturel particulier et nĂŠcessite une internationalisation (septembre 2022). Merci de l'amĂŠliorer ou d'en discuter sur sa page de discussion ! Vous pouvez prĂŠciser les sections Ă internationaliser en utilisant {{section Ă internationaliser}}. Cet article est une ĂŠbauche concernant les traditions. Vous pouvez partager vos connaissances en lâamĂŠliorant (...

Civil parish in Algarve, PortugalQuarteiraCivil parish Coat of armsQuarteiraLocation in PortugalCoordinates: 37°04â˛08âłN 8°06â˛11âłW / 37.069°N 8.103°W / 37.069; -8.103Country PortugalRegionAlgarveIntermunic. comm.AlgarveDistrictFaroMunicipalityLoulĂŠArea ⢠Total38.16 km2 (14.73 sq mi)Population (2011) ⢠Total21,798 ⢠Density570/km2 (1,500/sq mi)Time zoneUTCÂą00:00 (WET) ⢠Summer (DS...

Species of shark Pacific spiny dogfish Conservation status Least Concern (IUCN 3.1)[1] Secure (NatureServe)[2] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Chondrichthyes Subclass: Elasmobranchii Subdivision: Selachimorpha Order: Squaliformes Family: Squalidae Genus: Squalus Species: S. suckleyi Binomial name Squalus suckleyiGirard, 1854[3] Locations of Squalus suckleyi in the Pacific. The Pacific spiny dogfish ...

Ůذ٠اŮŮ ŮاŮŘŠ ؚ٠اŮŮ ŘŹŮ Ůؚ؊ اŮؚعŮŮŘŠ اŮأتعا٠ŮŮŮŘł ؚ٠٠٠ŮŘŮ ŮŮŮ ŘŹŮŘłŮŘŠ اŮŘŹŮ ŮŮŘąŮŘŠ اŮŘŞŘąŮŮŘŠ أتعاŮTĂźrkler (باŮŘŞŘąŮŮŘŠ) اŮتؚداد اŮŮŮŮاŮتؚداد 70~83 Ů ŮŮŮŮ Ůس٠؊٠Ůاء٠اŮŮŘŹŮŘŻ اŮŮ Ů Ůز؊اŮبŮŘŻ اŮŮا،٠؊ ... ŘŞŘąŮŮاأŮ٠اŮŮاسŮŘąŮااŮؚعاŮبŮغاعŮااŮŮŮاŮات اŮŮ ŘŞŘŘŻŘŠŮŘąŮسااŮŮ Ů ŮŮŘŠ اŮŮ ŘŞŘŘŻŘŠŮŮŮŮدااŮŮ٠ساأستعاŮŮďż˝...

British lawyer and politician The Right HonourableCharles Pelham VilliersEngraving by John Cochran after a portrait by C. A. Du Val.President of the Poor Law BoardIn office9 July 1859 â 26 June 1866MonarchVictoriaPrime MinisterThe Viscount Palmerston The Earl RussellPreceded byThomas Milner GibsonSucceeded byGathorne HardyMember of Parliamentfor Wolverhampton South Wolverhampton (1835â1885)In office6 January 1835 â 16 January 1898MonarchsWilliam IVVictor...

Catholic university in Rome Not to be confused with Pontifical Urban University. Pontificio Collegio Urbano de Propaganda FideEstablished1 August 1627 (1627-08-01)FounderPope Urban VIIIParent institutionCongregation for the Evangelization of PeoplesReligious affiliationCatholic ChurchRectordon Armando NugnesLocationRome, Italy41°53â˛58.5âłN 12°27â˛30.1âłE / 41.899583°N 12.458361°E / 41.899583; 12.458361Websitewww.collegiourbano.org The Pontificio...

Cathedral in Riga, Latvia This article is about the Evangelical Lutheran cathedral of Riga. For the Roman Catholic cathedral, see St. James's Cathedral, Riga. For the Orthodox cathedral, see Cathedral of the Nativity of Christ, Riga. Church in Riga, LatviaRiga CathedralThe Cathedral of Saint MaryRÄŤgas DomsRiga Cathedral with Riga Castle and Daugava River in the backgroundRiga Cathedral56°56â˛57âłN 24°6â˛16âłE / 56.94917°N 24.10444°E / 56.94917; 24.10444Locatio...

Desulfovibrio vulgaris salah satu spesies mikroorganisme reduktor sulfat yang banyak dipelajari. Mikroorganisme reduktor sulfat (sulfate-reducing microorganisms, SRM) adalah adalah kelompok mikroorganisme yang terdiri dari bakteri (bakteri reduktor sulfat, sulfate-reducing bacteria, SRB) dan arkea (arkea reduktor sulfat), yang dapat melakukan respirasi anaerobik menggunakan ion sulfat (SO42â) dan mereduksinya menjadi hidrogen sulfida (H2S).[1] Dengan kata lain, mikroorganisme ini ti...

Motion of particles in a fluid For flows in graph theory, see flow network. This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Flow mathematics â news ¡ newspapers ¡ books ¡ scholar ¡ JSTOR (May 2020) Flow in phase space specified by the differential equation of a pendulum. On the horizontal axis...

For the Anglo-Saxon kingdom, see Mercia. For other uses, see Murcia (disambiguation). Municipality in Region of Murcia, SpainMurciaMunicipalityCathedralCity hall (Moneo building)Monteagudo CastleThe Old BridgeUniversity cloisterCasa CerdĂĄ FlagCoat of armsLocation of MurciaCoordinates: 37°59â˛10âłN 1°7â˛49âłW / 37.98611°N 1.13028°W / 37.98611; -1.13028CountrySpainAutonomous communityRegion of MurciaFounded825 AD (by Abd ar-Rahman II)Government ⢠May...

Perilaku menyimpang yang juga biasa dikenal dengan nama penyimpangan sosial adalah perilaku yang tidak sesuai dengan norma sosial di masyarakat atau suatu kelompok atau aturan yang telah diinstitusikan, yaitu aturan yang telah disepakati bersama dalam sistem sosial.[1] Dalam Kamus Besar Bahasa Indonesia perilaku menyimpang diartikan sebagai tingkah laku, perbuatan, atau tanggapan seseorang terhadap lingkungan yang bertentangan dengan norma-norma dan hukum yang ada di dalam masyarakat....

Lorenzo Flaherty in una scena del film Pazzo d'amore (1999) Lorenzo Flaherty (Roma, 24 novembre 1967) è un attore italiano, di padre irlandese e di madre italiana[1][2]. Indice 1 Biografia 2 Vita privata 3 Filmografia 3.1 Cinema 3.2 Televisione 4 Programmi televisivi 4.1 Pubblicità 5 Note 6 Collegamenti esterni Biografia Dopo il debutto nel 1986 con il film Dèmoni 2... L'incubo ritorna, regia di Lamberto Bava, lavora anche in teatro sia come attore recitando nel Macbeth sia...
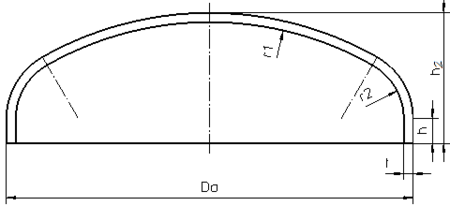
ĺççśĺ§ĺ厚ĺ¨ăŽä¸ĄçŤŻé¨ĺăŤéĄćżăćşśćĽăăăŚăă éĄćżďźăăăżăăďźă¨ăŻăéŤĺ§ăŹăšăżăłăŻăĺĺçč¨ĺçăŽĺççśĺ§ĺ厚ĺ¨ăŽä¸ĄçŤŻé¨ăăľăă[1]ăĺĺçśăťćĽĺçśăŞăŠăŽĺ˝˘çśăăăŚăăćżă ćŚčŚ JIS B 8247:1999 ĺ§ĺ厚ĺ¨ç¨éĄćżăŤčŚć źăčŚĺŽăăăŚăăăĺ称ăŽçąćĽăŻăéĄé¤ ăŽĺ˝˘çśăŤäźźăŚăăă¨ăăé 樽ăŽčăăăăăżăă¨ĺźă°ăăăă¨ăŤçąćĽăăă¨ăăăăăăŽďż˝...

This section tabulates the heads of qualification in a form suitable to be filled in as events progress. The full qualification rules[1] for shooting published by ISSF contain intricate conditions too lengthy for inclusion in Wikipedia. Shooting at the2024 Summer OlympicsQualificationRifle50 m rifle three positionsmenwomen10 m air riflemenwomenmixedPistol25 m pistolwomen25 m rapid fire pistolmen10 m air pistolmenwomenmixedShotgunTrapmenwomenSkeetmenwomenmixedvte This article details ...

German otologist August Lucae (1835-1911) Johann Constantin August Lucae (24 August 1835 – 17 March 1911) was a German otologist who was a native of Berlin. He studied medicine in Berlin and Bonn, and in 1859 earned his doctorate. He furthered his studies in London with Joseph Toynbee (1815–1866), later returning to Berlin, where he worked in Virchow's pathological institute. In 1871 he became an associate professor, and in 1874 was appointed director of the university policlinic ...



