Fermita-malfermita aro

En topologio, fermita-malfermita aro en topologia spaco estas aro, kiu estas kaj malfermita aro, kaj fermita aro.

Ekzemploj

Estu la spaco X kiu konsistas el la kunaĵo de la du intervaloj, [0,1] kaj [2,3]. La topologio sur X estas heredita kiel la subspaca topologio de la ordinara topologio sur la reela linio R. En X, la aro [0,1] estas fermita-malfermita, same la aro [2,3]. Ĉi tiu estas sufiĉe tipa ekzemplo: se spaco estas farita kiel kunaĵo el finia kvanto de disaj koneksaj spacoj kiel komponanto tiamaniere, la ĉiu el la komponantoj estas fermita-malfermita.

En ĉiu topologia spaco X, la malplena aro kaj la tuta spaco X estas fermita-malfermita.[1][2]

Kiel malpli bagatela ekzemplo, estu la spaco Q de ĉiuj racionalaj nombroj kun ilia ordinara topologio, kaj estu aro A de ĉiuj pozitivaj racionalaj nombroj kies kvadrato estas pli granda ol 2. Pro tio ke √2 estas ne en Q, A estas fermita-malfermita subaro de Q. Notu ke tamen A ne estas fermita-malfermita subaro de la reela linio R, ĝi estas nek malfermita nek fermita en R.

Ecoj

  • Aro estas fermita-malfermita se kaj nur se ĝia rando estas malplena.
  • Ĉiu fermita-malfermita aro estas unio de (eble malfinie multaj) koneksaj komponantoj.
  • Se ĉiuj koneksaj komponantoj de topologia spaco X estas malfermitaj (ekzemple, se X havas nur finie multajn komponantojn, aŭ se X estas loke koneksa), tiam aro estas fermita-malfermita en X se kaj nur se ĝi estas unio de koneksaj komponantoj.
  • Topologia spaco X estas koneksa se kaj nur se la nuraj fermitaj-malfermitaj aroj en X estas la malplena aro kaj X.
  • Topologia spaco X estas diskreta se kaj nur se ĉiu el ĝiaj subaroj estas fermita-malfermita.
  • La operacioj de formado de kunaĵoj kaj komunaĵoj igas la aron de fermitaj-malfermitaj subaroj de donita topologia spaco X bulea algebro. Ĉiu Bulea algebro povas esti ricevita tiamaniere el taŭga topologia spaco, vidu en kerna prezenta teoremo por buleaj algebroj.

Vidu ankaŭ

Eksteraj ligiloj

Read other articles:

Hopi

Untuk kegunaan lain, lihat Hopi (disambiguasi). Suku HopiSeorang wanita Hopi berbusana tradisionalJumlah populasi18.327 (2010) terdaftar[1]Daerah dengan populasi signifikan Amerika Serikat ( Arizona)BahasaHopi, InggrisKelompok etnik terkaitSuku bangsa Pueblo, suku bangsa Uto-Aztek Hopi adalah sebuah suku Amerika Asli yang biasanya hidup di Cagar Alam Hopi, timur laut Arizona. Menurut sensus 2010, terdapat 19.338 Hopi di Amerika Serikat.[1] Referensi ^ a b Bureau, U. ...

 

Kapal perusak Jepang Sagiri

Sagiri beralih ke halaman ini. Untuk nama desa di Azerbaijan, lihat Sığırlı. Sagiri mengarungi laut pada 10 Agustus 1936. Sejarah Kekaisaran Jepang Nama SagiriDipesan 1923 (Tahun Fiskal)Pembangun Perusahaan Dok UragaNomor galangan Perusak No. 50Pasang lunas 28 Maret 1929Diluncurkan 23 Desember 1929Mulai berlayar 31 Januari 1931Dicoret 15 Januari 1942Identifikasi Nomor lambung: 20Nasib Tenggelam oleh HNLMS K XVI pada 24 Desember 1941 Ciri-ciri umum Kelas dan jenis Kapal perusak kelas-...

 

Superclass (book)

2008 book by David Rothkopf Superclass AuthorDavid RothkopfCountryUnited StatesLanguageEnglishGenrePolitics, Globalization, Global governancePublished2008 Farrar, Straus and Giroux (U.S.)2008 Little, Brown (UK)Media typePrint (Hardback & Paperback) and audiobookPages376 p. (US hardback edition) & 400 p. (UK hardback edition)ISBN0-374-27210-7 (US hardback edition), ISBN 1-4087-0109-X (UK hardback edition)OCLC166378239Dewey Decimal305.5/2 22LC ClassHM1263 .R68 2008Precede...

Aki-ku, Hiroshima

Aki-ku 安芸区Distrik kotaLokasi Aki-ku di kota HiroshimaNegara JepangWilayahChūgokuPrefektur HiroshimaKotaHiroshimaLuas • Total94,1 km2 (363 sq mi)Populasi (Oktober 1, 2015) • Total79.353 • Kepadatan843,3/km2 (21,840/sq mi)Zona waktuUTC+9 (JST)Kode pos736-8501Alamat3-4-36 Funakoshiminami,Aki-ku, Hiroshima-shi, Hiroshima-kenSitus webSitus web resmi Aki-ku (安芸区code: ja is deprecated , Aki-ku) adalah salah satu dari...

 

MTs Negeri Tumpang

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. MTS NEGERI 7 MALANG (d/h MTS NEGERI TUMPANG)InformasiKepala SekolahPono, S.Ag, M.PdJumlah siswa± 500 siswaStatusNegeriAlamatLokasiJL. Raya Pandanajeng no. 25, Kecamatan : Tumpang, Malang 65156 Telp 0341-8561108, Malang, Jawa TimurSitus webwww.mtsn...

 

Clyde Walcott

West Indian Test cricketer Sir Clyde WalcottOBE DAPersonal informationFull nameClyde Leopold WalcottBorn(1926-01-17)17 January 1926Saint Michael, BarbadosDied26 August 2006(2006-08-26) (aged 80)BarbadosHeight6 ft 2 in (1.88 m)BattingRight-handedBowlingRight-arm fast-mediumRoleWicket-keeperInternational information National sideWest IndiesTest debut (cap 58)21 January 1948 v EnglandLast Test31 March 1960 v England Domestic team information ...

Gulf Cooperation Council Athletics Championships

The Gulf Cooperation Council Athletics Championship (also known as the GCC Athletics Championships) is a biennial international athletics competition between athletes from nations within the Cooperation Council for the Arab States of the Gulf. It was first held in 1986. In addition to the senior championship, the GCC also holds the Gulf Cooperation Council Youth Athletics Championships.[1] The GCC holds championships for various other sports, including golf, basketball and fencing. Th...

 

Climate change policy of the George W. Bush administration

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Climate change policy of the George W. Bush administration – news · newspapers · books · scholar · JSTOR (...

 

I Believe You ~Ai no Hana~

2007 single by Rina Aiuchi I Believe You ~Ai no Hana~Limited edition coverSingle by Rina Aiuchifrom the album Trip B-sideHey!Harmony (Limited edition)ReleasedMay 7, 2008 (2008-05-07)GenreJ-popLength5:05LabelGiza StudioSongwriter(s)Rina AiuchiKoji GotoProducer(s)Rina AiuchiKannonjiRina Aiuchi singles chronology Nemurenu Yo ni / Party Time Party Up(2007) I Believe You ~Ai no Hana~ (2008) Kimi to no Deai ~Good Bye My Days~ (2007) I Believe You ~Ai no Hana~ (I believe you ～愛の...

Modern Standard Arabic

Formal literary variety of Arabic Standard Arabic redirects here. For the classical language, see Classical Arabic. For the general article, see Arabic. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Modern Standard Arabic – news · newspapers · books · scholar · JSTOR (July 2020) (Learn how and when to remo...

 

Sonata per clarinetto e pianoforte (Bernstein)

Sonata per clarinetto e pianoforte(Clarinet Sonata)Bernstein nel 1944CompositoreLeonard Bernstein Tipo di composizioneSonata Epoca di composizione1941-1942 Prima esecuzione1942 Pubblicazione1942 DedicaDavid Oppenheim Durata media10 minuti Movimentidue Manuale La Sonata per clarinetto e pianoforte di Leonard Bernstein, scritta tra il 1941 e il 1942 e pubblicata nel 1942, fu il primo brano pubblicato da Bernstein.[1] È dedicato al clarinettista David Oppenheim, che Bernstein incontrò ...

 

1 Raja-raja 20

1 Raja-raja 20Kitab Raja-raja (Kitab 1 & 2 Raja-raja) lengkap pada Kodeks Leningrad, dibuat tahun 1008.KitabKitab 1 Raja-rajaKategoriNevi'imBagian Alkitab KristenPerjanjian LamaUrutan dalamKitab Kristen11← pasal 19 pasal 21 → 1 Raja-raja 20 (atau I Raja-raja 20, disingkat 1Raj 20) adalah bagian dari Kitab 1 Raja-raja dalam Alkitab Ibrani dan Perjanjian Lama di Alkitab Kristen. Dalam Alkitab Ibrani termasuk Nabi-nabi Awal atau Nevi'im Rishonim [נביאים ראשונים] dala...

Buah merah papua

Pandanus conoideus Klasifikasi ilmiah Kerajaan: Plantae (tanpa takson): Angiospermae (tanpa takson): Monokotil Ordo: Pandanales Famili: Pandanaceae Genus: Pandanus Spesies: P. Conoideus Nama binomial Pandanus conoideusLam. Biji Buah Merah (Pandanus conoideus Lamk.) Buah Merah adalah sejenis buah tradisional dari Pulau Papua. Khususnya masyarakat Wamena, Papua Pegunungan, buah ini disebut kuansu. Nama ilmiahnya Pandanus Conoideus karena tanaman ini termasuk tanaman keluarga pandan-pandan...

 

New Zealand cricket team in the United Arab Emirates in 2023

International cricket tour New Zealand men's cricket team in the UAE in 2023    United Arab Emirates New ZealandDates 17 – 20 August 2023Captains Muhammad Waseem Tim SoutheeTwenty20 International seriesResults New Zealand won the 3-match series 2–1Most runs Aryansh Sharma (76) Mark Chapman (129)Most wickets Junaid Siddique (5) Tim Southee (6)Player of the series Mark Chapman (NZ) The New Zealand men's cricket team toured the United Arab Emirates in August 2023 to play three...

 

Oshawa Car Assembly

Vehicle manufacturing facility in Oshawa, Ontario For the plant closed in 2009, see Oshawa Truck Assembly. Oshawa AssemblyEntrance to the plant as seen in 2013Operated1953–present [1]LocationOshawa, CanadaCoordinates43°52′03″N 78°51′59″W / 43.8676°N 78.8664°W / 43.8676; -78.8664IndustryAutomotiveProductsAutomobilesVolume5,100,000 sq ft (470,000 m2) [1]Address900 Park Rd S OshawaOwner(s)General Motors CanadaWebsitegm.ca/oshaw...

Tony Hoare

British computer scientist For the bicycle racer, see Tony Hoar. SirTony HoareFRS FREngTony Hoare in 2011BornCharles Antony Richard Hoare (1934-01-11) 11 January 1934 (age 90)Colombo, British CeylonEducation University of Oxford (BA, MA) Moscow State University Known for Quicksort Quickselect Hoare logic Null reference Communicating sequential processes Structured programming ALGOL SpouseJill PymChildren3AwardsTuring Award (1980) Harry H. Goode Memorial Award (1981) Faraday Meda...

 

財閥解体

財閥解体（ざいばつかいたい）は、国際政治方針で財閥などの巨大企業が解体される現象である。 日本では、1945年より1952年にかけて行われた連合国軍最高司令官総司令部（GHQ）の占領政策の1つ。→本項で詳述する。 ドイツでは、1947年より1951年にかけて連合国がIG・ファルベンインドゥストリーを解体した。 財閥の株券差し押さえを行うアメリカ軍（1946年）。株式�...

 

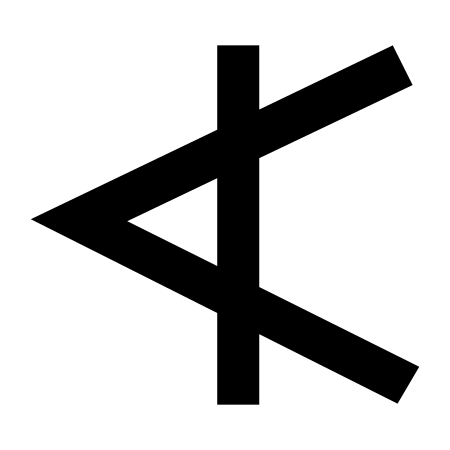
Alphabet grec

Alphabet grec « Alphabet grec » (Ellinikó alfávito) en grec moderne Caractéristiques Type Alphabet Langue(s) Grec ancien, grec moderne et de nombreuses langues par le passé Direction aujourd'hui, de gauche à droite Historique Époque VIIIe siècle av. J.-C. à nos jours Système(s) parent(s) Alphabet proto-cananéen  Alphabet phénicien   Alphabet grec Système(s) dérivé(s) arménien, copte, cyrillique, étrusque, glagolitique, gotique, latin Codag...

Manuel Teixeira Gomes

Portuguese president and politician For the linguist, see Manuel Teixeira (linguist). This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Manuel Teixeira Gomes – news · newspapers · books · scholar · JSTOR (December 2009) (Learn how and when to remove this message) His ExcellencyManuel Teixeira GomesGCSEPresident of PortugalIn of...

 

Director General of MI5

Head of the UK's Security Service Director Generalof the Security Service (MI5)IncumbentKen McCallumsince 30 March 2020; 4 years ago (2020-03-30)Security Service (MI5)ResidenceThames House, London, UKAppointerHome SecretaryTerm lengthNo fixed termInaugural holderVernon Kell1909FormationSecurity Service Act 1989Websitehttps://www.mi5.gov.uk/ The Director General of the Security Service is the head of the Security Service (commonly known as MI5), the United Kingdom's inte...