Read other articles:

Basilika Tempat Ziarah Bunda dari Medali Penuh MukjizatBasilika Minor Tempat Ziarah Bunda dari Medali Penuh MukjizatInggris: The Basilica Shrine of Our Lady of the Miraculous Medalcode: en is deprecated Basilika Tempat Ziarah Bunda dari Medali Penuh MukjizatLokasi500 E. Chelten AvePhiladelphia, PennsylvaniaNegara Amerika SerikatDenominasiGereja Katolik RomaSitus webwww.miraculousmedal.orgSejarahPendiriThe Congregation of the Mission of St. Vincent de Paul, Eastern Province, USAArsitektur...

Luigi ComenciniComencini pada 1971Lahir(1916-06-08)8 Juni 1916Salò, Lombardy, Kerajaan ItaliaMeninggal6 April 2007(2007-04-06) (umur 90)Roma, ItaliaTahun aktif1937–1991 Luigi Comencini (pengucapan bahasa Italia: [luˈiːdʒi komenˈtʃiːni]; 8 Juni 1916 – 6 April 2007)[1][2] adalah seorang sutradara asal Italia. Bersama dengan Dino Risi, Ettore Scola dan Mario Monicelli, ia dianggap sebagai salah satu master dari genre commedia all'italiana....

Serie C1 1978-1979 Competizione Serie C1 Sport Calcio Edizione 1ª Organizzatore Lega Nazionale Semiprofessionisti Date dal 1º ottobre 1978al 17 giugno 1979 Luogo Italia Partecipanti 36 Formula 2 gironi all'italiana A/R Risultati Vincitore Como (1º titolo)Matera (1º titolo) Altre promozioni ParmaPisa Retrocessioni BarlettaLatinaLuccheseModenaPadovaPaganeseSpeziaTrento Statistiche Miglior marcatore Sante Crepaldi (17) Incontri disputati 613 Gol segnati 1 065 (1,74 pe...

Untuk kegunaan lain, lihat Halmahera. Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Pulau Halmahera – berita · surat kabar · buku · cendekiawan · JSTOR HalmaheraGeografiLokasiAsia TenggaraKoordinat0°36′N 127°52′E / 0.600°N 127...

Street Fighter character Fictional character F.A.N.GStreet Fighter characterFirst appearanceStreet Fighter V (2016)Created byCapcomVoiced byJapaneseShigeru Chiba EnglishJesse MerlinIn-universe informationFighting styleChinese Kenpō with poisonOriginUnknownNationalityUnknown F.A.N.G (ファン, Fan) is a character in Capcom's Street Fighter series. He made his first appearance in Street Fighter V as one of sixteen playable characters at the game's launch in 2016. Within series lore, F.A.N.G i...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Desember 2022. Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Oktober 2022. Topik ...

For the parent molecule 9,10-anthraquinone, see anthraquinone Structure proposed for the pigment carmine. Anthraquinones (also known as anthraquinonoids) are a class of naturally occurring phenolic compounds based on the 9,10-anthraquinone skeleton. They are widely used industrially and occur naturally. The name anthraquinone was first used by German chemists Carl Graebe and Carl Theodore Liebermann in a 1868 publication describing the chemical synthesis of the red dye alizarin from anthracen...

Israeli military offensive in northern Palestine during the 1948 Arab-Israeli War Operation DekelPart of the 1948 Arab–Israeli WarDate8–18 July 1948LocationNorthern PalestineResult Israeli victoryBelligerents  Israel Defense Forces Arab Liberation ArmyCommanders and leaders Ben DunkelmanHaim Fish †[1] Units involved 7th Armored Brigade (Israel) Golani Brigade Operation Dekel (Hebrew: מבצע דקל, romanized: Mivtza Dekel, lit. 'Operation Palm Tree...

Village in West Yorkshire, England Human settlement in EnglandScholes-in-ElmetMain Street, ScholesScholes-in-ElmetShow map of LeedsScholes-in-ElmetLocation within West YorkshireShow map of West YorkshirePopulation2,266 OS grid referenceSE 37766 37254Metropolitan boroughCity of LeedsMetropolitan countyWest YorkshireRegionYorkshire and the HumberCountryEnglandSovereign stateUnited KingdomPost townLeedsPostcode districtLS15PoliceWest YorkshireFireWest Yorkshire...

この項目には、一部のコンピュータや閲覧ソフトで表示できない文字が含まれています（詳細）。 数字の大字（だいじ）は、漢数字の一種。通常用いる単純な字形の漢数字（小字）の代わりに同じ音の別の漢字を用いるものである。 概要 壱万円日本銀行券（「壱」が大字） 弐千円日本銀行券（「弐」が大字） 漢数字には「一」「二」「三」と続く小字と、「壱」「�...

2016年美國總統選舉 ← 2012 2016年11月8日 2020 → 538個選舉人團席位獲勝需270票民意調查投票率55.7%[1][2] ▲ 0.8 %   获提名人 唐納·川普 希拉莉·克林頓 政党 共和黨 民主党 家鄉州 紐約州 紐約州 竞选搭档 迈克·彭斯 蒂姆·凱恩 选举人票 304[3][4][註 1] 227[5] 胜出州/省 30 + 緬-2 20 + DC 民選得票 62,984,828[6] 65,853,514[6]...

French art dealer (1866–1939) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Ambroise Vollard – news · newspapers · books · scholar · JSTOR (February 2013) (Learn how and when to remove this message) Ambroise VollardVollard, in front of Picasso's Evocación. El entierro de CasagemasBorn(1866-07-03)3 July ...

Частина серії проФілософіяLeft to right: Plato, Kant, Nietzsche, Buddha, Confucius, AverroesПлатонКантНіцшеБуддаКонфуційАверроес Філософи Епістемологи Естетики Етики Логіки Метафізики Соціально-політичні філософи Традиції Аналітична Арістотелівська Африканська Близькосхідна іранська Буддій�...

Under-construction passenger rail system California High-Speed RailA map of planned high-speed rail routes in California. The separate Brightline West is indicated in yellow.OverviewOwnerCalifornia High-Speed Rail Authority an agency of the State of CaliforniaArea servedInitial Operating Segment (IOS) now being developed in the Central Valley (Merced to Bakersfield);Planned Phase 1 extensions:   north to San Francisco Bay Area   south to Greater Los Angeles;Futur...

Gaelic festival marking the start of spring Imbolc / St Brigid's DayBrigid's crossAlso calledLá Fhéile Bríde (Irish)Là Fhèill Brìghde (Scottish Gaelic)Laa'l Breeshey (Manx)Observed byHistorically: GaelsToday: Irish people, Scottish people, Manx people, Modern PagansTypeCultural,Christian (Roman Catholic, Anglican),Pagan (Celtic neopaganism, Wicca)Significancebeginning of spring, feast day of Saint BrigidCelebrationsfeasting, making Brigid's crosses and Brídeógs, vi...

Small single-serving cake, often frosted For the American rapper, see Cupcakke. For other uses, see Cupcake (disambiguation). CupcakePlain cupcake with strawberry icing, and chocolate cupcake with chocolate icingAlternative namesFairy cake, patty cake,[1] cup cakeTypeCakePlace of originUnited StatesMain ingredientsButter, sugar, eggs, flour; optionally frosting and other cake decorations  Media: Cupcake A cupcake (AmE), fairy cake (BrE), or bun (IrE) is a small cake designed ...

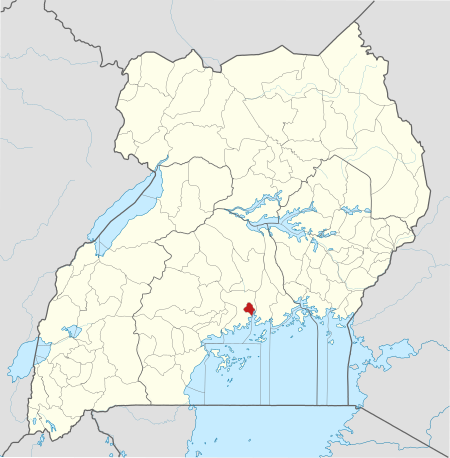
Place in Central Uganda, UgandaKigoowaKigoowaLocation in KampalaCoordinates: 00°21′45″N 32°36′54″E / 0.36250°N 32.61500°E / 0.36250; 32.61500Country UgandaRegionCentral UgandaDistrictKampala Capital City AuthorityDivisionNakawa DivisionElevation4,070 ft (1,240 m) Kigoowa is a location within the city of Kampala, Uganda's capital. Location Located in Nakawa Division, Kigoowa is bordered by Kulambiro to the north, Kiwaatule to the east, Ntinda to th...

See also: Timeline of the Tang dynasty Part of Chinese history, 618 c.e. – 907 c.e. The Tang dynasty at its height in the 660s The military history of the Tang dynasty encompasses the period of Chinese military activity from 618 to 907. The Tang dynasty and the preceding Sui dynasty share many similar trends and behaviors in terms of military tactics, strategy, and technology, so it can be viewed that the Tang continued the Sui tradition. Organization Main article: Administrative divisions ...

Quận 16, Paris Vị trí Bản đồ Paris  1   2   3   4   5   6   7   8   9   10   11   12   13   14   15   16   17   18   19   20  Hauts-de-Seine  Seine-Saint-Denis  Val-de-Marne  Paris và các vùng ngoại ô Hành chính Vùng Île-de-France Tỉnh Paris Quận trưởng ...

146 магнитных шариков, образующие октаэдр Октаэдральное число — разновидность многогранных фигурных чисел. Поскольку октаэдр можно рассматривать как две квадратные пирамиды, склеенные своими основаниями (см. рисунок), октаэдральное число определяется как сумма двух по�...