Principal curvature
| ||||||||||||||||||||||
Read other articles:
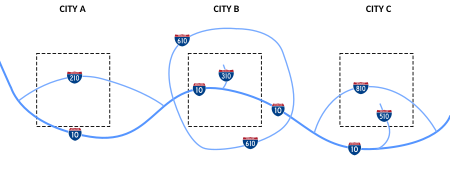
Dwight D. Eisenhower National System of Interstate and Defense HighwaysHighway shield for Interstate 295Interstate Highways in the 48 contiguous statesSystem informationFormedJune 29, 1956[1]Highway namesInterstatesInterstate X (I-X)System links Interstate Highway System Main Auxiliary Suffixed Business Future Auxiliary Interstate Highways (also called three-digit Interstate Highways) are a subset of highways within the United States' Interstate Highway System. The 323 auxi...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: Asuransi Bintang – berita · surat kabar · buku · cendekiawan · JSTORArtikel ini perlu dikembangkan agar dapat memenuhi kriteria sebagai entri Wikipedia.Bantulah untuk mengembangkan artikel ini. Jika tida...

Matius 16Potongan naskah Papirus Magdalen berisi Injil Matius, yang ditulis sekitar tahun 50-70 M.KitabInjil MatiusKategoriInjilBagian Alkitab KristenPerjanjian BaruUrutan dalamKitab Kristen1← pasal 15 pasal 17 → Matius 16 (disingkat Mat 16) adalah pasal keenam belas Injil Matius pada Perjanjian Baru dalam Alkitab Kristen, yang diyakini disusun menurut catatan Matius, salah seorang dari Keduabelas Rasul Yesus Kristus.[1][2][3][4] Teks Naskah aslinya...

Theory of cognitive linguistics Dan Sperber, who, with Deirdre Wilson, developed relevance theory Relevance theory is a framework for understanding the interpretation of utterances. It was first proposed by Dan Sperber and Deirdre Wilson, and is used within cognitive linguistics and pragmatics. The theory was originally inspired by the work of Paul Grice and developed out of his ideas, but has since become a pragmatic framework in its own right. The seminal book, Relevance, was first publishe...

José Leandro Andrade José Leandro Andrade nel 1926 circa Nazionalità Uruguay Altezza 180 cm Peso 79 kg Calcio Ruolo Centrocampista Termine carriera 1935 Carriera Squadre di club1 1921-1923 Bella Vista71 (17)1924-1930 Nacional180 (69)1931 Peñarol24 (6)1932-1933 River Plate (M)50 (11)1934 Atlanta18 (0)1935 Talleres (RdE)8 (3)1935 Peñarol10 (4) Nazionale 1923-1930 Uruguay34 (1) Palmarès Olimpiadi Oro Parigi 1924 Oro Amsterdam 1928 Mondi...

For other schools, see Cyprian (disambiguation) § Schools. Preparatory school in Eastbourne, Sussex, EnglandSt Cyprian's SchoolLocationEastbourne, SussexEnglandCoordinates50°46′07″N 0°15′51″E / 50.7685°N 0.2641°E / 50.7685; 0.2641InformationTypePreparatory SchoolBoarding schoolMottoForsan et haec olim meminisse juvabit.[a]Established1899FounderL.C. Vaughan WilkesClosed1943GenderMAge4 to 14Enrolmentc. 90Colour(s)Green, pale blue, blac...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...

In this Spanish name, the first or paternal surname is Hurtado de Mendoza and the second or maternal family name is Manrique. DonGarcía Hurtado de MendozaMarqués de CañeteCaballero de SantiagoRoyal Governor of ChileIn office1557–1561MonarchPhilip IIPreceded byFrancisco de AguirreRodrigo de QuirogaFrancisco de VillagraSucceeded byFrancisco de Villagra8th Viceroy of PeruIn officeJanuary 8, 1590 – July 24, 1596MonarchPhilip IIPreceded byFernando Torres de PortugalSucceede...

Alan ShepardAstronauta della NASANazionalità Stati Uniti StatusDeceduto Data di nascita18 novembre 1923 Data di morte21 luglio 1998 Selezione1959 (Gruppo 1 NASA) Primo lancio5 maggio 1961 Ultimo atterraggio9 febbraio 1971 Altre attivitàPilota collaudatore Tempo nello spazio9 giorni e 57 minuti Numero EVA2 Durata EVA9h 23min Missioni Mercury-Redstone 3 Apollo 14 Data ritiroagosto 1974 Modifica dati su Wikidata · Manuale Alan Bartlett Shepard Jr. (Derry, 18 novembre 1923 – Pebble...

ヨハネス12世 第130代 ローマ教皇 教皇就任 955年12月16日教皇離任 964年5月14日先代 アガペトゥス2世次代 レオ8世個人情報出生 937年スポレート公国(中部イタリア)スポレート死去 964年5月14日 教皇領、ローマ原国籍 スポレート公国親 父アルベリーコ2世(スポレート公)、母アルダその他のヨハネステンプレートを表示 ヨハネス12世(Ioannes XII、937年 - 964年5月14日)は、ロ...

لويس الثامن عشر (بالفرنسية: Louis XVIII) ملك فرنسا ونافارا فترة الحكمقانونياً: 11 يونيو 1795 - 16 سبتمبر 1824 بحكم الأمر الواقع: 11 أبريل 1814 - 20 مارس 1815 ثم 8 يوليو 1815 - 16 سبتمبر 1824 نوع الحكم ملكي نابليون الأول شارل العاشر معلومات شخصية الاسم الكامل لويس ستانسلاس اكزافييه الميلاد 17 نوفم...

Central agency devoted to Indian cinema National Film Development Corporation of IndiaIndustryFilm IndustryPredecessorFilm Finance CorporationFilms Division of IndiaFounded1975HeadquartersMumbai, IndiaArea servedNehru Centre, Dr. Annie Besant Road, Worli, Mumbai – 400 018, IndiaProductsFilmsOwner Ministry of Information and Broadcasting Government of India Websitewww.nfdcindia.com The National Film Development Corporation of India (NFDC) based in Mumbai is the central agency established in...

山崎怜奈2019年1月26日於KKBOX風雲榜頒獎典禮女艺人原文名山崎 怜奈(やまざき れな)罗马拼音Yamazaki Rena昵称Renachi(れなち)[1]Zakisan(ザキさん)[2]国籍 日本出生 (1997-05-21) 1997年5月21日(27歲) 日本東京都江戶川區[3][4]职业電視藝人、主持人语言日語、华語教育程度慶應義塾大學環境情報學部(畢業)母校郁文館中學校・高等學校(日语:�...

Circle of immediate corresponding curvature of a curve at a point Kissing circles redirects here. For Descartes' theorem on mutually tangent (kissing) circles, see Descartes' theorem. An osculating circle Osculating circles of the Archimedean spiral, nested by the Tait–Kneser theorem. The spiral itself is not drawn: we see it as the locus of points where the circles are especially close to each other.[1] An osculating circle is a circle that best approximates the curvature of a curv...

James Dyson James Dyson en 2015Información personalNacimiento 2 de mayo de 1947 (77 años)Cromer (Reino Unido) Nacionalidad BritánicaEducaciónEducado en Byam Shaw School of ArtUniversidad Real de ArteEscuela Gresham Información profesionalOcupación Inventor, diseñador industrial, emprendedor, agricultor y diseñador Afiliaciones Dyson Miembro de Royal Society Sitio web www.jamesdysonfoundation.com Distinciones Comendador de la Orden del Imperio británicoFellow of the Royal Academy of E...

Standing committee of the United States House of Representatives House Foreign Affairs CommitteeStanding committeeActiveUnited States House of Representatives118th CongressHistoryFormed1822LeadershipChairMichael McCaul (R) Since January 3, 2023Ranking memberGregory Meeks (D) Since January 3, 2023Vice chairVacantStructureSeats51Political partiesMajority (27) Republican (27) Minority (23) Democratic (23) JurisdictionPolicy areasForeign policy, aid, diplomacyOversight authorityDepa...

Politics of Nigeria Constitution Human rights Government President (list) Bola Tinubu Vice President Kashim Shettima Cabinet Federal Parastatals Legislature National Assembly of Nigeria Senate President Godswill Akpabio (APC) Deputy President Barau Jibrin (APC) (List of members of the Senate) House of Representatives Speaker Abbas Tajudeen (APC) Deputy Speaker Benjamin Okezie Kalu (APC) (List of members of the House) State delegations Abia Adamawa Akwa Ibom Anambra Bauchi Bayelsa Benue Borno...

Cet article est une ébauche concernant une compétition de football, le football féminin et le Brésil. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Division 1 Généralités Sport Football féminin Création 2013 Organisateur(s) CBF Éditions 5 Périodicité Annuelle Lieu(x) Brésil Participants 16 équipes Statut des participants Professionnel Hiérarchie Hiérarchie 1er niveau Niveau inférieur Sér...

Study of the hospitality industry This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) The examples and perspective in this article may not represent a worldwide view of the subject. You may improve this article, discuss the issue on the talk page, or create a new article, as appropriate. (July 2011) (Learn how and when to remove this message) This article needs additional citations for verifi...

Questa voce sull'argomento università degli Stati Uniti d'America è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. University of Vermont(EN) The University of Vermont and State Agricultural College UbicazioneStato Stati Uniti CittàBurlington Dati generaliNome latinoUniversitas Viridis Montiss SoprannomeCatamounts (sport); UVM MottoStudiis et Rebus Honestis Fondazione1791 FondatoreIra Allen TipoUniversità pubblica RettoreDaniel Mark Fo...


![{\displaystyle \left[I\!I_{ij}\right]={\begin{bmatrix}I\!I(X_{1},X_{1})&I\!I(X_{1},X_{2})\\I\!I(X_{2},X_{1})&I\!I(X_{2},X_{2})\end{bmatrix}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8bffd43b323463adccee7129a8191ca0ad70344b)
![{\displaystyle \left[I\!I_{ij}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2c25a532dc99d4e755b582b3e013b0c91c449144)






