Natural bundle

In differential geometry, a field in mathematics, a natural bundle is any fiber bundle associated to the s-frame bundle $F^{s}(M)$ for some $s\geq 1$ . It turns out that its transition functions depend functionally on local changes of coordinates in the base manifold $M$ together with their partial derivatives up to order at most $s$ .^[1]

The concept of a natural bundle was introduced by Albert Nijenhuis as a modern reformulation of the classical concept of an arbitrary bundle of geometric objects.^[2]

Definition

Let $Mf$ denote the category of smooth manifolds and smooth maps and $Mf_{n}$ the category of smooth $n$ -dimensional manifolds and local diffeomorphisms. Consider also the category ${\mathcal {FM}}$ of fibred manifolds and bundle morphisms, and the functor $B:{\mathcal {FM}}\to {\mathcal {M}}f$ associating to any fibred manifold its base manifold.

A natural bundle (or bundle functor) is a functor $F:{\mathcal {M}}f_{n}\to {\mathcal {FM}}$ satisfying the following three properties:

$B\circ F=\mathrm {id}$ , i.e. $B(M)$ is a fibred manifold over $M$ , with projection denoted by $p_{M}:B(M)\to M$ ;
if $U\subseteq M$ is an open submanifold, with inclusion map $i:U\hookrightarrow M$ , then $F(U)$ coincides with $p_{M}^{-1}(U)\subseteq F(M)$ , and $F(i):F(U)\to F(M)$ is the inclusion $p^{-1}(U)\hookrightarrow F(M)$ ;
for any smooth map $f:P\times M\to N$ such that $f(p,\cdot ):M\to N$ is a local diffeomorphism for every $p\in P$ , then the function $P\times F(M)\to F(N),(p,x)\mapsto F(f(p,\cdot ))(x)$ is smooth.

As a consequence of the first condition, one has a natural transformation $p:F\to B$ .

Finite order natural bundles

A natural bundle $F:Mf_{n}\to Mf$ is called of finite order $r$ if, for every local diffeomorphism $f:M\to N$ and every point $x\in M$ , the map $F(f)_{x}:F(M)_{x}\to F(N)_{f(x)}$ depends only on the jet $j_{x}^{r}f$ . Equivalently, for every local diffeomorphisms $f,g:M\to N$ and every point $x\in M$ , one has $j_{x}^{r}f=j_{x}^{r}g\Rightarrow F(f)|_{F(M)_{x}}=F(g)|_{F(M)_{x}}.$ Natural bundles of order $r$ coincide with the associated fibre bundles to the $r$ -th order frame bundles $F^{s}(M)$ .

A classical result by Epstein and Thurston shows that all natural bundles have finite order.^[3]

Examples

An example of natural bundle (of first order) is the tangent bundle $TM$ of a manifold $M$ .

Other examples include the cotangent bundles, the bundles of metrics of signature $(r,s)$ and the bundle of linear connections.^[4]

Notes

^ Palais, Richard; Terng, Chuu-Lian (1977), "Natural bundles have finite order", Topology, 16: 271–277, doi:10.1016/0040-9383(77)90008-8, hdl:10338.dmlcz/102222
^ A. Nijenhuis (1972), Natural bundles and their general properties, Tokyo: Diff. Geom. in Honour of K. Yano, pp. 317–334
^ Epstein, D. B. A.; Thurston, W. P. (1979). "Transformation Groups and Natural Bundles". Proceedings of the London Mathematical Society. s3-38 (2): 219–236. doi:10.1112/plms/s3-38.2.219.
^ Fatibene, Lorenzo; Francaviglia, Mauro (2003). Natural and Gauge Natural Formalism for Classical Field Theorie. Springer. doi:10.1007/978-94-017-2384-8. ISBN 978-1-4020-1703-2.

References

Kolář, Ivan; Michor, Peter; Slovák, Jan (1993), Natural operators in differential geometry (PDF), Springer-Verlag, archived from the original (PDF) on 2017-03-30, retrieved 2017-08-15
Krupka, Demeter; Janyška, Josef (1990), Lectures on differential invariants, Univerzita J. E. Purkyně V Brně, ISBN 80-210-0165-8
Saunders, D.J. (1989), The geometry of jet bundles, Cambridge University Press, ISBN 0-521-36948-7

Read other articles:

Chantal Goya

Pour les articles homonymes, voir Goya. Chantal GoyaChantal Goya en 2009.BiographieNaissance 10 juin 1942 (81 ans)Saïgon (Cochinchine, Indochine française)Nom de naissance Chantal de GuerreNationalité françaiseFormation Lycée français Charles-de-GaulleActivités Actrice, chanteusePériode d'activité depuis 1963Fratrie Marie-Josée de Guerre (d)Conjoint Jean-Jacques Debout (depuis 1966)Autres informationsTaille 1,65 mPoids 55 kgLabel RCAEMICBS DisquesAB DisquesPolyGramUniversalSon...

Paruh-perak

Paruh-perak Euodice Indian silverbill (Euodice malabarica)TaksonomiKerajaanAnimaliaFilumChordataKelasAvesOrdoPasseriformesFamiliEstrildidaeGenusEuodice Reichenbach, 1862 SpeciesSee text.lbs Euodice atau paruh-perak adalah genus burung pemakan biji kecil dalam keluarga Estrildidae . Spesies ini berasal dari zona kering di Afrika dan India dan biasa. Mereka dahulunya termasuk dalam genus Lonchura . Taksonomi Image Common Name Scientific name Distribution Paruh-perak Afrika Euodice cantans Centr...

Ulangan 5

Ulangan 5Bagian gulungan 4Q41 yang memuat Ulangan 5:1-6:1 termasuk Sepuluh Perintah Allah, dari antara Gulungan Laut Mati yang berasal dari abad ke-1 SM.KitabKitab UlanganKategoriTauratBagian Alkitab KristenPerjanjian LamaUrutan dalamKitab Kristen5← pasal 4 pasal 6 → Ulangan 5 (disingkat Ul 5) adalah bagian dari Kitab Ulangan dalam Alkitab Ibrani dan Perjanjian Lama di Alkitab Kristen. Merupakan kitab ke-5 dan terakhir dalam kumpulan kitab Taurat yang disusun oleh Musa.[1]...

Kota Sibolga

Kota SibolgaKotaTranskripsi bahasa daerah • Surat Batakᯘᯪᯅᯞᯬ᯲ᯎPanorama Kota Sibolga dari Tor Simarbarimbing LambangJulukan: Kota Berbilang KaumKota IkanMotto: Sibolga nauli(Batak Toba) Sibolga yang indahPetaKota SibolgaPetaTampilkan peta SumatraKota SibolgaKota Sibolga (Indonesia)Tampilkan peta IndonesiaKoordinat: 1°44′33″N 98°46′45″E / 1.7425°N 98.7792°E / 1.7425; 98.7792Negara IndonesiaProvinsiSumatera UtaraHa...

Nipple

This article is about the anatomical structure. For other uses, see Nipple (disambiguation). Part of the breast NippleA nipple, areola and breast of a female humanDetailsPart ofBreastIdentifiersLatinpapilla mammariaMeSHD009558TA98A16.0.02.004TA27105FMA67771Anatomical terminology[edit on Wikidata] The nipple is a raised region of tissue on the surface of the breast from which, in females, milk leaves the breast through the lactiferous ducts to breastfeed an infant.[1][2] Th...

William Holman Hunt

William Holman HuntSelf-portrait, 1867, Galleria degli Uffizi, FlorenceLahir(1827-04-02)2 April 1827Cheapside, LondonMeninggal7 September 1910(1910-09-07) (umur 83)Kensington, LondonKebangsaanInggrisPekerjaanPelukisTanda tangan William Holman Hunt OM (2 April 1827 – 7 September 1910) adalah seorang pelukis Inggris, dan salah satu pendiri Persaudaraan Pra-Raphael. Galeri Ilustrasi puisi My Beautiful Lady karya Thomas Woolner (1850) A Converted British Family Sheltering a Christian Miss...

Unione Sportiva Pistoiese 1926-1927

Questa voce sull'argomento stagioni delle società calcistiche italiane è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Voce principale: Unione Sportiva Pistoiese. Unione Sportiva PistoieseStagione 1926-1927Sport calcio Squadra Pistoiese Allenatore György Kőszegy Presidente Pindaro Polandri Prima Divisione3º posto nel girone C 1925-1926 1927-1928 Si invita a seguire il modello di voce Questa pa...

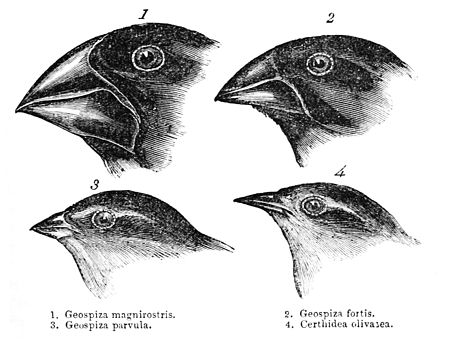
Evolutionary ideas of the Renaissance and Enlightenment

Changes in evolutionary philosophies Part of a series onEvolutionary biologyDarwin's finches by John Gould Index Introduction Main Outline Glossary Evidence History Processes and outcomes Population genetics Variation Diversity Mutation Natural selection Adaptation Polymorphism Genetic drift Gene flow Speciation Adaptive radiation Co-operation Coevolution Coextinction Divergence Convergence Parallel evolution Extinction Natural history Origin of life Common descent History of life Timeline of...

Mama (serial TV 2014)

MamaPoster promosiGenreDrama keluarga Melodrama RomansaDitulis olehYoo Yoon-kyungSutradaraKim Sang-hyupPemeranSong Yun-ahHong Jong-hyunJung Joon-hoMoon Jung-heeNegara asalKorea SelatanBahasa asliKoreaJmlh. episode24ProduksiProduser eksekutifKim Kyung-heeProduserKim Hee-yeolLokasi produksiKoreaDurasiSabtu dan Minggu pukul 21:45 (WSK)Rumah produksiPan EntertainmentRilis asliJaringanMunhwa Broadcasting CorporationRilis2 Agustus (2014-08-02) –19 Oktober 2014 (2014-10-19) Mama (H...

Sheila Reid

British actress (born 1937) For the track and field athlete, see Sheila Reid (athlete). This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Sheila Reid – news · newspapers · books · scholar · JSTOR ...

1967 French cantonal elections

Politics of France Constitutions Fifth Republic Declaration of the Rights of Man and of the Citizen Executive President (list) Emmanuel Macron (LREM) Prime Minister (list) Gabriel Attal (LREM) Government Attal Legislature National Assembly: Membership President: Yaël Braun-Pivet Senate President: Gérard Larcher Congress of the French Parliament Judiciary Constitutional Council Council of State Court of Cassation Court of Audit Cour de Justice de la République Administrative divisions Regi...

2022 in the United States

List of U.S Events in 2022 ← 2021 2020 2019 2022 in the United States → 2023 2024 2025 Decades: 2000s 2010s 2020s 2030s See also: History of the United States (2008–present) Timeline of United States history (2010–present) List of years in the United States 2022 in the United States2022 in U.S. states and territories States Alabama Alaska Arizona Arkansas California Colorado Connecticut Delaware Florida Georgia Hawaii Idaho Illinois Indiana Iowa Kansas Kentucky Louisiana Maine...

Robert Garcia (California politician)

American educator and politician (born 1977) Robert GarciaMember of the U.S. House of Representativesfrom California's 42nd districtIncumbentAssumed office January 3, 2023Preceded byKen Calvert (redistricted)28th Mayor of Long BeachIn officeJuly 15, 2014 – December 20, 2022Preceded byBob FosterSucceeded byRex RichardsonVice Mayor of Long BeachIn officeJuly 17, 2012 – July 15, 2014Preceded bySuja LowenthalSucceeded bySuja LowenthalMember of the Long Beach ...

Morton Feldman

American composer (1926–1987) Morton FeldmanFeldman in 1976Born(1926-01-12)January 12, 1926Queens, New York CityDiedSeptember 3, 1987(1987-09-03) (aged 61)Buffalo, New YorkWorksList of compositionsSpouseBarbara Monk Feldman (m.1987)Signature Morton Feldman (January 12, 1926 – September 3, 1987) was an American composer. A major figure in 20th-century classical music, Feldman was a pioneer of indeterminacy in music, a development associated with the experimental New York School of com...

Nicolas-Alexandre, marquis de Ségur

Nicolas-AlexandreImage of the marquis de SégurBorn1695FranceDied1755FranceOccupationBordeaux wine makerParentAlexandre de Ségur de Francs &Marie-Thérèse de Clausel Nicolas-Alexandre, marquis de Ségur (1695–1755) was a Bordeaux wine maker who during his lifetime was known as the Prince of Vines due to his ownership of some of the most famous Bordeaux chateaus-including Château Lafite, Château Latour, Château Mouton and Château Calon-Ségur. A hundred years after his death, the B...

Hidayat Jaelani

Biografi tokoh yang masih hidup ini tidak memiliki referensi atau sumber sehingga isinya tidak dapat dipastikan. Bantu memperbaiki artikel ini dengan menambahkan sumber tepercaya. Materi kontroversial atau trivial yang sumbernya tidak memadai atau tidak bisa dipercaya harus segera dihapus.Cari sumber: Hidayat Jaelani – berita · surat kabar · buku · cendekiawan · JSTOR (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Hidayat JaelaniPo...

دوقية ساكسونيا غوتا ألتنبورغ

دوقية ساكسونيا غوتا ألتنبورغ دوقية ساكسونيا غوتا ألتنبورغعلم دوقية ساكسونيا غوتا ألتنبورغشعار عاصمة غوتا نظام الحكم غير محدّد الانتماءات والعضوية الإمبراطورية الرومانية المقدسة التاريخ التأسيس 1680 النهاية 1826 تعديل مصدري - تعديل دوقية ساكسونيا غوتا ...

Guardian Bank Building

United States historic placeNew England BuildingU.S. National Register of Historic Places Show map of ClevelandShow map of OhioShow map of the United StatesLocation617–637 Euclid Ave., 614–626 Vincent Ave., Cleveland, OhioCoordinates41°30′1″N 81°41′20″W / 41.50028°N 81.68889°W / 41.50028; -81.68889Area1 acre (0.40 ha)Built1895ArchitectShepley, Rutan & Coolidge; Walker & WeeksArchitectural styleBeaux ArtsNRHP reference No.0000...

2016 European Athletics Championships – Women's long jump

2016 EuropeanAthletics ChampionshipsTrack events100 mmenwomen200 mmenwomen400 mmenwomen800 mmenwomen1500 mmenwomen5000 mmenwomen10,000 mmenwomen100 m hurdleswomen110 m hurdlesmen400 m hurdlesmenwomen3000 msteeplechasemenwomen4 × 100 m relaymenwomen4 × 400 m relaymenwomenRoad eventsHalf marathonmenwomenField eventsHigh jumpmenwomenPole vaultmenwomenLong jumpmenwomenTriple jumpmenwomenShot putmenwomenDiscus throwmenwomenHammer throwmenwomenJavelin throwmenwomenCombined eventsHeptathlonwomenD...

Digital cinema

Use of digital projectors in cinemas This article focuses on digital projection and distribution in cinemas. For digital image capture, see digital cinematography. Stadium seating rows closer to digital cinema screens offer significantly more immersive experiences. Digital cinema refers to the adoption of digital technology within the film industry to distribute or project motion pictures as opposed to the historical use of reels of motion picture film, such as 35 mm film. Whereas film r...

Vinci Malizon

/profillengkap.com/index.php/article/A Song of Ice and Fire fandom

/profillengkap.com/index.php/en/The Truth Is...

/profillengkap.com/index.php/article/MOST Bus

France 3 Limousin Poitou-Charentes

Alkitab bahasa Koptik

Ordo keagamaan

دوري النيجر الممتاز 2016–17

Marcell

Coyner Springs, Virginia

Galangan kapal

العلاقات اللبنانية الليبيرية