Knight's tour
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Kota Kovel bersama kota-kota kembarnya pada tahun 2009 Peta Ukraina Berikut ini adalah daftar tempat di Ukraina yang memiliki hubungan dengan komunitas daerah di negara lain. Hubungan ini disebut sebagai kota kembar. A Alchevsk[1][2][3] Dąbrowa Górnicza, Poland Dunaújváros, Hungary Feodosia, Ukraine Torez, Ukraine Alushta[4] Äänekoski, Finland Angarsk, Russia Capri, Italy Cassis, France Dubna, Russia Feodosia, Ukraine Georgiyevsk, Russia Krasnodon, Ukrain...

artikel ini perlu dirapikan agar memenuhi standar Wikipedia. Tidak ada alasan yang diberikan. Silakan kembangkan artikel ini semampu Anda. Merapikan artikel dapat dilakukan dengan wikifikasi atau membagi artikel ke paragraf-paragraf. Jika sudah dirapikan, silakan hapus templat ini. (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Hemotoraks Hemothoraks adalah kondisi yang terjadi ketika ada darah pada rongga pleura, yang terletak di antara dinding dada dan paru. Penumpukan ...

Isoprena Isoprena adalah nama umum (nama trivial) dari 2-metilbuta-1,3-diena. Senyawa ini biasa digunakan dalam industri, penyusun berbagai senyawa biologi penting, serta dapat berbahaya bagi lingkungan dan beracun bagi manusia bila terpapar secara berlebihan. Dalam suhu ruang isoprena berwujud cairan bening yang sangat mudah terbakar dan terpantik. Bila tercampur dengan udara sangat mudah meledak dan sangat reaktif bila dipanaskan. Pengangkutan isoprena memerlukan penanganan khusus. Secara i...

Universitas MelbourneArms of The University of MelbourneLatin: Universitas Melburnensiscode: la is deprecated MotoPostera Crescam LaudeWe grow in the esteem of future generationsJenisUniversitas negeriDidirikan1853Dana abadiA$1.129 billion (Desember 2009)KanselirAlex ChernovWakil KanselirGlyn DavisStaf akademik4,068 (2015)Jumlah mahasiswa45,411 (2015)Sarjana23,384 (2015)Magister18,417 (2015)LokasiParkville, Victoria, AustraliaKampusUrbanAfiliasiGroup of Eight, Universitas 21Situs webwww.uni...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (مارس 2020) هذه المقالة عن التغير المناخي والصناعة والمجتمع الأنظمة والقطاعات الصحة يتعرض البشر لآثار التغير المناخي بصورة مباشرة من خلال أنماط طقسية متقلبة (كدرجات الح...

Artikel ini membahas mengenai bangunan, struktur, infrastruktur, atau kawasan terencana yang sedang dibangun atau akan segera selesai. Informasi di halaman ini bisa berubah setiap saat (tidak jarang perubahan yang besar) seiring dengan penyelesaiannya. Fortune AraamesInformasi umumStatusDisetujuiLokasiDubai, Uni Emirat ArabPerkiraan rampung2008Data teknisJumlah lantai45Desain dan konstruksiArsitekDimensions Engineering ConsultantsPengembangNakheel Fortune Araames merupakan sebuah menara berti...

British actress and centenarian Dame Gwen Ffrangcon-DaviesDBEGwen Ffrangcon-Davies, on 27 June 1928BornGwen Lucy Ffrangcon-Davies(1891-01-25)25 January 1891London, EnglandDied27 January 1992(1992-01-27) (aged 101)Halstead, Essex, EnglandResting placeSt Peter and St Thomas Churchyard, Stambourne, Essex, EnglandNationalityBritishOccupationActressYears active1911–1991Known forActress and centenarian Dame Gwen Lucy Ffrangcon-Davies, DBE (25 January 1891 – 27 January 1992) was a...
2020年夏季奥林匹克运动会科索沃代表團科索沃国旗IOC編碼KOSNOC科索沃奧林匹克委員會網站www.noc-kosovo.org(英文)(阿爾巴尼亞文)(塞爾維亞文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員11參賽項目6个大项旗手开幕式:阿基爾·賈科瓦(英语:Akil Gjakova)和瑪琳達·開爾門蒂(柔道)[1]闭幕式�...

Earthquakes in 2021class=notpageimage| Approximate epicenters of the earthquakes in 2021 4.0–5.9 magnitude 6.0–6.9 magnitude 7.0–7.9 magnitude 8.0+ magnitude Strongest magnitude8.2 Mw United StatesDeadliest7.2 Mw Haiti 2,248 deathsTotal fatalities2,494Number by magnitude9.0+08.0–8.937.0–7.9166.0–6.91415.0–5.92,0464.0–4.914,643← 20202022 → This is a list of earthquakes in 2021. Only earthquakes of magnitude 6 or above are included, unless they resul...

Former Royal Air Force operations group No. 18 Group RAFRoyal Air Force EnsignActive1 April 1918 - 18 October 19191 September 1938 - 1 April 1996Country United KingdomBranch Royal Air ForceTypeRoyal Air Force groupPart ofRAF Coastal Command (1938 - 1969) RAF Strike Command (1969 - 1994)HeadquartersRAF Pitreavie CastleNorthwood HeadquartersMotto(s)Constant Endeavour[1]EngagementsWorld War I European theatre of World War II Battle of the Atlantic Military unit No. 18 Group (18...

Mexican theater of the Cold War, from 1964–1987 Mexican Dirty WarPart of the Cold War and Operation CondorMexican Army soldiers in the streets in 1968Date1964–1982[1][3]LocationMexicoResult Government victory Continued rule of the Institutional Revolutionary Party Most leftist guerrilla groups disbanded After the conflict Several acts of violence have not yet been clarified.[4] Political defeat of the PRI in the 2000 presidential elections before the National Actio...

Kronologi Alkitab adalah hasil penyusunan para pakar yang bermaksud mengkalibrasi berbagai silsilah dan catatan sejarah di dalam Alkitab Ibrani maupun Alkitab Kristen dengan sejarah umum. Sejumlah pakar Alkitab percaya bahwa dimungkinkan untuk mengurutkan kronologi tertentu sejarah umat manusia berdasarkan kepercayaan Yahudi dan Kristen.[1] Banyak pula peneliti yang menganggap hal itu sia-sia, misalnya David Long mengatakan bahwa upaya itu meletakkan dasar kreasionisme modern dengan m...

Municipality in State of Mexico, MexicoTezoyucaMunicipalityTezoyucaLocation in MexicoCoordinates: 19°45′11″N 99°11′15″W / 19.75306°N 99.18750°W / 19.75306; -99.18750Country MexicoStateState of MexicoMunicipal SeatTezoyucaArea • Total10.9 km2 (4.2 sq mi)Population (2005) • Total25,372Time zoneUTC-6 (Central Standard Time) • Summer (DST)UTC-5 (Central Daylight Time) Tezoyuca is a municipality in the...

Structure extending off of the Sun's surface Solar prominence seen in true color during totality of a Solar eclipse. In solar physics, a prominence, sometimes referred to as a filament,[a] is a large plasma and magnetic field structure extending outward from the Sun's surface, often in a loop shape. Prominences are anchored to the Sun's surface in the much brighter photosphere, and extend outwards into the solar corona. While the corona consists of extremely hot plasma, prominences co...

لمعانٍ أخرى، طالع الخربة (توضيح). قرية الخربة - قرية - تقسيم إداري البلد اليمن المحافظة محافظة صنعاء المديرية مديرية بني مطر العزلة عزلة ديان السكان التعداد السكاني 2004 السكان 380 • الذكور 213 • الإناث 167 • عدد الأسر 47 • عدد المساكن 34 معلوما�...

American public transit bus Motor vehicle GM New LookA GM New Look bus model T6H-5307N in service for the TTC (2008)OverviewManufacturerGM Truck and Bus (United States)GM Diesel Division (Canada)Production1959–1977 (U.S.)1962–1986 (Canada)[1]AssemblyPontiac, MichiganLondon, OntarioSaint-Eustache, QuebecBody and chassisClassTransit busBody styleMonocoque stressed-skinPowertrainEngine7.0 L (426 ci) Detroit Diesel 6V71 V6 diesel9.3 L (568 ci) Detroit Diesel 8V71 V8 die...

American college football season 1923 Washington Huskies footballRose Bowl, T 14–14 vs. NavyConferencePacific Coast ConferenceRecord10–1–1 (4–1 PCC)Head coachEnoch Bagshaw (3rd season)CaptainWayne HallHome stadiumHusky StadiumSeasons← 19221924 → 1923 Pacific Coast Conference football standings vte Conf Overall Team W L T W L T California $ 5 – 0 – 0 9 – 0 – 1 Washington^ 4 – 1 – 0 10 –...

1999–2000 concert tour by Bruce Springsteen and the E Street Band This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help improve this article by introducing more precise citations. (April 2024) (Learn how and when to remove this message) Bruce Springsteen and the E Street Band Reunion TourTour by Bruce Springsteen and the E Street BandStart dateApril 9, 1999End dateJuly 1, 2000Legs3No. of ...
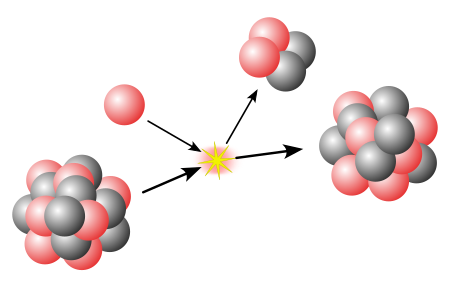
Vật lý hạt nhân Nucleus • Nucleons (Proton, Neutron) • Lực hạt nhân • Phản ứng hạt nhân Mô hình hạt nhânGiọt chất lỏng • Mô hình vỏ hạt nhân • Mô hình boson tương tácPhương pháp theo nguyên lý đầu Phân loại hạt nhânĐồng vị – bằng ZIsobars – bằng NĐồng neutron – bằng NIsodiapher – bằng N − Z Đồng phân – b�...

Seventh book of the Bible This article is about the biblical book. For other uses, see Judge (disambiguation). Judges in the Hebrew Bibleשופטים Italics indicate individuals not explicitly described as judges Book of Exodus Moses Book of Joshua Joshua Book of Judges Othniel Ehud Shamgar Deborah Gideon Abimelech Tola Jair Jephthah Ibzan Elon Abdon Samson First Book of Samuel Eli Samuel vte Tanakh (Judaism) Torah (Instruction)GenesisBereshitExodusShemotLeviticusWayiqraNumbersBe...

















