Darrieus–Landau instability
|
Read other articles:

The Einhorn–Brunner reaction is the designation for the chemical reaction of imides with alkyl hydrazines to form an isomeric mixture of 1,2,4-triazoles. It was initially described by the German chemist Alfred Einhorn in a paper, published in 1905, describing N-methylol compounds of amides.[1] In 1914 chemist Karl Brunner published a paper expanding on Einhorn's research of the reaction pictured below, thus resulting in the naming as the Einhorn-Brunner.[2] Substituted 1,2,4...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Februari 2023. KSAT-2, juga dikenal sebagai Hayato-2 adalah satelit Jepang dibangun oleh Kagoshima University sebagai penerbangan misi KSAT nya. Memiliki ukuran 100x100x100mm (tanpa diperpanjang antena boom) dan dibangun di sekitar standar 1U bus cubesat. Payload sa...

Former Electronics Company Not to be confused with the later company also founded by Clive Sinclair, Sinclair Research. This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article's lead section may be too short to adequately summarize the key points. Please consider expanding the lead to provide an accessible overview of all important aspects of the article. (October 2012) This article ...

Gravity Probe B КА «Gravity Probe B» в представлении художника Заказчик НАСА Производитель Lockheed Martin Оператор Стэнфордский университет[1] Задачи проверка эффектов ОТО Спутник Земли Стартовая площадка база Ванденберг Ракета-носитель Дельта-2 Запуск 20 апреля 2004 16:57:26 UTC COSPAR ID 2004-014A SCN ...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. Hu terukir dalam nisan dari Turki era Ottoman. Hu atau Huwa adalah nama Allah dalam Sufisme. Secara harafiah berarti Dia.[1] Dalam Sufisme Hu adalah istilah lain yang digunakan untuk Allah, dan digunakan sebagai nama Allah. Allah Hu berarti All...

History of Indian state of Bihar The Maurya Empire with capital at Patliputra spanned across the Indian subcontinent as well as over parts of modern-day Iran and modern-day Myanmar. History of South Asia Outline Palaeolithic (2,500,000–250,000 BC) Madrasian culture Soanian culture Neolithic (10,800–3300 BC) Bhirrana culture (7570–6200 BC) Mehrgarh culture (7000–3300 BC) Edakkal culture (5000–3000 BC) Chalcolithic (3500–1500 BC) Anarta tradition (c. 3950–1900 BC) Ahar-Banas...

Chronologies Publicité pour les supermarchés Casino vers 1966.Données clés 1963 1964 1965 1966 1967 1968 1969Décennies :1930 1940 1950 1960 1970 1980 1990Siècles :XVIIIe XIXe XXe XXIe XXIIeMillénaires :-Ier Ier IIe IIIe Chronologies géographiques Afrique Afrique du Sud, Algérie, Angola, Bénin, Botswana, Burkina Faso, Burundi, Cameroun, Cap-Vert, République centrafricaine, Comores, République du Congo, République dém...

Tempat perdagangan Kanton sekitar tahun 1850 Suasana di Tiongkok (1852, p.Vignette)[1] Sistem Kanton (1757–1842) berfungsi sebagai sarana bagi Tiongkok untuk mengendalikan perdagangan dengan barat dalam negaranya sendiri dengan memusatkan semua perdagangan di pelabuhan selatan di Kanton (kini Guangzhou). Dikenal dalam bahasa Tionghoa sebagai Yī kǒu tōng shāng (一口通商, Sistem perdagangan pelabuhan tunggal), kebijakan ini muncul pada tahun 1757 sebagai tanggapan terhadap apa...

Il duca di Parma Antonio Farnese, 1720 Ilario Giacinto Mercanti detto lo Spolverini (Parma, 13 gennaio 1657 – Parma, 4 agosto 1734) è stato un pittore italiano. Indice 1 Biografia 2 Opere 3 Note 4 Bibliografia 5 Voci correlate 6 Altri progetti 7 Collegamenti esterni Biografia Fu il migliore allievo di Francesco Monti e «non altrimenti che il suo maestro, si acquistò nome dipingendo battaglie né so se per esagerazione o per verità solea dirsi che i soldati del Monti minacciavano e quei ...

Place in North West, South AfricaVentersdorpVentersdorpShow map of North West (South African province)VentersdorpShow map of South AfricaVentersdorpShow map of AfricaCoordinates: 26°19′S 26°49′E / 26.317°S 26.817°E / -26.317; 26.817CountrySouth AfricaProvinceNorth WestDistrictDr Kenneth KaundaMunicipalityJB MarksEstablished1866Area[1] • Total53.88 km2 (20.80 sq mi)Population (2011)[1] • Total4,204 ...

Politics of Ivory Coast Constitution Human rights Government President Alassane Ouattara Vice President Tiémoko Meyliet Koné Prime Minister Robert Beugré Mambé Government Robert Beugré Mambé government Parliament National Assembly Speaker: Guillaume Soro Senate Speaker: Jeannot Ahoussou-Kouadio (TBC) Administrative divisions Districts Regions Departments Sub-prefectures Communes Villages Elections Recent elections Presidential: 20202025 Parliamentary: 20162021 Senatorial: 2018 Political...

Kerumunan di sekitar trem di Pitt St, 1949 Park Street di Sydney, New South Wales, Australia adalah sebuah jalan lintas besar di pusat CBD. Membentang dari George St di barat ke College St di timur, dan menjadi William St. Jalan ini membelah Hyde Park. Park Street terkenal karena menjadi kantor pusat perusahaan media terbesar di Australia, Publishing and Broadcasting Limited. Sebuah jaringan trem listrik pernah beroperasi di Park St antara Elizabeth dan College St. Ditutup tahun 1960. lbsJala...

1995 single by Bone Thugs-n-Harmony This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 1st of tha Month – news · newspapers · books · scholar · JSTOR (May 2007) (Learn how and when to remove this message) 1st of tha MonthSingle by Bone Thugs-n-Harmonyfrom the album E. 1999 Eternal B-sideDie Die DieReleasedAugus...

German World War II submarine U-250 being commissioned, 12 December 1943 History Nazi Germany NameU-250 Ordered5 June 1941 BuilderGermaniawerft, Kiel Yard number684 Laid down9 January 1943 Launched11 November 1943 Commissioned12 December 1943 Fate Sunk on 30 July 1944[1] Refloated by Soviet Union on 14 September 1944[1] Notes60°27′54″N 28°24′54″E / 60.46500°N 28.41500°E / 60.46500; 28.41500 Soviet Union NameTS-14 Acquired14 September 1944 Fa...
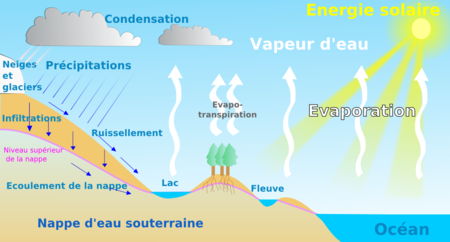
Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (septembre 2017). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références ». En pratique : Quelles sources sont attendues ?...

Cyrillic letter Cyrillic letter Je with beltPhonetic usage:[jɪ̞], [ɪ̞]The Cyrillic scriptSlavic lettersАА̀А̂А̄ӒБВГҐДЂЃЕЀЕ̄Е̂ЁЄЖЗЗ́ЅИІЇꙆЍИ̂ӢЙЈКЛЉМНЊОО̀О̂ŌӦПРСС́ТЋЌУУ̀У̂ӮЎӰФХЦЧЏШЩꙎЪЪ̀ЫЬѢЭЮЮ̀ЯЯ̀Non-Slavic lettersӐА̊А̃Ӓ̄ӔӘӘ́Ә̃ӚВ̌ԜГ̑Г̇Г̣Г̌Г̂Г̆Г̈г̊ҔҒӺҒ̌ғ̊ӶД́Д̌Д̈Д̣Д̆ӖЕ̃Ё̄Є̈ԐԐ̈ҖӜӁЖ̣ҘӞЗ̌З̣З̆ӠИ̃ӤҊҚӃҠҞҜК̣к̊қ̊ԚᴫЛ́ӅԮ�...

Para otros usos del término atocha, véase Atocha (desambiguación). Real Basílica de Nuestra Señora de Atocha Fachada de la Real Basílica de Nuestra Señora de AtochaDatos generalesTipo BasílicaEstilo arquitectura herrerianaLocalización Madrid (España)Coordenadas 40°24′20″N 3°41′01″O / 40.4055, -3.6837Construcción 1951Culto Iglesia católicaDiócesis Archidiócesis de MadridNombrado por Virgen de AtochaArquitecto Diego Méndez[editar datos en Wikidata&...

City in Israel City in IsraelPetah Tikva פֶּתַח תִּקְוָהCity (from 1937)Hebrew transcription(s) • Also spelledPetah Tiqwa (official)Petach Tikva, Petach Tikvah (unofficial) Emblem of Petah TikvaPetah TikvaShow map of Central IsraelPetah TikvaShow map of IsraelCoordinates: 32°05′20″N 34°53′11″E / 32.08889°N 34.88639°E / 32.08889; 34.88639Grid position139/166 PALCountry IsraelDistrictCentralFounded1878; ...

Cet article est une ébauche concernant une localité argentine. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Villa Ibáñez Héraldique Administration Pays Argentine Province San Juan Département Département d'Ullum Maire Alfredo Simón Ortiz Code postal J5409 Indicatif téléphonique 0264 Démographie Population 3 720 hab. (2001) Géographie Coordonnées 31° 27′ 52″ sud, 68°...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Reet Petite – news · newspapers · books · scholar · JSTOR (May 2014) (Learn how and when to remove this message) 1957 single by Jackie WilsonReet PetitePicture cover of 1986 reissueSingle by Jackie Wilsonfrom the album He's So Fine ReleasedAugust 1957Length2:40...











![{\displaystyle {\frac {\sigma }{S_{L}k}}={\frac {r}{r+1}}\left[{\sqrt {1+\left({\frac {r^{2}-1}{r}}\right)\left(1-{\frac {g}{S_{L}^{2}rk}}\right)}}-1\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9e0e12fc12867386ab41c5277214527d944a20a7)























