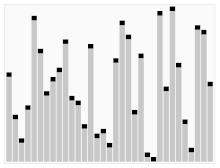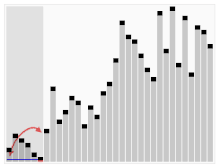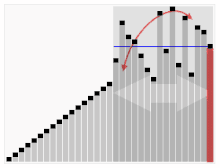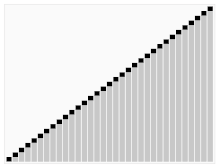
Comparison sort
|
Read other articles:

Irises, Vincent van Gogh Bunga-bunga Iris Vincent van Gogh, 1889 Cat minyak di atas kanvas, 71×93 cm (28×37 in) J. Paul Getty Museum, Los Angeles, California Bunga-bunga Iris adalah nama sebuah lukisan karya pelukis Vincent van Gogh. Merupakan satu dari sekian karyanya ketika ia berada di R.S. Jiwa Saint Paul-de-Mausole di Saint-Rémy-de-Provence, Prancis pada saat-saat terakhir menjelang kematiannya tahun 1890. Lukisan tersebut kemungkinan dipengaruhi oleh lukisan kayu Jepang, sepert...

Abraham Pietersen van DeursenBornbefore November 11, 1607HaarlemDiedc. 1670 (age 63)Known forCouncil of twelve menSpouseTryntie Melchior Abrahams (1611-1678)Parent(s)Pieter van Deursen (c1575-?) Maria or Paulina Vincke (c1575-?)RelativesMartin Van Buren, 3rd great-grandfather Abraham Pietersen van Deursen (before November 11, 1607 – c. 1670), aka Abraham Pietersen van Deusen, was an immigrant from Holland who settled in New Amsterdam and become one of the Council of 12 that was the fir...

Artikel ini sebagian besar atau seluruhnya berasal dari satu sumber. Diskusi terkait dapat dibaca pada the halaman pembicaraan. Tolong bantu untuk memperbaiki artikel ini dengan menambahkan rujukan ke sumber lain yang tepercaya. Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Februari 2023. Universitas MikroskilL...

العلاقات السعودية الفلبينية الفلبين السعودية تعديل مصدري - تعديل العلاقات السعودية الفلبينية تشير إلى العلاقات الثنائية بين الفلبين و المملكة العربية السعودية. أقيمت علاقات دبلوماسية رسمية بين البلدين في 24 أكتوبر 1969.[1] مقارنة بين البلدين مقارنة عام�...

County in South Hwanghae Province, North KoreaChaeryŏng County 재령군CountyKorean transcription(s) • Hanja載寧郡 • McCune-ReischauerChaeryŏng-gun • Revised RomanizationJaeryeong-gunCountryNorth KoreaProvinceSouth Hwanghae ProvinceAdministrative divisions1 ŭp, 1 rodongjagu, 24 riArea • Total327.7 km2 (126.5 sq mi)Population (2008[1]) • Total125,631 • Density380/km2 (990/sq mi)...

French entry in the Eurovision Song Contest 1979 Je suis l'enfant soleilSingle by Anne-Marie DavidB-sideJust Like Loving YouReleased1979GenreChansonLength3:00LabelPolydorComposer(s)Hubert GiraudLyricist(s)Eddy MarnayAnne-Marie David singles chronology Neşeli Gençleriz (1976) Je suis l'enfant soleil (1979) Trop (1979) Eurovision Song Contest 1979 entryCountryFranceArtist(s)Anne-Marie DavidLanguageFrenchConductorGuy MattéoniFinals performanceFinal result3rdFinal points106Entry chronology◄ ...

Pour les articles homonymes, voir Hobbit (homonymie). Le HobbitUn voyage inattendu Données clés Titre original The Hobbit: An Unexpected Journey Réalisation Peter Jackson Scénario Peter JacksonFran WalshPhilippa BoyensGuillermo del Toro Musique Howard Shore Acteurs principaux Martin FreemanIan McKellenRichard ArmitageAndy SerkisManu Bennett Sociétés de production New Line CinemaMetro-Goldwyn-MayerWingNut Films Pays de production États-Unis Nouvelle-Zélande Genre Fantasy Durée 169 mi...

Church in Corfu, GreeceCathedral of Saint Jacob and Saint ChristopherCathedral of Saint James and Saint Christopher, CorfuLocationCorfuCountryGreeceAdministrationDioceseRoman Catholic Archdiocese of Corfu, Zakynthos and Cephalonia (since 1553) Cathedral of Saint Jacob and Saint Christopher (Greek: Ιερός Καθολικός Μητροπολιτικός Ναός (Duomo) and (Greek: Καθεδρικός Ναός Αγίου Χριστοφόρου και Ιακώβου Κέρκυρας)) is th...

Questa voce o sezione sull'argomento album pop non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Rebel Heartalbum in studioArtistaMadonna Pubblicazione10 marzo 2015(Vedi date di pubblicazione) Durata55:06 (standard)74:26 (deluxe) Tracce14 (standard)19 (deluxe)25 (super deluxe) GenerePop[1] Etichetta...
2020年夏季奥林匹克运动会科索沃代表團科索沃国旗IOC編碼KOSNOC科索沃奧林匹克委員會網站www.noc-kosovo.org(英文)(阿爾巴尼亞文)(塞爾維亞文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員11參賽項目6个大项旗手开幕式:阿基爾·賈科瓦(英语:Akil Gjakova)和瑪琳達·開爾門蒂(柔道)[1]闭幕式�...

Keith SilversteinNama lainDavid Roach, Darin BugPekerjaanPengisi suaraTahun aktif1999–sekarangAgenDean Panero TalentSuami/istriRosemary Do (m. 2010)Anak3Situs webwww.keithsilverstein.com Keith Silverstein adalah pengisi suara Amerika, yang dikenal karena mengisi suaranya ke versi bahasa Inggris dari anime dan video game Jepang, yang berafiliasi dengan Bang Zoom! Entertainment, Viz Media, Studiopolis dan Funimation. Dia terkenal karena perannya sebag...

Former political party in the Dutch East Indies Indonesian Islamic Party Partai Islam IndonesiaAbbreviationPIILeaderSoekiman WirjosandjojoFounded4 December 1938 (1938-12-04)Dissolvedc. March 1942 (1942-03)Split fromIndonesian Islamic Union Party (PSII)IdeologyIslamic socialismNational affiliationIndonesian Political Federation (GAPI) The Indonesian Islamic Party (Indonesian: Partai Islam Indonesia, PII) was an Islamic political party in the Dutch East Indies (now...

Musée Paul et Alexandra KanellopoulosInformations généralesSite web (el + en) pacf.gr/enLocalisationLocalisation dème des Athéniens GrèceCoordonnées 37° 58′ 22″ N, 23° 43′ 33″ Emodifier - modifier le code - modifier Wikidata Le musée Paul et Alexandra Kanellopoulos (grec moderne : Μουσείο Παύλου και Αλεξάνδρας Κανελλοπούλου) est un musée d'antiquités situé à Athènes, en Grèce....

Disambiguazione – Se stai cercando altri significati, vedi Ossidiana (disambigua). OssidianaLipari - affioramento di ossidiana. È ben osservabile la struttura vetrosa di tipo fluidale.CategoriaRoccia magmatica SottocategoriaRoccia magmatica effusiva Composizione chimicasilicatica Minerali principaliplagioclasio, anfiboli, pirosseni Minerali accessoriolivina Strutturaamorfo Tessituraamorfo L'ossidiana è un vetro vulcanico la cui formazione è dovuta al rapidissimo raffreddamento della lav...

English musician For the organist, see David Patrick Gedge. This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentia...

US intelligence agency in charge of satellite intelligence National Reconnaissance OfficeNRO headquarters at nightAgency overviewFormedEstablished: August 25, 1960 (1960-08-25)Declassified: September 18, 1992 (1992-09-18)JurisdictionUnited StatesHeadquartersChantilly, Virginia, U.S.MottoSupra Et Ultra(Above And Beyond)Annual budgetClassifiedAgency executiveChristopher Scolese, Director[1]Troy Meink, Principal Deputy Director[2]Major General Christ...

This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources.Find sources: Rodobrana – news · newspapers · books · scholar · JSTOR (February 2021) This article includes a list of references, related reading, or external links, but its sources remain unclear because it lacks inline citations. Please help improve this article ...

Partai Tindakan Rakyat Nama dalam bahasa InggrisPeople's Action PartyNama dalam bahasa MelayuParti Tindakan RakyatNama dalam bahasa Mandarin人民行动党Rénmín XíngdòngdǎngNama dalam bahasa Tamilமக்கள் செயல் கட்சிMakkaḷ Ceyal KaṭciSingkatanPAPKetua umumGan Kim YongSekretaris JenderalLee Hsien LoongWakil KetuaMasagos ZulkifliAsisten Sekretaris JenderalHeng Swee KeatChan Chun SingPendiriLee Kuan YewLim Chin SiongS. RajaratnamToh Chin ChyeLim Kim SanDib...

1997 FIFA Confederations Cup1997 السعودية1997 FIFA Confederations Cup official logoTournament detailsHost countrySaudi ArabiaCityRiyadhDates12–21 DecemberTeams8 (from 6 confederations)Venue(s)1 (in 1 host city)Final positionsChampions Brazil (1st title)Runners-up AustraliaThird place Czech RepublicFourth place UruguayTournament statisticsMatches played16Goals scored52 (3.25 per match)Attendance333,500 (20,844 per match)Top scorer(s) Rom�...

إسيلورالشعارمعلومات عامةالشعار النصي Mieux voir le monde (بالفرنسية) البلد فرنسا التأسيس 1972[1] النوع منظمة الشكل القانوني شركة بأسهم مبسطة[2] المقر الرئيسي شارنتون لو بونت موقع الويب essilor.com المنظومة الاقتصاديةالشركة الأم EssilorLuxottica (en) الشركات التابعة Essilor (Germany) (en) Foster Grant ...