Primzahlencousin
|
Read other articles:

American media group and website This article is about the animal-focused media brand. For the satirical newspaper, see The Dodo (magazine). The DodoType of siteMedia brandAvailable inEnglishCreated byKerry Lauerman, Izzie LererParentVox MediaURLwww.thedodo.com LaunchedJanuary 2014; 10 years ago (2014-01)Current statusActive The Dodo is an American media brand and digital publisher focused on telling animals' stories. The Dodo is headquartered in New York...

У этого термина существуют и другие значения, см. Великий инквизитор (значения). Великий Инквизиторлат. Inquisitor Generalis Должность Появилась 1483 Первый Томас де Торквемада Последний Херонимо Кастельон-и-Салас Упразднена 1820 Великий инквизитор (лат. Inquisitor Generalis) — глава и...

La Governance della Rai è l'amministrazione della società concessionaria del servizio radiotelevisivo pubblico italiano, la Rai appunto, che include in particolare il Consiglio di amministrazione, che tipicamente nomina i direttori di rete e gli altri direttori editoriali. Tale gestione è stata oggetto di vari interventi legislativi, anche a seguito di numerose pronunce della Corte Costituzionale. La giurisprudenza della Consulta ha infatti riconosciuto che il servizio radiotelevisivo pubb...

First election of Scott Walker as Governor of Wisconsin 2010 Wisconsin gubernatorial election ← 2006 November 2, 2010 2012 (recall) → Turnout49.7% Nominee Scott Walker Tom Barrett Party Republican Democratic Running mate Rebecca Kleefisch Tom Nelson Popular vote 1,128,941 1,004,303 Percentage 52.3% 46.5% County results Precinct resultsWalker: 40–50% 50–60% 60–...

A-road in Yorkshire, England This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: A658 road – news · newspapers · books · scholar · JSTOR (March 2022) (Learn how and when to remove this message) A658The A658 dual carriageway plus a pedestrian walkway runs under the main runway at Leeds Bradford International Airp...

2005 film by Tetsuya Nomura Final Fantasy VII: Advent ChildrenJapanese theatrical release posterDirected byTetsuya NomuraWritten byKazushige NojimaProduced by Yoshinori Kitase Shinji Hashimoto Ichiro Hazama Starring Takahiro Sakurai Ayumi Ito Kenichi Suzumura Showtaro Morikubo Maaya Sakamoto Toshiyuki Morikawa Shōgo Suzuki CinematographyYasuharu YoshizawaEdited byKeiichi KojimaMusic byNobuo UematsuProductioncompanyVisual WorksDistributed bySquare Enix HoldingsRelease dates September 14,...

Связи между Синоптическими Евангелиями Синопти́ческие Ева́нгелия (др.-греч. συνοπτικός, букв. «со-наблюдающий» от σύν, «вместе» и ὄψις, «видение, зрительное восприятие») — три первые книги Нового Завета (Евангелия от Матфея, Марка и Луки). По своему содержанию синоптич...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Pour les articles homonymes, voir Ardachir et Ardachès. Ardachir III Drachme à l'effigie d'Ardachir III, frappé à Arrajan (en) en 629 Titre Empereur sassanide 6 septembre 628 – 27 avril 630(1 an, 7 mois et 21 jours) Prédécesseur Kavadh II Successeur Schahr-Barâz Biographie Dynastie Sassanides Date de naissance 621 Date de décès 27 avril 630 Lieu de décès Ctésiphon (Assuristan) Père Kavadh II Mère Anzoï ou Boré la « Romaine » modifier Ar...

Untuk kegunaan lain, lihat ATA. Allen Telescope ArrayNama alternatifATA Dinamakan sesuaiPaul Allen Bagian dariHat Creek Radio Observatory LokasiCalifornia, Pacific States RegionKoordinat40°49′04″N 121°28′24″W / 40.8178°N 121.4733°W / 40.8178; -121.4733Koordinat: 40°49′04″N 121°28′24″W / 40.8178°N 121.4733°W / 40.8178; -121.4733 OrganisasiRadio Astronomy LaboratorySETI Institute Ketinggian986&...

The UsedInformasi latar belakangAsalOrem, Utah, Amerika SerikatGenrePost hardcore[1] Emo[2] Screamo[3][4]Tahun aktif2001 - sekarangLabelRepriseArtis terkaitStrange Itch Dumb Luck Good Charlotte New Transit Direction RancidSitus webTheUsed.netAnggotaBert McCrackenQuinn AllmanJepha HowardDan WhitesidesMantan anggotaBranden Steineckert Justin Shekoski & Bert McCracken 2015 The Used merupakan sebuah grup musik yang berasal dari Orem, Utah. Anggotanya berjumlah ...

Ecumenical Patriarch of Constantinople from 1043 to 1059 Patriarch Michael I redirects here. For other uses, see Patriarch Michael I of Alexandria and Patriarch Michael I of Antioch. Michael I CerulariusEcumenical Patriarch of ConstantinopleThe enthronement of Michael I Cerularius, from the Madrid SkylitzesSeeConstantinopleInstalled1043Term ended21 January 1059PredecessorAlexius I StuditesSuccessorConstantine III LichoudasPersonal detailsBornMichael Keroulariosc. 1000Constantinople, Byzantine...
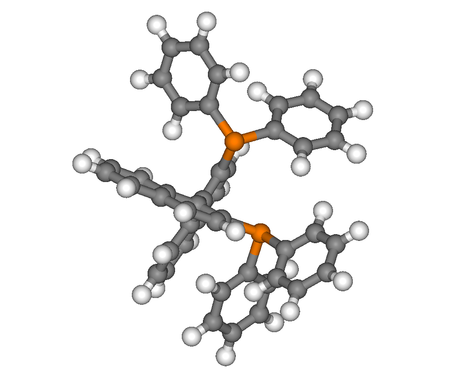
BINAP Names Preferred IUPAC name ([1,1′-Binaphthalene]-2,2′-diyl)bis(diphenylphosphane) Other names BINAP Identifiers CAS Number 76189-55-4 (R) Y76189-56-5 (S) Y 3D model (JSmol) (Rac): Interactive image ChEMBL ChEMBL1275990 ChemSpider 551072 (Rac) N ECHA InfoCard 100.114.880 EC Number 616-304-7 619-338-0 616-305-2 918-620-3 PubChem CID 634876 (+) UNII 4F1X2F8NA3 (R) YOX12238KWH (S) Y CompTox Dashboard (EPA) DTXSID40913327 InChI...

River in New York, United StatesMuddy KillModder KillMuddy Kill flowing through a horse farm just north of NY 17KLocationCountryUnited StatesStateNew YorkCountyOrangeTownMontgomeryPhysical characteristicsSource • locationUnnamed pond off Albany Post Road • coordinates41°34′04″N 74°13′31″W / 41.56778°N 74.22528°W / 41.56778; -74.22528 • elevation400 ft (120 m) MouthWallkill River ̶...

Untuk bahasan lebih luas tentang topik ini, lihat Pemanduan. Seorang pandu di Nigeria sedang membantu sebuah kapal milik Angkatan Laut Amerika Serikat untuk masuk ke pelabuhan di Lagos dengan menggunakan peta laut Pandu maritim, pandu laut, pandu pelabuhan, pandu kapal, atau pandu, adalah seorang pelaut yang bertugas memandu kapal untuk dapat melalui perairan yang berbahaya atau ramai, seperti pelabuhan atau muara sungai. Pandu maritim umumnya dianggap sebagai ahli di bidang navigasi, karena ...

Disused railway station in Ash Green, Guildford Ash Green HaltThe site of the station in 1990General informationLocationAsh Green, GuildfordEnglandGrid referenceSU902501Platforms2Other informationStatusDisusedHistoryPre-groupingLondon and South Western RailwayPost-groupingSouthern RailwayKey dates8 October 1849[1][2]Station opens as AshDecember 1876Station renamed Ash GreenSeptember 1891Station renamed Ash1 October 1895Station renamed Ash Green1 December 1926Station renamed As...

Arthur Wichmann dan anggota ekspedisi. Ekspedisi Papua Utara (1903) dilakukan di pantai utara Papua yang saat itu belum dikenal untuk mencari lapisan batubara yang dapat dimanfaatkan. Ekspedisi Papua Utara diluncurkan oleh Maatschappij ter Bevordering van het Natuurkundig Onderzoek der Nederlandsche Koloniën yang berkantor pusat di Amsterdam dan dipimpin oleh geolog Carl Ernst Arthur Wichmann, sehingga Ekspedisi Papua Utara dikenal pula sebagai Ekspedisi Wichmann. Di samping C.E.A. Wichmann,...

Indoor arena in Montreal Bell Center and Molson Centre redirect here. For the arena in Barrie, Ontario formerly known as the Barrie Molson Centre, see Sadlon Arena. For other uses, see Bell Center (disambiguation). Not to be confused with Place Bell in nearby Laval, Quebec. Bell CentreCentre Bell (French)Bell Centre in 2017Bell CentreLocation in MontrealShow map of MontrealBell CentreLocation in QuebecShow map of QuebecBell CentreLocation in CanadaShow map of CanadaFormer namesNew Montre...

Bodyguard of the Pope Not to be confused with Swiss Armed Forces. For the Swiss soldiers who have served as guards, see Swiss Guards. Pontifical Swiss GuardPontificia Cohors Helvetica (Latin)Guardia Svizzera Pontificia (Italian)Päpstliche Schweizergarde (German)Garde suisse pontificale (French)Guardia svizra papala (Romansh)Current banner of the Pontifical Swiss Guard of Pope Francis under the command of Christoph Graf[1]Active1506–15271548–17981800–180...

Sheridan leads the charge at Five Forks (Frederick Phisterer, 1912) The American Civil War saw extensive use of horse-mounted soldiers on both sides of the conflict. They were vital to both the Union Army and Confederate Army for conducting reconnaissance missions to locate the enemy and determine their strength and movement, and for screening friendly units from being discovered by the enemy's reconnaissance efforts. Other missions carried out by cavalry included raiding behind enemy lines,...












