Supertoroid
|
Read other articles:

Nigerian media executive Nduka ObaigbenaNduka Obaigbena at his 60th Birthday thanksgiving at the Cathedral Church of Christ, LagosBorn(1959-07-14)14 July 1959Ibadan, Western Region, NigeriaNationalityNigerian Place of Birth: Igbanke in Edo StateOrganization(s)Leaders and Company Limited, Delta StateKnown forPublisher, ThisDay Newspaper, Chairman, Arise News Channel Nduka Obaigbena CON, Duke of Owa Kingdom is the founding Chairman & Editor-in-Chief of the THISDAY Media Group and ARISE...

.gb البلد المملكة المتحدة تعديل مصدري - تعديل gb. هو نطاق إنترنت من صِنف مستوى النطاقات العُليا في ترميز الدول والمناطق، للمواقع التي تنتمي إلى المملكة المتحدة وكان يرمز إلى بريطانيا ولم يعد يستخدم.[1][2] مراجع ^ النطاق الأعلى في ترميز الدولة (بالإنجليزية). ORSN [ال�...

City in California, United States City in California, United StatesPlymouthCityMain Street in PlymouthNickname: Gateway to Shenandoah ValleyPlymouthLocation in CaliforniaShow map of CaliforniaPlymouthPlymouth (the United States)Show map of the United StatesCoordinates: 38°28′55″N 120°50′41″W / 38.48194°N 120.84472°W / 38.48194; -120.84472Country United StatesState CaliforniaCountyAmadorSettled1853IncorporatedFebruary 8, 1917[1]Governm...

1st-century AD Roman senator, orator and poet Silius Italicus Tiberius Catius Asconius Silius Italicus[1] (Latin: [ˈsiːlijʊs ɪˈt̪alɪkʊs], c. 26 – c. 101 AD) was a Roman senator, orator and epic poet of the Silver Age of Latin literature. His only surviving work is the 17-book Punica, an epic poem about the Second Punic War and the longest surviving poem in Classical Latin at over 12,000 lines. A painting by Joseph Wright of Derby depicting Silius Italicus at the tomb...

Tan Sri Abdul Samad bin Ismail (18 April 1924 – 4 September 2008), who often went by the moniker Pak Samad, was a Malaysian journalist, writer and editor. He was also one of the founding members of the Peoples Action Party in Singapore Early life Samad was born on 18 April 1924, in Singapore to Javanese immigrant parents,[1] where he also attended Victoria School.[2] He completed a Senior Cambridge certificate as a cub reporter at the daily newspaper, Utusan Me...

Television series This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Hardcastle and McCormick – news · newspapers · books · scholar · JSTOR (March 2023) (Learn how and when to remove this message) Hardcastle and McCormickTitle screen from season 1 and from the last ten episodes of season 2GenreCrime dramaAction...

Javi Martínez Martínez di DenmarkInformasi pribadiNama lengkap Javier Martínez AginagaTanggal lahir 2 September 1988 (umur 35)Tempat lahir Estella, SpanyolTinggi 189 cm (6 ft 2+1⁄2 in)Posisi bermain GelandangInformasi klubKlub saat ini Qatar SCNomor 8Karier junior1993–1995 Berceo1995–1997 Logroñés CD Arenas2000 Izarra2001–2005 OsasunaKarier senior*Tahun Tim Tampil (Gol)2005–2006 Osasuna B 32 (3)2006–2012 Athletic Bilbao 201 (22)2012–2021 FC Bayern M...

Austrian-born engineer (1849–1936) William de la BarreDe la Barre c. 1885Born(1849-04-15)April 15, 1849DiedMarch 24, 1936(1936-03-24) (aged 86)Known forrevolutionizing flour milling William de la Barre (April 15, 1849, in Vienna – March 24, 1936, in Minneapolis) was an Austrian Empire-born civil engineer who developed a new process for milling wheat into flour, using energy-saving steel rollers at the Washburn-Crosby Mills (now known as General Mills, Inc.) in Minneapo...

Cet article est une ébauche concernant une localité italienne et le Piémont. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Elva Église Santa Maria Assunta Administration Pays Italie Région Piémont Province Coni Code postal 12020 Code ISTAT 004083 Code cadastral D401 Préfixe tel. 00171 Démographie Gentilé elvesi Population 102 hab. (31-12-2010[1]) Densité 3,9 hab./km2 Géographie...

关于与「友谊勋章 (俄罗斯)」標題相近或相同的条目页,請見「友谊勋章 (消歧义)」。 友谊勋章类型单级勋章(仅设有一个等级)授予原因加强各民族友谊、交流与合作国家/地区俄罗斯 颁发单位 俄羅斯颁授资格俄罗斯国民及世界各民族人民設立時間1994年3月2日[1]首次颁发康斯坦丁·蒂托夫(萨马拉州州长)绶带 优先顺序上等荣誉勋章下等光荣父母�...

Protein-coding gene in humans IRAK2Available structuresPDBOrtholog search: PDBe RCSB List of PDB id codes3MOPIdentifiersAliasesIRAK2, IRAK-2, interleukin 1 receptor associated kinase 2External IDsOMIM: 603304 MGI: 2429603 HomoloGene: 1207 GeneCards: IRAK2 Gene location (Human)Chr.Chromosome 3 (human)[1]Band3p25.3Start10,164,919 bp[1]End10,243,745 bp[1]Gene location (Mouse)Chr.Chromosome 6 (mouse)[2]Band6 E3|6 52.82 cMStart113,615,428 bp[2]End113,67...

Antony Zeidra Abidin Wakil Gubernur Jambi ke-4Masa jabatan2005–2010GubernurZulkifli NurdinPendahuluUteng SuryadiatnaHasip Kalimuddin SyamPenggantiFachrori UmarAnggota DPR-RI Fraksi GolkarMasa jabatan2001 – 2004Pengganti Antar WaktuPendahuluUsman ErmulanPenggantiPetahanaDaerah pemilihanJambi Informasi pribadiLahir15 Oktober 1951 (umur 72)Bangko, Merangin, Keresidenan JambiKebangsaanIndonesiaPartai politikGolkarAnakPinto JayanegaraAlma materUniversitas IndonesiaProfesiPeng...

American soccer player For the United States Navy officer, see James H. Sands. James Sands Sands with Rangers in 2022Personal informationFull name James Hoban Sands[1]Date of birth (2000-07-06) July 6, 2000 (age 23)Place of birth Rye, New York, United StatesHeight 6 ft 1 in (1.86 m)Position(s) Center-back, defensive midfielderTeam informationCurrent team New York City FCNumber 6Youth career2010–2015 New York Soccer Club2015–2017 New York City FCSenior career*Ye...
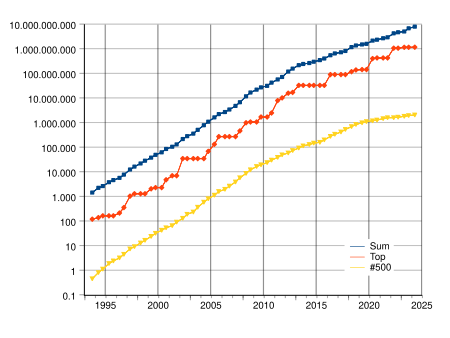
توب 500 الموقع الرسمي الموقع الرسمي تعديل مصدري - تعديل القدرة الإجمالية الحسابية لـ500 نظام حاسوب الأكثر قوة في العالم. مشروع توب500 (بالإنجليزية: TOP500) يرتب ويصف أنظمة الحاسوب (غير الموزعة) الخمس مائة الأقوى المعروفة عالميا. وقد بدأ المشروع في عام 1993 وينشر قائمة محدثة �...

Extension to the Poincaré group In mathematical physics, the conformal symmetry of spacetime is expressed by an extension of the Poincaré group, known as the conformal group. The extension includes special conformal transformations and dilations. In three spatial plus one time dimensions, conformal symmetry has 15 degrees of freedom: ten for the Poincaré group, four for special conformal transformations, and one for a dilation. Harry Bateman and Ebenezer Cunningham were the first to study ...

Questa voce sull'argomento calciatori francesi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Bryan ConstantNazionalità Francia Altezza183 cm Peso76 kg Calcio RuoloCentrocampista Squadra Villefranche Saint-Jean Beaulieu CarrieraGiovanili 2009-2013 Nizza Squadre di club1 2012-2015 Nizza 238 (6)2013-2016 Nizza5 (0)2016→ Fréjus St-Raphaël9 (1)2016-2017 Nizza 21 (0)2017...

Weather event in the United States Tornado outbreak of November 27–28, 2005Tornado damage near Plumerville, AR from November 27, 2005 TypeTornado outbreakDurationNovember 27 – 28, 2005 Tornadoesconfirmed73Max. rating1F3 tornadoDuration oftornado outbreak229 hours, 42 minutes Fatalities2 fatalities, 15 injuriesDamage$7.2 million [1]Areas affectedKansas, Missouri, Arkansas, Louisiana, Mississippi, Alabama1Most severe tornado damage; see Fujita scale2Time from first tornado to la...

Martial arts gym based in Deerfield Beach, Florida Kill Cliff Fight ClubEst.2017; 7 years ago (2017)Founded byHenri HooftGreg JonesPrimary trainersHenri HooftGreg JonesJason StroutKami BarziniCorey PeacockPast titleholdersVitor BelfortMichael ChandlerRashad EvansRobbie LawlerLuke RockholdLogan StorleyKamaru UsmanAung La NsangJason JacksonTraining facilitiesDeerfield Beach, Florida, U.S.WebsiteOfficial Website Kill Cliff FC, officially known as Kill Cliff Fight Club (formerly...

秦家住宅(京都市下京区油小路通仏光寺下る太子山町)「表屋造り」の町家で店舗棟は明治2年(1869年)頃の建築。京都市登録有形文化財。[1] 野口家住宅(京都市中京区油小路通錦小路下る藤本町)元治元年(1864年)の大火後に再建された。京都市指定有形文化財。[2] 重要伝統的建造物群保存地区の祇園新橋通(西方向を見る) 京町家(きょうまちや)と�...

English rock band (1963–1996) For their eponymous album, see Kinks (album). For other uses, see Kink. The KinksOriginal lineup in 1965From left: Pete Quaife, Dave Davies, Ray Davies and Mick AvoryBackground informationAlso known as The Ray Davies Quartet (1962–1963) The Ramrods (1963) The Pete Quaife Band (1963) The Bo-Weevils (1964) The Ravens (1963–1964) OriginLondon, EnglandGenresRockpopYears active1963–1997LabelsPyeCameoRepriseRCAAristaLondonMCAColumbiaKochGuardianUniversalPast me...



![{\displaystyle {\begin{array}{rcccl}-(a+1)&\leq &x&\leq &+(a+1)\\[4pt]-(b+1)&\leq &y&\leq &+(b+1)\\[4pt]-1&\leq &z&\leq &+1\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7e4db39e01a3ff09d456b3ac8c42d225960bc033)
![{\displaystyle {\begin{array}{rcccl}-(a-1)&\leq &x&\leq &+(a-1)\\[4pt]-(b-1)&\leq &y&\leq &+(b-1)\\[4pt]-\infty &\leq &z&\leq &+\infty \end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3d67df3a70f19f0122a783db1cfd52338e881be3)


![{\displaystyle {\begin{array}{rcccl}a-1&\leq &x&\leq &a+1\\[4pt]-1&\leq &z&\leq &+1\end{array}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/17fd3355afbc39e8e2157f9d017d0c02935db963)