Liouville's theorem (differential algebra)
|
Read other articles:

Halaman ini berisi artikel tentang istri Kaisar Nikolai I. Untuk Permaisuri Rusia yang dibunuh bersama keluarganya pada Revolusi Bolshevik, lihat Aleksandra Fyodorovna (istri Nikolai II). Aleksandra FyodorovnaАлекса́ндра ФёдоровнаLukisan Aleksandra oleh George Dawe, 1826.Permaisuri Kaisar RusiaPeriode1 Desember 1825 – 2 Maret 1855Penobatan3 September 1826Informasi pribadiKelahiranFriederike Luise Charlotte Wilhelmine(1798-07-13)13 Juli 1798Berlin, PrusiaKematian1 Novembe...

Untuk film Tamil tahun 1985 bernama sama, lihat Padikkadavan (film 1985) PadikkadavanSutradaraSurajProduserBharathi ReddyDitulis olehSurajPemeranDhanushTamannaahVivekAtul KulkarniPenata musikMani SharmaSinematograferA. VenkateshPenyuntingManojPerusahaanproduksiVijaya ProductionsDistributorSun PicturesTanggal rilis 14 Januari 2009 (2009-01-14) Durasi160 menitNegaraIndiaBahasaTamilPendapatankotor₹88 crore Padikkadavan (Indonesia: Pria Tak Terdidik) adalah sebuah film aksi-komedi be...

Об экономическом термине см. Первородный грех (экономика). ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Ран�...

Rogers Cup 2011Tanggal8 – 14 AgustusEdisike-1222010 Juara[[ Kesalahan ekspresi: Kata "rogers" tidak dikenal. 2011 – Putra|Tunggal Putra]] Andy MurrayWomen's singles Caroline WozniackiMen's doubles Bob Bryan / Mike BryanWomen's doubles Gisela Dulko / Flavia Pennetta ← 2010 ·Rogers Cup· 2012 → Canada Masters 2011 (diketahui sebagai 2011 Rogers Cup presented by National Bank dan 2011 Rogers Cup karena alasan sponsor) adalah turnamen tenis profesion...
Positive effect from the perceived value of a person For other uses, see Pride (disambiguation). Part of a series onEmotions Affect Classification In animals Emotional intelligence Mood Regulation Interpersonal Dysregulation Valence Emotions Acceptance Admiration Affection Amusement Anger Angst Anguish Annoyance Anticipation Anxiety Apathy Arousal Awe Belongingness Boredom Confidence Confusion Contempt Contentment Courage Curiosity Depression Desire Determination Disappointment Disgust Distru...

« Recensement » redirige ici. Pour les autres significations, voir Recensement (homonymie). Le recensement (du latin recensere, « passer en revue ») est une opération statistique de dénombrement d'une population. Les recensements démographiques existent depuis l'Antiquité (Chine, Égypte, Hébreux que la Bible mentionne à plusieurs reprises ; Rome), mais leur signification ainsi que leurs méthodes ont évolué. Ils ne sont mis en œuvre de façon systémati...

Defunct airline of Taiwan (1951–2016) For the defunct Kazakhstan airline, see Trans-Asia. Not to be confused with Transavia or AirAsia. TransAsia Airways復興航空 IATA ICAO Callsign GE TNA TRANSASIA Founded21 May 1951 (1951-05-21)(as Foshing Airlines)Commenced operations1 January 1992 (1992-01-01)(as TransAsia Airways)Ceased operations22 November 2016 (2016-11-22)HubsKaohsiungTaipei–SongshanTaipei–TaoyuanFrequent-flyer programLegend Flight...

Huruf KirilZhe dengan breve Penggunaan Fonetis:[d͡ʒ]Alfabet KirilHuruf SlaviaАА́А̀А̂А̄ӒБВГҐДЂЃЕЕ́ÈЕ̂ЁЄЖЗЗ́ЅИИ́ЍИ̂ЙІЇЈКЛЉМНЊОŌПРСС́ТЋЌУУ́ У̀У̂ӮЎФХЦЧЏШЩЪЫЬЭЮЯHuruf non-SlaviaӐА̊А̃Ӓ̄ӔӘӘ́Ә̃ӚВ̌ҒГ̑Г̣Г̌ҔӺҒ̌ӶД̌Д̣Д̆ӖЕ̄Е̃Ё̄Є̈ӁҖӜҘӞЗ̌З̱З̣ԐԐ̈ӠӢИ̃ҊӤҚӃҠҞҜК̣ԚӅԮԒӍӉҢԨӇҤО́О̀О̆О̂О̃ӦӦ̄ӨӨ̄Ө́Ө̆ӪҨԤР̌ҎҪС̣С̱Т̌Т̣ҬУ̃Ӱ Ӱ́�...

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、�...

Eric Foner Eric Foner (New York, 7 febbraio 1943) è uno storico statunitense. Ha scritto numerose opere circa le vicende politiche degli Stati Uniti, sulla storia del concetto di libertà, sugli inizi del Partito Repubblicano, oltre a lavori incentrati sulle biografie di noti afroamericani e sull'Era della Ricostruzione, ed è un membro della Columbia University dal 1982. È inoltre autore di svariati libri di testo universitari utilizzati negli Stati Uniti. Foner è uno dei principali stori...

Belief or behavior involving uncritical zeal or an obsessive enthusiasm For other uses, see Fanatic (disambiguation). The Fanatics of Tangier by Eugène Delacroix, Minneapolis Institute of Arts Fanaticism (from the Latin adverb fānāticē [fren-fānāticus; enthusiastic, ecstatic; raging, fanatical, furious][1]) is a belief or behavior involving uncritical zeal or an obsessive enthusiasm. Definitions Philosopher George Santayana defines fanaticism as redoubling your effort when you h...

Group of protists with at least one whip-like appendage Not to be confused with flagellation, fellate, or ciliate. This article is about eukaryotic flagellates. For bacterial flagella and their differences, see Flagellum. Flagellata from Ernst Haeckel's Artforms of Nature, 1904 Parasitic Excavata (Giardia lamblia) Green algae (Chlamydomonas) A flagellate is a cell or organism with one or more whip-like appendages called flagella. The word flagellate also describes a particular construction (o...

Overview of solar power in the U.S. state of Michigan Red-tailed hawk at University of Michigan NCRC solar array Solar power in Michigan has been growing in recent years due to new technological improvements, falling solar prices and a variety of regulatory actions and financial incentives. The largest solar farm in Michigan is Assembly Solar, completed in 2022, which has 347 MW of capacity. Small-scale solar provided 50% of Michigan solar electricity as recently as 2020 but multiple solar fa...

Illustrazione che raffigura il Sole al centro di un universo circolare, dal Dizionario Enciclopedico Brockhaus ed Efron (1890-1907) L'eliocentrismo (dal greco antico ἥλιος?, hḕlios, sole e κέντρον, kèntron, centro) è una teoria astronomica che pone il Sole al centro del sistema solare, con i pianeti che gli girano intorno. Storicamente, nell'eliocentrismo il Sole era ritenuto centro del cosmo, termine con cui si designava l'insieme degli astri noti, prima dell'introd...
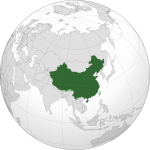
English that is influenced by a Chinese language This article is about Chinese English. For the play, see Chinglish (play). ChinglishA 2007 warning sign in Guilin states in Chinese: (When there are) thunderstorms / Please do not climb the mountain.Native toPRC and ROCRegionEast AsiaLanguage familyIndo-European GermanicWest GermanicIngvaeonicAnglo–FrisianAnglicEnglishChinglishEarly formsOld English Middle English Early Modern English Writing systemLatin (English alphabet)Language codesI...

Cet article est une ébauche concernant la philosophie. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Première de couverture de la Kritik der reinen Vernunft, 1781 Le criticisme, ou « philosophie critique », promeut l'idée que toute véritable recherche philosophique « consiste à commencer par analyser les fondements, l'étendue légitime et les limites de notre connaissance »[1]. La...

La sirenettaAriel (Halle Bailey) in una scena del filmTitolo originaleThe Little Mermaid Lingua originaleinglese Paese di produzioneStati Uniti d'America Anno2023 Durata135 min Rapporto2,39:1 Generefantastico, musicale, sentimentale RegiaRob Marshall Soggettodal film di Ron Clements e John Muskerdalla fiaba di Hans Christian Andersen SceneggiaturaDavid Magee ProduttoreRob Marshall, John DeLuca, Marc Platt, Lin-Manuel Miranda Produttore esecutivoJeffrey Silver Casa di produzioneWalt Di...

38th season of FIA Formula One motor racing This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 1984 Formula One World Championship – news · newspapers · books · scholar · JSTOR (October 2019) (Learn how and when to remove this message) 1984 FIA Formula OneWorld Championship Drivers' Champion: Niki LaudaConstruc...

Close-bodied gown or robe à l'anglaise of purple and white striped silk, French, 1785-90, LACMA, M.2007.211.931 A close-bodied gown, English nightgown, or robe à l'anglaise was a women's fashion of the 18th century. Like the earlier mantua, from which it evolved,[1] the back of the gown featured pleats from the shoulder, stitched down to mould the gown closely to the body until the fullness was released into the skirt. Through the 1770s, the back pleats became narrower and closer to...

Stefano Vecchia Thông tin cá nhânTên đầy đủ Stefano Giuseppe ArneVecchia HolmquistNgày sinh 23 tháng 1, 1995 (29 tuổi)Nơi sinh Thụy ĐiểnChiều cao 1,82 m (5 ft 11+1⁄2 in)Vị trí Tiền đạo / Tiền vệThông tin độiĐội hiện nay IK SiriusSố áo 22Sự nghiệp cầu thủ trẻNăm Đội0000–2002 Vasalunds IF2002–2013 IF BrommapojkarnaSự nghiệp cầu thủ chuyên nghiệp*Năm Đội ST (BT)2013–2016 IF Brommapojkarna 4...

































