Line-item veto in the United States

In United States government, the line-item veto, or partial veto, is the power of an executive authority to nullify or cancel specific provisions of a bill, usually a budget appropriations bill, without vetoing the entire legislative package. The line-item vetoes are usually subject to the possibility of legislative override as are traditional vetoes.

Governors

Forty-four of the 50 U.S. states give their governors some form of line-item veto power; Indiana, Nevada, New Hampshire, North Carolina, Rhode Island, and Vermont are the exceptions.[1] The Mayor of Washington, D.C., also has this power.[2][3]

Wisconsin

The Governor of Wisconsin is empowered with a sweeping line-item veto. Wisconsin governors have the power to strike out words, numbers, and even entire sentences from appropriations bills.[4]

According to scholars, Wisconsin has used four types of extraordinary partial vetoes.[5] The first, the "digit veto", was first used by Governor Patrick Lucey in 1973. In appropriation for $25 million, he vetoed the digit 2, resulting in an appropriation of $5 million.[5] Just two years later, Lucey introduced the "editing veto". In this instance, the word "not" was removed in the phrase "not less than 50 percent", thus resulting in the opposite effect than desired by the legislature.[6] In 1983, an even more extreme version, the "pick-a-letter" or "Vanna White veto" was introduced. Governor Anthony Earl edited a 121-word, five-sentence paragraph down to a one-sentence, 22-word paragraph to change an appeals process from the courts to the Public Service Commission. The final version, the "reduction veto", was introduced in 1993 by Governor Tommy Thompson. This resulted in a legislatively-appropriated amount being reduced arbitrarily by the governor.[5] This unprecedented usage has resulted in eight lawsuits and numerous amendment proposals. In the first lawsuit, State ex. rel. Wisconsin Telephone Co. v. Henry, the Wisconsin Supreme Court recognized the absolute partial veto power of the Governor as long as a workable, complete law remained, stating the governor had "the right to pass independently on every separable piece of legislation in an appropriation bill."[7]

In his first two terms as governor, Thompson used 1,500 line-item vetoes to cancel a total of $150 million in spending; none of these vetoes were overridden.[4] The only judicial limitation was Risser v. Klauser, which prohibited the "reduction veto", stating that "the constitution prohibits a write-in veto of monetary figures which are not appropriation amounts."[8] In 1990, a constitutional amendment was passed abolishing the "Vanna White veto," which allowed the governor to strike individual letters within words to create new words.[5] Yet, in 2011, Governor Scott Walker controversially crossed out 116 words in a pension-related section of the budget bill.[9]

In 2023, Governor Tony Evers used a line-item veto to extend what was supposed to be a two year temporary funding increase for schools to last over 400 years.[10]

Confederate States

Article 1, Section 7 of the Constitution of the Confederate States, adopted just before the start of the American Civil War, would have granted the President of the Confederate States the ability to "approve any appropriation and disapprove any other appropriation in the same bill," with such disapprovals returned to the Houses of Congress for reconsideration and potentially for override.[11]

Line-Item Veto Act of 1996

Main article: Line Item Veto Act of 1996
Clinton signing cancellation letters related to his Line-Item Vetoes for the Balanced Budget Act of 1997, August 11, 1997.

Presidents of the United States have repeatedly asked Congress to give them line-item veto power.[12] According to Louis Fisher in The Politics of Shared Power, Ronald Reagan said to Congress in his 1984 State of the Union address, "Tonight I ask you to give me what forty-three governors have: Give me a line-item veto this year. Give me the authority to veto waste, and I'll take the responsibility, I'll make the cut, I'll take the heat." Bill Clinton echoed the request in his State of the Union address in 1995.[13] Congress granted this power to the president by the Line Item Veto Act of 1996 to control "pork barrel spending", but in 1998 the U.S. Supreme Court ruled the act to be unconstitutional in a 6–3 decision in Clinton v. City of New York. The court found that exercise of the line-item veto is tantamount to a unilateral amendment or repeal by the executive of only parts of statutes authorizing federal spending, and therefore violated the Presentment Clause of the United States Constitution. Thus a federal line-item veto, at least in this particular formulation, would only be possible through a constitutional amendment. Prior to that ruling, President Clinton applied the line-item veto to the federal budget 82 times.[14][15]

Subsequent developments

Though the Supreme Court struck down the Line-Item Veto Act in 1998, President George W. Bush asked Congress to enact legislation that would return the line-item veto power to the Executive Authority. First announcing his intent to seek such legislation in his January 31, 2006, State of the Union address, President Bush sent a legislative proposal, the Legislative Line-Item Veto Act of 2006, to the Congress on March 6, 2006, urging its prompt passage. Senators Bill Frist (R-TN) and John McCain (R-AZ), and Republican Whip Senator Mitch McConnell (R-KY) jointly introduced this proposal. Representative Paul Ryan (R-WI) introduced his own version, the Legislative Line Item Veto Act of 2006, in March of that year.[16]

On that same day, Joshua Bolten, the Director of the Office of Management and Budget, gave a press conference on the President’s line-item veto proposal. Bolten explained that the proposed Act would give the President the ability to single out "wasteful" spending and to put such spending on hold. While the spending line-item is on hold, the President can send legislation to Congress to withdraw the particular line-item. The proposal would then be considered in both houses within ten days on an up or down basis and could be passed by a simple majority. Additionally, such proposals could not be filibustered.

When asked how this proposed legislation was different from the 1996 Line-Item Veto Act that the United States Supreme Court had declared illegal, Bolten said that whereas the former act granted unilateral authority to the Executive to disallow specific spending line items, the new proposal would seek Congressional approval of such line-item vetoes. Thus, for the President to successfully withdraw previously enacted spending, a simple majority of Congress is required to agree to specific legislation to that effect.

Though the 2006 line-item veto proposal was much weaker than the 1996 version, it nevertheless failed to find strong support in Congress. Senator Robert C. Byrd of West Virginia, called it "an offensive slap at Congress", asserting that the legislation would enable the President to intimidate individual members of any Congress by targeting the projects of his political opponents. He also complained that the line-item veto as proposed would take away Congress’s constitutional "power of the purse" and give it to the executive branch.

On June 8, 2006, Viet D. Dinh, Professor of Law at Georgetown University Law Center, and Nathan Sales, John M. Olin Fellow at Georgetown University Law Center, testified by written statement before the House Committee on the Budget on the constitutional issues in connection with the proposed legislation. Dinh and Sales argued that the Legislative Line Item Veto Act of 2006 satisfies the Constitution's Bicameralism and Presentment Clause, and therefore avoids the constitutional issues raised in the 1996 Act struck down by the Supreme Court. They also stated that the proposed Act is consistent with the basic principle that grants Congress broad discretion to establish procedures to govern its internal operations.

H.R. 4890, the Legislative Line-Item Veto Act, was approved by the House Budget Committee on June 14, 2006, by a vote of 24–9. It was approved in the full House on June 22. A similar version was included in the "Stop Over Spending Act of 2006",[17] authored by Senator Judd Gregg, in the Senate and approved by the Senate Budget Committee, but the full Senate failed to approve it, thereby preventing the Legislative Line-Item Veto Act from becoming law.[16]

Line Item Veto Re-Enactment Activity of 2009

In 2009, Senators Russ Feingold (D-WI) and John McCain introduced legislation of a limited version of the line-item veto. This bill would give the president the power to withdraw earmarks in new bills by sending the bill back to Congress minus the line-item vetoed earmark. Congress would then vote on the line-item vetoed bill with a majority vote under fast track rules to make any deadlines the bill had.[18][19][20] This bill was not passed.[21]

Debate

Some scholars, such as Louis Fisher, believe the line-item veto would give presidents too much power over government spending compared with the power of the Congress.[22][23]

See also

References

  1. ^ Duhon, Clara (July 6, 2023). "What is a line-item veto? What you need to know". The Hill. Retrieved July 2, 2024.
  2. ^ District of Columbia Home Rule Act (Pub. L. 93–198, 87 Stat. 777, enacted December 24, 1973)
  3. ^ Smith, Gregory. "R.I. Gov. Lincoln Chafee says governors in 46 states have line-item veto authority". Politifact. Providence Journal. Retrieved February 26, 2015.
  4. ^ a b Apple, R.W., Jr. "Line-Item Veto Would Begin Voyage Into a Vast Unknown", New York Times, March 27, 1995.
  5. ^ a b c d Radatz, Clark G. (January 2004). The Partial Veto in Wisconsin (PDF). Wisconsin Legislative Reference Bureau. Archived from the original (PDF) on January 17, 2013. Retrieved November 14, 2012.
  6. ^ Sabato, Larry J. (2007). A More Perfect Constitution. New York: Walker & Company. pp. 284–285. ISBN 978-0-8027-1621-7.
  7. ^ State ex. rel. Wisconsin Telephone Co. v. Henry, 218 Wis. 302, 314-315 (Wis. 1935).
  8. ^ Risser v. Klauser, 207 Wis. 2d 176, 191 (Wis. 1997).
  9. ^ Rothschild, Matthew (July 6, 2011). "Gov. Walker Uses "Vanna White Veto" to Rob New Public Sector Workers". The Progressive. Archived from the original on January 7, 2017. Retrieved November 14, 2012.
  10. ^ Griffiths, Brent D. "Wisconsin Gov. Tony Evers thwarted Republicans with a creative veto that increases school funding for the next 400 years". Business Insider.
  11. ^ "Constitution of the Confederate States; March 11, 1861". Avalon Project.
  12. ^ Madison, Lucy (August 10, 2012). "15 years after its brief existence, line-item veto eludes presidents". Political Hotsheet. CBS News. Retrieved August 16, 2012.
  13. ^ "Address Before a Joint Session of the Congress on the State of the Union". Transcript. The American Presidency Project. January 24, 1995. Retrieved October 4, 2013.
  14. ^ "Supreme Court Strikes Down Line-Item Veto". CNN. June 25, 1998. Archived from the original on October 8, 2008.
  15. ^ "History of Line Item Veto Notices". National Archives and Records Administration. Archived from the original on February 4, 2012. Retrieved February 1, 2006.
  16. ^ a b 109th Congress (2006) (March 7, 2006). "H.R. 4890 (109th)". Legislation. GovTrack.us. Retrieved October 15, 2018. Legislative Line Item Veto Act of 2006{{cite web}}: CS1 maint: numeric names: authors list (link)
  17. ^ S.3521 Stop Over Spending Act of 2006
  18. ^ Archived at Ghostarchive and the Wayback Machine: "Feingold, McCain, Ryan Introduce Line-item Veto to Curb Wasteful Spending". YouTube. Video of reintroduction of Line Item Veto Bill March 4, 2009
  19. ^ "Feingold, McCain, Ryan Introduce Line-item Veto to Curb Wasteful Spending". United States Senate. March 4, 2009. Archived from the original on January 5, 2011.
  20. ^ "Briefing by White House Press Secretary Robert Gibbs". whitehouse.gov. February 25, 2009 – via National Archives.
  21. ^ H.R. 1294 (111th) on GovTrack.us: : Congressional Accountability and Line-Item Veto Act of 2009
  22. ^ Michael G. Locklar, Is the 1996 Line Item Veto Constitutional?, 34 Hous. L. Rev. 1161 (1997); Louis Fisher, State Techniques to Blunt the Governor's Item-Veto Power (1996) (CRS Report No. 96-996 GOV) (listing the tactics used in states "to counteract, blunt, or neutralize the governor's item-veto power"); Legislative Line-Item Veto Proposals: Hearing Before the Senate Comm. on the Budget, 103rd Cong. 60 (1994) (statement of Louis Fisher); Richard Briffault, The Item Veto in State Courts, 66 Temple L. Rev. 1171, 1181 (1993) (describing how legislative control over the definition of "item" has eroded the power of governors who have the line-item veto); Louis Fisher & Neal Devins, How Successfully Can the States' Item Veto be Transferred to the President?, 75 Geo. L. J. 159 (1986).
  23. ^ Curry, James A.; Riley, Richard B.; Battistoni, Richard M.: Constitutional Government", Kendall Hunt, 2009, pg. 146

Read other articles:

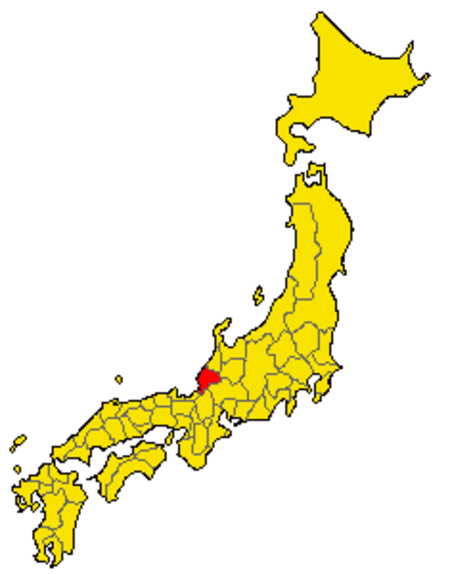
Provinsi Echizen

Provinsi Echizen (越前国code: ja is deprecated , echizen no kuni) adalah nama provinsi lama Jepang yang sekarang merupakan wilayah bagian timur prefektur Fukui. Echizen terkenal sebagai sentra produksi Washi, literatur tahun 774 sudah mencatat tentang pembuatan washi di Echizen. Washi terus menjadi produk andalan Echizen hingga sekarang. Echizen juga terkenal dengan produksi keramik yang bernilai seni tinggi dan merupakan salah satu dari enam pusat pembakaran keramik terkenal di Jepang be...

 

John Bird Sumner

Archbishop of Canterbury; Bishop of Chester; British Anglican bishop The Most Reverend and Right HonourableJohn Bird Sumner[1]Archbishop of CanterburyPortrait by Margaret Sarah CarpenterChurchChurch of EnglandDioceseCanterburyIn office1848–1862PredecessorWilliam HowleySuccessorCharles LongleyOther post(s)Bishop of Chester (1828–1848)OrdersOrdination1803Consecration1828Personal detailsBorn(1780-02-25)25 February 1780Kenilworth, Warwickshire, EnglandDied6 September 1862(1862-09...

 

F1000 (publisher)

Academic publisher For other uses, see F1000 (disambiguation). F1000Parent companyTaylor & Francis GroupFounded2000FounderVitek TraczCountry of originUnited KingdomHeadquarters locationLondonDistributionWorldwideKey peopleRebecca Lawrence (Managing Director)Publication typesReviews, scientific articles, posters, slidesNonfiction topicsScienceOfficial websitef1000.com F1000 (formerly Faculty of 1000) is an open research publisher for scientists, scholars, and clinical researchers. F1000 of...

Nemona

Pokémon Champion Not to be confused with Nimona. Fictional character NemonaPokémon characterNemona in Pokémon Scarlet and VioletFirst appearancePokémon Scarlet and Violet (2022)Voiced by English Yolis Arroyo (TV series)Olivia Vidas (ONA) Japanese Eri Kitamura (TV series)Eriko Matsui (ONA) Nemona, known in Japan as Nemo, (Japanese: ネモ) is a character in the 2022 video games Pokémon Scarlet and Violet. She is both a rival to the protagonist and the Champion of the Paldean region. She i...

 

Халкидонское христианство

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

 

سباق طواف فرنسا 1955

سباق طواف فرنسا 1955 الاسم سباق طواف فرنسا 1955 التاريخ 7-30 يوليو 1955 التاريخ بداية:7 يوليو 1955  نهاية:30 يوليو 1955  عدد المراحل 22 عدد الرياضيين 130 (نقطة البداية)،  و69 (نقطة النهاية)  المسافة 4495 الزمن 130 ساعة و29 دقيقة و26 ثانية البلد فرنسا بلجيكا سويسرا موناكو لوكسمبورغ  نقط�...

List of lynching victims in the United States

This article may be too long to read and navigate comfortably. Consider splitting content into sub-articles, condensing it, or adding subheadings. Please discuss this issue on the article's talk page. (January 2022) This list is incomplete; you can help by adding missing items. (December 2018) Two Mexican-American men, Francisco Arias and José Chamales, lynched in Santa Cruz, California, in 1877 The lynching of Frank McManus in Minneapolis, Minnesota for rape in 1882 This is a list of lynch...

 

俄亥俄州

  「俄亥俄」重定向至此。关于其他用法，请见「俄亥俄 (消歧义)」。 俄亥俄州 美國联邦州State of Ohio 州旗州徽綽號：七葉果之州地图中高亮部分为俄亥俄州坐标：38°27'N－41°58'N, 80°32'W－84°49'W国家 美國加入聯邦1803年3月1日，在1953年8月7日追溯頒定（第17个加入联邦）首府哥倫布（及最大城市）政府 • 州长（英语：List of Governors of {{{Name}}}]]） •&...

 

Ohio Department of Public Safety

Department of Public SafetyDepartment overviewHeadquartersCharles D. Shipley Building1970 West Broad StreetColumbus, OhioDepartment executiveTom Stickrath, Director of Public SafetyWebsitepublicsafety.ohio.gov The ODPS and ODOT campus in Columbus's Hilltop neighborhood The Ohio Department of Public Safety (ODPS) is the administrative department of the Ohio state government[1] responsible for the protection and safety of residents and visitors. The Department of Public Safety's headqua...

National Register of Historic Places listings in Wood County, Texas

Location of Wood County in Texas This is a list of the National Register of Historic Places listings in Wood County, Texas. This is intended to be a complete list of properties and districts listed on the National Register of Historic Places in Wood County, Texas. There are one district and nine individual properties listed on the National Register in the county. Two individually listed properties are Recorded Texas Historic Landmarks while the district contains more.       &...

 

Volvo T 24-25

En Volvo T 25. Volvo T 24 / T 25 är en traktor från Volvo. T 24 hade motor för fotogen, T 25 för bensin. I övrigt är de lika. Traktorn tillverkades mellan 1953 och 1959, och var en vidareutveckling av Volvo T 22-23. T24 tillverkades i ett antal av 9825 exemplar och T25 i 6 445 exemplar[1]. Andra utföranden Under åren 1953-1955 tillverkades den i grönmålat BM-utförande med Austinmotor med modellbeteckningarna 200 Teddy i bensinutförande och 210 Teddy i fotogenutförande i 2 400 exe...

 

Tuzkol, Raiymbek District

Lake in Kazakhstan For the lake in Pavlodar Region, see Tuzkol, Bayanaul District. TuzkolТұзкөлView of the lakeTuzkolLocationKegen DepressionCoordinates43°00′N 79°58′E / 43.000°N 79.967°E / 43.000; 79.967Typesalt lakeBasin countriesKazakhstanMax. length5.7 kilometers (3.5 mi)Max. width2.1 kilometers (1.3 mi)Surface area6.6 square kilometers (2.5 sq mi)Max. depth3 meters (9.8 ft)Residence timeUTC+6Shore length116.6 kilome...

哈萨克斯坦总统

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言，清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日（哈薩克蘇維埃社會主義共和國總統） 哈萨克斯坦 哈萨克斯坦政府...

 

روسات

روسات   تاريخ الإطلاق 1 يونيو 1990[1]  المكوك الحامل دلتا 2[1]  تاريخ الانحلال 23 أكتوبر 2011[2][3]  تعديل مصدري - تعديل   روسات أو مرصد روسات الفضائي في الفلك (بالإنجليزية :ROSAT) هو قمر صناعي لقياس الأشعة السينية الآتية من بعض الأجرام السماوية. بلغ وزن القمر ...

 

جائحة فيروس كورونا في منطقة إيلوكس 2020

هذه مقالة غير مراجعة. ينبغي أن يزال هذا القالب بعد أن يراجعها محرر؛ إذا لزم الأمر فيجب أن توسم المقالة بقوالب الصيانة المناسبة. يمكن أيضاً تقديم طلب لمراجعة المقالة في الصفحة المخصصة لذلك. (يونيو 2020) هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة و�...

Uroš Knežević

Serbian painter (1811–1876) Uroš KneževićA portrait of Knežević by Jovan Popović, 1845.Born(1811-01-02)2 January 1811Sremski Karlovci, Austrian EmpireDied21 October 1876(1876-10-21) (aged 65)Belgrade, Principality of SerbiaNationalitySerbianMovementRomanticism Uroš Knežević (Serbian Cyrillic: Урош Кнежевић; 2 January 1811 – 21 October 1876) was a Serbian painter. Knežević is best-known as portraitist, having produced more than 200 portraits, mostly of notab...

 

Messiah complex

State of mind where a person believes they are a savior or will be For the comic book, see X-Men: Messiah Complex. Byzantine mosaic image of Jesus of Nazareth, a widely recognizable messiah figure. The messiah complex is a mental state in which a person believes they are a messiah or prophet and will save or redeem people in a religious endeavour.[1][2] The term can also refer to a state of mind in which an individual believes that they are responsible for saving or assisting ...

 

Ulf von Euler

Ulf Svante von Euler Premio Nobel per la medicina 1970 Ulf Svante von Euler (Stoccolma, 7 febbraio 1905 – Stoccolma, 9 marzo 1983) è stato un medico e farmacologo svedese, premio Nobel per le sue ricerche sui neurotrasmettitori. Indice 1 Biografia 1.1 Ambiente familiare 1.2 Studi 1.3 Attività accademica 2 Ricerca 3 Note 4 Bibliografia 5 Altri progetti 6 Collegamenti esterni Biografia Ambiente familiare Apparteneva a una famiglia di eminenti scienziati: Il padre Hans von Euler-Chelpin ...

I'm Taking Off

2011 studio album by Nick CarterI'm Taking OffStudio album by Nick CarterReleasedMay 24, 2011Recorded2010–2011GenrePoppop rockLength42:10LabelKaotic INC604SonyProducerDavid JordanCarl FalkRami YacoubMatthew GerrardDan MuckalaBrent KutzleNoel ZancanellaMidi MafiaNick Carter chronology Now or Never(2002) I'm Taking Off(2011) Nick & Knight(2014) German cover Singles from I'm Taking Off Just One KissReleased: January 28, 2011 I'm Taking OffReleased: May 31, 2011 Love Can't WaitRele...

 

Binomial (polinomial)

Untuk kegunaan lain, lihat Binomial. Binomial dipangkatn empat terdiri dari lima suku. Dalam aljabar, binomial adalah suatu polinomial yang merupakan salah satu jumlah dari dua suku yang masing-masingnya merupakan monomial.[1] Binomial merupakan jenis polinomial rongga sederhana setelah monomial. Definisi Suatu binomial adalah polinomial yang merupakan salah satu penjumlahan dari dua monomial. Suatu binomial dalam bentuk tak tentu tunggal (atau juga dikenal sebagai binomial univariat)...