Harshavarman III

Harshavarman III
King of Cambodia
Reign1066–1080
PredecessorUdayadityavarman II
SuccessorJayavarman VI
Died1080
IssueSri Jayarajacudamani
Names
Narapatindravarman
Posthumous name
Sadaśivapada
FatherYasovarman I
ReligionHinduism

Harshavarman III (Khmer: ហស៌វរ្ម័នទី៣) was a king of Khmer who ruled from 1066 to about 1080 AD.[1] He succeeded his elder brother Udayadityavarman II[2]: 139 [3]: 376  and his capital was the so-called Second Yasodharapura, which had its center in Baphuon, built by his brother, and West Baray as its principal bàrày. He was married to queen Kambujarajalakshmi.

His reign was upset by internal rebellions that finally he was not able to battle out. So Harshavarman III was the last ruler of his dynasty. His successor, Jayavarman VI, was an usurper who came from Phimai area, on the Khorat Plateau, in present-day Thailand.[4] Harshavarman received the posthumous name of Sadaśivapada.[2]: 152 

He was named in stele K.908[5] at Preah Khan as a maternal ancestor of Jayavarman VII, even if a long dispute rose out of this issue.[6]

Between 1074 and 1080, the kingdom had to undergo the invasion by the Champa Prince Pang, a younger brother of the Champa king Harivarman IV, and himself the future king Paramabodhisattva. Sambhupra temples were destroyed and the inhabitants were taken into slavery to My Son, including the prince Sri Nandavarmadeva.[2]: 152 [7]: 72 

In 1076, Cambodia and Champa were driven by the Song Chinese in an attack against the Tonkin. The defeat of the Chinese army from Đại Việt brought before the retirement of its allies.[2]: 152 

Footnotes

  1. ^ W., Aphisit. "Angkor Era - Part II (1001 - 1181 A.D)". cambodia-travel. Cambodia Tours. Archived from the original on 3 December 2012. Retrieved 18 June 2015.
  2. ^ a b c d Coedès, George (1968). Walter F. Vella (ed.). The Indianized States of Southeast Asia. trans.Susan Brown Cowing. University of Hawaii Press. ISBN 978-0-8248-0368-1.
  3. ^ Higham, C., 2014, Early Mainland Southeast Asia, Bangkok: River Books Co., Ltd., ISBN 9786167339443
  4. ^ Higham, 2003, pp.107 ff
  5. ^ W., Aphisit. "Angkor Era - Part I (834 - 1000 A.D)". cambodia-travel. Cambodia Tours. Archived from the original on 11 September 2019. Retrieved 18 June 2015.
  6. ^ John, Sunday; et al. (April 11, 1997). "Preah Khan Conservation Project - Report VII Appendix A" (PDF). New York, USA: World Monuments Fund. pp. 27–28. Archived from the original (PDF) on May 16, 2011. Retrieved 2009-08-17.
  7. ^ Maspero, G., 2002, The Champa Kingdom, Bangkok: White Lotus Co., Ltd., ISBN 9747534991

References

Regnal titles
Preceded by Emperor of Angkor
1066–1080 		Succeeded by
Stub icon

This biography of a member of a Cambodian royal house is a stub. You can help Wikipedia by expanding it.

Read other articles:

Josip Broz Tito

Questa voce o sezione sugli argomenti politici e militari è priva o carente di note e riferimenti bibliografici puntuali. Commento: Numerosi paragrafi e frasi senza nessuna nota. Sebbene vi siano una bibliografia e/o dei collegamenti esterni, manca la contestualizzazione delle fonti con note a piè di pagina o altri riferimenti precisi che indichino puntualmente la provenienza delle informazioni. Puoi migliorare questa voce citando le fonti più precisamente. Segui i suggerimenti dei p...

 

Венгрия

У этого термина существуют и другие значения, см. Венгрия (значения). Венгриявенг. Magyarország Флаг Герб Гимн: «Isten, áldd meg a magyart!» Расположение Венгрии (тёмно-зелёный):— в Европе (светло-зелёный и тёмно-серый)— в Европейском союзе (светло-зелёный) История  • 895[1] Венгерск...

 

ترانزستور

أشكال مختلفة للمقاحل مقحل مبكر الترانزستور[1][2] أو المقحل (بالإنجليزية: Transistor)‏ (اختصاراً لكلمتي Transfer Resistor أي مُقاوِمُ النَقْل) وهي عنصر شبه موصل تعتبر أحد أهم مكونات الأدوات الإلكترونية الحديثة مثل الحاسوب، اخترعه العلماء الأمريكيون (والتر براتين) و (جون باردين) و ...

List of paintings by Charles Marion Russell

These are paintings by the American artist Charles Marion Russell Paintings by Charles Marion Russell Image Title When the Land Belonged to God, 1914 For Supremacy, 1895 (Intertribal warfare among the Blackfeet, Crow, and Sioux) The Tenderfoot, 1900 Smoke of a .45 (A shootout at a saloon) Loops and Swift Horses Are Surer than Lead (Cowboys in Montana catch a bear harassing the herd.) Lewis and Clark on the Lower Columbia Herd Quitters War Council on the Plains To The Victor Belongs The Spoil...

 

Mau-Mau-rörelsen

Mau-Mau-rörelsenGerillarörelse i Kenya Uppror Del avAfrikas avkolonisering LandKenya PlatsKenyakolonin Tidpunkt1952 Start­da­tum1952 Slutdatum1960  Staty som avbildar Dedan Kimathi i Nairobi, Kenya, en av ledarna för Mau-Mau-rörelsen. Mau-Mau-rörelsen var en gerillarörelse i Kenya, främst aktiv mellan 1952 och 1956.[1] Merparten av Mau-Mau utgjordes av medlemmar av kikuyu-stammen. Mau-Maus främsta mål var att överta den kenyanska mar...

 

Comté de Van Buren (Michigan)

Pour les articles homonymes, voir Comté de Van Buren. Comté de Van Buren(en) Van Buren County Le palais de justice du comté de Van Buren. Administration Pays États-Unis État Michigan Chef-lieu Paw Paw Fondation 29 octobre 1829 Démographie Population 76 258 hab. (2010) Densité 48 hab./km2 Géographie Coordonnées 42° 16′ nord, 86° 19′ ouest Superficie 158 200 ha = 1 582 km2 Superficie eau 124 200 ha =...

Pantanal (seri televisi 2022)

PantanalGenre Telenovela Barat Realisme magis BerdasarkanPantanaloleh Benedito Ruy BarbosaPengembangBruno LuperiSutradara Rogério Gomes Gustavo Fernandez Pemeran Marcos Palmeira Dira Paes Jesuíta Barbosa Irandhir Santos Alanis Guillen Penggubah lagu temaMarcus VianaLagu pembukaPantanaloleh Maria Bethânia & Almir SaterNegara asalBrasilBahasa asliPortugisJmlh. musim1Jmlh. episode167 (120 versi internasional)[1]ProduksiProduser eksekutifLucas ZardoProduser Luciana Monteiro Andrea...

 

County Rutland, Vermont

Pusat kota Rutland Lokasi di Vermont County Rutland adalah sebuah county yang terletak di negara bagian Vermont, Amerika Serikat. Pada sensus tahun 2020, jumlah penduduknya adalah 60.572 jiwa,[1] menjadikannya county terpadat kedua di Vermont. Ibu kota dan kotamadya terpadatnya adalah kota Rutland.[2] Sejarah Selama Perang Revolusi terdapat sejumlah benteng dan jalan yang melewati daerah yang sekarang dikenal sebagai County Rutland. Di Castleton ada Benteng Warren dan kemungki...

 

Gugus bola

Messier 80 gugus bola di rasi bintang Scorpius terletak kurang lebih 28,000 tahun cahaya dari matahari dan memiliki ratusan ribu bintang.[1] Gugus bola adalah kumpulan bintang yang berbentuk bola mengorbit inti galaksi sebagai satelit. Gugus bola sangat erat terikat oleh gravitasi, yang membuatnya berbentuk bulat dan kepadatan bintang yang relatif tinggi terhadap pusat galaksi. Nama kategori gugus bintang ini berasal dari bahasa Latin globulus-bola kecil. Referensi ^ Hubble Images a S...

Paris Aéroport

This article contains content that is written like an advertisement. Please help improve it by removing promotional content and inappropriate external links, and by adding encyclopedic content written from a neutral point of view. (December 2017) (Learn how and when to remove this template message) French subsidiary of Groupe ADP Paris AéroportLogo Paris Aéroport since April 2016OwnerGroupe ADPCountryFranceIntroducedApril 2016MarketsTransportation, aviationTaglineParis vous aime (Paris love...

 

Indigenous peoples in Chile

This article is about indigenous peoples of Chile. For other indigenous peoples, see List of indigenous peoples. Indigenous peoples in Chile or Native Chileans form about 13% of the total population of Chile. According to the 2017 census, almost 2,200,000 people declare having indigenous origins.[1] Most Chileans are of partially indigenous descent; however, indigenous identification and its legal ramifications are typically reserved to those who self-identify with and are accepted w...

 

List of museums in Lincolnshire

Wikimedia Commons has media related to Museums in Lincolnshire. This list of museums in Lincolnshire, England contains museums which are defined for this context as institutions (including nonprofit organizations, government entities, and private businesses) that collect and care for objects of cultural, artistic, scientific, or historical interest and make their collections or related exhibits available for public viewing. Also included are non-profit art galleries and university art galler...

Министерство природных ресурсов и экологии Российской Федерации

Министерство природных ресурсов и экологии Российской Федерациисокращённо: Минприроды России Общая информация Страна  Россия Юрисдикция Россия Дата создания 12 мая 2008 Предшественники Министерство природных ресурсов Российской Федерации (1996—1998)Министерство охраны...

 

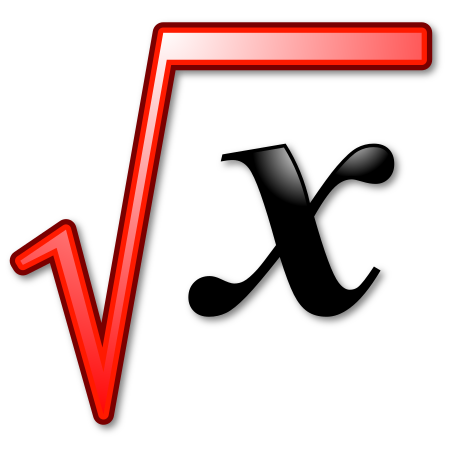
Teorema di Artin-Wedderburn

In algebra astratta, il teorema di Artin-Wedderburn è un teorema che consente la classificazione degli anelli semisemplici (anelli che sono scomponibili come somma diretta di anelli semplici). In base al teorema, ogni anello semisemplice può essere scomposto nel prodotto diretto di particolari anelli di matrici. Il teorema, introdotto da Joseph Wedderburn per i soli anelli semplici, fu in seguito generalizzato da Emil Artin nella forma attuale. Indice 1 Enunciato 1.1 Corollari 1.2 Esempi 2 ...

 

ヨハネス12世 (ローマ教皇)

ヨハネス12世 第130代 ローマ教皇 教皇就任 955年12月16日教皇離任 964年5月14日先代 アガペトゥス2世次代 レオ8世個人情報出生 937年スポレート公国（中部イタリア）スポレート死去 964年5月14日 教皇領、ローマ原国籍 スポレート公国親 父アルベリーコ2世（スポレート公）、母アルダその他のヨハネステンプレートを表示 ヨハネス12世（Ioannes XII、937年 - 964年5月14日）は、ロ...

Cannabinodiol

Chemical compound Not to be confused with cannabinol or cannabidiol. CannabinodiolClinical dataATC codeNoneLegal statusLegal status CA: Schedule II UK: Under Psychoactive Substances Act Identifiers IUPAC name 5'-Methyl-4-pentyl-2'-(prop-1-en-2-yl)-[1,1'-biphenyl]-2,6-diol CAS Number39624-81-2 YPubChem CID11551346ChemSpider9726124UNIICNY5ZTN8E3CompTox Dashboard (EPA)DTXSID401045922 Chemical and physical dataFormulaC21H26O2Molar mass310.437 g·mol−13D model (JSmol)Interact...

 

18-й танковый корпус (СССР)

18-й танковый Знаменско-Будапештский Краснознамённый орденов Суворова и Кутузова корпус(18-й ТК) Вооружённые силы ВС СССР Род войск (сил) Бронетанковые и механизированные войска Вид формирования Танковый корпус Почётные наименования «Знаменский» «Будапештский» Формиро�...

 

Fourierism

Ideology asserting the inevitability of communal association Fourierism is the set of ideas first put forward by French utopian socialist François Marie Charles Fourier (1772–1837). Fourierism (/ˈfʊəriərɪzəm/)[1] is the systematic set of economic, political, and social beliefs first espoused by French intellectual Charles Fourier (1772–1837). Based upon a belief in the inevitability of communal associations of people who worked and lived together as part of the human future...

Rajat Arora

Rajat AroraaRajat AroraaLahirRajat Arora23 Juli 1975 (umur 49)Delhi, IndiaNama lainRajat AroraaAlmamaterAsian Academy of Film & Television[1]PekerjaanPenulis, sutradaraTahun aktif2005 – sekarang Rajat Aroraa (lahir 23 Juli 1975) adalah seorang penulis independen dalam industri film dan televisi India. Ia dikenal atas film-filmnya Once Upon a Time in Mumbai (2010), The Dirty Picture (2011), Kick (2014), Gabbar Is Back (2015) dan Taxi No. 9211 (2006) Referensi ^ ht...

 

Cali (chanteur)

Pour l’article homonyme, voir Cali (homonymie). Cali Cali en concert lors du festival du Bout du Monde en août 2013.Informations générales Nom de naissance Bruno Caliciuri Naissance 28 juin 1968 (56 ans)Perpignan (Pyrénées-Orientales) Activité principale Auteur-compositeur-interprète Genre musical Chanson française, rock français, folk, jazz Années actives Depuis 1997 Labels BMG Site officiel www.calimusic.fr modifier Cali en 2008 au Festival de Bobital. Bruno Caliciuri, dit...