Coherence (fairness)
|
Read other articles:

LG Household & Health Care Ltd.Nama asli주식회사 LG생활건강Nama latinJusikhoesa LG (El-Ji) SaenghwalgeongangJenisPublikKode emitenKRX: 051900IndustriBarang konsumenPendahuluLG ChemDidirikanApril 2001; 22 tahun lalu (2001-04)KantorpusatSeoul, Korea SelatanWilayah operasiSeluruh duniaTokohkunciSuk Y. Cha (Wakil Chairman dan CEO)ProdukKosmetik, Barang Rumah Tangga, MinumanPendapatanKRW 4.677 milyar (2014)[1]Laba operasiKRW 511 milyar (2014)[1]Laba bersihKRW 355 m...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. Fanny CadeoLahirStefania Cadeo11 September 1970 (umur 53)Lavagna, ItaliaKebangsaanItaliaPekerjaan Aktris penyanyi model Tahun aktif1992–sekarangTinggi170 cm (5 ft 7 in)Suami/istriStefano Caviglia (m.&#...

Jack Grealish Jack Grealish bermain untuk Manchester City (2023)Informasi pribadiNama lengkap Jack Peter Grealish[1]Tanggal lahir 10 September 1995 (umur 28)[2]Tempat lahir Birmingham, InggrisTinggi 175 cm (5 ft 9 in)[3]Posisi bermain Sayap, gelandang serangInformasi klubKlub saat ini Manchester CityNomor 10Karier junior2001 Highgate United2001–2012 Aston VillaKarier senior*Tahun Tim Tampil (Gol)2012–2021 Aston Villa 185 (29)2013–2014 → Not...
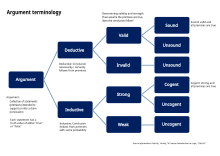
For other uses, see Validity. Argument whose conclusion must be true if its premises are In logic, specifically in deductive reasoning, an argument is valid if and only if it takes a form that makes it impossible for the premises to be true and the conclusion nevertheless to be false.[1] It is not required for a valid argument to have premises that are actually true,[2] but to have premises that, if they were true, would guarantee the truth of the argument's conclusion. Valid ...

Taman InokashiraTaman Inokashira saat musim sakura bermekaran.LokasiMusashino dan Mitaka, Tokyo, JapanArea385,84402 meter persegi (0,09534413 ekar)Dibuat1 Mei 1917 Taman Inokashira (井の頭恩賜公園code: ja is deprecated , Inokashira Onshi Kōen) adalah taman yang berada di antara Musashino dan Mitaka di sebelah barat Tokyo, Jepang. Telaga Inokashira (井の頭池code: ja is deprecated , Inokashira ike) dan sumber air sungai Kanda (神田上水code: ja is deprecated , Kanda jōsui), dibu...

2014 soundtrack album by John PowellHow to Train Your Dragon 2Soundtrack album by John PowellReleasedJune 13, 2014Recorded2012–2014GenreFilm scoreLength1:11:23LabelRelativity Music GroupJohn Powell chronology Rio 2(2014) How to Train Your Dragon 2(2014) Pan(2015) Singles from How to Train Your Dragon 2 Into a FantasyReleased: June 9, 2014 How to Train Your Dragon 2: Music from the Motion Picture is a soundtrack album to the 2014 film How to Train Your Dragon 2, and was released by ...

Aspect of Asian history See also: Asiatic-Pacific Theater vtePacific War Central Pacific Pearl Harbor Marshalls–Gilberts raids K Doolittle Raid Midway Gilberts and Marshalls Marianas and Palau Volcano and Ryukyu Truk Ocean Island Indian Ocean (1941–1945) Japanese merchant raids Andaman Islands Homfreyganj massacre Christmas Island 1st Indian Ocean Ceylon Bay of Bengal 2nd Indian Ocean Southeast Asia Indochina (1940) Franco-Thai War Thailand Malaya Hong Kong Singapore Indochina (1945) Mala...

American actress (1974–2019) Not to be confused with Lisa Sheridan-Paolini. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Lisa Sheridan – news · newspapers · books · scholar · JSTOR (March 2019) (Learn how and when to remove this message) Lisa SheridanBornElizabeth Childress Sheridan[1]December 5...

Indian ceremony celebrating cinema of 1971 This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 19th National Film Awards – news · newspapers · books · scholar · JSTOR (May 2013) (Learn how and when to remove this message) 19th National Film AwardsAwarded forBest of Indian cinema in 1971Awarded byMinistry of Info...

この項目には、一部のコンピュータや閲覧ソフトで表示できない文字が含まれています(詳細)。 数字の大字(だいじ)は、漢数字の一種。通常用いる単純な字形の漢数字(小字)の代わりに同じ音の別の漢字を用いるものである。 概要 壱万円日本銀行券(「壱」が大字) 弐千円日本銀行券(「弐」が大字) 漢数字には「一」「二」「三」と続く小字と、「壱」「�...

بيجو أكسبرت، منتج مما صُنـِـعَ بين 1995 و 2004، زاوية بصيرة ورائية دايهاتسو هايجت بيكأب عربة النقل أو سيارة نفعية (بالإنجليزية: Van) (بالفرنسية: Camionnette) (أو فان بالعاميّة) هي نوع من أنواع العربات التي تستعمل لنقل البضائع أو مجموعات من الناس.[1][2][3] بالعادة تكون عربة ذ...

1987 single by MotörheadEat the RichSingle by Motörheadfrom the album Rock 'n' Roll B-sideCradle to the Grave / Just 'cos You Got the Power (12 only)ReleasedNovember 1987 (UK)[1]Recorded1987StudioMaster Rock StudiosRedwood, London, UKGenreHeavy metalspeed metalLength4:36LabelGWRSongwriter(s)Phil CampbellWürzelLemmyPhil TaylorProducer(s)MotörheadGuy BidmeadMotörhead singles chronology Deaf Forever (1986) Eat the Rich (1987) Ace of Spades (live) (1988) Music videoEat the Rich on Y...

Jordan Veretout Veretout con la nazionale Under-19 francese nel 2012 Nazionalità Francia Altezza 177 cm Peso 75 kg Calcio Ruolo Centrocampista Squadra Olympique Marsiglia CarrieraGiovanili 1999-2003 Belligné2003-2011 NantesSquadre di club1 2011-2015 Nantes130 (13)2015-2016 Aston Villa25 (0)2016-2017→ Saint-Étienne35 (3)2017-2019 Fiorentina69 (13)2019-2022 Roma98 (20)2022- Olympique Marsiglia56 (5)Nazionale 2010-2011 Francia U-188 (2)2011-2...

قائمة بلديات النرويج الرقم[1](أيزو 3166-2:NO) الاسم المركز الإداري المقاطعة عدد النسمة[2] المساحة(km²)[3] الخريطة شعار كتابة اللغة[4] العمدة[5] الحزب 0101 هالدن هالدن Ostfold !أوستفولد &&&&&28٬092 642.34 بوكمول Per Kristian Dahl حزب العمال 0104 موس، النرويج موس، النرويج Ost...

Independent report on alleged corruption Distribution of the report Putin. Corruption. Putin. Corruption. (Russian: Путин. Коррупция., romanized: Putin. Korruptsiya.) is an independent report on alleged corruption in Vladimir Putin’s inner circle published by the leaders of opposition liberal democratic People's Freedom Party in Russia. The report was presented by them at the press conference on 28 March 2011. This is the first large-scale project of the People’s Freedo...

French Open singles finalistsLocationParis FranceCreated1968(56 finals, including 2023)Men's most14: Rafael NadalMen's most consecutive5: Rafael NadalWomen's most9: Chris EvertSteffi GrafWomen's most consecutive4: Chris EvertMartina NavratilovaSteffi GrafMost meetingsMen's (4 times):Nadal vs. Federer (4–0) Women's (4 times):Evert vs. Navratilova (3–1)Official website Main article: French Open The French Open is a Grand Slam tier tennis tournament held in Paris at the Stade Roland Ga...

Cahaya dari Timur: Beta MalukuPoster filmSutradaraAngga Dwimas SasongkoProduserGlenn FredlyAngga Dwimas SasongkoDitulis olehSwastika NoharaM. Irfan RamliAngga Dwimas SasongkoPemeranChicco JerikhoShafira UmmAbdurrahman ArifBurhanuddin OhorellaAufa AssegafBebeto LeutuallyJajang C. NoerPenata musikNikita DompasSinematograferRoby TaswinPenyuntingYoga KrispratamaPerusahaanproduksiVisinema PicturesTanggal rilis19 Juni 2014 (2014-06-19)Durasi150 MenitNegaraIndonesiaBahasaBahasa IndonesiaB...

City in Karnataka, India City in Karnataka, IndiaBhadravati Steel TownCityNickname: BenkipuraBhadravatiLocation in Karnataka, IndiaCoordinates: 13°50′24″N 75°42′07″E / 13.840°N 75.702°E / 13.840; 75.702Country IndiaStateKarnatakaDistrictShivamoggaNamed forBhadra RiverGovernment • BodyCity Municipal CouncilArea[1] • Total67.0536 km2 (25.8895 sq mi)Elevation597 m (1,959 ft)Population (201...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目格式需要修正以符合格式手册。 (2023年3月6日)请协助補充相关的内部链接,并使用百科全书的语气来改善这篇条目。 此條目應避免有陳列雜項、瑣碎資料的部分。 (2023年3月6日)請協助將有關資料重新編排成連貫性的文章,安置於適當章節或條目內。 本條目或許劃分有過多章節以致內容分散。 (202...

Olite - ErriberriVue du palais.Noms officiels (eu) Erriberri (depuis 2010)(es) OliteNom local (eu) ErriberriGéographiePays EspagneComarque Zona Media-Erdialdea (d)Mérindades Mérindade d'Olite (chef-lieu)Communauté forale NavarrePartie de Intermunicipalité de Mairaga-Zona Media/Erdialdea (d), Intermunicipalité des services sociaux de base de la zone d'Olite (d), Zone mixte de NavarreSuperficie 83,2 km2Altitude 388 mCoordonnées 42° 26′ 45″ N, 1°&...














![{\displaystyle [(b_{i})_{i\in S},(c_{i})_{i\notin S}]\in M{\big (}h;(t_{i})_{i=1}^{n}{\big )}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8ca82f401a46db3dfdc6dab2f34047e1b0819e60)
