Character (mathematics)

In mathematics, a character is (most commonly) a special kind of function from a group to a field (such as the complex numbers). There are at least two distinct, but overlapping meanings.^[1] Other uses of the word "character" are almost always qualified.

Multiplicative character

A multiplicative character (or linear character, or simply character) on a group G is a group homomorphism from G to the multiplicative group of a field (Artin 1966), usually the field of complex numbers. If G is any group, then the set Ch(G) of these morphisms forms an abelian group under pointwise multiplication.

This group is referred to as the character group of G. Sometimes only unitary characters are considered (thus the image is in the unit circle); other such homomorphisms are then called quasi-characters. Dirichlet characters can be seen as a special case of this definition.

Multiplicative characters are linearly independent, i.e. if $\chi _{1},\chi _{2},\ldots ,\chi _{n}$ are different characters on a group G then from $a_{1}\chi _{1}+a_{2}\chi _{2}+\dots +a_{n}\chi _{n}=0$ it follows that $a_{1}=a_{2}=\cdots =a_{n}=0$ .

Character of a representation

The character $\chi :G\to F$ of a representation $\phi \colon G\to \mathrm {GL} (V)$ of a group G on a finite-dimensional vector space V over a field F is the trace of the representation $\phi$ (Serre 1977), i.e.

\chi _{\phi }(g)=\operatorname {Tr} (\phi (g))

for

g\in G

In general, the trace is not a group homomorphism, nor does the set of traces form a group. The characters of one-dimensional representations are identical to one-dimensional representations, so the above notion of multiplicative character can be seen as a special case of higher-dimensional characters. The study of representations using characters is called "character theory" and one-dimensional characters are also called "linear characters" within this context.

Alternative definition

If restricted to finite abelian group with $1\times 1$ representation in $\mathbb {C}$ (i.e. $\mathrm {GL} (V)=\mathrm {GL} (1,\mathbb {C} )$ ), the following alternative definition would be equivalent to the above (For abelian groups, every matrix representation decomposes into a direct sum of $1\times 1$ representations. For non-abelian groups, the original definition would be more general than this one):

A character $\chi$ of group $(G,\cdot )$ is a group homomorphism $\chi :G\rightarrow \mathbb {C} ^{*}$ i.e. $\chi (x\cdot y)=\chi (x)\chi (y)$ for all $x,y\in G.$

If $G$ is a finite abelian group, the characters play the role of harmonics. For infinite abelian groups, the above would be replaced by $\chi :G\to \mathbb {T}$ where $\mathbb {T}$ is the circle group.

References

^ "character in nLab". ncatlab.org. Retrieved 2017-10-31.

Artin, Emil (1966), Galois Theory, Notre Dame Mathematical Lectures, number 2, Arthur Norton Milgram (Reprinted Dover Publications, 1997), ISBN 978-0-486-62342-9 Lectures Delivered at the University of Notre Dame
Serre, Jean-Pierre (1977), Linear Representations of Finite Groups, Graduate Texts in Mathematics, vol. 42, Translated from the second French edition by Leonard L. Scott, New York-Heidelberg: Springer-Verlag, doi:10.1007/978-1-4684-9458-7, ISBN 0-387-90190-6, MR 0450380

External links

"Character of a group", Encyclopedia of Mathematics, EMS Press, 2001 [1994]

Read other articles:

Birmingham City F.C.

Birmingham CityNama lengkapBirmingham City Football ClubJulukanBlues[1][2]Berdiri1875; 148 tahun lalu (1875) as Small Heath AllianceStadionSt Andrew's(Kapasitas: 29,409 (restricted to c. 21,000 pending completion of repairs)[3][4])PemilikBirmingham Sports Holdings 51.00%Shelby Companies Ltd 45.64%[5][6]KetuaTom Wagner [7]Wayne RooneySitus webSitus web resmi klub Kostum kandang Kostum tandang Musim ini Birmingham City Foot...

2010 United States Senate election in Louisiana

2010 United States Senate election in Louisiana ← 2004 November 2, 2010 2016 → Nominee David Vitter Charlie Melançon Party Republican Democratic Popular vote 715,415 476,572 Percentage 56.55% 37.67% Parish results Vitter: 40-50% 50-60% 60–70% 70-80% Melançon: 40–50% 50–60% ...

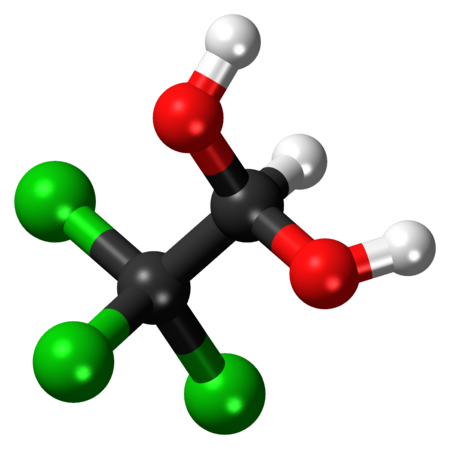
Chloral hydrate

Chemical sedative and hypnotic drug Chloral hydrate Carbon, C Chlorine, Cl Oxygen, O Hydrogen, H Names Preferred IUPAC name 2,2,2-Trichloroethane-1,1-diol Other names Trichloroacetaldehyde monohydrateTradenames:AquachloralChloradormChloratol[1]NoctecNovo-ChlorhydrateSomnosSomnote Identifiers CAS Number 302-17-0 Y 3D model (JSmol) Interactive image Beilstein Reference 1698497 ChEBI CHEBI:28142 Y ChEMBL ChEMBL455917 Y ChemSpide...

Alessandra Sardoni

Italian journalist Alessandra SardoniBorn5 May 1964RomeNationalityItalianOccupationJournalist Alessandra Sardoni (born 5 May 1964) is an Italian journalist and television presenter. Biography Sardoni was born in Rome on 5 May 1964. She studied with Tullio De Mauro and graduated with a degree in Philosophy of language. In 1991 Sardoni worked for the Naples section of la Repubblica. In March 1994, she became a member of the Lazio order of journalists. Sardoni became a political commentator and ...

Brigata Maiella

Questa voce o sezione sull'argomento partigiani non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Brigata MaiellaDistintivo da braccio Descrizione generaleAttiva1943-1945 Nazione Italia Servizio Regio Esercito[1] RuoloUnità di partigiani inquadrata nella VIII Armata britannica Dimensione1360 n...

Pavel Vízner

Czech tennis player This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Pavel Vízner – news · newspapers · books · scholar · JSTOR (September 2015) (Learn how and when to remove this template messa...

Marie dari Rumania

Marie dari EdinburghPermaisuri RumaniaMemerintah sebagai pasangan10 October 1914 – 20 July 1927Penobatan15 October 1922Informasi pribadiKelahiran(1875-10-29)29 Oktober 1875Taman Eastwell, Kent, Inggris, Britania RayaKematian18 Juli 1938(1938-07-18) (umur 62)Istana Pelișor, Sinaia, RumaniaPemakaman24 Juli 1938Katedral Curtea de ArgeșWangsaSaxe-Coburg dan GothaNama lengkapMarie Alexandra VictoriaAyahAlfred, Adipati Saxe-Coburg dan GothaIbuAdipati Wanita Agung Maria Alexandrovna da...

2001 New Zealand local elections

Local elections in New Zealand 2001 New Zealand local elections 13 October 2001 2004 → Politics of New Zealand Constitution The Crown Monarch King Charles III Governor-General (list) Cindy Kiro Realm of New Zealand Executive government List of governments (current) Cabinet Ministers Prime Minister (list) Christopher Luxon Executive Council State services departments Legislature54th New Zealand Parliament King-in-Parliament House of Representatives Speaker: Gerry Brownlee Offic...

Saluran siaga

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

الحزب الإسلامي الماليزي

الحزب الإسلامي الماليزي (باس) (بالملايوية: Parti Islam Se-Malaysia)‏[1](بالملايوية: Persatuan Islam Se-Tanah Melayu)‏[2](بالملايوية: Persatuan Islam Se-Malaya)‏[2](بالإنجليزية: Islamic Party of Malaya)‏[2](بالإنجليزية: Pan-Malayan Islamic Party)‏[2](بالإنجليزية: Islamic Party of Malaysia)‏[3](بالملايوية: Persatuan Ulamak Se-Malaya)‏ ...

محمد عبد الحليم أبو غزالة

المشير عبد الحليم أبو غزالة القائد العام للقوات المسلحة وزير الدفاع والإنتاج الحربي نائب رئيس مجلس الوزراء في المنصب1981 – 1989 الرئيس محمد حسني مبارك أحمد بدوي يوسف صبري أبو طالب رئيس أركان حرب القوات المسلحة في المنصب1980 – 1981 أحمد بدوي عبد رب النبي حافظ مدير إدارة المخابرات...

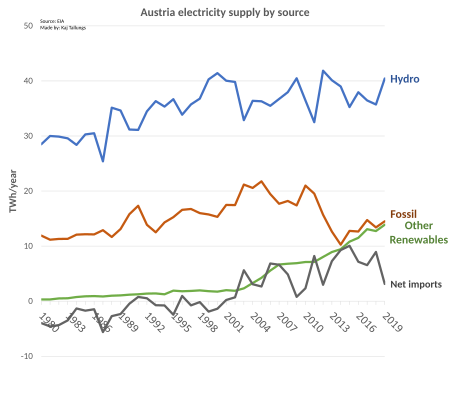
Energy in Austria

A small wind turbine in Frastanz, Vorarlberg, Austria Energy in Austria describes energy and electricity production, consumption and import in Austria. Austria is very reliant on hydro as an energy source, supported by imported oil and natural gas supplies. It is planned by 2030 to become 100% electricity supplied by renewable sources, primarily hydro, wind and solar.[citation needed] Targets The Austrian energy plan made in 2020 has the following targets:[1] Carbon neutralit...

Daftar Wakil Bupati Tapanuli Selatan

Wakil Bupati Tapanuli SelatanPetahanaRasyid Assaf Dongoran, M.Si.sejak 26 Februari 2021Masa jabatan5 tahunDibentuk1996Pejabat pertamaDrs. H. Syahruddin NasutionSitus webwww.tapselkab.go.id Berikut ini adalah daftar Wakil Bupati Tapanuli Selatan dari masa ke masa. No Wakil Bupati Mulai Jabatan Akhir Jabatan Prd. Ket. Bupati 1 Drs. H.Syahruddin Nasution 1996 1999 1 Drs. H.Sualoon Siregar Jabatan kosong 1999 2000 - Ir.Suangkupon SiregarM.Sc.(Penjabat) 2 H.Herry Lontung Siregar...

Satuan Radar 214

Satuan Radar 214Komando Sektor Pertahanan Udara Nasional ILambang SATRAD 214 TegalNegara IndonesiaCabang Tentara Nasional IndonesiaTipe unitKomando Pertahanan NasionalBagian dariKosek IKNMotoLabda Pratiyata Yudha (Kemahiran, Kesaktian, Peperangan)Situs webwww.kohanudnas.mil.idTokohKomandanLetkol Lek I Ketut Wiratmaja Satuan Radar 214/Tegal (atau Satrad 214/Tegal) merupakan unsur pertahanan udara yang berada langsung dibawah komando Kosek IKN. Di wilayah utara Provinsi Jawa Tengah yang be...

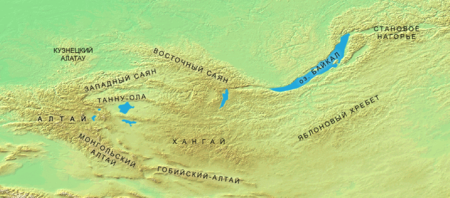
Южная Сибирь

Южная Сибирь регион Страна Россия Западная и центральная части горной страны Ю́жная Сиби́рь — часть Сибири, выделяемая по различным основаниям. 1. По физико-географическим условиям Южная Сибирь, это: горная страна, протянувшаяся с запада на восток от Западно-Сибир�...

Shershaah

ShershaahSutradaraVishnuvardhanProduserHiroo Yash JoharKaran JoharApoorva MehtaShabbir BoxwalaAjay ShahHimanshu GandhiDitulis olehSandeep SrivastavaPemeranSidharth MalhotraKiara AdvaniPenata musikSkor:John Stewart EduriLaguTanishk BagchiB PraakJaaniJasleen RoyalJaved-MohsinVikram MontroseSinematograferKamaljeet NegiPenyuntingA. Sreekar PrasadPerusahaanproduksiDharma ProductionsKaash EntertainmentDistributorAmazon Prime VideoTanggal rilis 12 Agustus 2021 (2021-08-12)[1] Dura...

Albufeira

Albufeira Ciudad de Portugal y Municipio de Portugal BanderaEscudo AlbufeiraLocalización de Albufeira en Portugal Coordenadas 37°05′23″N 8°14′45″O / 37.0897, -8.24583Idioma oficial PortuguésEntidad Ciudad de Portugal y Municipio de Portugal • País Portugal • Región Algarve • Distrito FaroFreguesias 5Eventos históricos • Fundación 1504Superficie • Total 140,91 km²Altitud • Media 22 m s. n. m.Poblaci...

دلفوس (كانساس)

لمعانٍ أخرى، طالع دلفوس (توضيح). دلفوس الإحداثيات 39°16′29″N 97°45′59″W / 39.2747°N 97.7664°W / 39.2747; -97.7664 [1] سبب التسمية دلفوس تقسيم إداري البلد الولايات المتحدة[2] التقسيم الأعلى مقاطعة أوتاوا خصائص جغرافية المساحة 1.655198 كيلومتر مربع1.6...

From Caligari to Hitler

Book by Siegfried Kracauer First edition(publ. Princeton University Press) From Caligari to Hitler: A Psychological History of the German Film is a book by film critic and writer Siegfried Kracauer, published in 1947. Background This work of film theory is one of the first major studies of German film between World War I and World War II and is best known for proposing a link between the apolitical and escapist orientation of Weimar-era cinema and later German totalitarianism. Kracauer—know...

John Leslie (physicist)

Scottish mathematician and physicist (1766–1832) For other people named John Leslie, see John Leslie (disambiguation). John LeslieJohn LeslieBorn10 April 1766 (1766-04-10)Largo, FifeDied3 November 1832 (1832-11-04) (aged 66)NationalityBritishKnown forStudies of heat Leslie cubeAwardsRumford Medal (1804)Scientific careerFieldsMathematics Physics Sir John Leslie by Sir John Steell Sir John Leslie, FRSE KH (10 April 1766 – 3 November 1832) was a Scottish mathematician and...

Deepwater Horizon

/profillengkap.com/index.php/Aksara Jawa

/profillengkap.com/index.php/article/York Mills Collegiate Institute

Kramat Sukoharjo, Tanggul, Jember

1995–96 London Broncos season

Sierradyne

Names of the Celts

Diomedes, el cacique de la junta

Keith Lee (basketball)

Template talk:Padma Bhushan Award recipients 2010–2019

Mill Creek, Washington

Federica Cesarini