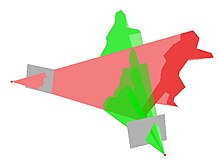
3D reconstruction from multiple images
|
Read other articles:

National governing body of cycle racing in Ecuador FEC logo The Ecuadorian Cycling Federation or FEC (in Spanish: Federación Ecuatoriana de Ciclismo) is the national governing body of cycle racing in Ecuador. The FEC is a member of the UCI and COPACI. External links Federación Ecuatoriana de Ciclismo official website vteSports governing bodies in Ecuador (ECU)Summer Olympic Sports Aquatics Diving Swimming Synchronized Swimming Water Polo Archery Athletics Badminton Basketball Boxing Canoein...

Untuk tempat lain yang bernama sama, lihat Prambanan (disambiguasi). SlemanKecamatanPeta lokasi Kecamatan SlemanNegara IndonesiaProvinsiJawa TengahKabupatenKlatenPemerintahan • Camat-Populasi • Total41,789 jiwaKode Kemendagri33.10.01 Kode BPS3310010 Luas24,43 km²Desa/kelurahan16 Candi Sojiwan Prambanan (Jawa: ꦥꦿꦩ꧀ꦧꦤꦤ꧀) adalah sebuah kecamatan di Kabupaten Klaten, Jawa Tengah. Di sebelah barat kecamatan ini berbatasan dengan Kapanewon Prambanan...

Penyamakan di Marrakesh Penyamakan adalah suatu proses mengubah kulit mentah menjadi kulit tersamak. Hampir semua jenis hewan ternak mengalami penyamakan kulit, seperti sapi, kerbau, kambing, kelinci, domba, dan ikan pari. Penyamakan juga dilakukan pada beberapa kulit hewan buas antara lain ular, harimau dan buaya.[1] Referensi ^ Salinan arsip. Diarsipkan dari versi asli tanggal 2019-06-21. Diakses tanggal 2019-06-14. Pranala luar Wikimedia Commons memiliki media mengenai Tannin...

Kushite Empire redirects here. For the kingdom south of Egypt, see Kingdom of Kush. Kushite rule in Egypt during the third intermediate period Twenty-fifth Dynasty of Egypt754 BC–656 BC Statues of various rulers of the late 25th Dynasty–early Napatan period. From left to right: Tantamani, Taharqa (rear), Senkamanisken, again Tantamani (rear), Aspelta, Anlamani, again Senkamanisken; Kerma Museum.[1] Kushite heartland, and Kushite Empire of the 25th dynasty of Egypt, circa 700 BC.&#...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Februari 2023. Pierre-Philibert Maubant Pierre-Philibert Maubant (20 September 1803 – 21 September 1839) adalah seorang pastor kelahiran Vassy, Perancis. Setelah ditahbiskan menjadi imam, dia bergabung dengan Serikat Misi Paris (Paris Foreign Mission...

Mitra Bola UtamaNama lengkapMBU Baruna Nusantara FC BanyuwangiJulukanLaskar SamudraStadionStadion DiponegoroBanyuwangi(Kapasitas: 5.000)PemilikHariyonoPelatihBambang SomantriLigaLiga 3 Jawa TimurBabak 16 besar (Zona Jawa Timur) Kostum kandang Kostum tandang Mitra Bola Utama atau MBU Baruna Nusantara FC Banyuwangi adalah klub sepak bola amatir yang berbasis di Kabupaten Banyuwangi, Jawa Timur. Pada tahun 2011, klub ini berhasil menjuarai Divisi III musim 2010-2011 sekaligus mendapat promosi ke...

Japanese manga series Tokyo YamimushiFirst tankōbon volume cover東京闇虫GenreCrime[1] MangaWritten byYūki HondaPublished byHakusenshaMagazineYoung AnimalDemographicSeinenOriginal runAugust 27, 2010 – January 11, 2013Volumes7 MangaTokyo Yamimushi -2nd Scenario- PandoraWritten byYūki HondaPublished byHakusenshaMagazineYoung AnimalDemographicSeinenOriginal runFebruary 22, 2013 – January 22, 2016Volumes8 Tokyo Yamimushi (東京闇虫) is a Japanese manga ser...

Marine nationale Création 1961 Pays Côte d'Ivoire Branche Forces armées de Côte d'Ivoire Effectif 1 400 hommes (en 2011) Garnison Lokodjro, San-Pédro, Adiaké Devise « C'est par la terre qu'un peuple grandit. C'est par la mer qu'il devient fort » Commandant Vice-amiral N'guessan Kouamé Celestin modifier La Marine nationale ivoirienne est la composante navale (navires de guerre) et l'une des quatre composantes des Forces armées de Côte d'Ivoire. La marine d...

MaybellineJenisAnak perusahaanIndustriKosmetikDidirikan1915; 109 tahun lalu (1915)PendiriThomas Lyle WilliamsKantorpusatNew York City, New York, United StatesWilayah operasiSeluruh duniaTokohkunci Leonardo Chavez, President (Global)[1] David Greenberg, President (US)[2] IndukL'OréalSitus webmaybelline.com Adriana Lima, salah satu model internasional yang wajahnya kerap menghiasi iklan Maybelline Maybelline adalah produk kosmetik internasional yang didirikan pada tahun 19...

Seafood product Dried shredded squidChinese name (by strand)Traditional Chinese魷魚絲Simplified Chinese鱿鱼丝Hanyu Pinyinyóu yú sīJyutpingjau4 jyu4*2 si1 Literal meaningsquid strandTranscriptionsStandard MandarinHanyu Pinyinyóu yú sīYue: CantoneseJyutpingjau4 jyu4*2 si1Southern MinHokkien POJjiû-hî-chhuì (鰇魚絲)Chinese name (by slice)Traditional Chinese魷魚片Simplified Chinese鱿鱼片Hanyu Pinyinyóu yú piànJyutpingjau4 jyu4*2 pin3 TranscriptionsStandard Man...

Type of coffee drink This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (December 2012) (Learn how and when to remove this template message) This article needs additional citations for verification. Pl...

Politique européenne de voisinage (relations extérieures de l'UE) : États membres Candidats reconnus Candidats potentiels Partenariat oriental Accord de partenariat et de coopération avec la Russie États membres de l'Association européenne de libre-échange Partenariat Euromed Autres pays de l'UPM La participation de l'Union européenne aux organisations internationales est une question qui relève des relations extérieures de l'Union européenne et de la position politique selon ...

Busur melingkar berwarna merah merupakan geodesik dalam model cakram Poincaré, diproyeksikan ke geodesik berwarna cokelat dari hiperboloid berwarna hijau. Animasi sebagian {7,3} ubin hiperbolik hiperboloid diputar ke dalam perspektif Poincare. Dalam geometri, model hiperboloid, juga dikenal sebagai model Minkowski, dinamai Hermann Minkowski adalah sebuah model pada geometri hiperbolik dimensi- n {\displaystyle n} yang dimana titik-titik tersebut diwakili oleh titik-titik dari lembaran depan ...

زيارة الوزير الهولندي (بلوك) لكل من النيجر ونجيريا وتونس بعثة الاتحاد الأوروبي لبناء القدرات في النيجر هي مهمة بناء القدرات مدنية في النيجر، أطلقها الاتحاد الأوروبي في عام 2012 بموجب سياسة الأمن والدفاع المشتركة. منذ عام 2018، رئيس البعثة هو فرانك فان دير مورين، ضابط شرطة بلجي...

TeXworks Тип TeX-редактор Разработчики Jonathan Kew, Stefan Loffler, Charlie Sharpsteen Написана на XML, C++ Интерфейс Qt Операционные системы Windows, Linux, Mac OS X Языки интерфейса многоязычный[1] Последняя версия 0.6.6 (11 марта 2021) Репозиторий github.com/TeXworks/texw… Лицензия GNU GPL[2] Сайт github.com/TeXworks Меди�...

American conservative political commentator and YouTuber Bill WhittleWhittle in 2014Born (1959-04-07) April 7, 1959 (age 65)New York City, U.S.Alma materUniversity of FloridaOccupations Author director screenwriter editor internet personality blogger Websitebillwhittle.com This article is part of a series onConservatismin the United States Schools Compassionate Fiscal Fusion Libertarian Moderate Movement Neo Paleo Progressive Social Traditionalist Principles American exceptionalism ...

Questa voce o sezione sull'argomento guerra è priva o carente di note e riferimenti bibliografici puntuali. Sebbene vi siano una bibliografia e/o dei collegamenti esterni, manca la contestualizzazione delle fonti con note a piè di pagina o altri riferimenti precisi che indichino puntualmente la provenienza delle informazioni. Puoi migliorare questa voce citando le fonti più precisamente. Segui i suggerimenti del progetto di riferimento. La guerra elettronica (in inglese electronic wa...

English brewery founded in 1787 CourageIndustryBrewingFounded1787FounderJohn CourageProductsBeerOwnerMarston's BreweryWebsitetakecourage.info Courage Brewery was an English brewery, founded by John Courage in 1787 in London, England. History Courage & Co Ltd was started by John Courage at the Anchor Brewhouse in Horsleydown, Bermondsey, in 1787. He was a Scottish shipping agent of French Huguenot descent.[1] It became Courage & Donaldson in 1797. By 1888, it had been reg...

River in Newfoundland and Labrador, CanadaChurchill RiverChurchill River and waterfalls, LabradorLocation of the mouthNative nameMishtashipu (Innu)LocationCountryCanadaProvinceNewfoundland and LabradorPhysical characteristicsSource • locationSmallwood Reservoir, Labrador • elevation466 m (1,529 ft) Mouth • locationAtlantic OceanLength856 km (532 mi)Basin size79,800 km2 (30,800 sq mi)Discha...

Nikolaj Andreevič Rimskij-Korsakov Nikolaj Andreevič Rimskij-Korsakov (in russo Николай Андреевич Римский-Корсаков?; Tichvin, 18 marzo 1844 – Ljubensk, 21 giugno 1908) è stato un compositore e docente russo, particolarmente noto per la sua fine orchestrazione, basata soprattutto sulla valorizzazione dei timbri degli strumenti e del loro singolo colore che diventa fondamentale nella tessitura musicale. Le sue composizioni più famose sono Shahrazād, La...