مايكل هايز (مصارع)
| |||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Содержание 1 Методы получения низких температур 1.1 Испарение жидкостей 1.2 Дросселирование 1.3 Расширение с совершением внешней работы 1.4 Адиабатическое размагничивание 1.5 Эффект Пельтье 1.6 Криостат растворения 2 Измерение низких температур 3 История физики низких темпера...

German physician and astrologer (1505–1577) Achilles GasserBorn(1505-11-03)3 November 1505Lindau, Holy Roman EmpireDied4 December 1577(1577-12-04) (aged 72)Mixed Imperial City of Augsburg, Holy Roman EmpireKnown forComet observations, research on European history and geographyScientific careerFieldsAstronomycartography Achilles Pirmin Gasser[1] (3 November 1505 – 4 December 1577)[2] was a German physician and astrologer. He is now known as a well-connected human...

American musician and lead vocalist of Hall & Oates (born 1946) For other people with similar names, see Darryl Hall (disambiguation). Daryl HallHall in 2011Background informationBirth nameDaryl Franklin HohlAlso known asDaryl HohlDaryl F. HallD. Franklin HallBorn (1946-10-11) October 11, 1946 (age 77)Pottstown, Pennsylvania, U.S.OriginPhiladelphia, Pennsylvania, U.S.Residence: Connecticut / London, EnglandGenresRocksoulblue-eyed soulR&Bpop rockblues rocksoft rockalternative coun...

American screenwriter and film producer Carl ForemanCBECarl Foreman in 1961Born(1914-07-23)July 23, 1914Chicago, Illinois, U.S.DiedJune 26, 1984(1984-06-26) (aged 69)Beverly Hills, California, U.S.Occupation(s)Screenwriter, film producerSpouses Estelle Barr Evelyn Smith[1] Children3, including Jonathan and Amanda Carl Foreman, CBE (July 23, 1914 – June 26, 1984) was an American screenwriter and film producer who wrote the award-winning films The Bridge on the River Kwai and Hig...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Syrup made from the hydrolysis of starch Glucose syrup on a black surface Glucose syrup, also known as confectioner's glucose, is a syrup made from the hydrolysis of starch. Glucose is a sugar. Maize (corn) is commonly used as the source of the starch in the US, in which case the syrup is called corn syrup, but glucose syrup is also made from potatoes and wheat, and less often from barley, rice and cassava.[1]p. 21[2] Glucose syrup containing over 90% glucose is used in i...

Graph with nodes connected in a closed chain This article is about connected, 2-regular graphs. For other uses, see Cyclic graph. CycleGirthnAutomorphisms2n (Dn)Chromatic number3 if n is odd2 otherwiseChromatic index3 if n is odd2 otherwiseSpectrum { 2 cos ( 2 k π n ) ; k = 1 , ⋯ , n } {\displaystyle \left\{2\cos \left({\frac {2k\pi }{n}}\right);k=1,\cdots ,n\right\}} [1]Properties2-regularVertex-transitiveEdge-transitiveUnit distanceHamiltonianEulerianNotationC...
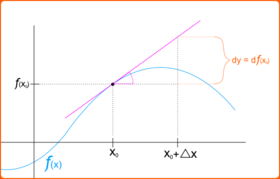
Notion in calculus For other uses of differential in mathematics, see Differential (mathematics). Part of a series of articles aboutCalculus ∫ a b f ′ ( t ) d t = f ( b ) − f ( a ) {\displaystyle \int _{a}^{b}f'(t)\,dt=f(b)-f(a)} Fundamental theorem Limits Continuity Rolle's theorem Mean value theorem Inverse function theorem Differential Definitions Derivative (generalizations) Differential infinitesimal of a function total Concepts Differentiation notation Second d...

Keuangan bagian dari Ekonomi Pasar uangPasar Bond · Pasar bursa efek (Ekuitas) · Devisa · Derivatif · Komoditi · Uang · Spot (tunai) · Pasar OTC · Real estat · Ekuitas swasta Pelaku pasarInvestor · Spekulan · Lembaga Investor Keuangan korporasiStruktur keuangan · Penganggaran pemodalan · Manajemen risiko keuangan · Merger dan A...

Aspect of German and Russian history Ethnic group Germans from RussiaRusslanddeutscheРоссийские немцыTotal population~3 millionRegions with significant populations Germany~2.3 million Kazakhstan226,092 (2021)[1] Russia195,256 (2021)[2] Ukraine33,302 (2001)[3]LanguagesGerman, Russian, Ukrainian, KazakhReligionHistorically:[4][5]Lutheran majorityCatholic minorityCurrently:[6]majorityLutheran, Baptist, Pentecostal...

Circle of latitude that is 43 degrees north of the Earth's equatorial plane 43°class=notpageimage| 43rd parallel north Map all coordinates using OpenStreetMap Download coordinates as: KML GPX (all coordinates) GPX (primary coordinates) GPX (secondary coordinates) The 43rd parallel north is a circle of latitude that is 43 degrees north of the Earth's equatorial plane. It crosses Europe, the Mediterranean Sea, Asia, the Pacific Ocean, North America, and the Atlantic Ocean. The South Dakota-Neb...

American cognitive scientist Not to be confused with Alan Newell. Allen NewellBorn(1927-03-19)March 19, 1927San Francisco, CaliforniaDiedJuly 19, 1992(1992-07-19) (aged 65)Pittsburgh, PennsylvaniaAlma materStanford UniversityPrinceton UniversityCarnegie Mellon UniversityKnown forInformation Processing LanguageLogic TheoristGeneral Problem SolverSoarUnified Theories of CognitionAwardsA.M. Turing Award (1975)IJCAI Award for Research Excellence (1989)IEEE Emanuel R. Piore Award (1...

لمعانٍ أخرى، طالع سيدني سميث (توضيح). الأميرال السير وليم سيدني سميث (بالإنجليزية: Sidney Smith)، و(بالإنجليزية: William Sidney Smith) معلومات شخصية اسم الولادة وليم سيدني سميث الميلاد 21 يونيو 1764(1764-06-21)لندن الوفاة 26 مايو 1840 (75 سنة)باريس مكان الدفن مقبرة بير لاشيز[1][2][3...

Genus of flowering plants Brunia The leaves and the knobby inflorescences of Brunia sp. (family Bruniaceae) Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Clade: Asterids Order: Bruniales Family: Bruniaceae Genus: BruniaLam. Brunia is a genus of shrubs of the family Bruniaceae, native to the cape region of South Africa.[1] Taxonomy The genus was described by Carl Linnaeus and published in Species Plantarum 1: 199 in the year 1753. Th...

Constituency of the National Assembly of France 5th constituency of MarneinlineConstituency of the National Assembly of FranceThe constituency shown within Champagne-ArdenneIncumbent deputyCharles de CoursonLCDepartmentMarneCantonsAnglure, Avize, Écury-sur-Coole, Fère-Champenoise, Heiltz-le-Maurupt, Saint-Remy-en-Bouzemont-Saint-Genest-et-Isson, Sompuis, Thiéblemont-Farémont, Vertus, Vitry-le-François-Est, Vitry-le-François-OuestRegistered voters76,921 (2017) Politics of France Politica...

Museum, Public library in New PlymouthPuke ArikiNorth building of Puke ArikiFormer nameTaranaki Museum, New Plymouth Public LibraryEstablished1848 (library), 1919 (museum)LocationNew PlymouthCoordinates39°03′25″S 174°04′19″E / 39.05694°S 174.07194°E / -39.05694; 174.07194TypeMuseum, Public libraryManagerColleen MullinWebsitepukeariki.com Puke Ariki is a combined museum and library at New Plymouth, New Zealand which opened in June 2003. It is an amalgamatio...

Pour les autres navires du même nom, voir HMS Challenger. Pour les articles homonymes, voir Challenger. Cet article est une ébauche concernant un bateau ou un navire. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Le HMS Challenger durant son voyage d'exploration 1872-1876. Le HMS Challenger (HMS pour His/Her Majesty's Ship), cinquième du nom, est une corvette de la Royal Navy, à assistance vapeur, lancée ...

Disambiguazione – Se stai cercando l'omonimo film sudcoreano del 2003, vedi Yeokjeon-e sanda. Questa voce o sezione sull'argomento film drammatici non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Questa voce sull'argomento film drammatici è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Il mistero Von BulowJeremy ...

Usiacurí Municipio Vista aérea e iglesia de Santo Domingo de Guzmán BanderaEscudo Otros nombres: Pesebre del Atlántico UsiacuríLocalización de Usiacurí en Colombia UsiacuríLocalización de Usiacurí en AtlánticoCoordenadas 10°44′34″N 74°58′33″O / 10.742777777778, -74.975833333333Entidad Municipio • País Colombia • Departamento AtlánticoAlcalde Katherine Pasos Zapata (2020-2023)Eventos históricos • Fundación 17 de septiembre de...
