Ký hiệu Steinhaus–Moser
|
Read other articles:
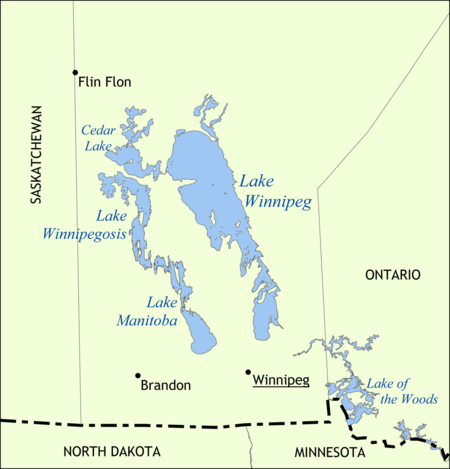
Danau WinnipegPetaLetakManitoba, CanadaKoordinat52°7′N 97°15′W / 52.117°N 97.250°W / 52.117; -97.250Koordinat: 52°7′N 97°15′W / 52.117°N 97.250°W / 52.117; -97.250Jenis perairanSebelumnya bagian dari Danau Agassiz glasial, reservoirAliran masuk utamaWinnipeg River, Saskatchewan River, Red RiverAliran keluar utamaNelson RiverWilayah tangkapan air982.900 km2 (379.500 sq mi)Terletak di negaraKanada, Amerika SerikatPan...

Uang kertas Rp1.000 Tahun Emisi 2016 (bagian depan) Uang kertas Rp1.000 Tahun Emisi 2016 (bagian belakang) Rp1.000 (dibaca: seribu rupiah) (Penulisan baku: Rp1.000,00) adalah nilai nominal uang kertas dan yang pernah dicetak dan hingga 2022 masih beredar secara resmi di Indonesia. Untuk tahun 2014 sampai dengan 2015, Bank Indonesia tidak mencetak uang kertas pecahan Rp1.000. Uang dengan nominal Rp1.000 diedarkan dan dicetak dengan beberapa emisi dan seri.[butuh rujukan] Rp1.000 diceta...

Makam keluarga Jean-Claude Pascal di pemakaman Montparnasse, Paris Jean-Claude Pascal (24 Oktober 1927 – 5 Mei 1992), nama lahir Jean-Claude Villeminot, adalah seorang komedian dan penyanyi asal Prancis. Pascal memenangkan Kontes Lagu Eurovision 1961 mewakili Luxembourg dengan lagu Nous les amoureux. Pranala luar Jean-Claude Pascal di IMDb (dalam bahasa Inggris) lbsDaftar pemenang Kontes Lagu EurovisionNegara pemenang1950-an Swiss Belanda Prancis Belanda 1960-an Prancis Luxemb...

Chinese commercial satellite system Jilin-1吉林一号Program overviewCountry People's Republic of ChinaOrganizationChang Guang Satellite Technology CompanyPurposeCommercial earth observationStatusActiveProgram historyDuration2015–presentFirst flight7 October 2015Successes130Failures4Launch site(s)JSLCTSLCTai Rui BargeVehicle informationLaunch vehicle(s)CZ-2DKZ-1ACZ-6CZ-8CZ-11KZ-11SQX-1Ceres-1SD-3 Jilin-1 (simplified Chinese: 吉林一号; traditional Chinese: 吉林一號; pinyin&...

American jam band This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: The String Cheese Incident – news · newspapers · books · scholar · JSTOR (May 2009) (Learn how and when to remove this template message) The String Cheese IncidentBackground informationOriginCrested Butte, Colorado, United StatesGenresJam band...

Questa voce o sezione sull'argomento biografie è priva o carente di note e riferimenti bibliografici puntuali. Sebbene vi siano una bibliografia e/o dei collegamenti esterni, manca la contestualizzazione delle fonti con note a piè di pagina o altri riferimenti precisi che indichino puntualmente la provenienza delle informazioni. Puoi migliorare questa voce citando le fonti più precisamente. Segui i suggerimenti del progetto di riferimento. Angelo Majorana Calatabiano Ministro delle f...

Loi favorisant la diffusion et la protection de la création sur internet Données clés Présentation Titre Loi no 2009-669 du 12 juin 2009 favorisant la diffusion et la protection de la création sur internet Abréviation LCI Pays France Type Loi ordinaire Branche Propriété intellectuelle Adoption et entrée en vigueur Législature XIIIe législature de la Ve République Gouvernement Gouvernement François Fillon (2) Adoption Assemblée nationale : 12 mai 2009, Sénat : 13...

烏克蘭總理Прем'єр-міністр України烏克蘭國徽現任杰尼斯·什米加尔自2020年3月4日任命者烏克蘭總統任期總統任命首任維托爾德·福金设立1991年11月后继职位無网站www.kmu.gov.ua/control/en/(英文) 乌克兰 乌克兰政府与政治系列条目 宪法 政府 总统 弗拉基米尔·泽连斯基 總統辦公室 国家安全与国防事务委员会 总统代表(英语:Representatives of the President of Ukraine) 总...

ديوميد الإحداثيات 65°45′30″N 168°57′06″W / 65.758333333333°N 168.95166666667°W / 65.758333333333; -168.95166666667 [1] تاريخ التأسيس 28 أكتوبر 1970 تقسيم إداري البلد الولايات المتحدة[2] خصائص جغرافية المساحة 2.84 ميل مربع7.357539 كيلومتر مربع (1 أبريل 2010) ارتفاع 29 متر عدد ...

2022 Japanese animated film by Takehiko Inoue The First Slam DunkTheatrical release posterJapaneseTHE FIRST SLAM DUNK[1] Directed byTakehiko InoueScreenplay byTakehiko InoueBased onSlam Dunkby Takehiko InoueProduced byToshiyuki MatsuiStarringShugo NakamuraJun KasamaShinichiro Kamio[2][3]Subaru KimuraKenta MiyakeEdited byRyuichi Takita[3]Music bySatoshi TakebeTakuma MitamuraAnimation byHideki Takahashi(key animator)Kiyotaka Oshiyama(key animator)Naoya Wada(key a...

Ōno 大野市Kota BenderaLambangLokasi Ōno di Prefektur FukuiNegara JepangWilayahChūbuPrefektur FukuiPemerintahan • Wali kotaShiho IshiyamaLuas • Total872 km2 (337 sq mi)Populasi (Oktober 1, 2015) • Total33.109 • Kepadatan37,97/km2 (9,830/sq mi)Zona waktuUTC+9 (JST)Kode pos912-8666Simbol • PohonFagus crenata• BungaMagnolia kobus• BurungHorornis diphone• IkanGasterosteus aculeatusNomor telep...

Building in Southland Dr. S.W., CalgarySouthland Leisure CentreMain EntranceGeneral informationAddress2000 Southland Dr. S.W.Town or cityCalgaryCoordinates50°57′45″N 114°06′29″W / 50.96249°N 114.10797°W / 50.96249; -114.10797Opened1983Technical detailsFloor area227,000 square feetWebsitehttp://www.calgary.ca/CSPS/Recreation/Pages/Leisure-centres/Southland.aspx View of the Water park and Wave pool The Southland Leisure Centre is an indoor leisure centre situ...

Basketball position This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Combo guard – news · newspapers · books · scholar · JSTOR (June 2011) (Learn how and w...

2021 UK local government election Map showing the results of the 2021 Gateshead Metropolitan Borough Council election The 2021 Gateshead Metropolitan Borough Council election took place on 6 May 2021 to elect members of Gateshead Metropolitan Borough Council in England on the same day as other local elections.[1] One-third of the seats were up for election, with two wards (Birtley and Lamesley) electing two councillors.[2] Results 2021 Gateshead Metropolitan Borough Council el...

Isaac PriceNazionalità Irlanda del Nord Altezza175 cm Peso70 kg Calcio RuoloCentrocampista Squadra Standard Liegi CarrieraGiovanili 2010-2022 Everton Squadre di club1 2022-2023 Everton2 (0)2023- Standard Liegi19 (1) Nazionale 2019 Irlanda del Nord U-172 (0)2021 Irlanda del Nord U-194 (0)2022 Irlanda del Nord U-213 (0)2023- Irlanda del Nord12 (2) 1 I due numeri indicano le presenze e le reti segnate, per le sole partite di campionato.Il simbolo → indica un trasferim...

Il mutilato di guerra è il militare che nel corso di un conflitto bellico subisce una mutilazione o menomazione. Se ha una disabilità viene definito invalido di guerra. Distintivo d'onore di mutilato di guerra italiano Indice 1 Storia 2 In Italia 2.1 L'Associazione nazionale 3 Note 4 Voci correlate 5 Altri progetti Storia Fin dai tempi remoti era previsto un intervento pubblico in favore di coloro che combattendo perdevano l'integrità della persona: ad Atene si provvedeva all'educazione de...

Species of cactus Astrophytum asterias Conservation status Vulnerable (IUCN 3.1)[1] CITES Appendix I (CITES)[2] Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Order: Caryophyllales Family: Cactaceae Subfamily: Cactoideae Genus: Astrophytum Species: A. asterias Binomial name Astrophytum asterias(Zucc.) Lem.[3] Astrophytum asterias is a species of cactus in the genus Astrophytum, and is native to small p...

2017 Chinese TV series or program Tientsin MysticTheatrical release posterGenreFantasySuspenseBased onHe Shenby Tianxia BachangWritten byLiu ChenglongYang HongweiZhang ChaoLiu MengshaLi YangDirected byTian LiStarringLi XianZhang Ming'enWang ZixuanChen YumiCountry of originChinaOriginal languageMandarinNo. of seasons1No. of episodes24ProductionRunning time45 minsProduction companiesiQiyiKongfu PicturesOriginal releaseNetworkiQiyiReleaseJuly 19, 2017 (2017-07-19) Tientsin Mystic ...

Themed land at Disney theme parks This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Tomorrowland Disney Parks – news · newspapers · books · scholar · JSTOR (April 2024) (Learn how and when to remove this message) TomorrowlandDisneyland's Tomorrowland at nightThemeFuture, science, technology, outer space, ...

The dependency network approach provides a system level analysis of the activity and topology of directed networks. The approach extracts causal topological relations between the network's nodes (when the network structure is analyzed), and provides an important step towards inference of causal activity relations between the network nodes (when analyzing the network activity). This methodology has originally been introduced for the study of financial data,[1][2] it has been e...






