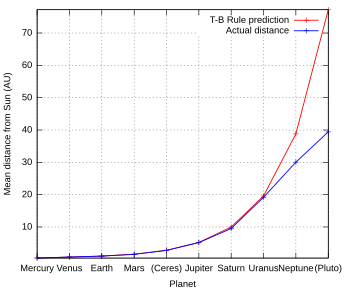
Định luật Titius–Bode
|
Read other articles:

American news and talk television show The Today Show redirects here. For other shows, see Today (1982 TV program) and Today (disambiguation) § Television. TodayAlso known asThe Today ShowGenreMorning news/talkCreated bySylvester WeaverPresented by Savannah Guthrie Hoda Kotb Al Roker Craig Melvin Carson Daly Third Hour: Sheinelle Jones Craig Melvin Dylan Dreyer Al Roker Hoda & Jenna: Hoda Kotb Jenna Bush Hager Saturday: Peter Alexander Laura Jarrett Joe Fryer Angie Lassman Sunday: W...

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目需要补充更多来源。 (2018年3月17日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:羅生門 (電影) — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 �...

For the FM radio station in Duluth, Minnesota (1940–1950), see WEBC-FM. This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (October 2015) (Learn how and when to remove this template message) This ar...

Chinese lunar probe launched in 2007 Chang'e 1Mission typeLunar orbiter[1][2]OperatorChina National Space AdministrationCOSPAR ID2007-051A SATCAT no.32273Mission durationPlanned: 1 year Achieved: 1 year, 4 months, 4 days Spacecraft propertiesLaunch mass2,350 kg[3] Start of missionLaunch date24 October 2007, 10:05:04.602 (2007-10-24UTC10:05:04Z) UTCRocketChang Zheng 3ALaunch siteXichang LC-3 End of missionDisposalDeorbited (Moon impact)Deca...

Part of a series onBritish law Acts of Parliament of the United Kingdom Year 1801 1802 1803 1804 1805 1806 1807 1808 1809 1810 1811 1812 1813 1814 1815 1816 1817 1818 1819 1820 1821 1822 1823 1824 1825 1826 1827 1828 1829 1830 1831 1832 1833 1834 1835 1836 1837 1838 1839 1840 1841 1842 1843 1844 1845 1846 1847 1848 1849 1850 1851 1852 1853 1854 1855 1856 1857 1858 1859 1860 1861 1862 1863 1864 1865 1866 1867 1868 1869 1870 1871 1872 1873 1874 1875 1876 1877 1878 ...

Canadian ice hockey player (born 1991) For persons of a similar name, see Daniel Carr (disambiguation). Ice hockey player Daniel Carr Carr at the 2019 AHL All-Star GameBorn (1991-11-01) November 1, 1991 (age 32)Sherwood Park, Alberta, CanadaHeight 6 ft 0 in (183 cm)Weight 186 lb (84 kg; 13 st 4 lb)Position Left wingShoots LeftNL teamFormer teams HC LuganoMontreal CanadiensVegas Golden KnightsNashville PredatorsWashington CapitalsNational team Cana...

Società Italiana ResineLogo Stato Italia Fondazione1931[1] a Milano Fondata daGiacomo Alessandro Girardi[1] Chiusura1981 (confluita in Eni) Sede principaleMilano Persone chiaveAngelo Rovelli Settore Chimica Chimica industriale ProdottiResine fenoliche Dipendenti13 000 (1975) Slogan«tutto dal petrolio» Modifica dati su Wikidata · Manuale La SIR, acronimo di Società Italiana Resine, conosciuta in Sardegna come Sarda Industria Resine[2][3], è s...

Tabel asosiasi antar huruf, rumah besar bulan, konstelasi Zodiak standar, dan musim, dari Shams al-Ma'ārif. Simiyah (Sīmiyā’; dari bahasa Arab Simah سِمة yang artinya tanda Yunani: σημεία, tanda-tanda) juga rūḥāniyya, atau ‘ilm al-ḥikma (Arab: روحانية dan علم الحكمةcode: ar is deprecated , berarti spiritualitas dan epistemologi kebijaksanaan, masing-masing) adalah doktrin yang umumnya ditemukan dalam tradisi Sufi-gaib yang dapat disimpulkan dari gagasan ...

Rubah kelabu Urocyon cinereoargenteus Status konservasiRisiko rendahIUCN22780 TaksonomiKerajaanAnimaliaFilumChordataKelasMammaliaOrdoCarnivoraFamiliCanidaeGenusUrocyonSpesiesUrocyon cinereoargenteus (Schreber, 1775) Tata namaProtonimCanis cinereoargenteus DistribusiPersebaran rubah kelabu lbs Rubah kelabu (Urocyon cinereoargenteus) adalah sebuah spesies mamalia omnivora dari keluarga Canidae. Spesies tersebut tersebar di Amerika Utara dan Amerika Tengah. Referensi ^ Cypher; et al. (2008). Uro...

L'ALÉAC a été approuvé par le Costa Rica, la République Dominicaine, Le Salvador, le Guatemala, le Honduras, le Nicaragua et les États-Unis d'Amérique. LLes présidents Francisco Flores Pérez, Ricardo Maduro, George W. Bush, Abel Pacheco, Enrique Bolaños et Alfonso Portillo. L'accord de libre-échange entre l'Amérique centrale, les États-Unis d'Amérique et la République Dominicaine (communément appelé accord de libre-échange d'Amérique centrale, ALÉAC) est un traité ratifi�...

淡江高峰塔倒塌事件高峰塔B座、C座公寓,與倒塌的A座公寓結構類似 (2012)日期1993年12月11日,30年前(1993-12-11)时间下午1时35分(马来西亚标准时间,周六)地点 马来西亚雪兰莪淡江(英语:Ulu Klang)山景花园(英语:Taman Hillview)高峰塔坐标3°10′33.4″N 101°45′42.1″E / 3.175944°N 101.761694°E / 3.175944; 101.761694坐标:3°10′33.4″N 101°45′42.1″E&...

Small computer with a CPU made out of a microprocessor Microcomputing redirects here. For the hobbyist magazine, see Kilobaud Microcomputing. For a small computer on an integrated circuit, see microcontroller. The Commodore 64 was one of the most popular microcomputers of its era, and is the best-selling model of home computers of all time.[1] Raspberry Pi, a popular modern-class microcomputer A microcomputer is a small, relatively inexpensive computer having a central processing unit...

Artikel ini perlu dikembangkan dari artikel terkait di Wikipedia bahasa Inggris. (Agustus 2023) klik [tampil] untuk melihat petunjuk sebelum menerjemahkan. Lihat versi terjemahan mesin dari artikel bahasa Inggris. Terjemahan mesin Google adalah titik awal yang berguna untuk terjemahan, tapi penerjemah harus merevisi kesalahan yang diperlukan dan meyakinkan bahwa hasil terjemahan tersebut akurat, bukan hanya salin-tempel teks hasil terjemahan mesin ke dalam Wikipedia bahasa Indonesia. Jan...

この記事には複数の問題があります。改善やノートページでの議論にご協力ください。 中立的な観点に基づく疑問が提出されています。(2020年11月) 独自研究が含まれているおそれがあります。(2020年11月) 2020年アメリカ合衆国大統領選挙2020 United States presidential election 2016年 ← 2020年11月3日 → 2024年 投票率 62.08% [1] 7.1% 候補者 ジョー・バイデン ド�...

Elisabetta di BrandeburgoDuchessa consorte di Brunswick-Calenberg-GöttingenIn carica7 luglio 1525 - 30 luglio 1540 NascitaCölln, 24 agosto 1510 MorteIlmenau, 25 maggio 1558 Luogo di sepolturaChiesa di san Giovanni a Schleusingen PadreGioacchino I, elettore di Brandeburgo MadreElisabetta di Danimarca Consorte diEric I, duca di Brunswick-Lüneburg Elisabetta di Brandeburgo (Cölln, 24 agosto 1510 – Ilmenau, 25 maggio 1558) è stata una principessa del Brandeburgo, duchessa consorte di...

Burial place of Joseph Smith and family in Illinois Gravestone for Joseph, Emma, and Hyrum Smith. The Smith Family Cemetery, in Nauvoo, Illinois, is the burial place of Joseph Smith, his wife Emma, and brother Hyrum. Joseph Smith's parents Joseph Smith Sr. and Lucy Mack Smith are also buried there,[1] as are Joseph Smith's brothers Samuel and Don Carlos. Others buried there include Robert B. Thompson and Emma Smith's second husband Lewis C. Bidamon. Overlooking the Mississippi River, ...

La carte des climats de Köppen-Geiger présentée par le US National Library of Medicine du National Institutes of Health[1] Af Am Aw et As BWh BWk BSh BSk Csa Csb Csc Dsa Dsb Dsc Dsd Cwa Cwb Cwc Dwa Dwb Dwc Dwd Cfa Cfb Cfc Dfa Dfb Dfc Dfd ET EF La classification de Köppen est une classification des climats fondée sur les précipitations et les températures. C'est le botaniste Wladimir Peter Köppen qui l'a inventée en 1900 en combinant la carte mondiale de la végétation publiée en 18...

ランドローバーLand Rover 種類 自動車所持会社 ジャガーランドローバー使用開始国 イギリス主要使用国 世界使用開始 1948年関連ブランド ジャガー旧使用会社 ランドローバー・リミテッドウェブサイト www.landrover.comテンプレートを表示 ランドローバー・リミテッドLand Rover Limited種類 株式会社略称 ランドローバー本社所在地 イギリスソリフル設立 1978年[1]業種 自動�...

Rabbis and poskim of the 11th–15th centuries Rabbinical eras Chazal Zugot Tannaim Amoraim Savoraim Geonim Rishonim Acharonim vte Rishonim (Hebrew: [ʁiʃoˈnim]; Hebrew: ראשונים, lit. 'the first ones'; sing. ראשון, Rishon) were the leading rabbis and poskim who lived approximately during the 11th to 15th centuries, in the era before the writing of the Shulchan Aruch (שׁוּלחָן עָרוּך, Set Table, a common printed code of Jewish law, 1563 CE) and...

2024年 7月(文月) 日 月 火 水 木 金 土 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 日付の一覧 各月 1 2 3 4 5 6 7 8 9 10 11 12 7月26日(しちがつにじゅうろくにち)は、グレゴリオ暦で年始から207日目(閏年では208日目)にあたり、年末まであと158日ある。 できごと 南米諸国の解放者、ホセ・デ・サン=マルティン(左画像)とシモン・ボリバル(右)が�...