Участник:Kaban2009
|
Read other articles:

Chet HuntleyChet Huntley, 1968Lahir10 Desember 1911Cardwell, Montana, Amerika SerikatMeninggal20 Maret 1974(1974-03-20) (umur 62)Big Sky, Amerika SerikatKebangsaanAmerika SerikatPekerjaanpembawa berita Chester Robert Chet Huntley (10 Desember 1911 – 20 Maret 1974) adalah pembawa berita di televisi Amerika Serikat. Karier Huntley dilahirkan di Cardwell, Montana. Setelah lulus Sekolah Menengah Atas Whitehall di Whitehall, Montana, ia kuliah Montana State College di Bozeman...

Peta Distrik Jhelum dan sungai Jhelum yang menjadi perbatasan dengan bagian barat Distrik Gujrat. Distrik Jhelum adalah sebuah distrik di Provinsi Punjab, Pakistan. Wilayah Distrik Jhelum terletak di sebelah barat Distrik Gujrat dan dilalui oleh sungai Jhelum. Keberadaan sungai Jhelum membuat lahan pertanian di Distrik Jhelum menjadi subur dan dapat menerapkan pertanian tadah hujan.[1] Referensi ^ Saleemi, Sanna (2016). Climate Change Adaptation Strategies among Farmers in the Gujrat ...

1969 novel by A.E. van Vogt This article is about the science fiction novel by A. E. van Vogt. For the English folk group, see The Silkie. This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: The Silkie novel – news · newspapers · books · scholar · JSTOR (April 2014) (Learn how and when to remove this template message) First ...

Design of road signs The examples and perspective in this article deal primarily with the United States and do not represent a worldwide view of the subject. You may improve this article, discuss the issue on the talk page, or create a new article, as appropriate. (December 2010) (Learn how and when to remove this template message) Traffic sign design involves any tasks in the process of designing traffic signage. Traffic signs may provide information about the law, warn about dangerous condi...

For places within the historic county boundaries (before 1974), see List of places historically in Lancashire. BlackburnBlackpoolBurnleyChorleyLancasterOrmskirkPrestonclass=notpageimage| Some of the larger settlements of Lancashire. This is a list of places within the ceremonial county of Lancashire, England. Contents: A B C D E F G H I J K L M N O P Q R S T U V W X Y Z See also External links A Abbey Village Abbeystead Accrington Acre Adlington Aldcliffe Altham Anderton Andertons ...

The following is an incomplete list of notable sushi restaurants. Sushi is a Japanese food composed of specially prepared vinegared rice combined with varied ingredients such as (chiefly) seafood (often uncooked), vegetables, egg, and occasionally tropical fruits. Styles of sushi and its presentation vary widely, but the key ingredient is sushi rice, also referred to as shari or sumeshi. Numerous traditions surround not only the preparation of sushi, but also its service and consumption. Int...

Music festival held in Gelsenkirchen, Germany Rock Hard FestivalGenreHeavy metalDatesweekend of PentecostLocation(s)Gelsenkirchen, GermanyYears active2003–presentWebsitewww.rockhardfestival.de Rock Hard Festival is a heavy metal festival organized and sponsored by the Rock Hard magazine. First established in Lichtenfels, Bavaria, Germany, in 1990 on an irregular basis,[1] mostly for magazine anniversaries, it is held annually in Gelsenkirchen, since 2003. Its 2016 edition was taken ...

この項目には、一部のコンピュータや閲覧ソフトで表示できない文字が含まれています(詳細)。 数字の大字(だいじ)は、漢数字の一種。通常用いる単純な字形の漢数字(小字)の代わりに同じ音の別の漢字を用いるものである。 概要 壱万円日本銀行券(「壱」が大字) 弐千円日本銀行券(「弐」が大字) 漢数字には「一」「二」「三」と続く小字と、「壱」「�...

Drs.Taufan SoekarnoputraM.A. Informasi pribadiLahirMohammad Taufan Soekarnoputra(1955-03-27)27 Maret 1955Istana Bogor, Jawa Barat, IndonesiaMeninggal17 Januari 1986(1986-01-17) (umur 30)Jakarta, IndonesiaMakamTaman Pemakaman Umum Karet BivakKebangsaanIndonesiaSuami/istriIryani Levana Danubrata (m. 1981)Orang tuaSoekarno (ayah) Hartini (ibu)Alma materInstitut Teknologi Bandung California State UniversitySunting kotak info • L • B Drs. M...

Pub in Richmond, London The Old Ship, RichmondLocation within London Borough of Richmond upon ThamesGeneral informationTypePublic houseLocation3 King Street, Richmond in the London Borough of Richmond upon Thames Listed Building – Grade IIOfficial nameOld Ship Public HouseDesignated25 June 1983Reference no.1286531 The Old Ship is a Grade II listed[1][2] public house at 3 King Street, Richmond in the London Borough of Richmond upon Thames. It was built in the 18th centur...

American professional wrestler Dick MurdochBirth nameHoyt Richard Murdoch[1]Born(1946-08-16)August 16, 1946[2]Waxahachie, Texas, United States[1]DiedJune 15, 1996(1996-06-15) (aged 49)[3]Amarillo, Texas, United States[3]Cause of deathMyocardial infarction[3]Alma materWest Texas State University[4]Children1FamilyFrankie Murdoch (father)[5]Killer Tim Brooks (cousin)Professional wrestling careerRing name(s)Big Daddy Murdoch[...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Fictional character from television series NCIS This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article describes a work or element of fiction in a primarily in-universe style. Please help rewrite it to explain the fiction more clearly and provide non-fictional perspective. (February 2019) (Learn how and when to remove this message) This article may contain minor, trivial or unrelate...

هذه المقالة تحتاج للمزيد من الوصلات للمقالات الأخرى للمساعدة في ترابط مقالات الموسوعة. فضلًا ساعد في تحسين هذه المقالة بإضافة وصلات إلى المقالات المتعلقة بها الموجودة في النص الحالي. (ديسمبر 2020) هوغو دي يونغ مناصب [1] في المنصب27 مايو 2010 – 26 أكتوبر 2017 [1]...

NATO Cooperative Cyber Defence Centre of Excellence Об'єднаний центр передових технологій з кібероборони НАТО Абревіатура NATO CCD COEТип агентство НАТОЗасновано 14 травня 2008[2] і 2008[3]Країна ЕстоніяШтаб-квартира Таллінн (59°25′23″ пн. ш. 24°46′03″ сх. д. / 59.42324722224999789° ...

Italian soldier Gian Giacomo Medici, Il Medeghino, in a 16th-century engraving Gian Giacomo Medici (25 January 1498 – 8 November 1555) was an Italian condottiero who became a noted Spanish general, Duke of Marignano and Marquess of Musso and Lecco in Lombardy. Biography Gian Giacomo Medici was the brother of Giovanni Angelo Medici, who was later to be elected Pope as Pius IV. They were scions of an impoverished, though patrician, family of Milan not connected with the Medici of Florence, in...
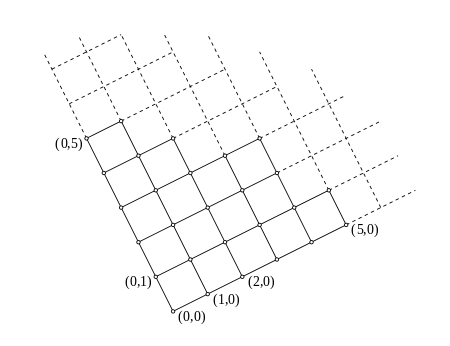
For the business concept, see purchase order. Hasse diagram of the product order on N {\displaystyle \mathbb {N} } × N {\displaystyle \mathbb {N} } In mathematics, given a partial order ⪯ {\displaystyle \preceq } and ⊑ {\displaystyle \sqsubseteq } on a set A {\displaystyle A} and B {\displaystyle B} , respectively, the product order[1][2][3][4] (also called the coordinatewise order[5][3][6] or componentwise order[2]...

Bilateral relationsAmerican-Norwegian relations Norway United States Diplomatic missionNorwegian Embassy, Washington, D.C.United States Embassy, Oslo The United States and Norway have a very long tradition of positive relations. The American Revolution of 1776 had a profound impact on Norway, and the democratic ideals of the United States Constitution served as a model for the authors of Norway's own Constitution of 1814. The close relationship between the two nations was reinforced by massiv...

Number whose divisors add to a multiple of that number Demonstration, with Cuisenaire rods, of the 2-perfection of the number 6 In mathematics, a multiply perfect number (also called multiperfect number or pluperfect number) is a generalization of a perfect number. For a given natural number k, a number n is called k-perfect (or k-fold perfect) if the sum of all positive divisors of n (the divisor function, σ(n)) is equal to kn; a number is thus perfect if and only if it is 2-perfect. A numb...