Бетонг (город, Таиланд)

У этого термина существуют и другие значения, см. Бетонг.
Город
Бетонг
тайск. เบตง
5°46′23″ с. ш. 101°04′21″ в. д.HGЯO
Страна  Таиланд
Провинция Яла
Внутреннее деление 27 тамбонов
История и география
Основан 1939
Площадь 78 км²
Высота центра 269 м
Тип климата тропический
Часовой пояс UTC+7:00
Население
Население 26 641 человек (2018)
Плотность 341 чел./км²
Официальный язык тайский
Цифровые идентификаторы
Почтовый индекс 95000
betongcity.go.th  (тайск.)
Показать/скрыть карты
Бетонг на карте
Бетонг
Бетонг
Логотип Викисклада Медиафайлы на Викискладе

Бетонг (тайск. เบตง) — город в Таиланде.

Название

Слово Бетонг является искажённым словом малайского языка означающего бамбук.

География

Город находится в провинции Яла в южной части Таиланда примерно в 890 км к югу от Бангкока. Через город протекает река Бетонг.

Бетонг располагается вблизи границы Таиланда с Малайзией. Бетонг является также самым южным городом Таиланда[1][2].

Население

По состоянию на 2018 год население города составляет 26 668 человек. Плотность населения — 341 чел/км². Численность женского населения (51,6 %) превышает численность мужского (48,4 %)[3]. Большинство являются малайцами, тайцами и китайцами[4][5]

51,92 % населения исповедует буддизм, 47,75 % исповедует ислам и около 1,4 % исповедует другие религии, включая христианство[6].

Экономика

Более 80 % населения Бетонга заняты в сельском хозяйстве. Также в городе развит туризм[7].

Транспорт

Дорожный

Через Бетонг проходит шоссе 410, которое начинается в Яле, и шоссе 76 из Перака, Малайзия.

Воздушный

В январе 2022 года в 13 км от города был открыт аэропорт Бетонга. Аэропорт управляется компанией Department of Airports.[8][9] Рассчитанный на 300 прибытий в час, аэропорт может обслуживать до миллиона пассажиров в год, принося району 3 миллиарда бат прибыли. Первой авиакомпанией, запустившей регулярные рейсы в Бетонг стала Nok Air.[8][10]

Примечания

  1. ↑ Attractions : Betong Town (недоступная ссылка) (англ.)
  2. ↑ Betong Airport to be built in Yala, southern of Thailand Архивная копия от 28 октября 2019 на Wayback Machine (англ.) (тайск.)
  3. ↑ รายงานสถิติจำนวนประชากรและบ้าน ประจำปี พ.ศ.2558 (тайск.). Дата обращения: 24 апреля 2016.
  4. ↑ เทศบาลเมืองเบตง ข้อมูลทั่วไป Архивировано 5 марта 2016 года.. เรียกดูเมื่อ 5 มีนาคม 2556
  5. ↑ ฐานข้อมูลรางวัลพระปกเกล้า. เทศบาลเมืองเบตง. เรียกดูเมื่อ 5 มีนาคม 2556
  6. ↑ แผนพัฒนาท้องถิ่น (พ.ศ. 2561-2565) เทศบาลเมืองเบตง. — เทศบาลเมืองเบตง, 2019. — P. 37. Архивная копия от 11 января 2022 на Wayback Machine
  7. ↑ The lessons of local governments. Betong (тайск.) (недоступная ссылка — история).
  8. ↑ 1 2 Karnjanatawe, Karnjana (19 августа 2021). "Betong airport set to open next month". Bangkok Post. Дата обращения: 19 августа 2021.
  9. ↑ "Govt plans DMK-Betong flight roster". Bangkok Post. 19 мая 2019. Дата обращения: 19 мая 2019.
  10. ↑ Nanuam, Wassana (5 июля 2019). "Betong airport runway will be extended, says army chief". Bangkok Post. Дата обращения: 5 июля 2019.

Read other articles:

Cook–Levin theorem

Boolean satisfiability is NP-complete and therefore that NP-complete problems exist In computational complexity theory, the Cook–Levin theorem, also known as Cook's theorem, states that the Boolean satisfiability problem is NP-complete. That is, it is in NP, and any problem in NP can be reduced in polynomial time by a deterministic Turing machine to the Boolean satisfiability problem. The theorem is named after Stephen Cook and Leonid Levin. An important consequence of this theorem is that ...

 

 

Christmas Humphreys

Christmas HumphreysChristmas HumphreysLahir(1901-02-15)15 Februari 19017 Royal Crescent, London, InggrisMeninggal13 April 1983(1983-04-13) (umur 82)St John's Wood, LondonKebangsaanBritania RayaPekerjaanBarrister; hakim, penulisTahun aktif1924–1976 Travers Christmas Humphreys (15 Februari 1901 – 13 April 1983)[1] adalah seorang barrister, Queen's Counsel, dan hakim Britania di Old Bailey.[2][3] Bersama dengan istrinya, Aileen Faulkner, Christmas Humphreys...

 

 

Roerich Pact

International treaty for the protection of important cultural heritage Roerich PactThe Treaty on Protection of Artistic and Scientific Institutions and Historic MonumentsSigned15 April 1935LocationWashington, D.C.Effective26 August 1935Conditiontwo ratifying statesSignatories21Parties10DepositaryPan American Union The Treaty on the Protection of Artistic and Scientific Institutions and Historic Monuments or Roerich Pact is an inter-American treaty. The most important idea of the Roerich Pact ...

ماكس بينغهام

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (مارس 2019) سير  ماكس بينغهام (بالإنجليزية: Max Bingham)‏  مناصب عضو مجلس نواب تسمانيا   عضو خلال الفترة10 مايو 1969  – 13 يونيو 1984  الدائرة الإنتخابية دنيسون  [لغ�...

 

 

Demographics of Malta

Demographics of MaltaPopulation pyramid of Malta in 2020Population519,186 (2021 census)Growth rate0.67% (2022 est.)Birth rate9.73 births/1,000 populationDeath rate8.48 deaths/1,000 populationLife expectancy83.2 years • male81.11 years • female85.38 yearsFertility rate1.5 childrenInfant mortality rate4.53 deaths/1,000 live birthsNet migration rate5.49 migrant(s)/1,000 populationSex ratioTotal1.02 male(s)/female (2022 est.)At birth1.04 male(s)/femaleNationalityNati...

 

 

俄亥俄州

  「俄亥俄」重定向至此。关于其他用法，请见「俄亥俄 (消歧义)」。 俄亥俄州 美國联邦州State of Ohio 州旗州徽綽號：七葉果之州地图中高亮部分为俄亥俄州坐标：38°27'N－41°58'N, 80°32'W－84°49'W国家 美國加入聯邦1803年3月1日，在1953年8月7日追溯頒定（第17个加入联邦）首府哥倫布（及最大城市）政府 • 州长（英语：List of Governors of {{{Name}}}]]） •&...

Fábio Simplício

Fábio Simplício Informasi pribadiNama lengkap Fábio SimplícioTanggal lahir 23 September 1979 (umur 44)Tempat lahir São Paulo, BrasilPosisi bermain GelandangKarier senior*Tahun Tim Tampil (Gol)2000-2004 São Paulo 2004-2006 Parma 2006-2010 Palermo 2010-2012 Roma 2012-2013 Cerezo Osaka 2014- Vissel Kobe Tim nasional2009 Brasil 1 (0) * Penampilan dan gol di klub senior hanya dihitung dari liga domestik Fábio Simplício (lahir 23 September 1979) adalah pemain sepak bola asal Brasil. S...

 

 

De la Terre à la Lune

Pour les articles homonymes, voir De la Terre à la Lune (homonymie). De la Terre à la Lune trajet direct en 97 heures 20 minutes Arrivée du projectile tout juste fabriqué. Auteur Jules Verne Pays France Genre Roman d'anticipation Éditeur Pierre-Jules Hetzel Collection Voyages extraordinaires Date de parution 1865 Illustrateur Henri de Montaut (gravures de François Pannemaker) Chronologie Voyage au centre de la Terre Les Enfants du capitaine Grant modifier  De la Terre à la Lune, ...

 

 

Contea di Dubois

Questa voce sull'argomento contee dell'Indiana è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Contea di DuboisconteaLocalizzazioneStato Stati Uniti Stato federato Indiana AmministrazioneCapoluogoJasper Data di istituzione1817 TerritorioCoordinatedel capoluogo38°21′36″N 86°52′48″W38°21′36″N, 86°52′48″W (Contea di Dubois) Superficie1 127 km² Abitanti39 674 (2000) Densità35,2 ab./km² Altre informazioniFus...

Hilaliens

(fr)Banu Hilal(ar)بَنُو هِلَالٍHilalites Tribu arabe des Banu Hilal, dans le sud d'Oran en Algérie, 1888. Période Xe – XIIIe siècle Ethnie Arabes (Arabes du Nord) Hawazin Langue(s) Arabe Religion Islam Région d'origine Arabie Région actuelle Algérie Tunisie Libye Égypte Maroc Mauritanie Arabie saoudite Syrie Irak Qatar Yémen Émirats arabes unis modifier  Les Banu Hilal, Hilalites ou Hilaliens (en arabe : بنو هلال) étaient une confédération ...

 

 

The Last Nude

2012 novel by Ellis Avery The Last Nude AuthorEllis AveryCover artistTamara de LempickaLanguageEnglishGenreHistorical novelPublisherRiverhead BooksPublication date2012Publication placeUnited StatesMedia typePrintPages320 pp (first edition, hardback)ISBN978-1594488139 (first edition, hardback)OCLC883297216 The Last Nude is a work of historical fiction by Ellis Avery published by Riverhead in the US in 2012. Set primarily in 1927 Paris, the book is largely inspired by events in the li...

 

 

بطولة أوروبا للألعاب المائية 1958

بطولة أوروبا للألعاب المائية 1958 البطولة بطولة أوروبا للألعاب المائية رقم الموسم التاسع التاريخ 31 أغسطس–6 سبتمبر 1958 المكان بودابست،  المجر الفائزون الأول   الاتحاد السوفيتي الثاني   المملكة المتحدة الثالث   هولندا 1954 1962 تعديل مصدري - تعديل   بطولة أورو�...

Slugger Labbe

NASCAR crew chief Slugger LabbeLabbe at Kentucky Speedway in 2016Personal informationFull nameRichard LabbeNicknameSluggerNationalityAmericanBorn (1968-06-14) June 14, 1968 (age 56)Saco, MaineSportCountryUnited StatesSportNASCAR Camping World Truck Series, NHRA Richard Slugger Labbe (born June 14, 1968) is an American technical consultant who currently works for Toyota Racing Development. He had previously been employed in NASCAR as a crew chief for Yates Racing, Dale Earnhardt, Inc...

 

 

2022 Tanauan local elections

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 2022 Tanauan local elections – news · newspapers · books · scholar · JSTOR (November 2021) (Learn how and w...

 

 

KIAI

Not to be confused with kiai. Radio station in Mason City, IowaKIAIMason City, IowaBroadcast areaMason City, IowaAustin–Albert Lea, MinnesotaFrequency93.9 MHzBranding93.9 The Country MooseProgrammingFormatCountryAffiliationsPremiere NetworksPerformance Racing NetworkWestwood OneOwnershipOwnerAlpha Media(Alpha 3E Licensee LLC)Sister stationsKGLO, KLSS-FM, KRIB, KYTCHistoryFirst air date1985 (as KNIQ)Former call signsKNIQ (1985–1991)Former frequencies93.5 MHz (1985-1992)Call sign meaningK-I...

Marco Brandolin

Questa voce o sezione sull'argomento calciatori è priva o carente di note e riferimenti bibliografici puntuali. Sebbene vi siano una bibliografia e/o dei collegamenti esterni, manca la contestualizzazione delle fonti con note a piè di pagina o altri riferimenti precisi che indichino puntualmente la provenienza delle informazioni. Puoi migliorare questa voce citando le fonti più precisamente. Segui i suggerimenti del progetto di riferimento. Marco BrandolinNazionalità Italia Cal...

 

 

Emblème de Macao

Cet article est une ébauche concernant Macao. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. L'actuel emblème entra en vigueur le 20 décembre 1999 quand le Portugal rétrocéda Macao à la république populaire de Chine. Le blason a maintenant la désignation de « blason régional ». Il représente en cercle les éléments du drapeau de Macao. Dans le cercle blanc on peut lire dans la légende le...

 

 

花園橋

「花園橋」のその他の用法については「花園橋 (曖昧さ回避)」をご覧ください。 花園橋（2014年3月） 花園橋（はなぞのはし）は埼玉県大里郡寄居町赤浜と深谷市荒川の間に架かり、荒川を渡る埼玉県道296号菅谷寄居線の道路橋である。花園大橋とも呼ばれる[1]。 概要 河口から89.8 kmの地点に架かる[2] 総延長879.80メートル[3]、幅員9.75メートル[4&#...

Nicola Quaglio

Nicola QuaglioDati biograficiPaese Italia Altezza185 cm Peso115 kg FamiliariIsidoro Quaglio (zio) Rugby a 15 RuoloPilone Squadra Rovigo CarrieraAttività di club[1] 2009-16 Rovigo98 (30)2021- Rovigo Attività in franchise 2016-21 Benetton44 (5) Attività da giocatore internazionale 2016-2019 Italia14 (0) 1. A partire dalla stagione 1995-96 le statistiche di club si riferiscono ai soli campionati maggiori professionistici di LegaIl simbolo → indica un trasferimento i...

 

 

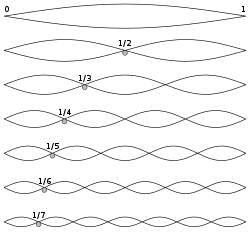
Fundamental frequency

Lowest frequency of a periodic waveform, such as sound Vibration and standing waves in a string, The fundamental and the first six overtones The fundamental frequency, often referred to simply as the fundamental (abbreviated as f0), is defined as the lowest frequency of a periodic waveform.[1] In music, the fundamental is the musical pitch of a note that is perceived as the lowest partial present. In terms of a superposition of sinusoids, the fundamental frequency is the lowest freque...