Read other articles:

Bagian dari seriGereja Katolik menurut negara Afrika Afrika Selatan Afrika Tengah Aljazair Angola Benin Botswana Burkina Faso Burundi Chad Eritrea Eswatini Etiopia Gabon Gambia Ghana Guinea Guinea-Bissau Guinea Khatulistiwa Jibuti Kamerun Kenya Komoro Lesotho Liberia Libya Madagaskar Malawi Mali Maroko Mauritania Mauritius Mesir Mozambik Namibia Niger Nigeria Pantai Gading Republik Demokratik Kongo Republik Kongo Rwanda Sao Tome dan Principe Senegal Seychelles Sierra Leone Somalia Somaliland ...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada November 2022. A.I. RisingSutradaraLazar BodrožaProduserAleksandar Protić, Jonathan EnglishSkenarioDimitrije VojnovBerdasarkanCerpen 1980-an:Zoran NeškovićPemeran Sebastian Cavazza Stoya Marusa Majer Kirsty Besterman Penata musikNemanja MosurovicSinematogr...

The Blood of WolvesPoster filmNama lainNama JepangKanji 孤狼の血 SutradaraKazuya ShiraishiProduserKazuto AmanoSkenarioJun'ya IkegamiBerdasarkanKorō no Chioleh Yūko YuzukiPemeran Kōji Yakusho Tori Matsuzaka Yōko Maki NaratorIssei FutamataPenata musikGorō YasukawaPenyuntingHitomi KatōDistributorToeiTanggal rilis 12 Mei 2018 (2018-05-12) Durasi126 menitNegaraJepangBahasaJepangPendapatankotor$5,908,700[1] The Blood of Wolves (Jepang: 孤狼の血code: ja is depr...

Сухопутные войска Италииитал. Esercito Italiano Герб сухопутных войск Италии Страна Италия Подчинение Министерство обороны Италии Входит в Вооружённые силы Италии Тип вид вооружённых сил Функция сухопутные войска Численность 93 100 (2023)[1] Дислокация Рим Снаряжение см. н...

Taman Nasional Way KambasIUCN Kategori II (Taman Nasional)TN Way KambasLokasi TNWK di SumatraLetakKabupaten Lampung Timur, Lampung, Sumatra, IndonesiaKota terdekatBandar Lampung MetroKoordinat4°55′S 105°45′E / 4.917°S 105.750°E / -4.917; 105.750Koordinat: 4°55′S 105°45′E / 4.917°S 105.750°E / -4.917; 105.750Luas1.300 km2[1]Didirikan1989[1]Pengunjung2.553 (tahun 2007[2])Pihak pengelolaKementerian Lingkungan...

American politician George Meade BowersGeorge M. Bowers during his tenure as U.S. Commissioner of Fish and Fisheries.Member of the U.S. House of Representativesfrom West Virginia's 2nd districtIn officeMay 9, 1916 – March 3, 1923Preceded byWilliam Gay Brown Jr.Succeeded byRobert E. Lee Allen Personal detailsBorn(1863-09-13)September 13, 1863Gerrardstown, West Virginia, U.S.DiedDecember 7, 1925(1925-12-07) (aged 62)Martinsburg, West VirginiaPolitical partyRepublican...

Officer's rank in the Schutzstaffel (SS) HauptsturmführerSS collar patchesCountry Nazi GermanyService branch Schutzstaffel Sturmabteilung National Socialist Motor Corps National Socialist Flyers CorpsAbbreviationHstufNATO rank codeOF-2Formation1934Abolished1945Next higher rankSturmbannführerNext lower rankObersturmführerEquivalent ranksHauptmann Hauptsturmführer Albert Klett, photographed in 1945 Hauptsturmführer (German: [ˈhaʊpt.ʃtʊʁmˌfyːʁɐ], lit. 'head storm...

Hindi cinema 1920s 1920 1921 1922 1923 19241925 1926 1927 1928 1929 1930s 1930 1931 1932 1933 19341935 1936 1937 1938 1939 1940s 1940 1941 1942 1943 19441945 1946 1947 1948 1949 1950s 1950 1951 1952 1953 19541955 1956 1957 1958 1959 1960s 1960 1961 1962 1963 19641965 1966 1967 1968 1969 1970s 1970 1971 1972 1973 19741975 1976 1977 1978 1979 1980s 1980 1981 1982 1983 19841985 1986 1987 1988 1989 1990s 1990 1991 1992 1993 19941995 1996 1997 1998 1999 2000s 2000 2001 2002 2003 20042005 2006 200...

Research institute in Slovenia The Central European Institute Søren Kierkegaard The Central European Institute Søren Kierkegaard was established after the 4th International Philosophical Symposium of Miklavž Ocepek,[1] organized by KUD Apokalipsa in June 2013 to commemorate the bicentennial of the birth of Søren Kierkegaard at the initiative of dr. Primož Repar,[2] who committed himself to the lifelong study of the thoughts of the pioneer of existentialism and is also the...

Sceaux 行政国 フランス地域圏 (Région) イル＝ド＝フランス地域圏県 (département) オー＝ド＝セーヌ県郡 (arrondissement) アントニー郡小郡 (canton) 小郡庁所在地INSEEコード 92071郵便番号 92330市長（任期） フィリップ・ローラン（2008年-2014年）自治体間連合 (fr) メトロポール・デュ・グラン・パリ人口動態人口 19,679人（2007年）人口密度 5466人/km2住民の呼称 Scéens地理座標 北緯48度4...

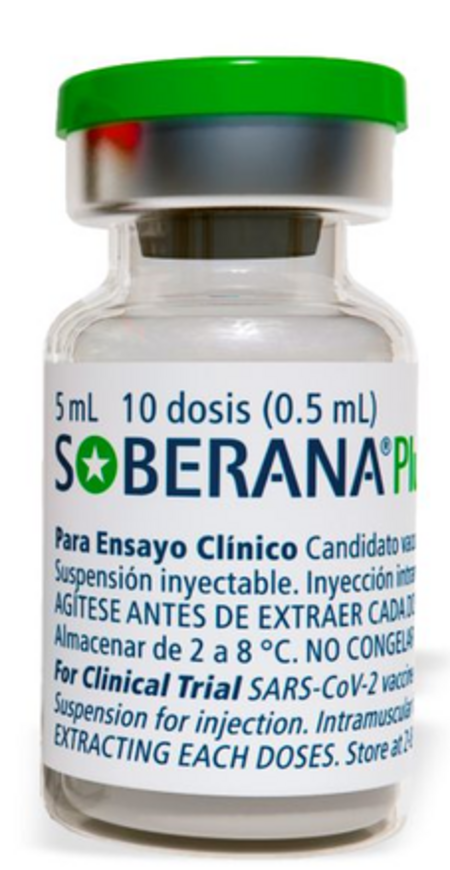
Vaccine Soberana PlusA vial of Soberana PlusVaccine descriptionTargetSARS-CoV-2Vaccine typeConjugateClinical dataTrade namesPasteurOther namesFINLAY-FR-1ARoutes ofadministrationIntramuscularLegal statusLegal status Full list of Soberana Plus authorizations Part of a series on theCOVID-19 pandemicScientifically accurate atomic model of the external structure of SARS-CoV-2. Each ball is an atom. COVID-19 (disease) SARS-CoV-2 (virus) Cases Deaths Timeline 2019 2020 January responses February res...

مصباح يعمل على غاز الأستيلين. المصباح الكربيدي أو مصباح غاز الأستلين هي مصابيح بدائية تنتج وتحرق غاز الأسيتيلين، والذي ينتج عن تفاعل كربيد الكالسيوم مع الماء. [1] مصابيح غاز الأسيتيلين استخدمت سابقاً لإنارة المباني، كالمنارات، ومصابيح السيارات والدراجات...

乔冠华 中华人民共和国外交部部长 中国人民对外友好协会顾问 任期1974年11月—1976年12月总理周恩来 → 华国锋前任姬鹏飞继任黄华 个人资料性别男出生(1913-03-28)1913年3月28日 中華民國江蘇省盐城县逝世1983年9月22日(1983歲—09—22)（70歲） 中华人民共和国北京市籍贯江蘇鹽城国籍 中华人民共和国政党 中国共产党配偶明仁（1940年病逝） 龚澎（1970年病逝） 章含�...

Elle WoodsReese Witherspoon bermain sebagai Elle Woods dalam dua film Legally BlondePenampilanperdanaLegally BlondePenampilanterakhirLegally BlondesPenciptaAmanda BrownPemeranReese WitherspoonInformasiJenis kelaminPerempuanPekerjaanPengacaraKewarganegaraanAmerika Serikat Elle Woods adalah karakter protagonis novel karya Amanda Brown, Legally Blonde dan tokoh dalam film yang berjudul sama. Latar belakang Elle adalah seorang gadis pirang yang atraktif dari California. Dia kuliah di Harvard Law ...

French statesman (1851–1925) Léon BourgeoisPresident of the Council of Ministers of FranceIn office1 November 1895 – 29 April 1896PresidentFélix FaurePreceded byAlexandre RibotSucceeded byJules MélinePresident of the SenateIn office14 January 1920 – 22 February 1923Preceded byAntonin DubostSucceeded byGaston DoumerguePresident of the Chamber of DeputiesIn office6 June 1902 – 12 January 1904Preceded byPaul DeschanelSucceeded byHenri BrissonMinister of Fore...

  Administrative law in MongoliaMongolian LawsConstitution of Mongolia Mongolian nationality law Administrative law in Mongolia LGBT rights in MongoliaMongolia Administrative law General principles Administrative court Delegated legislation Exhaustion of remedies Justiciability Ministerial act Ouster clause Prerogative writ Certiorari Habeas corpus Mandamus Prohibition Quo warranto Rulemaking Ultra vires Grounds for judicial review Fettering of discretion Legitimate expectation Nond...

Allsvenskan IF Elfsborg – Malmö FF (1–2) i en match på Borås Arena.Säsong2018Nya lag för säsongenIF BrommapojkarnaDalkurd FFTrelleborgs FFVinnareAIK(6:e allsvenska titeln)(12:e SM-titeln)NedflyttadeDalkurd FFTrelleborgs FFIF Brommapojkarna (via kval)Champions LeagueAIKEuropa LeagueIFK NorrköpingMalmö FFStatistikSpelade matcher240Totalt antal mål654 (2,7 per match)Bästa målgörare Paulinho (20 mål)Största hemmavinstHäcken 6–0 Brommapojkarna(26 augusti 20...

Collection of Leonardo da Vinci's writings A Treatise on Painting (Trattato della pittura), 1651 A Treatise on Painting (Trattato della pittura) is a collection of Leonardo da Vinci's writings entered in his notebooks under the general heading On Painting. The manuscripts were begun in Milan while Leonardo was under the service of Ludovico Sforza and gathered together by his heir Francesco Melzi. An abridged version of the treatise was first published in France in 1651. After Melzi's manuscri...

Hot metal typesetting machine (1886–1980s) Paper tape controlled Linotype Model 5cS, manufactured in Germany (on display at Deutsches Museum, Munich) The Linotype machine (/ˈlaɪnətaɪp/ LYNE-ə-type) is a line casting machine used in printing which is manufactured and sold by the former Mergenthaler Linotype Company and related companies.[1] It was a hot metal typesetting system that cast lines of metal type for one-time use. Linotype became one of the mainstays for typesetting, ...

Diagrama de la función y de los rectángulos de suma de Riemann. En matemáticas, una partición Π de un intervalo cerrado [a, b] en los números reales es una secuencia finita de la forma a = x0 < x1 < x2 <... < xn = b. Estas particiones se utilizan en la teoría de la integral de Riemann y la integral de Riemann-Stieltjes. Refinamiento de una partición Se dice que una partición Π' es más fina que una partición Π cuando Π es un subconjunto de Π', es decir, cuando la par...