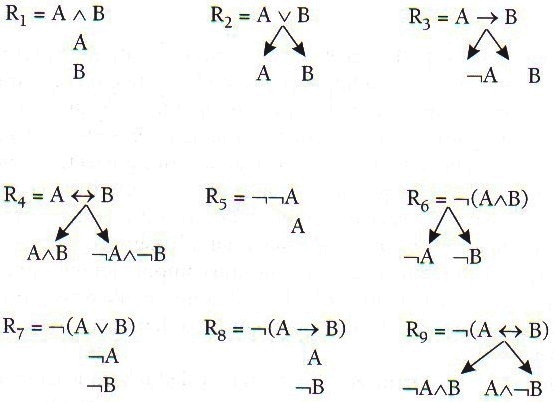
Método dos Tableaux
|
Read other articles:

Ir. H.Irfan Suryanagara Wakil Ketua DPRD Jawa BaratMasa jabatan22 Oktober 2014 – 1 September 2019Menjabat bersama Periode 2014–19 Irianto MS Syafiuddin Haris Yuliana Abdul Haris Bobihoe PresidenJoko WidodoGubernurAhmad HeryawanIwa Karniwa (Plt.)Mochammad Iriawan (Plt.)Ridwan Kamil PenggantiAchmad Ru'yatIneu Purwadewi SundariAde BarkahOleh SolehKetua DPRD Jawa BaratMasa jabatan16 Oktober 2009 – 31 Agustus 2014PresidenSusilo Bambang YudhoyonoGubernurAhmad Heryaw...

Universitas TartuTartu ÜlikoolLatin: Universitas Tartuensiscode: la is deprecated Nama sebelumnyaAcademia Gustaviana, Universitas Dorpat/Yuryev, Universitas Negeri TartuJenisUmumDidirikan1632 (ditutup 1710–1802)RektorToomas AsserJumlah mahasiswa17.100 (2008)LokasiTartu, Estonia58°22′52″N 26°43′13″E / 58.38111°N 26.72028°E / 58.38111; 26.72028KampusPerkotaanWarnabiru, putihNama julukanUTAfiliasiCBUR, EUA, Grup Coimbra, Utrecht Network , Atomium CultureSit...

Part of a series onBritish law Acts of Parliament of the United Kingdom Year 1801 1802 1803 1804 1805 1806 1807 1808 1809 1810 1811 1812 1813 1814 1815 1816 1817 1818 1819 1820 1821 1822 1823 1824 1825 1826 1827 1828 1829 1830 1831 1832 1833 1834 1835 1836 1837 1838 1839 1840 1841 1842 1843 1844 1845 1846 1847 1848 1849 1850 1851 1852 1853 1854 1855 1856 1857 1858 1859 1860 1861 1862 1863 1864 1865 1866 1867 1868 1869 1870 1871 1872 1873 1874 1875 1876 1877 1878 ...

غوانجو (بالصينية: 广州市) خريطة الموقع تقسيم إداري البلد الصين [1][2] العاصمة يويكسيو عاصمة لـ غوانغدونغ (971–) التقسيم الأعلى غوانغدونغ (14 أكتوبر 1949–) خصائص جغرافية إحداثيات 23°08′N 113°16′E / 23.13°N 113.26°E / 23.13; 113.26 [3] المساحة 7248.86 �...

Species of flowering plant Blepharidachne kingii Conservation status Apparently Secure (NatureServe)[1] Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Monocots Clade: Commelinids Order: Poales Family: Poaceae Genus: Blepharidachne Species: B. kingii Binomial name Blepharidachne kingii(S.Wats.) Hack. Blepharidachne kingii is a species of grass known by the common name King's eyelashgrass. It is native to the Great Basin in the Unite...

Les troupes indépendantistes se battent contre les royalistes d'Agualongo. La peinture du XIXe siècle montre Bolívar à la tête de son armée régulière. Une armée régulière est l'armée officielle d'un État ou pouvoir légalement constitué. Elle s'oppose aux armées irrégulières, telles que les guérillas, armées privées, mercenaires, etc. L'armée régulière se divise habituellement en armée permanente, qui est toujours sur les armes, et armée de réserve, qui est acti...

Daily prayers in Sikhism A typical Nitnem Gutka, or Sikh Prayer book, extremely common in many Sikh households Part of a series onSikh practices and discipline Foundations of Practice Simran Sewa Three pillars Naam Japo Kirat Karo Vand Chhako Discipline Five Virtues Five Thieves Five Ks Rehat Prohibitions in Sikhism Daily Prayers and Hymns Nitnem Amrit Velā Communal Practices Daswandh Langar Kirtan Liturgical Practices Akhand Pāth Sadharan Pāth Ardās Hukam Sanskars Naam Karan Amrit Sanska...

American soldier William Harvey CarneyCarney, wearing the Medal of Honor he received in 1900.Born(1840-02-29)February 29, 1840Norfolk, Virginia, U.S.DiedDecember 9, 1908(1908-12-09) (aged 68)New Bedford, Massachusetts, U.S.Place of burialOak Grove Cemetery, New Bedford, Massachusetts, U.S.AllegianceUnited States (Union)Service/branchU.S. Army (Union Army)Years of service1863–1864RankSergeantUnit 54th Regiment Massachusetts Volunteer InfantryBattles/warsAmerican Civil War Secon...

Excessive concern for one's own appearance, or importance For other uses, see Vanity (disambiguation). Vainglory redirects here. For the Old English poem, see Vainglory (Old English poem). For the video game, see Vainglory (video game). Vanitas (Latin for vanity) by Léon Bazille Perrault, 1886 Vanity is the excessive belief in one's own abilities or attractiveness to others. Prior to the 14th century, it did not have such narcissistic undertones, and merely meant futility.[1] The rel...

Street in Dublin, Ireland This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Gardiner Street – news · newspapers · books · scholar · JSTOR (December 2020) (Learn how and when to remove this message) Gardiner StreetElevated railway bridge crossing Gardiner StreetNative nameSráid Ghairdinéir (Irish)Sráid ...

Artikel ini bukan mengenai Park Gun-hyung. Dalam nama Korean ini, nama keluarganya adalah Park. Park Geun-hyungLahir7 Juni 1940 (umur 83)Jeongeup, Provinsi Jeolla Utara, Korea SelatanNama lainPark Keun-hyongPendidikanUniversitas Chung-Ang - Teater dan FilmPekerjaanAktorTahun aktif1963–sekarangAgenSB EntertainmentAnakPark Sang-hoon (son)Nama KoreaHangul박근형 Hanja朴根瀅 Alih AksaraBak Geun-hyeongMcCune–ReischauerPak Kŭn-hyŏng Park Geun-hyung (lahir 7 Juni 1940) adal...

Contoh artefak yang ditemukan di Banpo. Banpo (Hanzi: 半坡; Pinyin: Bànpō) adalah suatu situs arkeologi yang terletak di lembah Sungai Kuning sedikit di timur Xi'an, Cina dan pertama kali ditemukan pada tahun 1953. Situs ini mengandung peninggalan beberapa pemukiman Neolitik yang terkelola baik dan berasal dari sekitar 4500 SM. Situs ini mencakup suatu wilayah yang luas berukuran sekitar 60 km² dan dikelilingi oleh parit dengan lebar lima sampai enam meter yang kemungkinan m...

Swimming pool with artificial waves This article may need to be rewritten to comply with Wikipedia's quality standards. You can help. The talk page may contain suggestions. (July 2021) An outdoor wave pool in action A wave pool is a swimming pool in which there are artificially generated, large waves, similar to those of the ocean. Wave pools are often a major feature of water parks, both indoors and outdoors, as well as some leisure centres. Manufacturers Wave pools are constructed by many d...

2016年美國總統選舉 ← 2012 2016年11月8日 2020 → 538個選舉人團席位獲勝需270票民意調查投票率55.7%[1][2] ▲ 0.8 % 获提名人 唐納·川普 希拉莉·克林頓 政党 共和黨 民主党 家鄉州 紐約州 紐約州 竞选搭档 迈克·彭斯 蒂姆·凱恩 选举人票 304[3][4][註 1] 227[5] 胜出州/省 30 + 緬-2 20 + DC 民選得票 62,984,828[6] 65,853,514[6]...

Toxocaridae Telur Toxocara berembrio Klasifikasi ilmiah Domain: Eukaryota Kerajaan: Animalia Filum: Nematoda Kelas: Chromadorea Ordo: Ascaridida Famili: ToxocaridaeHartwich, 1954 Genus[1][2] Neoascaris Porrocaecum Toxocara Toxocaridae adalah keluarga nematoda zoonosis yang menginfeksi canidae dan felidae dan menyebabkan toksokariasis pada manusia (visceral larva migrans dan okular larva migrans).[3] Cacing ini tidak dapat bereproduksi pada manusia. Infeksi Infeksi T. ...
Town in New South Wales, AustraliaDilpurraNew South WalesDilpurraLocation in New South WalesCoordinates35°12′S 143°42′E / 35.200°S 143.700°E / -35.200; 143.700Population29 (SAL 2021)[1]Postcode(s)2734Location 57 km (35 mi) from Moulamein 15 km (9 mi) from Swan Hill LGA(s)Murray River CouncilCountyWakoolState electorate(s)MurrayFederal division(s)Farrer Dilpurra is a village community in the south western part of the Riverina in New ...

أتش 264الشعارمعلومات عامةجزء من إم بي إي جي - 4ITU-T recommendations H-series (en) المُطوِّر إم بي إي جيمجموعة خبراء ترميز الفيديو موقع الويب itu.int…[1] (الإنجليزية، الإسبانية، الفرنسية) نوع الوسائط video/H264[2] H.263 (en) Recommendation H.265: High efficiency video coding (en) ترميز الفيديو عالي الفعالية تعديل - تعد...

King of Sweden since 1973 For other Swedish royalty named Carl, see Carl of Sweden (disambiguation). Carl XVI GustafCarl Gustaf in 2018King of SwedenReign15 September 1973 – presentEnthronement19 September 1973PredecessorGustaf VI AdolfHeir apparentVictoriaPrime ministers See list Olof PalmeThorbjörn FälldinOla UllstenIngvar CarlssonCarl BildtGöran PerssonFredrik ReinfeldtStefan LöfvenMagdalena AnderssonUlf Kristersson Born (1946-04-30) 30 April 1946 (age 78)Haga Palace, Solna, Swe...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (July 2012) (Learn how and when to remove this message) The neutrality of this article is disputed. Relevant discussion may be found on the talk page....

Mountain in Switzerland and Italy For the mountain of the Cottian Alps, see Monte San Giorgio (Cottian Alps). Monte San GiorgioMonte San Giorgio (right), among Lake Lugano and Monte Generoso (left, background)Highest pointElevation1,097 m (3,599 ft)Prominence758 m (2,487 ft)[1]Coordinates45°54′49″N 8°56′59″E / 45.91361°N 8.94972°E / 45.91361; 8.94972GeographyMonte San GiorgioLocation in SwitzerlandShow map of Canton of Ticin...