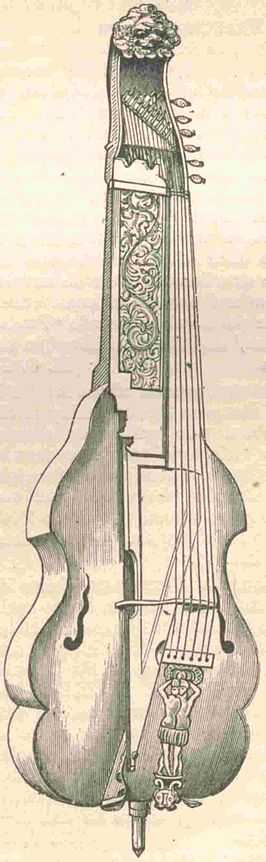
Barytone
| ||||||||||||||||||||||||||||||
Read other articles:

Unsolved murders in 19th century London The Thames Torso Murders, often called the Thames Mysteries or the Embankment Murders, were a sequence of unsolved murders of women occurring in London, England from 1887 to 1889. The series included four incidents which were filed as belonging to the same series. None of the cases were solved, and only one of the four victims was identified. In addition, other murders of a similar kind, taking place between 1873 and 1902, have also been associated with...

Simon van der MeerSimon van der MeerLahir(1925-11-24)24 November 1925Den Haag, BelandaMeninggal4 Maret 2011(2011-03-04) (umur 85)Jenewa, SwissKebangsaanBelandaDikenal ataspendinginan stokastikPenghargaanPenghargaan Nobel dalam FisikaKarier ilmiahBidangFisika Simon van der Meer (24 November 1925 – 4 Maret 2011) adalah seorang fisikawan akselerator Belanda yang menemukan konsep pendinginan stokastik pada pertumbukan, yang memungkinkan penemuan partikel W dan Z pada benda t...

برج التنين (龍) أحد الأبراج الصينية الأثني عشر. التنين هو الحيوان الوحيد الخرافي الممثل في الأبراج الصينية, فالبقية حيوانات حقيقية. وهو بالعادة يرافق رمز «فروع الأرض» (辰). يتصف مولود هذا العام بطبعة الودود الحساس, الصدق والشجاعة. يتفق بالأخص مع مواليد ابراج الفأر، الأفعى، ال...

German composer, musicologist, opera director and conductor (1943–2021) Udo ZimmermannZimmermann in 2006Born6 October 1943Dresden, Saxony, GermanyDied22 October 2021(2021-10-22) (aged 78)Dresden, GermanyEducation Dresdner Kreuzchor Musikhochschule Dresden Occupations Composer Musicologist Conductor Opera director Organizations Musikhochschule Dresden Dresden Center for Contemporary Music Leipzig Opera Deutsche Oper Berlin Udo Zimmermann (6 October 1943[1] – 22 October 2021 ...

Shunsuke Maeda Informasi pribadiNama lengkap Shunsuke MaedaTanggal lahir 9 Juni 1986 (umur 37)Tempat lahir Prefektur Nara, JepangPosisi bermain PenyerangKarier senior*Tahun Tim Tampil (Gol)2004-2007 Sanfrecce Hiroshima 2007-2011 Oita Trinita 2010 →FC Tokyo 2012-2015 Consadole Sapporo 2016- Gainare Tottori * Penampilan dan gol di klub senior hanya dihitung dari liga domestik Shunsuke Maeda (lahir 9 Juni 1986) adalah pemain sepak bola asal Jepang. Karier Shunsuke Maeda pernah bermain un...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (نوفمبر 2019) كأس الاتحاد الأوروبي للسيدات 2004–05 تفاصيل الموسم دوري أبطال أوروبا للسيدات النسخة 4 التاريخ بداية:...

Pour les articles homonymes, voir Crommelin. Andrew CrommelinAndrew CrommelinBiographieNaissance 6 février 1865IrlandeDécès 20 septembre 1939 (à 74 ans)LondresNom de naissance Andrew Claude de la Cherois CrommelinNationalité britanniqueFormation Trinity CollegeMarlborough CollegeActivité AstronomeFamille Famille Crommelin (d)Père Nicolas de la Cherois (d)Mère Annie Mulholland (d)Autres informationsA travaillé pour Observatoire royal de GreenwichDistinctions Prix Jules-Janssen (...

TermodinamikaMesin panas klasik Carnot Cabang Klasik Statistik Kimia Termodinamika kuantum Kesetimbangan / Tak setimbang Hukum Awal Pertama Kedua Ketiga Sistem Keadaan Persamaan keadaan Gas ideal Gas nyata Wujud zat Kesetimbangan Volume kontrol Instrumen Proses Isobarik Isokorik Isotermis Adiabatik Isentropik Isentalpik Quasistatik Politropik Ekspansi bebas Reversibel Ireversibel Endoreversibilitas Siklus Mesin kalor Pompa kalor Efisiensi termal Properti sistemCatatan: Variabel konjugat ...

—— Permukiman di Uni Emirat Arab —— Al Mankhoolالمنخول Negara Uni Emirat Arab Emirat Dubai Kota Dubai Jumlah daerah 317 Statistik permukiman Luas 1 km2 Jumlah penduduk 16,013[1] (2000) Kepadatan penduduk 16,013/km2 Permukiman sekitarnya Umm Hurair, Al Karama, Al Rifa, Al Jafiliya Koordinat 25°14′17″N 55°17′33″E / 25.23806°N 55.29250°E / 25.23806; 55.29250 Al Mankhool (bahasa Arab: المنخول) merupakan sebuah ...

Charles Wilkes Charles WilkesInformación personalNacimiento 1798Nueva YorkFallecimiento 1877, 78 añosWashington D. C.Sepultura Cementerio Nacional de Arlington Nacionalidad EstadounidenseFamiliaPadres John de Ponthieu Wilkes Mary Magdalene Wilkes EducaciónEducado en Universidad de Columbia Información profesionalÁrea oficial de la Marina, explorador, botánico, pteridólogoAños activo desde 1818Cargos ocupados Comandante de Expedición Wilkes (1838-1842) Abreviatura en botánica Wi...

British politician (born 1965) For the English cricketer, see David Warburton (cricketer). David WarburtonFRSAOfficial portrait, 2019Member of Parliamentfor Somerton and FromeIn office7 May 2015 – 19 June 2023Preceded byDavid HeathSucceeded bySarah Dyke Personal detailsBorn (1965-10-28) 28 October 1965 (age 58)Reading, Berkshire, EnglandPolitical partyConservative (suspended 2022)Spouse Harriet Baker-Bates (m. 2002)Children2EducationRoyal College...

仁賢天皇 『御歴代百廿一天皇御尊影』より「仁賢天皇」第24代天皇 在位期間仁賢天皇元年1月5日 - 同11年8月8日時代 伝承の時代(古墳時代)先代 顕宗天皇次代 武烈天皇崩御 広高宮陵所 埴生坂本陵漢風諡号 仁賢天皇諱 大脚大為億計別称 億計天皇大石尊意祁命意富祁王父親 市辺押磐皇子(履中天皇皇子)母親 荑媛皇后 春日大娘皇女(雄略天皇皇女)子女 高橋大娘皇女�...

Este artigo não cita fontes confiáveis. Ajude a inserir referências. Conteúdo não verificável pode ser removido.—Encontre fontes: ABW • CAPES • Google (N • L • A) (Maio de 2020) Augustus Aikhomu (20 de outubro de 1939 – 17 de agosto de 2011)foi um almirante aposentado da Marinha nigeriana, que serviu como vice-presidente de facto da Nigéria, durante a junta militar de Ibrahim Babangida entre 1986-1993. vdeVice–presid...

Laws describing the frequency of oscillation of a stretched string A string half the length (1/2), four times the tension (4), or one-quarter the mass per length (1/4) is an octave higher (2/1). If the tension on a string is ten lbs., it must be increased to 40 lbs. for a pitch an octave higher.[1] A string, tied at A, is kept in tension by W, a suspended weight, and two bridges, B and the movable bridge C, while D is a freely moving wheel; all allowing one to demonstrate Mersenne's l...

Quartz fuméCatégorie IX : silicates[1] Quartz Fumé- Massif du Mont Blanc - Haute Savoie, France (15x10 cm) Général Classe de Strunz 4.DA.05 4 OXIDES (Hydroxides, V[5,6] vanadates, arsenites, antimonites, bismuthites, sulfites, selenites, tellurites, iodates) 4.D Metal:Oxygen = 1:2 and similar 4.DA With small cations 4.DA.05 Quartz SiO2Space Group P 3121,P 3221Point Group 3 2 Formule chimique O2Si SiO2Identification Masse formulaire[2] 60,084...

Questa voce o sezione sull'argomento matematica non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Segui i suggerimenti del progetto di riferimento. Nell'algebra astratta, una branca della matematica, un monoide è una struttura algebrica dotata dell'operazione binaria associativa e di un elemento neutro. I monoidi sono studiati nella teoria dei semigruppi ...

Branch of mathematics studying (smooth) functions of manifolds For the mathematical object, see Geometric topology (object). A Seifert surface bounded by a set of Borromean rings; these surfaces can be used as tools in geometric topology In mathematics, geometric topology is the study of manifolds and maps between them, particularly embeddings of one manifold into another. History Geometric topology as an area distinct from algebraic topology may be said to have originated in the 1935 classif...

Failed uprising in Cuba against the Spanish Empire (1879-80) Little WarCalixto García, the main organizer of the conflictDateAugust 26, 1879 – December 3, 1880(1 year, 3 months and 1 week)LocationCubaResult Spanish victoryBelligerents Cuban Rebels SpainCommanders and leaders Calixto García Gregorio Benítez † Guillermo Moncada (POW) Arcadio Leyte-Vidal † José Maceo (POW) Rafael Maceo (POW) Serafín Sánchez Limbano Sánchez (POW) F...

Route of administration where a substance is taken through the mouth Oral administrationOral administration of a tabletOther namesBy mouth, per os (PO)[edit on Wikidata] A health professional demonstrates how to offer oral medication to a dummy. Oral administration of a liquid Oral administration is a route of administration whereby a substance is taken through the mouth, swallowed, and then processed via the digestive system. This is a common route of administration for many medications....

For the 2001 Doctor Who audio story, see The Stones of Venice (audio drama). Book on Venetian art by John Ruskin The Stones of Venice The Nature of Gothic in a Kelmscott Press edition. First page of text, with ornamented border.AuthorJohn RuskinLanguageEnglishPublication date1851 - 1853Publication placeUnited KingdomPreceded byThe Seven Lamps of Architecture The Stones of Venice is a three-volume treatise on Venetian art and architecture by English art historian John Ruskin, first ...