Read other articles:

Artikel ini bukan mengenai Taiping, Perak. Pulau Taiping Pulau dipersengketakanNama lain: Itu AbaTàipíng Dǎo (太平島/太平岛) (Tionghoa)Ligao Island (Inggris)Pulo ng Ligaw (Filipina)Đảo Ba Bình (Vietnam)Huángshānmǎ Jiāo (黃山馬礁/黄山马礁) (Tionghoa)Huángshānmǎ Zhì (黃山馬峙/黄山马峙) (Tionghoa)Nagashima (長島) (Jepang) Foto Stasiun Antariksa Internasional dari Pulau Taiping (kiri) dan Karang Zhongzhou (kanan) Geografi [[Berkas:Pulau PratasPulau TaipingKa...

2022 Cheltenham Borough Council election ← 2021 5 May 2022 (2022-05-05) 2024 → 21 out of 40 seats to Cheltenham Borough Council21 seats needed for a majority   First party Second party   Party Liberal Democrats Conservative Last election 31 seats, 42.6% 7 seats, 37.5% Seats won 18 1 Seats after 31 6 Seat change 1 Popular vote 19,595 9,867 Percentage 55.1% 27.7% Swing 12.5% 9.8%   Third party Fourth party   Part...

University in Kenya Great Lakes University of KisumuGlukTypePrivate UniversityEstablished1998Vice-ChancellorProf Hazel Miseda MumboUndergraduatesOfferedPostgraduatesOfferedDoctoral studentsOfferedAddressGreat Lakes University of Kisumu P.O.BOX 2224 - 40100, Kisumu, KENYA, Kisumu, KenyaCampusMain Campus (Kibos)Millimani Campus (Kisumu Town)Nairobi Campus. (Centro House - Westlands)ColorsGreen, blue and yellow    NicknameGLUKWebsitewww.gluk.ac.ke Great Lakes University of Kisumu ...

Christmas tradition 2005 Calgary Hitmen teddy bear toss The teddy bear toss is a popular Christmas season promotion most common at junior ice hockey and minor league hockey games. Fans are encouraged to bring teddy bears or other stuffed toys to the game, and to throw them onto the ice when the home team scores its first goal. The toys are gathered to be donated as presents to hospitals and charities. In many cases, the players themselves personally donate some of the bears to children at are...

Exact statistical hypothesis test A permutation test (also called re-randomization test or shuffle test) is an exact statistical hypothesis test making use of the proof by contradiction. A permutation test involves two or more samples. The null hypothesis is that all samples come from the same distribution H 0 : F = G {\displaystyle H_{0}:F=G} . Under the null hypothesis, the distribution of the test statistic is obtained by calculating all possible values of the test statistic under possible...

Search and Rescue Training UnitUnit badgeActive1979 – 2016DisbandedMay 2016Country United KingdomBranch Royal Air ForceTypeTraining unitRoleHelicopter search and rescue trainingPart of RAF Strike Command Defence Helicopter Flying School Home stationRAF ValleyMotto(s)That lives may be savedAircraft Whirlwind HAR.10 Wessex HAR.2 Griffin HT1 AW139 Military unitThe Search and Rescue Training Unit (SARTU) of the Royal Air Force was located on the southern side of RAF Valley on th...

  提示：此条目页的主题不是中華人民共和國最高領導人。 中华人民共和国 中华人民共和国政府与政治系列条目 执政党 中国共产党 党章、党旗党徽 主要负责人、领导核心 领导集体、民主集中制 意识形态、组织 以习近平同志为核心的党中央 两个维护、两个确立 全国代表大会 （二十大） 中央委员会 （二十届） 总书记：习近平 中央政治局 常务委员会 中央书记处 �...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言，清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日（哈薩克蘇維埃社會主義共和國總統） 哈萨克斯坦 哈萨克斯坦政府...

Religious building in Marrakesh, Morocco Zawiya of Sidi Abu al-Abbas al-Sabtiزاوية سيدي بلعباس ⵣⴰⵡⵉⵢⴰ ⵙⵉⴷⵉ ⴱⵍⵄⴻⴱⴰⵙOne of the main courtyards of the complex, with the mausoleum on the right and the minaret on the leftReligionAffiliationIslamLocationLocationMarrakesh, MoroccoGeographic coordinates31°38′20.1″N 7°59′27.9″W / 31.638917°N 7.991083°W / 31.638917; -7.991083ArchitectureTypezawiyaStyleSaadian, Moroccan...

Leang PadaeloGua Padaelo', Leang Padaelo', Gua Padaelok, Leang Padaelok, Gua PadaeloLokasiKampung Pangia, Dusun Pattunuang, Desa Samangki, Kecamatan Simbang, Kabupaten Maros, Sulawesi Selatan, IndonesiaKedalaman54 mGeologikarst / batu kapur / batu gampingFiturlebar mulut gua antara 5–10 meter dan jenis vertikalSitus webvisit.maroskab.go.idcagarbudaya.kemdikbud.go.id Leang Padaelo atau Gua Padaelo (Inggris: Padaelo Cave ) adalah sebuah gua di Kawasan Karst Maros-Pangkep, Taman Nasional ...

Walt Disney World's Magic Kingdom in Bay Lake, Florida is the most visited theme park in the world. In 2016, Orlando, Florida was the most visited destination in the United States, and continues to be one of the most visited destinations in the world. The Grand Canyon of Arizona attracts approximately 4.41 million visitors annually. This article is part of a series on theEconomy of theUnited States Economic history Agricultural history Banking history Petroleum history Shipbuilding Indu...

Alfred BoisseauAlfred Boisseau self-portrait 1842BornFebruary 1823Paris, FranceDied7 October 1901Buffalo, New York, U.S.Resting placeBuffalo, New York, U.S.Known forPainter Alfred Boisseau (1823–1901) was an American/Canadian artist who was born in Paris, France. He was known as a painter and photographer, who specialized in paintings of North American Natives and the West. As a young man in his 20s, Boisseau immigrated to the United States, settling first in New Orleans, where his bro...

Infinite sequence of differential equations In mathematics, the KdV hierarchy is an infinite sequence of partial differential equations which contains the Korteweg–de Vries equation. Details Let T {\displaystyle T} be translation operator defined on real valued functions as T ( g ) ( x ) = g ( x + 1 ) {\displaystyle T(g)(x)=g(x+1)} . Let C {\displaystyle {\mathcal {C}}} be set of all analytic functions that satisfy T ( g ) ( x ) = g ( x ) {\displaystyle T(g)(x)=g(x)} , i.e. periodic functio...

Stack-oriented programming language This article relies excessively on references to primary sources. Please improve this article by adding secondary or tertiary sources. Find sources: Factor programming language – news · newspapers · books · scholar · JSTOR (July 2019) (Learn how and when to remove this message) FactorParadigmmulti-paradigm: concatenative (stack-based), functional, object-orientedDeveloperSlava PestovFirst appeared2003Stable...

  لمعانٍ أخرى، طالع دسمان (توضيح). يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (مارس 2016) دسمان أحياء مدينة الكويت قديماً. المحافظة: العاصمة الدائرة الانتخاب�...

English-language newspaper in the capital of Ukraine Kyiv PostType of siteNewsAvailable inEnglishHeadquartersKyiv, UkraineOwnerAdnan Kivan, operating as Businessgroup LLCEditorBohdan Nahaylo, Chief EditorCEOLuc ChénierURLwww.kyivpost.com CommercialYesRegistrationNot requiredLaunchedOctober 18, 1995; 28 years ago (1995-10-18)[1][2]Current statusNews mediaISSN1563-6429 This article's lead section may be too short to adequately summarize the key point...

Cet article est une ébauche concernant une localité hongroise. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Pour les articles homonymes, voir Doc. Dóc Blason de Dóc Dóc Dóc Administration Pays Hongrie Comitat (megye) Csongrád-Csanád (Grande Plaine méridionale) District (járás) Szeged Rang Commune Bourgmestre(polgármester) Mandat Tóth Margit (indépendant) (2014-2019) Code postal 6766 Indicatif t�...

Part of a series onArtificial intelligence Major goals Artificial general intelligence Intelligent agent Recursive self-improvement Planning Computer vision General game playing Knowledge reasoning Natural language processing Robotics AI safety Approaches Machine learning Symbolic Deep learning Bayesian networks Evolutionary algorithms Hybrid intelligent systems Systems integration Applications Bioinformatics Deepfake Earth sciences Finance Generative AI Art Audio Music Government Healthcare...

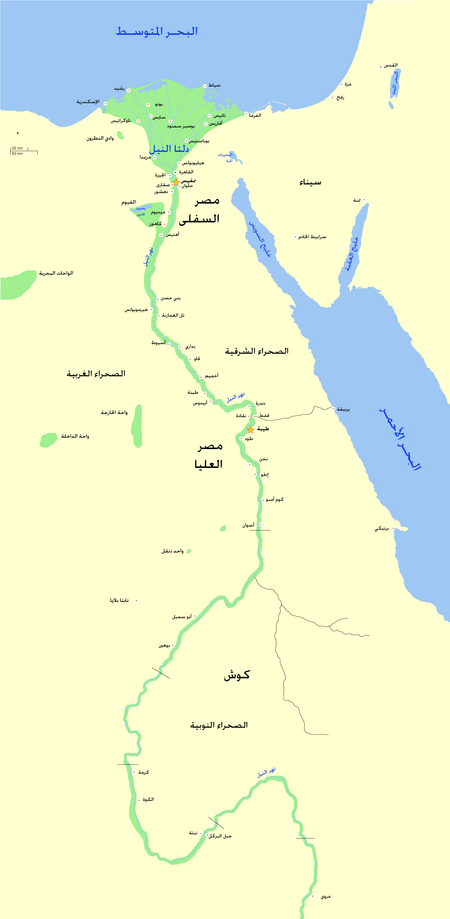
مصر العليا مملكة مصر العليا غير معروف – 3150 ق.م صعيد مصرالتاج الأبيض لمصر العليا مقاطعات مصر العليا القديمة عاصمة ثينيس نظام الحكم ملكية مطلقة اللغة الرسمية اللغة المصرية الديانة ديانة قدماء المصريين ملك غير معروف (الأول) غير معروف نارمر (الأخير) 3150 ق.م التاريخ التأسي�...

† Родригесский ожереловый попугай Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлад�...