Galería de imaxes de eleccións de Galicia
|
Read other articles:

Hasan KleibKleib pada 2021 Utusan Tetap Indonesia untuk Perserikatan Bangsa-Bangsa di Jenewa ke-15Masa jabatan17 November 2017 – 2020PresidenJoko Widodo PendahuluTriyono WibowoPenggantiFebrian Alphyanto RuddyardUtusan Tetap Indonesia untuk Perserikatan Bangsa-Bangsa ke-17Masa jabatan10 Agustus 2010 – 2011PresidenSusilo Bambang Yudhoyono PendahuluMarty NatalegawaPenggantiDesra Percaya Informasi pribadiLahir1 Oktober 1960 (umur 63)[butuh rujukan]Cirebo...

GrímsvötnEyjafjallajökullHeklaHengillKrýsuvíkKatlaLakiÖræfajökullSurtseyAskjaEldfellKraflaLangjökullHofsjökullSnæfellsjökullLjósufjöllÞeistareykjabungaBárðarbunga Peta koordinat semua menggunakan: OpenStreetMap Unduh koordinat sebagai: KML Berikut adalah daftar gunung berapi di Islandia. Di dalam daftar ini terdapat 130 gunung berapi aktif dan mati, dan 18 di antaranya pernah meletus semenjak berdirinya permukiman pertama di Islandia sekitar tahun 900 M. Daftar Nama Ket...

2007 Japanese anime This article is about the anime. For the original game, see MapleStory. This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: MapleStory TV series – news · newspapers · books · scholar · JSTOR (November 2015) (Learn how and when to remove this template message)MapleStoryCover of the Japane...

Berikut daftar episode serial Ben 10 seri 2016, kelanjutan dari sekuel keempat Ben 10: Omniverse. Pemain Tara Strong - Ben Tennyson Montserrat Hernandez - Gwen Tennyson David Kaye - Grandpa Max alias Max Tennyson Greg Cipes - Kevin 11 alias Kevin Levin Dwight Schultz - Dr Animo Yuri Lowenthal - Vilgax Daryl Sabara - Heatblast Season 1 The Filth Waterfilter The Ring Leader Riding the Storm Out The Clocktopus Take 10 Growing Pains Shhh! Brief Career of Lucky Girl Animo Farm Clown College Adven...

العلاقات البوروندية الكمبودية بوروندي كمبوديا بوروندي كمبوديا تعديل مصدري - تعديل العلاقات البوروندية الكمبودية هي العلاقات الثنائية التي تجمع بين بوروندي وكمبوديا.[1][2][3][4][5] مقارنة بين البلدين هذه مقارنة عامة ومرجعية للدولتين: وجه ...

6th-century sermon by Gildas De Excidio et Conquestu Britanniae (Latin: On the Ruin and Conquest of Britain, sometimes just On the Ruin of Britain) is a work written in Latin by the probably 6th-century AD British cleric St Gildas. It is a sermon in three parts condemning the acts of Gildas' contemporaries, both secular and religious, whom he blames for the dire state of affairs in sub-Roman Britain. It is one of the most important sources for the history of Britain in the 5th and 6th ce...

Sculpture in Los Angeles, California, U.S. Night SailThe sculpture in 2022ArtistLouise NevelsonLocationLos Angeles, California, U.S.Coordinates34°3′9″N 118°15′9.5″W / 34.05250°N 118.252639°W / 34.05250; -118.252639 Night Sail is a 1985 sculpture by Louise Nevelson, installed in Los Angeles, California, United States.[1][2][3] The artwork weighs 33 tons,[4] and has been described by the Los Angeles Times as a mysterious, Cubis...

Bupati SikkaPetahanaAdrianus Firminussejak 20 September 2024Masa jabatan5 tahunDibentuk1958Pejabat pertamaD. P. C. Ximenes da SilvaSitus webwww.sikkakab.go.id/welcome Berikut adalah Daftar Bupati Sikka dari masa ke masa. No. Potret Nama (masa hidup) Mulai Menjabat Selesai Menjabat Prd. Jabatan Sebelumnya Wakil Bupati Ket. sebelum dilakukan pemilihan bupati definitif, Don Paulus Centis Ximenes da Silva yang sebelumnya merupakan Kepala Dewan Pemerintahan Daerah Swatantra Sikka ditunjuk seb...
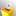
Beberapa pemimpin yang sering kali disebut memimpin suatu rezim totaliter, dari kiri ke kanan, atas ke bawah, meliputi Joseph Stalin, mantan Sekretaris Jenderal Partai Komunis Uni Soviet; Adolf Hitler, mantan Führer Jerman; Mao Zedong, mantan Ketua Partai Komunis Tiongkok; Benito Mussolini, mantan Duce Italia; dan Kim Il-sung, Pemimpin Abadi Korea Utara. Bagian dari seri PolitikBentuk dasar dari pemerintahan Struktur kekuatan Konfederasi Federasi Hegemoni Kerajaan Negara kesatuan Sumber keku...

Chemical compound 2C-CPIdentifiers IUPAC name 2-(4-cyclopropyl-2,5-dimethoxyphenyl)ethanamine CAS Number2888537-46-8PubChem CID163192739Chemical and physical dataFormulaC13H19NO2Molar mass221.300 g·mol−13D model (JSmol)Interactive image SMILES COc1cc(c(cc1CCN)OC)C1CC1 InChI InChI=1S/C13H19NO2/c1-15-12-8-11(9-3-4-9)13(16-2)7-10(12)5-6-14/h7-9H,3-6,14H2,1-2H3Key:WSMVNFNZKKZCPU-UHFFFAOYSA-N 2C-CP (2C-cP) is a recreational designer drug from the substituted phenethylamine family, with psy...

Pour les articles homonymes, voir Corps expéditionnaire. Corps expéditionnaire français en Extrême-Orient Insigne du CEFEO. Création 1945 Dissolution 28 avril 1956 Pays Union française Guerres Guerre d'Indochine Commandant historique Général LeclercGénéral de LattreGénéral Salan modifier Insigne d'épaule du CEFEO. Combat au FM 24/29 en 1952. Parachutistes français en Indochine en mai 1952. Hommes de la 1re compagnie étrangère parachutiste de mortiers lourds en 1953...

Численность населения республики по данным Росстата составляет 4 003 016[1] чел. (2024). Татарстан занимает 8-е место по численности населения среди субъектов Российской Федерации[2]. Плотность населения — 59,00 чел./км² (2024). Городское население — 76,72[3] % (20...

مارسيلو بيريرا معلومات شخصية الميلاد 27 مايو 1995 (العمر 28 سنة)تيغوسيغالبا الطول 1.84 م (6 قدم 1⁄2 بوصة) مركز اللعب مدافع الجنسية هندوراس معلومات النادي النادي الحالي نادي كرة القدم موتاجوا [لغات أخرى] الرقم 5 مسيرة الشباب سنوات فريق ديبورتيفو أوليم�...

Political term in Imperial Japan Mainland JapanNative name: 内地Passports for passengers between Mainland Japan and Okinawa during 1952–1972.GeographyLocationJapanDemographicsEthnic groupsJapanese peopleAinu peopleRyukyuan people Mainland Japan (内地, naichi, lit. inner lands) is a term used to distinguish Japan's core land area from its outlying territories. It is most commonly used to distinguish the country's four largest islands (Hokkaidō, Honshū, Kyūshū and Shikoku) from smalle...

Olivetan redirects here. For the French translator of the Bible, see Pierre Robert Olivétan. Catholic monastic order Depiction of an Olivetan monk, 16th century The Olivetans, formally known as the Order of Our Lady of Mount Olivet, are a monastic order. They were founded in 1313 and recognised in 1344. They use the Rule of Saint Benedict and are a member of the Benedictine Confederation, where they are also known as the Olivetan Congregation, but are distinguished from the Benedictines in t...

Bài viết này cần thêm chú thích nguồn gốc để kiểm chứng thông tin. Mời bạn giúp hoàn thiện bài viết này bằng cách bổ sung chú thích tới các nguồn đáng tin cậy. Các nội dung không có nguồn có thể bị nghi ngờ và xóa bỏ. Hình họcHình chiếu một mặt cầu lên mặt phẳng. Đại cươngLịch sử Phân nhánh Euclid Phi Euclid Elliptic Cầu Hyperbol Hình học phi Archimedes Chiếu Afin Tổng hợp Giải tích Đ...

Episodes in the life of Buddha Jagdishpur relief, photographed in 1872. A very rare survival of a large scale depiction of the Eight Great Events.[1] Over 3 metres high, perhaps late 10th-century. The Eight Great Events (ashtammaha-pratharya)[2] are a set of episodes in the life of Gautama Buddha that by the time of the Pala Empire of North India around the 9th century had become established as the standard group of narrative scenes to encapsulate the Buddha's life and teachin...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (مارس 2016) واقعمعلومات عامةيدرسه علم الوجودفلسفة ممثلة بـ وجودactuality (en) النقيض subjective reality (en) تعديل - تعديل مصدري - تعديل...

Bulus Bulus (Amyda cartilaginea) dari Mentaya Hulu, Kotawaringin Timur Status konservasi Rentan (IUCN 2.3) Klasifikasi ilmiah Kerajaan: Animalia Filum: Chordata Kelas: Reptilia Ordo: Testudines Famili: Trionychidae Genus: Amyda Spesies: A. cartilaginea Nama binomial Amyda cartilagineaBoddaert, 1770 Bulus (Amyda cartilaginea) adalah sejenis labi-labi (kura-kura bertempurung lunak) anggota suku Trionychidae. Disebut berpunggung lunak karena sebagian perisainya terdiri dari tulang ra...

Bounds the order of the group of automorphisms of a compact Riemann surface of genus g > 1 In mathematics, Hurwitz's automorphisms theorem bounds the order of the group of automorphisms, via orientation-preserving conformal mappings, of a compact Riemann surface of genus g > 1, stating that the number of such automorphisms cannot exceed 84(g − 1). A group for which the maximum is achieved is called a Hurwitz group, and the corresponding Riemann surface a Hurwitz su...





















