Théorème de réarrangement de Riemann
|
Read other articles:

UraydhPermukimanUraydhLocation in the Kingdom of Saudi ArabiaKoordinat: 24°38′N 46°43′E / 24.633°N 46.717°E / 24.633; 46.717Koordinat: 24°38′N 46°43′E / 24.633°N 46.717°E / 24.633; 46.717Negara Arab SaudiPemerintahan • Gubernur Pangeran RiyadhFaisal bin Bandar Al Saud • Wali kotaIbraheem Mohammed Al-SultanKetinggian612 m (2,008 ft)Zona waktuUTC+3 (AST) • Musim panas (DST)UTC+3 (A...

Stasiun Pasar Minggu B14 Peron Stasiun Pasar MingguLokasiJalan Raya Pasar MingguPejaten Timur, Pasar Minggu, Jakarta Selatan, 12520IndonesiaKoordinat6°16′52″S 106°50′26″E / 6.28111°S 106.84056°E / -6.28111; 106.84056Koordinat: 6°16′52″S 106°50′26″E / 6.28111°S 106.84056°E / -6.28111; 106.84056Ketinggian+36 mOperator KAI Commuter Letakkm 18+480 lintas Jakarta-Manggarai-Bogor/Nambo[1] Jumlah peronSatu peron sisi dan...

Val TaleggioPosizione della Val TaleggioStati Italia Regioni Lombardia Province Bergamo Lecco Località principaliTaleggio, Vedeseta, Morterone Comunità montanaComunità montana della Valle Brembana Nome abitantivaltaleggini Sito web Modifica dati su Wikidata · ManualeCoordinate: 45°52′59.88″N 9°36′00″E / 45.8833°N 9.6°E45.8833; 9.6 La Val Taleggio (Al Taècc in bergamasco) è una valle della Lombardia. Particolarmente conosciuta per la p...

Species of mammal Mountain cottontail Conservation status Least Concern (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Mammalia Order: Lagomorpha Family: Leporidae Genus: Sylvilagus Species: S. nuttallii Binomial name Sylvilagus nuttallii(Bachman, 1837) Mountain cottontail range Mountain cottontail in snow. The mountain cottontail or Nuttall's cottontail (Sylvilagus nuttallii) is a species of mammal in the family Leporida...

This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: David Kazhdan – news · newspapers · books · scholar · JSTOR (February 2013) (Learn how and when to remove this message) Soviel-Israeli mathematici...

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

2004 single by Mario Let Me Love YouSingle by Mariofrom the album Turning Point B-sideWhizReleasedOctober 4, 2004 (2004-10-04)[1]StudioSoundvilla (Miami Beach, Florida)GenreR&BLength4:09LabelJSongwriter(s)Scott StorchKameron HouffShaffer SmithProducer(s)Scott StorchMario singles chronology C'mon (2003) Let Me Love You (2004) How Could You (2005) Music videoLet Me Love You on YouTube Let Me Love You is a song by American singer Mario, released as a single on October ...

Protein-coding gene in the species Homo sapiens SPTAN1Available structuresPDBOrtholog search: PDBe RCSB List of PDB id codes2FOT, 3F31, 3FB2IdentifiersAliasesSPTAN1, EIEE5, NEAS, SPTA2, spectrin alpha, non-erythrocytic 1, DEE5External IDsOMIM: 182810 MGI: 98386 HomoloGene: 2353 GeneCards: SPTAN1 Gene location (Human)Chr.Chromosome 9 (human)[1]Band9q34.11Start128,552,558 bp[1]End128,633,662 bp[1]Gene location (Mouse)Chr.Chromosome 2 (mouse)[2]Band2 B|2 20.9...

Sports events held in the Dominican Republic Central American and Caribbean Sports GamesHost citySanto DomingoCountry Dominican RepublicEdition12thNations23Athletes1,928Opening27 February 1974 (1974-02-27)Closing13 March 1974 (1974-03-13)Opened byJoaquín Balaguer[1]Athlete's OathPorfirio Veras[1]Torch lighterAlberto Torres[1]Main venueEstadio Olímpico Juan Pablo Duarte[1]← 1970 Panama City1978 Medellín ͛...

LGBT rights in GuyanaGuyanaStatusIllegalPenaltyUp to life in prison (not enforced; decriminalisation proposed)Gender identityNoMilitaryNoDiscrimination protectionsNoneFamily rightsRecognition of relationshipsNo recognition of same-sex relationshipsAdoptionUnclear Lesbian, gay, bisexual, and transgender (LGBT) people in Guyana face legal and societal challenges not experienced by non-LGBT residents. Guyana is the only country in South America, and the only mainland country in the Americas, wh...

This article needs to be updated. Please help update this article to reflect recent events or newly available information. (July 2020) The NationalsCurrent season, competition or edition: 2022 The NationalsSportEsportsFounded2018First season2019PresidentKevin Chung[1]CommissionerRen Vitug[1]No. of teams6CountryPhilippinesTV partner(s)eGG Network5 PlusLevel on pyramid1Relegation toNoneRelatedcompetitionsChallenger SeriesAcademy SeriesOfficial websiteOfficial Website, archived f...

Road in France You can help expand this article with text translated from the corresponding article in French. (May 2023) Click [show] for important translation instructions. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than simply copy-pasting machine-translated text into the English Wikipedia. Do not translate text that appears unreli...

Marie-Jacques PerrierLahirMarie-Jacques Renée Perrier(1924-11-22)22 November 1924Paris, PrancisMeninggal29 November 2012 (usia 88)Paris, PrancisKebangsaanPrancisPendidikanÉcole du LouvrePekerjaanPenyanyi, Jurnalis mode, aktris suara, Penulis, Kolektor seniTahun aktif1934–2012Anak1 anak perempuan Marie-Jacques Perrier (22 November 1924 – 29 November 2012) merupakan seorang penyanyi, jurnalis fashion, voice actress, sosialita, penulis buku dan kolektor seni.[1]...
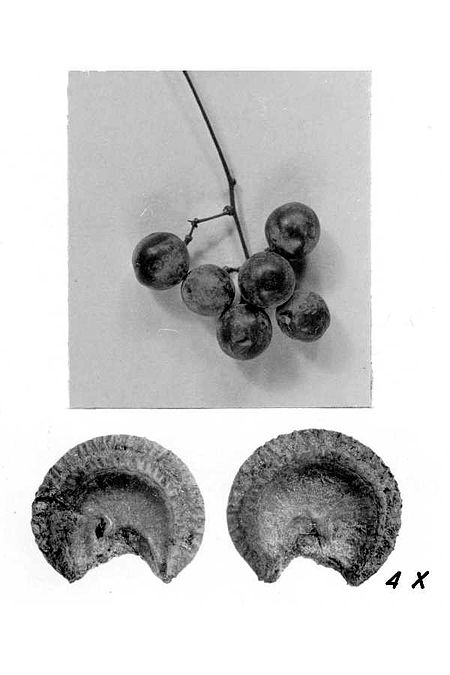
Genus of flowering plants Menispermum Fruit and seed of Menispermum canadense Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Order: Ranunculales Family: Menispermaceae Subfamily: Menispermoideae Genus: MenispermumL. Species See text Menispermum (moonseed) is a small genus of deciduous climbing woody vines in the moonseed family (Menispermaceae). Plants in this genus have small dioecious flowers, and clusters of small grape-like drupes.[1...

حفلة كوكتيل المؤلف ت. س. إليوت أول عرض 21 يناير 1950 لغة العمل الإنجليزية IBDB 2651 تعديل مصدري - تعديل حفلة كوكتيل (بالإنجليزية: The Cocktail Party) مسرحية للكاتب البريطاني ت. س. إليوت.[1][2][3] تستمد مادتها الأساسية من مسرحية أليسيستس للمسرحي الإغريقي القدي�...

世界遺產標誌由米歇爾·奧里夫(法语:Michel Olyff)設計,於1978年第二屆世界遺產委員會通過[1]:141。圖案代表人類文化(內側方形)與地球自然(外側圓形)密不可分[2]:36。圓形外側環繞的文字為「世界遺產」的英文、法文,與西班牙文。依據聯合國教科文組織規定,此圖像的使用,外圈文字可選擇出現或不出現[3] 世界遺產(英語:World Heritage;法語:Pat...

Nicolás MaduroMaduro pada tahun 2013 Presiden VenezuelaPetahanaMulai menjabat 19 April 2013Penjabat: 5 Maret 2013 – 19 April 2013Wakil PresidenJorge Arreaza (2013–16) Aristóbulo Istúriz (sejak 2016)PendahuluHugo ChávezPenggantiPetahanaSeekretaris Jenderal Gerakan Non-BlokMasa jabatan17 September 2016 – 2019PendahuluHassan RouhaniPenggantiilham aliyevPresiden pro tempore Uni Negara Amerika SelatanPetahanaMulai menjabat 23 April 2016PendahuluTabaré VázquezPenggantiP...

كاتدرائية ألكسندر نيفيسكيХрам-паметник Свети Александър Невски (بالبلغارية) التسميةنسبة الاسم إلى ألكسندر نيفيسكي معلومات عامةنوع المبنى كاتدرائية متحف مزار سياحي المكان صوفيا المنطقة الإدارية Oborishte District (en) — صوفيا البلد بلغاريا الإهداء ألكسندر نيفيسكي الديانة الك...

Number with a real and an imaginary part This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Complex number – news · newspapers · books · scholar · JSTOR (July 2022) (Learn how and when to remove this message) A complex number can be visually represented as a pair of numbers (a, b) forming a vector on a diagra...

Pour les articles homonymes, voir club. Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (novembre 2013). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références ». En pratique ...












