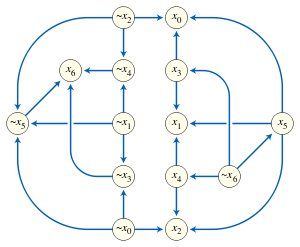
Problème 2-SAT
|
Read other articles:

2016 American filmThe PhenomTheatrical release posterDirected byNoah BuschelWritten byNoah BuschelProduced byJeff RiceJeff ElliottStarringJohnny SimmonsYul VazquezSophie Kennedy ClarkPaul GiamattiEthan HawkeCinematographyRyan SamulMusic byAleks de CarvalhoProductioncompaniesBest PitcherBron Capital PartnersCrystal WealthDistributed byRLJ EntertainmentRelease dates April 17, 2016 (2016-04-17) (Tribeca Film Festival) June 24, 2016 (2016-06-24) (United State...

Kegubernuran Amman محافظة العاصمةKegubernuranNegaraYordaniaIbu kotaAmmanSubdivisi Daftar Distrik PusatDistrik MarkaDistrik Al-QwesmehDistrik GabunganDistrik Wadi Al SeerDistrik NaourDistrik SahabDistrik Al JizahDistrik Muwaqqar Pemerintahan • GubernurSaed ShihabLuas • Total7.579 km2 (2,926 sq mi)Populasi (2015) • Total4.007.000 • Kepadatan530/km2 (1,400/sq mi)Zona waktuGMT +2 • Musim panas (DST)+...

Stephano (satelit) adalah satelit alami dari planet Uranus. Uranus, adalah sebuah planet ketujuh dari Tata Surya, memiliki 27 bulan yang diketahui, yang semuanya dinamai karakter dari karya-karya William Shakespeare dan Alexander Pope. Referensi http://solarsystem.nasa.gov/planets/profile.cfm?Object=Uranus&Display=Moons Diarsipkan 2014-07-28 di Wayback Machine.

Languages of the Democratic Republic of the CongoMap showing the distribution of the four national languages in the CongoOfficialFrenchNationalKituba, Lingala, Swahili and TshilubaIndigenousMore than 200SignedAmerican Sign Language (Francophone African Sign Language)Keyboard layoutFrench AZERTYLingua francaFrench, Kikongo ya leta, Lingala, Swahili and Tshiluba Part of a series on theCulture of the Democratic Republic of the Congo History People Languages Cuisine Religion Music Media Rad...

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、�...

1986 film by Hal Ashby 8 Million Ways to DieTheatrical release posterDirected byHal AshbyWritten byOliver StoneR. Lance HillRobert Towne (uncredited)Based on8 Million Ways To Dieby Lawrence BlockProduced byStephen J. RothMark DamonCharles MulvehillJohn W. HydeStarring Jeff Bridges Rosanna Arquette Alexandra Paul Andy Garcia CinematographyStephen H. BurumEdited byRobert LawrenceStuart H. PappéMusic byJames Newton HowardProductioncompanyProducers Sales OrganizationDistributed byPSO Internation...

Административная карта Сент-Люсии В административном отношении Сент-Люсия подразделяется на 11 приходов (англ. quarters). В каждом из них имеются органы местного самоуправления — городские и деревенские советы и управления. Общие сведения № Приход Адм. центр Площадь,км...

هنودمعلومات عامةنسبة التسمية الهند التعداد الكليالتعداد قرابة 1.21 مليار[1][2]تعداد الهند عام 2011ق. 1.32 مليار[3]تقديرات عام 2017ق. 30.8 مليون[4]مناطق الوجود المميزةبلد الأصل الهند البلد الهند الهند نيبال 4,000,000[5] الولايات المتحدة 3,982,398[6] الإمار...

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府(Oguzkhan Presidential Palace)機關所在地阿什哈巴德任命者直接选举任期7年,可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關(英语:National Council of Turkmenistan) ...

此條目可能包含不适用或被曲解的引用资料,部分内容的准确性无法被证實。 (2023年1月5日)请协助校核其中的错误以改善这篇条目。详情请参见条目的讨论页。 各国相关 主題列表 索引 国内生产总值 石油储量 国防预算 武装部队(军事) 官方语言 人口統計 人口密度 生育率 出生率 死亡率 自杀率 谋杀率 失业率 储蓄率 识字率 出口额 进口额 煤产量 发电量 监禁率 死刑 国债 ...

This article is about the Scottish village Killearn. For Barons called Killearn, see Baron Killearn and Miles Lampson, 1st Baron Killearn. Human settlement in ScotlandKillearnScottish Gaelic: Cill Fhearann, orig. Ceann FhearannScots: Killern, orig. KynhernMain street in KillearnKillearnLocation within the Stirling council areaPopulation1,910 (mid-2020 est.)[1]OS grid referenceNS522860• Edinburgh50 mi (80 km)• London400 mi (650 km)C...

Artikel ini membutuhkan rujukan tambahan agar kualitasnya dapat dipastikan. Mohon bantu kami mengembangkan artikel ini dengan cara menambahkan rujukan ke sumber tepercaya. Pernyataan tak bersumber bisa saja dipertentangkan dan dihapus.Cari sumber: Universitas Purdue – berita · surat kabar · buku · cendekiawan · JSTOR Universitas PurdueJenisPublik Unggulan Tanah-hibah Universitas laut-hibah Langit-hibahDidirikan6 Mei 1869Dana abadiAS$1,91 miliar (2012) ...

Sixth director of the FBI; American attorney For other people with similar names, see Robert Muller (disambiguation). Robert MuellerOfficial portrait, 2011Special Counsel for the United States Department of JusticeIn officeMay 17, 2017 – May 29, 2019Appointed byRod RosensteinPreceded byOffice establishedSucceeded byOffice abolished6th Director of the Federal Bureau of InvestigationIn officeSeptember 4, 2001 – September 4, 2013PresidentGeorge W. BushBarack ObamaDeputyThom...

American politician For the Australian cartoonist, see Norm Rice (cartoonist). This biography of a living person needs additional citations for verification. Please help by adding reliable sources. Contentious material about living persons that is unsourced or poorly sourced must be removed immediately from the article and its talk page, especially if potentially libelous.Find sources: Norm Rice – news · newspapers · books · scholar · JSTOR (January 20...
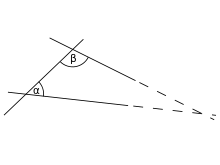
Mathematical model of the physical space Plane geometry redirects here. For other uses, see Plane geometry (disambiguation). Detail from Raphael's The School of Athens featuring a Greek mathematician – perhaps representing Euclid or Archimedes – using a compass to draw a geometric construction. GeometryProjecting a sphere to a plane OutlineHistory (Timeline) Branches Euclidean Non-Euclidean Elliptic Spherical Hyperbolic Non-Archimedean geometry Projective Affine Synthetic Analyt...

2015 film directed by Josh Trank Fantastic FourTheatrical release posterDirected byJosh TrankScreenplay by Jeremy Slater Simon Kinberg Josh Trank Based onFantastic Fourby Stan LeeJack KirbyProduced by Matthew Vaughn Simon Kinberg Gregory Goodman Hutch Parker Robert Kulzer Starring Miles Teller Michael B. Jordan Kate Mara Jamie Bell Toby Kebbell Reg E. Cathey Tim Blake Nelson CinematographyMatthew JensenEdited by Elliot Greenberg Stephen Rivkin Music by Marco Beltrami Philip Glass Productionco...

Korban-korban utama pada Pembantaian Kucheng Pembantaian Kucheng (Hanzi: 古田教案; Pinyin: Gǔtián Jiào'àn; Foochow yang diromanisasikan: Kŭ-chèng Gáu-áng) adalah sebuah pembantaian orang Kristen Barat yang dilakukan di Gutian, Fujian, China pada 1 Agustus 1895. Pada hari itu, sekelompok Buddhis, Zhaijiao menyerang para misionaris Britania yang berlibur musim panas di Gutian Huashan, membunuh sebelas orang dan menghancurkan dua rumah. Pembantaian Kucheng dianggap sebagai salah ...

American politician (1868–1943) Bertha Knight LandesBertha Knight Landes c. 192634th Mayor of SeattleIn officeJune 7, 1926 – June 4, 1928Preceded byEdwin J. BrownSucceeded byFrank E. Edwards Personal detailsBornBertha Ethel Knight(1868-10-19)October 19, 1868Ware, MassachusettsDiedNovember 29, 1943(1943-11-29) (aged 75)Ann Arbor, MichiganResting placeEvergreen Washelli Memorial ParkNationalityAmericanPolitical partyRepublicanSpouseHenry M. LandesAlma materIndiana University B...

Robert EllisEllis dalam The Sphinx, 1933LahirRobert Ellis du Reel(1892-06-27)27 Juni 1892Brooklyn, New York, Amerika SerikatMeninggal29 Desember 1974(1974-12-29) (umur 82)Santa Monica, California, Amerika SerikatPekerjaanPemeranPenulis naskahSutradaraTahun aktif1913–1950Suami/istriMay Allison (1920–1923)Helen Logan Robert Ellis (27 Juni 1892 – 29 Desember 1974) adalah seorang pemeran, penulis naskah dan sutradara Amerika Serikat. Ia tampil dalam 166 film antara ...

Questa voce sull'argomento calciatori kenioti è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Francis KahataNazionalità Kenya Altezza178 cm Peso67 kg Calcio RuoloCentrocampista Squadra Kenya Police CarrieraGiovanili 2005-2009 Thika Utd Squadre di club1 2006-2010 Thika Utd? (?)2010-2011→ University of Pretoria? (?)2011-2014 Thika Utd? (?)2014→ Tirana12 (3)2014-2...