Inégalité de Remez
|
Read other articles:
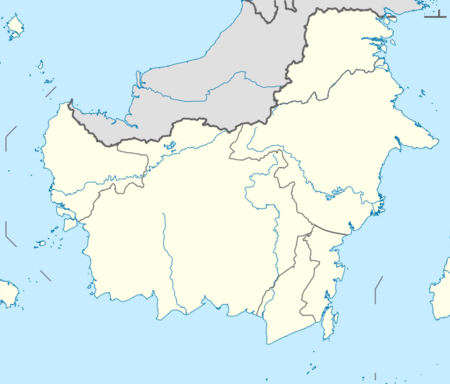
Kerajaan Pulau Laut Kerajaan Kepangeranan Pulau Laut dan Pulau Sebuku dengan dependensinya, setelah bergabung dengan Hindia Belanda disebut Landschap Pulau Laut dan Pulau Sebuku adalah Landschap atau suatu wilayah pemerintahan swapraja yang dikepalai seorang bumiputera bagian dari Afdeeling Pasir en de Tanah Boemboe dalam pemerintahan kolonial Hindia Belanda di bawah kekuasaan Asisten Residen GH Dahmen yang berkedudukan di Samarinda. Wilayah tersebut disebut kerajaan pulau laut yang didirikan...

Evangelicalism in Hungary Baptist Church of HungaryMagyarországi Baptista EgyházClassificationEvangelical ChristianityTheologyBaptistAssociationsBaptist World AllianceHeadquartersBudapest, HungaryOrigin1920Congregations290Members11,208Official websitebaptist.hu The Baptist Church of Hungary (Hungarian: Magyarországi Baptista Egyház) is a Baptist Christian denomination in Hungary. It is affiliated with the Baptist World Alliance. The headquarters is in Budapest. History Pécs Baptist Churc...

Pusat kota Zhuhai Zhuhai (Hanzi: 珠海) merupakan sebuah kota setingkat kabupaten yang terletak di daerah pantai provinsi Guangdong, RRT. Terletak di delta Sungai Mutiara, Zhuhai berbatasan dengan Jiangmen di barat laut, Zhongshan di utara, dan Makau di selatan. Kota ini memiliki luas wilayah 1.653 km². Dengan memiliki jumlah penduduk sebanyak 1.410.000 jiwa (2005), kota ini merupakan pusat ekonomi, politik, budaya, dan sosial di tenggara Tiongkok setelah Guangzhou. Administrasi Zhuhai...

Ташкентское землетрясение 41°19′26″ с. ш. 69°16′20″ в. д.HGЯO Дата и время 26 апреля 1966 года Магнитуда по шкале Рихтера 5,2 по шкале Рихтера Глубина гипоцентра от 8 до 3 км Расположение эпицентра Ташкент Затронутые страны (регионы) СССР Узбекская ССР Казахская ССР Кир�...

Public secondary school in Bladensburg, Maryland, United StatesBladensburg High SchoolAddress4200 57th AvenueBladensburg, MarylandUnited StatesCoordinates38°56′27″N 76°55′3″W / 38.94083°N 76.91750°W / 38.94083; -76.91750InformationTypePublic secondaryMottoWhere Excellence Is A Deliberate PracticeEstablished1936School districtPrince George's County Public SchoolsPrincipalLisa Faulkner-JonesGrades9–12Enrollment1,896 (2016-17)[1]Color(s) ...

Pour les articles homonymes, voir Alain, Émile Chartier et Chartier. AlainNaissance 3 mars 1868Mortagne-au-PercheDécès 2 juin 1951 (à 83 ans)Le VésinetSépulture Cimetière du Père-Lachaise, Grave of Alain (d)Nationalité françaiseFormation École normale supérieureLycée Pierre-CorneilleInfluencé par Jules Lagneau, René Descartes, Emmanuel Kant, Platon, Aristote, Auguste Comte, Georg Wilhelm Friedrich Hegel, Baruch SpinozaAdjectifs dérivés alinien[1]Distinctions Concours g�...

Presidential campaign for the 2022 Philippine presidential elections Bongbong Marcos 2022 presidential campaignCampaigned for2022 Philippine presidential electionCandidateBongbong Marcos Senator of the Philippines(2010–2016) Ilocos Norte's 2nd district representative(1992–1995; 2007–2010) Governor of Ilocos Norte(1983–1986; 1998–2007) Vice Governor of Ilocos Norte(1980–1983) Sara Duterte Mayor of Davao City (2010–2013; 2016–present) Vice Mayor of Davao City (2007–2010)Affili...

Equestrian rodeo sport Chuckwagon racingChuckwagons racing toward the finish line at the 2009 Calgary StampedeCharacteristicsTypeEquestrian rodeo sportPresenceOlympicNoParalympicNoWorld GamesNo Chuckwagon racing is an equestrian rodeo sport in which drivers in a chuckwagon led by a team of Thoroughbred horses race around a track. The sport is most popular in the Prairie Provinces of Canada, where the World Professional Chuckwagon Association and the Canadian Professional Chuckwagon Associatio...

Синелобый амазон Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:ЗавропсидыКласс:Пт�...

Imad Faraj Nazionalità Francia Altezza 177 cm Peso 64 kg Calcio Ruolo Ala, centrocampista Squadra AEK Larnaca Carriera Giovanili 2006-2016 Lilla Squadre di club1 2017-2018 Lilla7 (0)2016-2019 Lilla 251 (12)2019-2020→ Belenenses3 (0)2020 Lilla 24 (0)2020-2021→ R.E. Mouscron27 (1)2021- AEK Larnaca0 (0) Nazionale 2015 Francia U-167 (1)2015-2016 Francia U-172 (0)2016-2017 Francia U-188 (0)2017 Francia U-192 (0) 1 I due numeri indicano le pres...

Peta negara menunjukkan dinaungi oleh posisi mereka dalam Happy Planet Index (2006). Peringkat negara tertinggi adalah hijau terang, sedangkan terendah adalah coklat Happy Planet Index (HPI) adalah suatu indeks dampak kesejahteraan manusia dan lingkungan yang diperkenalkan oleh New Economics Foundation (NEF) pada Juli 2006. Pranala luar The Happy Planet Index Diarsipkan 2009-09-26 di Wayback Machine. Situs resmi dari New Economics Foundation Diarsipkan 2021-03-17 di Wayback Machine. Artikel b...

Not to be confused with Latour or La Tour. City in Maharashtra, IndiaLaturCityMarathwada Martyr Monument (Marathwada Hutatma Smarak), located in the cityLaturLocation in Maharashtra, IndiaShow map of MaharashtraLaturLatur (India)Show map of IndiaCoordinates: 18°24′N 76°34′E / 18.40°N 76.56°E / 18.40; 76.56CountryIndiaStateMaharashtraRegionMarathwadaDivisionAurangabadDistrictLaturSettledPossibly 7th century ADGovernment • TypeMayor–Council •...

This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: 1932 Washington Senators season – news · newspapers · books · scholar · JSTOR (July 2013) (Learn how and when to remove this message) Major League Baseball team season 1932 Washington SenatorsLeagueAmerican LeagueBallparkGriffith StadiumCityWashington, D....

本條目存在以下問題,請協助改善本條目或在討論頁針對議題發表看法。 此條目可参照葡萄牙語維基百科和英語維基百科相應條目来扩充,其中部分條目在對應語言版為高品質條目。 (2024年3月10日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶...

Absorbent towel made from paper Some of this article's listed sources may not be reliable. Please help improve this article by looking for better, more reliable sources. Unreliable citations may be challenged and removed. (February 2017) (Learn how and when to remove this message) A roll of paper towels (kitchen roll) A paper towel is an absorbent, disposable towel made from paper.[1] In Britain, paper towels for kitchen use are also known as kitchen rolls, kitchen paper, or kitchen t...

Erysimum cheiri Pour les articles homonymes, voir Ravenelle (homonymie). Erysimum cheiri Giroflée des muraillesClassification Règne Plantae Division Magnoliophyta Classe Magnoliopsida Ordre Brassicales Famille Brassicaceae Genre Erysimum EspèceErysimum cheiri(L.) Crantz, 1769 Classification phylogénétique Classification phylogénétique Ordre Brassicales Famille Brassicaceae La giroflée des murailles (Erysimum cheiri, syn. Cheiranthus cheiri), aussi appelée violier jaune ou ravenelle, ...

MS Independence of the Seas adalah kapal pesiar kelas Freedom dioperasikan oleh Royal Caribbean cruise line yang mulai beroperasi pada April 2008. Kapal 15-dek dapat menampung 4.370 penumpang dan dilayani oleh 1.360 awak. Kapal ini dibangun di galangan kapal Aker Finnyards Turku, Finlandia, builder dari Freedom of the Seas dan Liberty of the Seas, kapal adiknya dari kelas Freedom. Pada 154.407 gross ton, ia bergabung Freedom of the Seas dan Liberty of the Seas sebagai kapal pesiar terbesar d...

مقاطعة كوس الإحداثيات 44°36′50″N 71°20′24″W / 44.613773°N 71.339943°W / 44.613773; -71.339943 [1] [2] تاريخ التأسيس 1803 تقسيم إداري البلد الولايات المتحدة[3][4] التقسيم الأعلى نيوهامبشير[1] العاصمة لانكاستر التقسيمات الإدارية برلينكارولكلا�...

For military history, see Military history of Nova Scotia. Part of a series on theHistory of Nova ScotiaBluenose Events Port Royal established 1605 Conquest of Acadia 1710 Halifax established 1749 Bay of Fundy Campaign 1755 Fall of Louisbourg 1758 Representative Government established 1758 Halifax Treaties 1760–61 First significant Scottish immigration 1773 Battle of Fort Cumberland 1776 Birchtown established 1783 Capture of USS Chesapeake 1813 Freedom of the Press 1835 First Acadian MLA e...

Esta ilustración representa, de izquierda a derecha, las cinco áreas principales de la farmacología, las cuales son el cerebro, los riñones, el metabolismo, los radicales libres, las mitocondrias y la membrana plasmática. El arcoiris y el prisma representan la fotobiología y los vasos de precipitado la bioquímica La farmacología (del griego, pharmacon (φάρμακον), fármaco y logos (λόγος), ciencia)[1] es la rama de las ciencias farmacéuticas que estudia la historia...






![{\textstyle \left[-1,1+\sigma \right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/62717b2aa87cd979af08e19bb930543d74f14bbc)



![{\displaystyle [1,+\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e753c04c83a9874ecd5cd798b95b3b1e720e8c60)


























![{\displaystyle {\begin{aligned}\int _{x\in E}|p(x)|^{p}\,{\mbox{d}}x&\geq \int _{x\in E\setminus E_{\lambda }}|p(x)|^{p}\,{\mbox{d}}x\\[6pt]&\geqslant \lambda ^{p}{\frac {1}{2}}{\textrm {mes}}(E)\\[6pt]&={\frac {1}{2}}{\textrm {mes}}(E)\left({\frac {{\textrm {mes}}(E)}{2\,C\,\mathrm {mes} (J)}}\right)^{p(n-1)}e^{-p\max _{k}|\Re e(\lambda _{k})|\,\mathrm {mes} (J)}\max _{x\in J}|p(x)|^{p}\\[6pt]&\geqslant {\frac {1}{2}}{\frac {{\textrm {mes}}(E)}{{\textrm {mes}}(J)}}\left({\frac {{\textrm {mes}}(E)}{2\,C\,\mathrm {mes} (J)}}\right)^{p(n-1)}e^{-p\max _{k}|\Re e(\lambda _{k})|\,\mathrm {mes} (J)}\int _{x\in J}|p(x)|^{p}\,{\mbox{d}}x,\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/410c311252324e5b3edb30d57726be04737c781c)


