IL-2 Sturmovik: Great Battles

Este artículo trata sobre el videojuego de 2013. Para los videojuegos de 2001 y 2011, véanse IL-2 Sturmovik (videojuego) e IL-2 Sturmovik: Cliffs of Dover.
IL-2 Sturmovik: Great Battles
Información general
Desarrollador 1C Game Studios
Distribuidor 1C Company
Datos del juego
Género Simulador de vuelo de combate
Modos de juego Un jugador y multijugador
Datos del software
Motor Digital Nature Engine Ver y modificar los datos en Wikidata
Versión actual ()
Plataformas Windows
Desarrollo
Lanzamiento 19 de noviembre de 2013
Enlaces
[editar datos en Wikidata]

IL-2 Sturmovik: Great Battles, originalmente lanzado al mercado en 2013 bajo el título IL-2 Sturmovik: Battle of Stalingrad, es un simulador de vuelo de combate ambientado en la Segunda Guerra Mundial. Pertenece a la tercera generación de simuladores IL-2 Sturmovik, originados en 2001 con el primero de ellos, portador de ese mismo título, aunque hoy en día a esa primera generación se la conoce más por el título de su última compilación, IL-2 Sturmovik: 1946, lanzada al mercado en 2006. A la segunda generación corresponde IL-2 Sturmovik: Cliffs of Dover, simulador lanzado en 2011.

IL-2 Sturmovik: Battle of Stalingrad fue desarrollado en 2013 por la empresa rusa 1C Game Studios, resultante de una asociación entre la 1C Company y el desarrollador 777 Studios. El motor de juego de Battle of Stalingrad era el Digital Nature Engine, el mismo que el de otro simulador de 777 Studios, Rise of Flight (2009), ambientado este en la Primera Guerra Mundial. A partir de 2017, cambios en el motor de juego y la llegada de nuevos módulos ambientados en otras batallas, diferentes de la de Stalingrado, llevaron a 1C Game Studios a bautizar la nueva serie de simuladores como IL-2 Sturmovik: Great Battles y a rebautizar también su motor de juego, ya desde entonces llamado Digital Warfare Engine.

Teatros de operaciones

En 2013, el primer teatro de operaciones disponible para los jugadores fue el del primer juego en la serie, IL-2 Sturmovik: Battle of Stalingrad, ambientado en la Batalla de Stalingrado (1942-1943). En dicho módulo, los jugadores pueden pilotar aviones de la Fuerza Aérea Soviética (como el Lavochkin LaGG-3) o de la Luftwaffe (como el Messerschmitt Bf 109 F-4). Otros módulos han ido siendo estrenados paulatinamente desde entonces, lo que permite a los jugadores que los compran jugar en otras batallas de la Segunda Guerra Mundial e incluso en batallas aéreras de la Primera (con el módulo Flying Circus).

Desarrollo y lanzamiento al mercado

Logotipo original de IL-2 Sturmovik: Battle of Stalingrad (2013).

El inicio del desarrollo de IL-2 Sturmovik: Battle of Stalingrad fue anunciado oficialmente por 1C Game Studios en diciembre de 2012.[1]​ El juego fue finalmente lanzado al mercado el 19 de noviembre de 2013 como un acceso en línea para todos aquellos usuarios que hubiesen comprado la Edición Premium.[2]​ La Edición Estándar tardó casi un año en ser lanzada al mercado, también en línea en internet, el 22 de octubre de 2014.[3]​

IL-2 Sturmovik: Battle of Stalingrad es el título número 11 en la lista de productos IL-2 Sturmovik pero es en realidad el tercer simulador de la serie siguiendo el tipo de motor de juego. Los dos motores anteriores fueron el de la serie clásica, iniciada en 2001 con IL-2 Sturmovik y terminada en 2009 con IL-2 Sturmovik: Birds of Prey, y el de IL-2 Sturmovik: Cliffs of Dover, de 2011, que tuvo su propio motor de juego. IL-2 Sturmovik: Battle of Stalingrad usa el motor de juego de Rise of Flight, el simulador de Primera Guerra Mundial desarrollado y lanzado en 2009 por 777 Studios.

Nuevos módulos y creación de la marca Great Battles

En 2016 1C Game Studios lanzó el acceso inicial (en inglés early access) de la edición Premium de un nuevo módulo titulado IL-2 Sturmovik: Battle of Moscow. En 2017 1C declaró que los dos módulos publicados hasta entonces (Battle of Stalingrad y Battle of Moscow) hacían parte de una nueva serie de simuladores titulada IL-2 Sturmovik: Great Battles. En 2018 se publicó el tercer módulo (IL-2 Sturmovik: Battle of Kuban) y en 2019 tres módulos más (IL-2 Sturmovik: Battle of Bodenplatte, IL-2 Sturmovik: Flying Circus y IL-2 Sturmovik: Tank Crew). Flying Circus y Tank Crew tienen la particularidad de no estar versados en la aviación de la Segunda Guerra Mundial: Flying Circus está concebido para simular combates aéreos de la Primera Guerra Mundial y Tank Crew para conducir carros de combate de la Segunda.

Todos los módulos de la serie Great Battles son videojuegos de simulación en standalone, es decir que cada uno de ellos puede ser adquirido y utilizado con total independencia del resto.

Aviones pilotables en Battle of Stalingrad

De noviembre de 2013 a octubre de 2014 los poseedores de la Edición Premium de IL-2 Sturmovik: Battle of Stalingrad tuvieron acceso a una versión alfa del simulador, con dos aviones pilotables, el caza ruso Lavochkin LaGG-3 y el caza alemán Messerschmitt Bf 109 F-4. Desde la salida al mercado de la Edición Estándar de IL-2 Sturmovik: Battle of Stalingrad, el 22 de octubre de 2014, el acceso a los aviones pilotables es el siguiente:

  • Los poseedores de la Edición Premium adquieren los ocho aviones de la Edición Estándar además de los dos cazas siguientes:

Referencias

  1. ↑ «1C Game Studios anuncia, el 11 de diciembre de 2012, tanto la creación de la empresa como (por asociación de 1C Company y 777 Studios) como la puesta en desarrollo de un nuevo simulator titulado IL-2 Sturmovik: Battle of Stalingrad». Archivado desde el original el 5 de noviembre de 2013. Consultado el 21 de noviembre de 2013. 
  2. ↑ Early access now open, anuncio oficial del lanzamiento de IL-2 Sturmovik: Battle of Stalingrad en el sitio oficial del juego (19 de noviembre de 2013)
  3. ↑ We proudly present to you..., anuncio oficial, del 22 de octubre de 2014, del lanzamiento en línea de IL-2 Sturmovik: Battle of Stalingrad, para los usuarios que han decidido adquirir la Edición Estándar.

Véase también

Enlaces externos

Read other articles:

Giovanni da Verrazzano

Giovanni da Verrazzano. Giovanni da Verrazzano (c. 1485 – c. 1528) adalah pengelana Italia ke Amerika Utara, dengan membawa nama Prancis. Ia terkenal sebagai orang Eropa pertama yang melewati pantai Atlantik Amerika Utara dari South Carolina hingga Newfoundland, termasuk New York Harbor dan Teluk Barragansett tahun 1524. Pranala luar Biography at the Dictionary of Canadian Biography Online Diarsipkan 2009-10-05 di Wayback Machine. Giovanni da Verrazzano (Giovanni da Verrazano) at Greve in C...

 

بول زاميكنيك

بول زاميكنيك   معلومات شخصية الميلاد 22 نوفمبر 1912   كليفلاند  الوفاة 27 أكتوبر 2009 (96 سنة) [1]  بوسطن  مكان الدفن مقبرة ماونت أوبورن  مواطنة الولايات المتحدة  عضو في الأكاديمية الوطنية للعلوم،  والأكاديمية الأمريكية للفنون والعلوم  الحياة العملية الم�...

 

NCSM Iroquois (DDG 280)

Cet article est une ébauche concernant une unité ou formation militaire canadienne. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Pour les articles homonymes, voir NCSM Iroquois. NCSM Iroquois Le NCSM Iroquois en 2009 Autres noms DDG 280 Type Destroyer Classe Classe Iroquois Histoire A servi dans  Marine royale canadienne Chantier naval Marine Industries Ltd, Québec. Quille posée 15 janvier 1969 Lance...

بييترو مارينو

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (سبتمبر 2018) بييترو مارينو معلومات شخصية الميلاد 21 نوفمبر 1986 (العمر 37 سنة)رية قلورية  الطول 2.00 م (6 قدم 6 1⁄2 بوصة) مركز اللعب حارس مرمى الجنسية إيطاليا  م�...

 

Chittagong-2

Constituency of Bangladesh's Jatiya Sangsad Chittagong-2Constituencyfor the Jatiya SangsadDistrictChittagong DistrictDivisionChittagong DivisionElectorate376,485 (2018)[1]Current constituencyCreated1973Parliamentary PartyAwam LeagueMember of ParliamentKhadizatul AnwarCouncil areaFatikchhari UpazilaPrev. ConstituencyChittagong-1 (Constituency 278)Next ConstituencyChittagong-3 (Constituency 280) Chittagong-2 is a constituency represented in the Jatiya Sangsad (National Parliament) of Ba...

 

Constand Viljoen

Constand Viljoen pada 1984 Constand Viljoen SSA SD SOE SM MMM MP (lahir 28 Oktober 1933) adalah seorang mantan komandan militer dan politikus Afrika Selatan. Ia dikenal karena menghindarkan perpecahan kekerasan bersenjata dengan mengamankan orang kulit putih Afrika Selatan sebelum pemilihan umum pasca-apartheid. Ia menikahi Christina Susanna Heckroodt dan memiliki empat putra dan seorang putri.[1] Referensi ^ Gen. Constand Viljoen. Volkstaat.net. Boerevolkstaa...

Kassala

Town in Kassala State, Sudan Town in Kassala State, SudanKassala كسلاTownKassalaLocation in SudanCoordinates: 15°27′N 36°24′E / 15.450°N 36.400°E / 15.450; 36.400Country SudanStateKassala StatePopulation (2002) • Total957,000[1] Kassala (Arabic: كسلا) is the capital of the state of Kassala in eastern Sudan. In 2002 its population was recorded to be 957,000.[1] Built on the banks of the Gash River, it is a market town and...

 

USS Card

Bogue-class escort carrier of the US Navy USS Card (CVE-11), in 1943 History United States OwnerWar Shipping Administration (WSA) OperatorIsthmian Steamship Company Orderedas type (C3-S-A1 hull), MC hull 178 [1] Awarded30 October 1940 BuilderSeattle-Tacoma Shipbuilding Corporation, Tacoma, Washington Cost$3,286,653 Yard number10 Way number2 Laid down27 October 1941 Launched21 February 1942 Sponsored byMrs. J. Perry FateSold for scrapping on 14 May 1971 United States NameCard NamesakeC...

 

Boasian anthropology

Anthropology model Franz Boas (1858–1942) founder of the Boasian tradition in American anthropology Boasian anthropology was a school within American anthropology founded by Franz Boas in the late 19th century. Overview Boasian anthropology was based on the four-field model of anthropology uniting the fields of cultural anthropology, linguistic anthropology, physical anthropology, and archaeology under the umbrella of anthropology. It was based on an understanding of human cultures as malle...

コルク

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。（このテンプレートの使い方）出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL（2017年4月） コルクを打ち抜いて作った瓶の栓 コルク（木栓、�...

 

Governor of the Virgin Islands

Representative of the British monarch to the British Virgin Islands This article is about the British official. For the American official, see List of governors of the United States Virgin Islands. Governor of the Virgin IslandsStandard of the governorArms of the British Virgin IslandsIncumbentDaniel Prucesince 29 January 2024StyleHis ExcellencyResidenceGovernment House, British Virgin IslandsAppointerKing of the United KingdomFormation1887First holderEdward John Cameron as Administrator...

 

Suzanne Pleshette

Suzanne PleshetteBiographieNaissance 31 janvier 1937New YorkDécès 19 janvier 2008 (à 70 ans)Los AngelesSépulture Hillside Memorial ParkNationalité américaineFormation Finch College (en)Neighborhood Playhouse School of the TheatreFiorello H. LaGuardia High School of Music & Art and Performing ArtsUniversité de SyracuseActivité ActricePériode d'activité 1957-2004Conjoints Troy Donahue (1964)Tom Poston (de 2001 à 2007)Autres informationsDistinction Étoile du Hollywood Walk o...

Blyth Offshore Wind Farm

Blyth Offshore Wind FarmBlyth Offshore Wind Turbines in January 2004Location of Blyth Offshore Wind Farm in NorthumberlandCountryEngland, United KingdomLocationBlyth, NorthumberlandCoordinates55°08′09″N 01°29′25″W / 55.13583°N 1.49028°W / 55.13583; -1.49028StatusDecommissioned & RemovedCommission dateDecember 2000Decommission dateMarch 2019Construction cost€4.6 millionOwner(s)E.ONShell RenewablesNUONBorder WindOperator(s)E.ON EDF ...

 

2020年夏季奧林匹克運動會波蘭代表團

2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl（英文）（波兰文）2020年夏季奥林匹克运动会（東京）2021年7月23日至8月8日（受2019冠状病毒病疫情影响推迟，但仍保留原定名称）運動員206參賽項目24个大项旗手开幕式：帕维尔·科热尼奥夫斯基（游泳）和马娅·沃什乔夫斯卡（自行车）[1]闭幕式：卡罗利娜·纳亚（皮划艇）&#...

 

Савинков, Борис Викторович

В Википедии есть статьи о других людях с такой фамилией, см. Савинков. Борис Викторович Савинков Дата рождения 19 (31) января 1879 или 31 января 1879(1879-01-31)[1] Место рождения Харьков, Российская империя[2] Дата смерти 7 мая 1925(1925-05-07)[2][3][…] (46 лет) Место смерт�...

Banded stilt

Species of Australian bird in the family Recurvirostridae Banded stilt Banded stilts,Rottnest Island Conservation status Least Concern  (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Aves Order: Charadriiformes Family: Recurvirostridae Genus: CladorhynchusG.R. Gray, 1840 Species: C. leucocephalus Binomial name Cladorhynchus leucocephalus(Vieillot, 1816) Banded stilt natural range Synonyms[2] Recurvirostra leucoceph...

 

The Legend of Spyro: Dawn of the Dragon

2008 video game This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: The Legend of Spyro: Dawn of the Dragon – news · newspapers · books · scholar · JSTOR (July 2009) (Learn how and when to remove this message) 2008 video gameThe Legend of Spyro:Dawn of the DragonDeveloper(s)Étranges Libellules (console)Tantalus...

 

Tin Yat Estate

Public housing estate in Tin Shui Wai, Hong Kong Tin Yat EstateTin Yat EstateGeneral informationLocation76 Tin Shui Road, Tin Shui WaiNew Territories, Hong KongCoordinates22°28′03″N 113°59′56″E / 22.467372°N 113.999013°E / 22.467372; 113.999013StatusCompletedCategoryPublic rental housingPopulation14,589[1] (2016)No. of blocks9[2]No. of units3,330[2]ConstructionConstructed2001; 23 years ago (2001)AuthorityHong K...

Sławomir Mrożek

Polish dramatist, writer and cartoonist Sławomir MrożekWarsaw, 21 May 2006Born(1930-06-29)29 June 1930Borzęcin, PolandDied15 August 2013(2013-08-15) (aged 83)Nice, FranceOccupationDramatist, writerNationalityPolishCitizenshipPolish, FrenchNotable worksTangoThe PoliceNotable awardsKościelski Award (1962)Austrian State Prize for European Literature (1972)Legion of Honour (2003)[1]Grand Cross of the Order of Polonia Restituta (2013)[2]Signature Sławomir Mrożek (29 June...

 

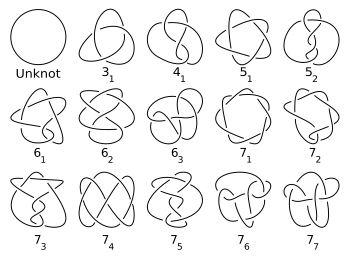
Prime knot

Non-trivial knot which cannot be written as the knot sum of two non-trivial knots Simplest prime link In knot theory, a prime knot or prime link is a knot that is, in a certain sense, indecomposable. Specifically, it is a non-trivial knot which cannot be written as the knot sum of two non-trivial knots. Knots that are not prime are said to be composite knots or composite links. It can be a nontrivial problem to determine whether a given knot is prime or not. A family of examples of prime knot...