Zero moment point
|
Read other articles:

Artikel ini bukan mengenai keiretsu Mitsubishi. Mitsubishi CorporationGedung Marunouchi Park, kantor pusat Mitsubishi Corporation di Marunouchi, Chiyoda, Tokyo.Nama asli三菱商事株式会社Nama latinMitsubishi Shōji Kabushiki-gaishaSebelumnyaKowa Jitsugyo KaishaMitsubishi ShojiJenisPublik (K.K)Kode emitenTYO: 8058LSE: MBCTOPIX Core 30 ComponentIndustriPerusahaaan dagangDidirikanDidirikan pada tahun 1918Didirikan kembali pada tahun 1954KantorpusatMarunouchi, Chiyoda, Tokyo, JepangCabang22...

Main-belt asteroid 78 DianaOrbital diagramDiscoveryDiscovered byKarl Theodor Robert LutherDiscovery dateMarch 15, 1863DesignationsMPC designation(78) DianaPronunciation/daɪˈænə, daɪˈeɪnə/ dy-A(Y)N-ə[1]Named afterDiāna (Roman mythology)Minor planet categoryMain beltAdjectivesDianian (/daɪˈeɪniən/ dy-AY-nee-ən)Orbital characteristics[2]Epoch December 31, 2006 (JD 2454100.5)Aphelion473.182 Gm (3.163 AU)Perihelion310.686 Gm (2.077&...

Pour les articles homonymes, voir Wöhler. Friedrich WöhlerFriedrich Wöhler.BiographieNaissance 31 juillet 1800Eschersheim (Landgraviat de Hesse-Cassel, Saint-Empire romain germanique)Décès 23 septembre 1882 (à 82 ans)Göttingen (royaume de Prusse, Empire allemand)Sépulture StadtfriedhofNationalités allemandeprussienneÉlectorat de HesseLandgraviat de Hesse-CasselFormation Université de MarbourgUniversité de HeidelbergActivités Chimiste, professeur d'université, biochimisteAu...

Former province of Japan Echizen Province越前国pre-Meiji period Japan701 AD–1871Map of Japanese provinces (1868) with Echizen Province highlightedCapitalEchizenArea • Coordinates36°24′N 136°30′E / 36.400°N 136.500°E / 36.400; 136.500 History • Ritsuryō system implemented 701 AD• Disestablished 1871 Today part ofFukui Prefecture Echizen Province (越前国, Echizen-no-kuni) was a province of Japan in the area that is today th...

Subprefecture and commune in Occitania, FranceFlorac Trois Rivières Florac-Tres-Rius (Occitan)Subprefecture and communeLocation of Florac Trois Rivières Florac Trois RivièresShow map of FranceFlorac Trois RivièresShow map of OccitanieCoordinates: 44°19′26″N 3°35′38″E / 44.324°N 3.594°E / 44.324; 3.594CountryFranceRegionOccitaniaDepartmentLozèreArrondissementFloracCantonFlorac Trois RivièresIntercommunalityGorges Causses CévennesGovernment ...
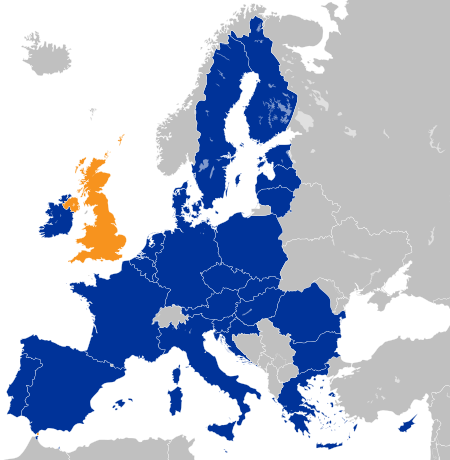
UK withdrawal from the European Union (2020) For other uses, see Brexit (disambiguation). Part of a series of articles onBrexit Withdrawal of the United Kingdom from the European Union Glossary of terms Background European Communities Act 1975 EC membership referendum UK rebate Bruges speech No. No. No. Maastricht Rebels Black Wednesday European Union (Amendment) Act 2008 European Union Act 2011 UK opt-outs from EU legislation Euroscepticism in the UK UK opinion polling on EU membership Campa...

Pinacothèque nationale d'AthènesMusée Aléxandros-SoútzosFaçade principale de la Pinacothèque nationale d'Athènes.Informations généralesType Musée d'art, musée national (d), pinacothèqueOuverture 1900Surface 20 760 m2 (extension en 2021)Visiteurs par an environ 290 000Sites web (el) www.nationalgallery.gr(en) www.nationalgallery.gr/enCollectionsCollections Peintures grecques du XVe au XXe sièclePeintures occidentales à partir du XIVe siècle.N...

Joanna CassidyJoanna Cassidy, 2007.LahirJoanna Virginia Caskey2 Agustus 1945 (umur 78)Haddonfield, New Jersey, A.S.PekerjaanAktrisTahun aktif1965–sekarangSuami/istriKennard C. Kobrin (m. 1964; c. 1974)Anak2 Joanna Cassidy (nee Joanna Virginia Caskey, lahir 2 Agustus 1945) adalah seorang aktris Amerika. Dia dikenal karena perannya sebagai Zhora Salome di Blade Runner (1982) dan Dolores di Who Framed Roger Rabbit (1988).[1]...

For the 1934 American film, see She Was a Lady (film). She Was a Ladyalso Angels of Doomalso The Saint Meets His Match First editionAuthorLeslie CharterisCountryUnited KingdomLanguageEnglishSeriesThe SaintGenreMystery novelPublisherHodder and StoughtonPublication date1931Media typePrint (Hardback & Paperback)Preceded byAlias the Saint (UK)Wanted for Murder (US) Followed byThe Holy Terror She Was a Lady is the title of a mystery novel by Leslie Charteris featuring...

LA to the Moon TourLana Del Rey mentre si esibisce a Houston nel febbraio 2018Tour di Lana Del ReyAlbumLust for Life Inizio Minneapolis 5 gennaio 2018 Fine Madrid 20 aprile 2018 Tappe4 Spettacoli23 in Nord America4 in Sud America3 in Oceania6 in Europa36 totali Cronologia dei tour di Lana Del Rey The Endless Summer Tour(2015) The Norman Fucking Rockwell Tour(2019) Il LA to the Moon Tour è il quarto tour musicale della cantautrice statunitense Lana Del Rey, a supporto del suo quinto album in ...

Railway station in Jiangmen, Guangdong Jiangmen江门Station buildingGeneral informationLocationXinhui District, Jiangmen, GuangdongChinaLine(s)Guangzhou–Zhuhai railway (freight only)Guangzhou–Zhuhai intercity railwayShenzhen–Zhanjiang high-speed railwayZhuhai-Zhaoqing High-Speed RailwayOther informationStation codeTMIS code: 23498Telegraph code: JOQPinyin code: JMEHistoryOpened29 December 2012 (freight)15 November 2020 (passenger)Services Preceding station China Railway High-speed Foll...

أحمد زكي أبو شادي معلومات شخصية الميلاد 9 فبراير 1892 [1][2] القاهرة الوفاة 12 أبريل 1955 (63 سنة) واشنطن العاصمة مكان الدفن مقبرة فورت لينكولن [لغات أخرى] مواطنة الدولة العثمانية (1892–1914) السلطنة المصرية (1914–1922) المملكة المصرية (1922–1953) جمهورية ...

莎拉·阿什頓-西里洛2023年8月,阿什頓-西里洛穿著軍服出生 (1977-07-09) 1977年7月9日(46歲) 美國佛羅里達州国籍 美國别名莎拉·阿什頓(Sarah Ashton)莎拉·西里洛(Sarah Cirillo)金髮女郎(Blonde)职业記者、活動家、政治活動家和候選人、軍醫活跃时期2020年—雇主內華達州共和黨候選人(2020年)《Political.tips》(2020年—)《LGBTQ國度》(2022年3月—2022年10月)烏克蘭媒�...

طبق كراهي اللحم المطبخ الباكستاني (بالأردية: پاکستانی پکوان) هو مطبخ عريق وراق من مزيج من المأكولات المختلفة المعروفة باكستان. ومن المعروف عن المطبخ الباكستاني ثرائه ونكهته الغنية.[1] تختلف الاطباق داخل باكستان اختلافا كبيرا من منطقة إلى أخرى، والتي تعكس التنوع العرقي ...

Halaman ini berisi artikel tentang penulis dan jurnalis. Untuk pemberi pengumuman dan eksekutif radio olahraga, lihat David J. Halberstam. David HalberstamLahir(1934-04-10)10 April 1934New York City, New York, ASMeninggal23 April 2007(2007-04-23) (umur 73)Menlo Park, California, ASPekerjaanJurnalis, penulis, sejarawanKebangsaanAmerikaPendidikanUniversitas HarvardGenreNon-fiksiPasanganElżbieta Czyżewska (1965-1977; diceraikan)Jean Sandness Butler (1979-2007; dia meninggal; 1 anak) David...

‹ 1838 und 1839 • • 1842 und 1843 › Wahlen zum Senat 1840 und 1841 Siegel des Senats der Vereinigten Staaten 18 Senatoren der Klasse II (sowie außerordentliche Wahlen) Whigs Davor 23 Danach 29 57 % der Sitze Demokraten Davor 29 Danach 22 43 % der Sitze Wahlergebnisse nach Bundesstaat 19 Sitze Whigs 6 Sitze Demok...

Historical Chinese concept During the late Zhou dynasty, the inhabitants of the Central Plains began to make a distinction between Hua and Yi (Chinese: 華夷之辨; pinyin: huáyí zhībiàn), referred to by some historians as the Sino–barbarian dichotomy.[1] They defined themselves as part of cultural and political region known as Huaxia, which they contrasted with the surrounding regions home to outsiders, conventionally known as the Four Barbarians (literally, four Yi). ...

Road tunnel in Bergen, Norway Fløyfjell TunnelFløyfjelltunnelenThe entrance to the tunnel is in the lower right part of the pictureOverviewLocationVestland, NorwayCoordinates60°24′13″N 5°20′11″E / 60.40361°N 5.33639°E / 60.40361; 5.33639Route E16 / E39OperationOpened1989OperatorNorwegian Public Roads AdministrationTraffic43,189 (2010)TechnicalLength3,825 metres (12,549 ft) (northbound tube) 3,195 metres (10,482 ft) (southbound tube) The Fløyfje...

Defunct provincial electoral district in Alberta, Canada Calgary-Fort Alberta electoral district2010 boundariesDefunct provincial electoral districtLegislatureLegislative Assembly of AlbertaDistrict created1996District abolished2017First contested1997Last contested2015 Calgary-Fort was a provincial electoral district in Alberta, Canada, mandated to return a single member to the Legislative Assembly of Alberta using the first past the post method of voting from 1997 to 2019. History The Riding...

Historic site in Queensland, AustraliaBryntirionBryntirion, 2013Location287 Wickham Terrace, Spring Hill, City of Brisbane, Queensland, AustraliaCoordinates27°27′53″S 153°01′19″E / 27.4648°S 153.022°E / -27.4648; 153.022Design period1840s–1860s (mid-19th century)Built1861–1930sBuilt forEdward Barton SoutherdenArchitectJames Furnival Queensland Heritage RegisterOfficial nameBryntirionTypestate heritage (landscape, built)Designated17 December 1993Referen...






















