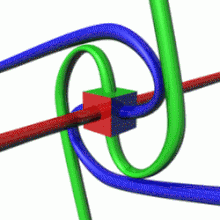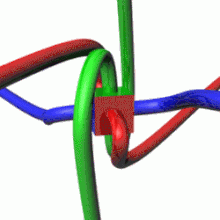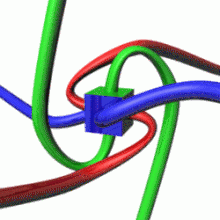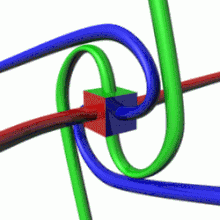
Spin-1/2
|
Read other articles:

HelplessNama lainHangul화차 Hanja火車 Alih Aksara yang DisempurnakanHwachaMcCune–ReischauerHwach‘a SutradaraByun Young-jooProduserShin Hye-eun Oh Ki-minDitulis olehByun Young-jooBerdasarkanAll She Was Wortholeh Miyabe MiyukiPemeranKim Min-hee Lee Sun-kyun Jo Sung-haPenata musikKim Hong-jibSinematograferKim Dong-youngPenyuntingPark Gok-jiPerusahaanproduksiFilament PicturesDistributorCJ E&MTanggal rilis 8 Maret 2012 (2012-03-08) Durasi117 menitNegaraKorea SelatanBah...

Hjalmar Bergman di Wadköping Örebro ialah sebuah kota di Swedia yang berpenduduk 95.354 jiwa, terletak di Daerah Örebro, bekas provinsi Närke, Svealand. Kota ini terkenal akan Örebro slott, yang terletak di sebuah pulau di Svartaan, yang mengalir melalui kota ini. Wikimedia Commons memiliki media mengenai Örebro. Artikel bertopik geografi atau tempat Swedia ini adalah sebuah rintisan. Anda dapat membantu Wikipedia dengan mengembangkannya.lbs

Jaranan dangdutSumber aliranMelayu, Indonesia, Gamelan, JarananSumber kebudayaanMelayu dan JawaAlat musik yang biasa digunakanTabla (dapat diganti dengan ketipung), kendang, drum set, suling, tamborin, gitar (akustik atau elektrik), bass, terompet, kenong, dll. Kecapi, seruling dan sitar pada relief Borobudur, foto c. 1890 Musik dari Indonesia Garis waktuContoh Jenis KlasikDaerahTradisionalJazzHip hopPopRokR&BKeroncongDangdut Bentuk khusus Jawa KidungTembangLanggamGamelanAngklungCal...

American ice hockey player and coach Ice hockey player Peter Laviolette Laviolette in 2014Born (1964-12-07) December 7, 1964 (age 59)Franklin, Massachusetts, U.S.Height 6 ft 2 in (188 cm)Weight 200 lb (91 kg; 14 st 4 lb)Position DefenseShot LeftPlayed for New York RangersCurrent NHL coach New York RangersCoached for New York IslandersCarolina HurricanesPhiladelphia FlyersNashville PredatorsWashington CapitalsNational team United StatesNHL Draft Un...

For other uses, see Jessica (disambiguation). JessicaA painting depicting Shylock and Jessica by Maurycy Gottlieb. The first use of the name Jessica is found in William Shakespeare's The Merchant of Venice. Act 2, Scene 3: (2.3.1) Enter Iessica and the Clowne.Pronunciation/ˈdʒɛsɪkə/GenderFemaleOriginWord/nameEarly Modern English, derived from Hebrew, ultimately from the triconsonantal root ס־כ־ה, 'to see, behold, look for'Meaninghe will see/behold/look forRegion of originMes...

كلمة ثينج (بالإنجليزية: thing) والتي تعني بالعربية شيء.[1][2][3] (ثينج باللغة الإسكندنافية القديمة والإنجليزية القديمة، والايسلندية هي (þing), وفي اللغات الإسكندنافية الأكثر حداثة ينطقونها (ting). هو اسم جمعية أو مجموعة كانت تنظم شؤون القبائل الجرمانيون, وقامت أيضا باد...

У этого термина существуют и другие значения, см. Верба (значения). БМ-21У «Верба» Классификация реактивная система залпового огня Шасси КрАЗ-6322–010[1][2] История Страна-разработчик Украина Годы производства с декабря 2015 Количество выпущенных 113[3] шт. Разме...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article ne cite pas suffisamment ses sources (août 2019). Si vous disposez d'ouvrages ou d'articles de référence ou si vous connaissez des sites web de qualité traitant du thème abordé ici, merci de compléter l'article en donnant les références utiles à sa vérifiabilité et en les liant à la section « Notes et références ». En pratique : Quelles sources sont attendues ? Com...

Census-designated place in California, United StatesRunning SpringsCensus-designated placeLocation in San Bernardino County and the state of CaliforniaRunning SpringsLocation in the United StatesCoordinates: 34°12′28″N 117°6′30″W / 34.20778°N 117.10833°W / 34.20778; -117.10833Country United StatesState CaliforniaCountySan BernardinoArea[1] • Total4.213 sq mi (10.912 km2) • Land4.204 sq mi (...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题,请协助参考外语维基百科扩充条目。请勿直接提交机械翻译,也不要翻译不可靠、低品质内容。依版权协议,译文需在编辑摘要注明来源,或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌:《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. (أبريل 2019) لامار توماس معلومات شخصية الميلاد 12 فبراير 1970 (54 سنة) أوكالا مواطنة الولايات المتحدة الحياة العملية المدرسة الأم جامعة ميامي المهنة لاعب كرة قد�...

Painting by Gustav Klimt Water Serpents IIArtistGustav KlimtYear1904–1907MediumOil on canvasDimensions80 cm × 145 cm (31 in × 57 in)LocationPrivate collection, Asia Water Serpents II, also referred to as Wasserschlangen II, is an oil painting made by Gustav Klimt in 1907. It is the follow-up painting to the earlier painting Water Serpents I. Like the first painting, Water Serpents II deals with the sensuality of women's bodies and same-sex relatio...

هاكان ميلد معلومات شخصية الميلاد 14 يونيو 1971 (53 سنة) ترولهتان الطول 1.82 م (5 قدم 11 1⁄2 بوصة) مركز اللعب وسط الجنسية السويد المسيرة الاحترافية1 سنوات فريق م. (هـ.) 1989–1993 غوتبورغ 92 (9) 1993–1995 سيرفيت 21 (1) 1995–1996 غوتبورغ 23 (5) 1996–1998 ريال سوسيداد 50 (1) 1998–2001 غوتبورغ 66 ...

Mirah darahUmumKategoriKalsedonRumus(unit berulang)Silika (silikon dioksida, SiO2)Sistem kristalTrigonalIdentifikasiMassa molekul60 g/molWarnaMerah kecoklatanBelahanTidak adaFrakturTidak rata, serpihan, konkoidKekerasan dalam skala Mohs6–7Kilaukaca, kusam, berminyak, halusGoresPutihDiafaneitasTembus cahayaBerat jenis2.59–2.61Referensi[1] Mirah darah adalah batuan mineral akik merah kecokelatan yang biasanya digunakan sebagai batu permata. Batu ini adalah ragam dari mineral silika ...

Flat transitional edge between two faces of a manufactured object For the concept in mathematics, see chamfer (geometry). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Chamfer – news · newspapers · books · scholar · JSTOR (April 2019) (Learn how and when to remove this message) A chamfer with a lark's tong...

هذه المقالة تحتاج للمزيد من الوصلات للمقالات الأخرى للمساعدة في ترابط مقالات الموسوعة. فضلًا ساعد في تحسين هذه المقالة بإضافة وصلات إلى المقالات المتعلقة بها الموجودة في النص الحالي. (مارس 2018) لمعانٍ أخرى، طالع مقاطعة مورغان (توضيح). مقاطعة مورغان الإحداثيات ...

سوق المدينةمعلومات عامةالتقسيم الإداري حلب القديمة البلد سوريا الإحداثيات 36°12′N 37°09′E / 36.2°N 37.15°E / 36.2; 37.15 تعديل - تعديل مصدري - تعديل ويكي بيانات الشارع الرئيسي في سوق المدينة 36°12′N 37°09′E / 36.200°N 37.150°E / 36.200; 37.150 سوق المدينة أحد أسواق حلب القديمة.&...

YG Entertainment Inc.Logo resmi sejak 2013.[1]Nama asliYG 엔터테인먼트Nama latinYG enteoteinmeonteuJenisPublicKode emitenKRX: 122870IndustriEntertainmentRetailGenreVariousDidirikan24 Februari 1996; 28 tahun lalu (1996-02-24)PendiriYang Hyun-sukKantor pusat397-5 Hapjeong-dong, Mapo-gu, Seoul, South KoreaWilayah operasiWorldwideTokoh kunciYang Min-sukHwang Bo-kyung (co-CEOs)[2]Pendapatan{ US$ 228.81 juta (2021)Laba operasi US$ 32.02 juta (2021)Laba bersihUS$ 6.53 juta...

Russian-Filipino pair skater In this name that follows Eastern Slavic naming customs, the patronymic is Sergeyevich and the family name is Korovin. Alexander KorovinKorovin at the 2019 UniversiadeFull nameAlexander Sergeyevich KorovinNative nameАлександр Сергеевич КоровинOther namesAleksandr KorovinBorn (1994-02-15) 15 February 1994 (age 30)Pervouralsk, RussiaHeight1.81 m (5 ft 11+1⁄2 in)Figure skating careerCountry Philippines ...

German lithographer and painter Self-portrait (1810) Portrait of Bertel Thorvaldsen (c.1810) Rudolph Friedrich Carl Suhrlandt (19 December 1781, in Ludwigslust – 2 February 1862, in Schwerin) was a German portrait painter and lithographer. Biography His father, Johann Heinrich Suhrlandt, was a court painter for Grand Duke Fredrick Francis I of Mecklenburg. His first art lessons came from his father. In 1799, he became a student at the Dresden Academy of Fine Arts, with a royal scholarship a...