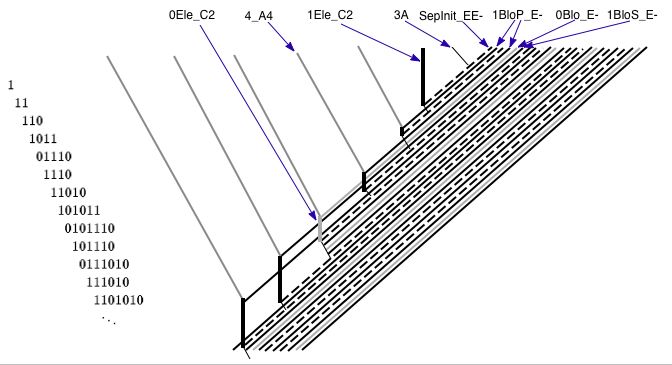
Rule 110
|
Read other articles:

لمعانٍ أخرى، طالع وزارة الصحة (توضيح). وزارة الصحة العمومية Ministère de la Santé publique مقر الوزارة. البلد تونس المقر الرئيسي شارع جبل الأخضر، باب سعدون، 1029 تونس العاصمة - تونس النوع وزارة اللغات الرسمية العربية، الفرنسية الرئيس قيس سعيد الوزير علي مرابط المالية المواز�...

This article is about the city in Germany. For other uses, see Marburg (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Marburg – news · newspapers · books · scholar · JSTOR (August 2013) (Learn how and when to remove this template message) Town in Hesse, GermanyMarburg TownView of Marburg, ...

ليس هناك أسلوب استشهاد مُحدد مُستعمل في هذه المقالة. فضلاً، ساهم في تطويرها من خلال توحيد أسلوب الاستشهاد المستعمل فيها. (يناير 2011) نهائي كأس العالم لكرة القدمالحدثكأس العالم لكرة القدم 1966 إنجلترا ألمانيا الغربية 4 2 بعد الوقت الإضافيالتاريخ30 يوليو 1966الملعبويمبلي، لندنالح...

American cable television, telephone, and Internet service provider This article is about the American communications company. For the Colombian broadcasting company, see RCN TV. A major contributor to this article appears to have a close connection with its subject. It may require cleanup to comply with Wikipedia's content policies, particularly neutral point of view. Please discuss further on the talk page. (January 2016) (Learn how and when to remove this template message) RCN CorporationC...

Karl MarlantesKarl MarlantesBiographieNaissance 24 décembre 1944 (79 ans)AstoriaNationalité américaineDomicile WoodinvilleFormation Université YaleSeaside High School (en)University CollegeJonathan Edwards College (en)Activités Officier, écrivain, romancier, réalisateur de documentaireAutres informationsArme Corps des Marines des États-UnisGrade militaire First lieutenantConflit Guerre du Viêt NamDistinctions Liste détailléeBronze StarNavy CrossAir MedalPurple HeartBourse Rhod...

Voce principale: Saronno Foot-Ball Club 1910. Saronno Foot Ball ClubStagione 1951-1952Sport calcio Squadra Saronno Allenatore Felice Renoldi Presidente Gino Colombo Serie C11º posto nel girone A. Retrocesso in IV Serie. 1950-1951 1959-1960 Si invita a seguire il modello di voce Questa voce raccoglie le informazioni riguardanti il Saronno Foot Ball Club nelle competizioni ufficiali della stagione 1951-1952. Rosa N. Ruolo Calciatore A R. Broggini A P. Caretti A M. Crippa C Donzelli C L. ...

Protein found in humans EREGAvailable structuresPDBOrtholog search: PDBe RCSB List of PDB id codes1K36, 1K37, 5E8DIdentifiersAliasesEREG, EPR, ER, Ep, epiregulinExternal IDsOMIM: 602061 MGI: 107508 HomoloGene: 1097 GeneCards: EREG Gene location (Human)Chr.Chromosome 4 (human)[1]Band4q13.3Start74,365,145 bp[1]End74,388,749 bp[1]Gene location (Mouse)Chr.Chromosome 5 (mouse)[2]Band5|5 E1Start91,222,481 bp[2]End91,241,505 bp[2]RNA expression p...

1999 single by Chris Cornell For the Vince Neil song Can't Change Me, see Exposed (Vince Neil album). Can't Change MeSingle by Chris Cornellfrom the album Euphoria Morning B-sideFlutter Girl,Nowhere But YouReleased1999Recorded1998Studio11 AD Studios in Los Angeles, California[1]GenreAlternative rockLength3:23LabelInterscopeSongwriter(s)Chris CornellProducer(s)Chris CornellNatasha ShneiderAlain JohannesChris Cornell singles chronology Sunshower (1998) Can't Change Me (1999) Preaching t...

Ираклеониты — ученики гностика Ираклеона (II век). Упоминаются как особая секта Епифанием и Августином; при крещении и миропомазании они соблюдали обряд помазания елеем и при этом произносили воззвания на арамейском языке, которые должны были освободить душу от власт�...

Dinosaurs in the Jurassic Park franchise Promotional image for Jurassic World: Fallen Kingdom, featuring multiple dinosaurs from the film. Jurassic Park, later also referred to as Jurassic World,[1] is an American science fiction adventure media franchise. It focuses on the cloning of dinosaurs through ancient DNA, extracted from mosquitoes that have been fossilized in amber. The franchise explores the ethics of cloning and genetic engineering, and the morals behind de-extinction. The...

2003 video gameDungeons & Dragons: HeroesCover art by Glenn FabryDeveloper(s)Atari Interactive Hunt Valley StudioPublisher(s)Atari InteractiveProducer(s)Martin DeRisoDesigner(s)Brenda BrathwaiteProgrammer(s)Will GeeArtist(s)David ThompsonComposer(s)Gary SpinradPlatform(s)XboxReleaseNA: September 16, 2003EU: November 14, 2003AU: November 21, 2003Genre(s)Hack and slashMode(s)Single-player, multiplayer Dungeons & Dragons: Heroes is a hack and slash video game with RPG elements. It was pu...

Ця стаття потребує додаткових посилань на джерела для поліпшення її перевірності. Будь ласка, допоможіть удосконалити цю статтю, додавши посилання на надійні (авторитетні) джерела. Зверніться на сторінку обговорення за поясненнями та допоможіть виправити недоліки. Мат...

此條目需要补充更多来源。 (2021年7月4日)请协助補充多方面可靠来源以改善这篇条目,无法查证的内容可能會因為异议提出而被移除。致使用者:请搜索一下条目的标题(来源搜索:美国众议院 — 网页、新闻、书籍、学术、图像),以检查网络上是否存在该主题的更多可靠来源(判定指引)。 美國眾議院 United States House of Representatives第118届美国国会众议院徽章 众议院旗...

اقتحام المسجد الأقصى جزء من القضية الفلسطينية المعلومات البلد دولة فلسطين الموقع القدس الإحداثيات 31°46′34″N 35°14′09″E / 31.77617°N 35.23583°E / 31.77617; 35.23583 التاريخ 15 أبريل 2022(2 سنوات، و1 شهر، و1 أسبوع، و5 أيام) الخسائر الإصابات جرح 152 مدني فلسطيني و3 من ضباط الش...

An approach to explaining social and cultural phenomena by studying their history This article is about philosophical theories known collectively as historicism. For the school of historiography, see Historism. For the school of art and architecture, see Historicism (art). For the method of interpreting the Book of Revelation, see Historicism (Christianity). For historicism in music, see Musical historicism.This article has multiple issues. Please help improve it or discuss these issues on th...

Конь Юлий и большие скачки Жанры приключения, комедия Техника анимации компьютерная рисованная анимация Режиссёры Дарина ШмидтКонстантин Феоктистов Авторы сценария Вадим СвешниковМаксим СвешниковАлександр Боярский Роли озвучивали см. в статье Композиторы Георгий Ж�...

Balaksuji di Rumah si Pitung di Marunda, Jakarta. Balaksuji adalah tangga yang berada di depan rumah panggung Betawi.[1] Di kalangan orang Sunda, tangga semacam ini dijuluki golodog.[2] Dalam kebudayaan Betawi, balaksuji dianggap memiliki unsur filosofis. Balaksuji diyakini menjadi sarana untuk menghalau bencana. Selain itu, sebelum memasuki rumah melalui balaksuji, orang Betawi harus menyucikan diri terlebih dahulu dengan membasuh kakinya. Tujuannya adalah agar sang pemilik s...

Principle of war This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Economy of force – news · newspapers · books · scholar · JSTOR (July 2008) (Learn how and when to remove this message) Part of a series onWarOutline History Prehistoric Ancient Post-classical castles Early modern pike and shot napoleonic Late m...

مجزرة كنصفرة وكفرعويد جزء من الحرب الأهلية السورية معلومات عامة التاريخ 19 ديسمبر - 20 ديسمبر 2011 الموقع منطقة جبل الزاوية، محافظة إدلب، سوريا النتيجة نتائج المجزرة: سقوط مئات القتلى من أهالي المنطقة على أيدي الجيش. توجه المجلس الوطني السوري بدعوة إلى مجلس الأمن الدولي لعقد �...

دار الكتب الوطنية (حلب) إحداثيات 36°12′13″N 37°09′10″E / 36.20361111°N 37.15277778°E / 36.20361111; 37.15277778 معلومات عامة الموقع حلب الدولة سوريا سنة التأسيس 1924 النوع مكتبة وطنية معلومات أخرى تعديل مصدري - تعديل دار الكتب الوطنية بحلب دار الكتب الوطنية أو المكت...