Quadrupole magnet
|
Read other articles:

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada September 2016. Nahir Oyal Informasi pribadiTanggal lahir 17 Desember 1990 (umur 33)Tempat lahir Swedia, SödertäljeTinggi 175 m (574 ft 2 in)Posisi bermain MidfielderInformasi klubKlub saat ini Syrianska FC (on loan from Djurgårdens IF)N...
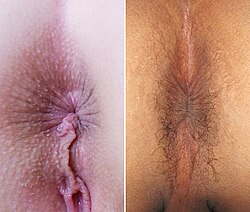
AnusAnus perempuan (kiri) dan laki-laki (kanan).PengidentifikasiTA98A05.7.05.013TA23022FMA15711Daftar istilah anatomi[sunting di Wikidata] Pada manusia, anus (dari Latin anus artinya cincin, lingkaran)[1][2] atau dubur adalah bagian pembukaan luar dari rektum. Sepasang otot sfingter mengontrol keluarnya feses dari tubuh selama buang air besar, yang merupakan fungsi utama anus. Otot-otot ini disebut sfingter anal internal dan sfingter anal eksternal, keduanya berupa otot me...

Courts adalah sebuah perusahaan ritel furnitur dan elektronik, didirikan awalnya pada 1850 di Britania Raya oleh William Henry Court dan Albert Court di Canterbury sebagai Courts Bros.[1][2] Perusahaan ini kemudian berpindah tangan pada tahun 1945 ke Cohen bersaudara, yang kemudian mulai memperluas usahanya menjadi 34 toko di Inggris pada 1959 dan masuk ke Bursa Saham London (LSE) pada tahun tersebut. Dalam perkembangannya, Courts plc kemudian mulai berekspansi ke berbagai neg...

Species of bird Black-capped bulbul Conservation status Least Concern (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Aves Order: Passeriformes Family: Pycnonotidae Genus: Rubigula Species: R. melanictera Binomial name Rubigula melanictera(Gmelin, JF, 1789) Synonyms Muscicapa melanictera J. F. Gmelin, 1789 Pycnonotus melanicterus Rubigula melanictera The black-capped bulbul (Rubigula melanictera), or black-headed yellow bul...

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...

لمعانٍ أخرى، طالع جورج مولر (توضيح). جورج مولر معلومات شخصية اسم الولادة (بالإنجليزية: George Edwin Mueller) الميلاد 16 يوليو 1918[1] سانت لويس الوفاة 12 أكتوبر 2015 (97 سنة) [2][1] إرفاين مواطنة الولايات المتحدة عضو في الأكاديمية الوطنية للهندسة ا...

1980 Japanese science fiction film VirusOriginal title 復活の日 Directed byKinji FukasakuScreenplay by Koji Takada Gregory Knapp Kinji Fukasaku[1] Based onFukkatsu no hiby Sakyo KomatsuProduced byHaruki Kadokawa[1]Starring Masao Kusakari Sonny Chiba George Kennedy Robert Vaughn Olivia Hussey Glenn Ford Chuck Connors Henry Silva CinematographyDaisaku Kimura[1]Edited byAkira Suzuki[1]Music byKentarō Haneda[1]ProductioncompanyHaruki Kadokawa Office ...

この記事は検証可能な参考文献や出典が全く示されていないか、不十分です。出典を追加して記事の信頼性向上にご協力ください。(このテンプレートの使い方)出典検索?: コルク – ニュース · 書籍 · スカラー · CiNii · J-STAGE · NDL · dlib.jp · ジャパンサーチ · TWL(2017年4月) コルクを打ち抜いて作った瓶の栓 コルク(木栓、�...

هذه المقالة عن المجموعة العرقية الأتراك وليس عن من يحملون جنسية الجمهورية التركية أتراكTürkler (بالتركية) التعداد الكليالتعداد 70~83 مليون نسمةمناطق الوجود المميزةالبلد القائمة ... تركياألمانياسورياالعراقبلغارياالولايات المتحدةفرنساالمملكة المتحدةهولنداالنمساأسترالي�...

Charles Hermite intorno al 1887. Charles Hermite (Dieuze, 24 dicembre 1822 – Parigi, 14 gennaio 1901) è stato un matematico francese che diede rilevanti contributi a campi quali teoria dei numeri, forme quadratiche, teoria degli invarianti, polinomi ortogonali, funzioni ellittiche e algebra. Egli fu il primo a dimostrare che la costante e {\displaystyle e} , la base dei logaritmi naturali, è un numero trascendente. I suoi metodi furono usati successivamente da Ferdinand von Lindemann per ...

In this Spanish name, the first or paternal surname is Durán and the second or maternal family name is Cartín. Costa Rican doctor and politician Dr. Carlos DuránActing President of Costa RicaIn office7 November 1889 – May 8, 1890Preceded byBernardo SotoSucceeded byJosé Joaquín Rodríguez Personal detailsBorn12 November 1852San JoséDied23 November 1924 (aged 72)San José Carlos Durán Cartín (12 November 1852 – 23 November 1924), a doctor of medicine who had trained...
This is a list of Japanese television series. The programs are listed alphabetically and are followed by the genre of the show and the date of the original run. For a chronological list, see List of Japanese television programs by date. Contents Top !$@ 0–9 A B C D E F G H I J K L M N O P Q R S T U V W X Y Z See also 0–9 009-1 - Anime, 2006 8 Man - Anime, 1963-1964 A Abarenbō Shōgun - Jidaigeki, 1978-2003, 2004, 2008 Ai no Gakko Cuore Monogatari - Anime, 1981 Aikurushii - Drama, 20...

概要 この画像投稿者(もしくは関係する他の編集者)は、コモンズ移行後の即時削除を望んでいません。 この画像またはメディアファイルは、ウィキメディア・コモンズでFile:川井稲荷山古墳出土 三角縁神獣鏡.JPGとして入手できる場合があります。このファイルのライセンスはウィキメディア・コモンズに準拠している可能性がありますが、編集者はローカルコピー�...

This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Human rights in Africa – news · newspapers · books · scholar · JSTOR (April 2009) (Learn how and when to re...

Questa voce sull'argomento centri abitati del Goiás è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Carmo do Rio Verdecomune LocalizzazioneStato Brasile Stato federato Goiás MesoregioneCentro Goiano MicroregioneCeres AmministrazioneSindacoDelson José Santos TerritorioCoordinate15°21′15″S 49°42′25″W15°21′15″S, 49°42′25″W (Carmo do Rio Verde) Altitudine629 e 598 m s.l.m. Superficie418,544 km² Abitanti8 ...

River located in and along the countries of Iran, Armenia, Azerbaijan, and Turkey Araks redirects here. For other uses, see Araks (disambiguation). Arax redirects here. For other uses, see Arax (disambiguation). Araz redirects here. For other uses, see Araz, Azerbaijan and Araz (given name). ArasArmenian: Արաքս, Azerbaijani: Araz, Persian: ارس, Turkish: ArasThe Aras with Nakhchivan (Azerbaijan) to the right and Iran to the leftAras River highlighted on a map of the Kura River watershe...

British Army general and anti-personnel-munition innovator Henry ShrapnelPortrait of Shrapnel, 1817Born(1761-06-03)3 June 1761Bradford on Avon, WiltshireDied13 March 1842(1842-03-13) (aged 80)SouthamptonAllegianceUnited KingdomService/branchBritish ArmyYears of service1779–1825RankLieutenant-generalUnitRoyal ArtilleryBattles/wars American Revolutionary War French Revolutionary Wars Flanders Campaign (WIA) Napoleonic Wars Lieutenant-General Henry Scrope Shrapnel (3 June 1761 �...

一般県道 埼玉県道385号武蔵丘陵森林公園広瀬線 路線延長 4,857m 起点 熊谷市万吉 終点 熊谷市熊谷運動公園前交差点 接続する主な道路(記法) 国道140号 ■テンプレート(■ノート ■使い方) ■PJ道路 熊谷市広瀬付近 埼玉県道385号武蔵丘陵森林公園広瀬線(さいたまけんどう385ごう むさしきゅうりょうしんりんこうえんひろせせん)は、埼玉県熊谷市万吉の埼玉県道17...

Although prototype diesel locomotives ran in Britain before World War II, the railways of both the Republic and Northern Ireland changed over much more rapidly from steam to diesel traction than those in Britain, due to the island's limited coal reserves and (in the Republic) an ageing steam locomotive fleet. Northern Ireland operated several diesel shunters as early as the 1930s. CIÉ's first diesels consisted of five shunters built by CIÉ in 1947/48. The initial two diesel mainline locomo...

Village in Mozhaysky District, Moscow Oblast, Russia For other places with the same name, see Borodino. Village in RussiaBorodinoVillageBorodinoShow map of Moscow OblastBorodinoShow map of RussiaCoordinates: 55°32′N 35°49′E / 55.533°N 35.817°E / 55.533; 35.817CountryRussia The main monument to the Battle of Borodino The Kutuzov Obelisk in Borodino The Flag of Borodinskoye Rural Settlement, of which the village of Borodino is the administrative centre The Borodi...
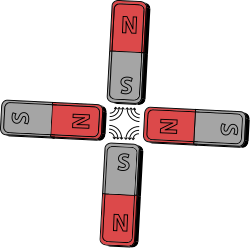






![{\displaystyle [B\rho ]=p/q}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d03a61c7a9087342f07acfa278294ddd1a9b1d02)


![{\displaystyle \kappa ={\frac {G}{[B\rho ]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/008271e0dcb6b947bec953a9e60043c747f2fe55)




