Polar space
|
Read other articles:

Nama ini menggunakan cara penamaan Portugis. Nama keluarga pertama atau maternalnya adalah Palmeira dan nama keluarga kedua atau paternalnya adalah de Paula. Marcos PalmeiraPalmeira in 2007Lahir19 Agustus 1963 (umur 60)Rio de Janeiro, BrasilPekerjaanPemeran, pembawa acara televisi, produserTahun aktif1968–sekarangSuami/istri Vanessa Barum (m. 1993; c. 1998) Amora Mautner (m. 2005; c. 2...

Penghargaan Filmfare untuk Debut Laki-Laki TerbaikDeskripsiPenampilan Debut Terbaik oleh seorang AktorNegaraIndiaDipersembahkan olehFilmfareDiberikan perdana1989Pemegang gelar saat iniSooraj Pancholi, Hero (2016)Situs webfilmfare.comsssss Penghargaan Filmfare untuk Debut Laki-Laki Terbaik diberikan di Penghargaan Filmfare tahunan untuk film-film Hindi untuk menghargai sebuah penampilan yang ditampilkan oleh seorang laki-laki. Penghargaan tersebut dan Penghargaan Filmfare untuk Debut Perempuan...

Le symbole de risques des rayonnements ionisants Tableau périodique avec les éléments colorés selon la demi-vie de leur isotope le plus stable. Éléments qui contiennent au moins un isotope stable. Éléments radioactifs : l'isotope le plus stable possède une demi-vie de plus de 4 millions d'années. Eléments radioactifs : l'isotope le plus stable possède une demi-vie de 800 à 34000 ans/ Éléments radioactifs : l'isotope le plus stable possède une demi-vie de 1 jour ...

Big BangPoster film Korea SelatanSutradaraJeong-woo ParkDitulis olehJeong-woo ParkPemeranHang-Seon JangWoo-seong KamSeong-jin KangSu-ro KimJung-Hee MooDistributorSio Film and Bravo EntertainmentTanggal rilisKorea Selatan14 Maret 2007Durasi118 menitNegaraBahasaKorea Big Bang (bahasa Korea: Ssonda) adalah film Korea Selatan yang disutradarai oleh Jeong-woo Park. Film ini dirilis pada tanggal 14 Maret 2007. Pranala luar Ssonda di IMDb (dalam bahasa Inggris) [1] Artikel bertopik film Kor...

† Человек прямоходящий Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:Синапсиды�...
Skip to table of contents This is the talk page for discussing WikiProject Television and anything related to its purposes and tasks. Put new text under old text. Click here to start a new topic. New to Wikipedia? Welcome! Learn to edit; get help. Assume good faith Be polite and avoid personal attacks Be welcoming to newcomers Seek dispute resolution if needed ShortcutsWT:TVWT:WPTV Archives: Index, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, ...

River in New York, United StatesSchoharie CreekSchoharie Creek flowing into the Mohawk River, from Tribes Hill Park in Amsterdam, New York.Map of the Schoharie Creek drainage basinLocationCountryUnited StatesStateNew YorkPhysical characteristicsSourceIndian Head Mountain MouthMohawk River • locationFort Hunter • coordinates42°56′28″N 74°17′32″W / 42.94111°N 74.29222°W / 42.94111; -74.29222 • elevation274&...

Public policy school at the University of Georgia School of Public and International AffairsThe seal of the School of Public and International Affairs at the University of GeorgiaTypeUniversity SchoolEstablished2001Academic affiliationTPCDeanMatthew R. AuerLocationAthens, Georgia, USAWebsitespia.uga.edu The School of Public and International Affairs, also referred to as SPIA, is a political science, international affairs and public policy school within The University of Georgia (UGA) in Athen...

Alberto La Volpe Deputato della Repubblica ItalianaDurata mandato1994 –1996 LegislaturaXII GruppoparlamentareGruppo misto (21 aprile 1994-16 maggio 1994)Alleanza dei Progressisti (16 maggio 1994-21 febbraio 1995)Patto dei Democratici (21 febbraio 1995-8 maggio 1996) Circoscrizione3-Sulmona Sito istituzionale Dati generaliPartito politicoPSDIPSI (fino al 1994)Socialisti Italiani (1994-1998)Socialisti Democratici Italiani (1998-2007) Titolo di studioLaurea in Giur...

† Палеопропитеки Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:СинапсидыКласс:�...

Mountains in South Island, New Zealand Dunstan MountainsThe Dunstan Mountains as viewed from the Lowburn SugarloafHighest pointPeakDunstanElevation1,667 m (5,469 ft)[1]Coordinates44°52′S 169°35′E / 44.867°S 169.583°E / -44.867; 169.58345°02′56″S 169°22′34″E / 45.049°S 169.376°E / -45.049; 169.376DimensionsLength51 km (32 mi) 45°Width19 km (12 mi) 135°Area826 km2 (319&#...
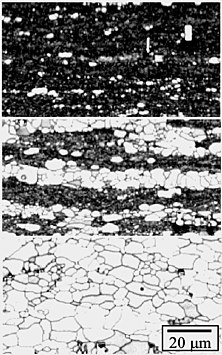
Process of forming new, defect-free crystal grains within a material CrystallizationFundamentals Crystal Crystal structure Nucleation Concepts Crystallization Crystal growth Recrystallization Seed crystal Protocrystalline Single crystal Methods and technology Boules Bridgman–Stockbarger method Van Arkel–de Boer process Czochralski method Epitaxy Flux method Fractional crystallization Fractional freezing Hydrothermal synthesis Kyropoulos method Laser-heat...

1979 film by Peter Yates For other uses, see Breaking Away (disambiguation). Breaking AwayTheatrical release posterDirected byPeter YatesWritten bySteve TesichProduced byPeter YatesStarringDennis ChristopherDennis QuaidDaniel SternJackie Earle HaleyBarbara BarriePaul DooleyRobyn DouglassCinematographyMatthew F. LeonettiEdited byCynthia ScheiderMusic byPatrick WilliamsDistributed by20th Century-FoxRelease date July 13, 1979 (1979-07-13) Running time101 minutes[1]CountryU...

American engineer (1944–2023) Michael HorodniceanuHorodniceanu in 2013BornMihai Horodniceanu(1944-08-04)August 4, 1944Bucharest, RomaniaDiedJune 22, 2023(2023-06-22) (aged 78)Forest Hills, New York, U.S.NationalityRomanianAmericanOccupationCivil Engineer 2013, discussing the Second Avenue Subway 2015, 34 St-Hudson Yards Opening Michael Horodniceanu (born Mihai Horodniceanu; August 4, 1944 – June 22, 2023) was a Romanian-born American civil engineer who served as traffic commissioner ...

Lebong AtasKecamatanNegara IndonesiaProvinsiBengkuluKabupatenLebongPemerintahan • CamatTuti Maryani, MM,M.SiPopulasi • Total- jiwaKode Kemendagri17.07.02 Kode BPS1707040 Desa/kelurahan6 desa Lebong Atas adalah sebuah kecamatan di Kabupaten Lebong, Bengkulu, Indonesia. Kantor camat Lebong Atas lbsKecamatan Lebong Atas, Kabupaten Lebong, BengkuluDesa Blau Daneu Sukau Kayo Tabeak Blau Tabeak Blau I Tik Tebing lbsKabupaten Lebong, Bengkulu Bupati: Kopli Ansori Wakil B...

بطولة العالم لسباق الدراجات على الطريق القميص القزحي الذي يحمله بطل العالم خلال الموسم الموالي معلومات عامة الرياضة سباق الدراجات على الطريق انطلقت 1927 المنظم الاتحاد الدولي لسباق الدراجات (UCI) التواتر كل سنة في شهر سبتمبر الموقع الرسمي www.uci.ch قائمة الفائزين آخر بطل إيط...

Los Angeles-class nuclear-powered attack submarine of the US Navy For other ships with the same name, see USS Augusta. USS Augusta (SSN-710) History United States NamesakeAugusta, Maine Awarded10 December 1973 BuilderGeneral Dynamics Corporation Laid down1 April 1983 Launched21 January 1984 Acquired5 December 1984 Commissioned19 January 1985 Decommissioned11 February 2009 Stricken11 February 2009 HomeportGroton, Connecticut MottoProtecting The Frontier Since 1754 Any Mission, Any Time Nicknam...

English-language profanity For other uses, see Fuck (disambiguation). A protester's sign using the word fuck on Tax March Day, April 15, 2017 in Washington, D.C. U.S. Fuck is an English-language profanity which often refers to the act of sexual intercourse, but is also commonly used as an intensifier or to convey disdain. While its origin is obscure, it is usually considered to be first attested to around 1475.[1] In modern usage, the term fuck and its derivatives (such as fucker and ...

Disambiguazione – Se stai cercando altri significati, vedi Adam West (disambigua). Adam West interpreta Batman nel 1966 Adam West, nato William West Anderson (Walla Walla, 19 settembre 1928 – Los Angeles, 9 giugno 2017), è stato un attore e doppiatore statunitense, noto per aver interpretato il ruolo di Batman nell'omonima serie televisiva negli anni sessanta e per aver preso parte alla serie I Griffin. Indice 1 Biografia 1.1 Primi ruoli 1.2 Anni sessanta 1.2.1 Batman 1.3 Carriera succe...

Theory that seeks to organize subjective feelings into discrete categories Affect theory is a theory that seeks to organize affects, sometimes used interchangeably with emotions or subjectively experienced feelings, into discrete categories and to typify their physiological, social, interpersonal, and internalized manifestations. The conversation about affect theory has been taken up in psychology, psychoanalysis, neuroscience, medicine, interpersonal communication, literary theory, critical ...































