Matt Bianco
| |||||||||||||||||||||||||||
Read other articles:

Jutta WachowiakLahir13 Desember 1940 (umur 83)Berlin, JermanPekerjaanPemeranTahun aktif1962 – kini Jutta Wachowiak (lahir 13 Desember 1940) adalah seorang pemeran asal Jerman. Ia tampil dalam lebih dari 60 film dan acara televisi sejak tahun 1962. Ia membintangi film tahun 1986 So Many Dreams, yang masuk dalam Festival Film Internasional Berlin ke-37.[1] Filmografi pilihan Follow Me, Scoundrels (1964) KLK Calling PTZ - The Red Orchestra (1971) The Fiancee (1980) So Many D...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Oktober 2022. HMS Virulent adalah kapal selam kelas V milik Angkatan Laut Britania Raya. Kapal ini dibangun pada masa Perang Dunia Kedua sebagai bagian dari gelombang kedua produksi kapal selam kelas V yang dipesan pada 21 Mei 1942 sejumlah 18 kapal. Kapal ini diban...

Town in VirginiaOccoquan, VirginiaTownTown of OccoquanMill Street, the center of Occoquan's historic and commercial districtLocation in Prince William County and the state of Virginia.Coordinates: 38°40′58″N 77°15′39″W / 38.68278°N 77.26083°W / 38.68278; -77.26083Country United StatesState VirginiaCountyPrince William[1]Area[2] • Total0.22 sq mi (0.57 km2) • Land0.17 sq mi (0.45 ...

2002 novel by Steve Lyons The Crooked World AuthorSteve LyonsSeriesDoctor Who book:Eighth Doctor AdventuresRelease number57SubjectFeaturing:Eighth DoctorFitz and AnjiPublisherBBC BooksPublication dateJune 2002Pages288ISBN0-563-53856-2Preceded byThe Book of the Still Followed byHistory 101 The Crooked World is a BBC Books original novel written by Steve Lyons and based on the long-running British science fiction television series Doctor Who.[1] It features the Eigh...

Paul Lambert Lambert melatih Aston Villa pada 2013Informasi pribadiNama lengkap Paul Lambert[1]Tanggal lahir 7 Agustus 1969 (umur 54)Tempat lahir Linwood, Renfrewshire, SkotlandiaTinggi 180 cm (5 ft 11 in)[2]Posisi bermain GelandangKarier senior*Tahun Tim Tampil (Gol)1986–1993 St. Mirren 227 (14)1993–1996 Motherwell 103 (6)1996–1997 Borussia Dortmund 44 (1)1997–2005 Celtic 193 (14)2005–2006 Livingston 7 (0)Total 574 (35)Tim nasional1990 Skotlandi...

Pour les articles homonymes, voir Brionnais (homonymie). Brionnais Châteauneuf, village du Brionnais. Pays France Région française BourgogneRhône-Alpes Département français Saône-et-LoireLoire Villes principales Semur-en-BrionnaisMarcignyCharlieuLa ClayetteChauffailles Coordonnées 46° 15′ 53″ nord, 4° 05′ 36″ est Superficie approximative 800 km2 Relief collines, de 250 à 800 m d'altitude Production élevage de bovins (charolais) Régions ...

Liga Super EropaMulai digelar18 April 2021; 2 tahun lalu (2021-04-18)WilayahEropaJumlah tim80SemboyanThe Best Clubs,The Best Players Every WeekSitus webSitus web resmi Liga Super Eropa (nama badan resmi: European Super League Company, S.L.), juga dikenal dengan nama The Super League atau European Super League, adalah sebuah bakal kompetisi sepak bola antarklub internasional yang mempertemukan klub-klub besar Eropa sebagai tandingan dari Liga Champions UEFA. Setelah beragam spekulasi terk...

Cimetière des Champeaux de MontmorencyPays FranceCommune MontmorencyMise en service XVIIe siècleCoordonnées 48° 59′ 38″ N, 2° 19′ 23″ EIdentifiantsFind a Grave 2581141Sauvons nos tombes 32159Localisation sur la carte d’Île-de-Francemodifier - modifier le code - modifier Wikidata Le cimetière des Champeaux, créé au début du XVIIe siècle à Montmorency (Val-d'Oise), a la particularité d'être le plus grand cimetière polonais de Fran...

Voce principale: Torino Football Club. Torino FCStagione 2006-2007Sport calcio Squadra Torino Allenatore Alberto Zaccheroni Gianni De Biasi[1] Presidente Urbano Cairo Serie A16º Coppa ItaliaSecondo turno Maggiori presenzeCampionato: Abbiati (36)Totale: Abbiati (38) Miglior marcatoreCampionato: Rosina (9)Totale: Rosina (12) StadioOlimpico (25.500) Abbonati17 489[2] Maggior numero di spettatori23 695 vs Inter (13 gennaio 2007)[2] Minor numero di spettato...

Kingdom مملكة كاباون دويلة ناشئة بعد المملكة المورية الرومانية Rump state, exclave 578–708 مملكة كاباون (8) وغيرها من الممالك البربرية في أواخر القرن السادس. نظام الحكم ملكية الديانة مسيحية (الكنيسة الرومانية الكاثوليكية) الملك (غير معروف) 578-708 التاريخ الفترة التاريخية العصور الوسطى إنه�...

Voce principale: Promozione 1983-1984. Promozione Lombarda 1983-1984 Competizione Promozione Sport Calcio Edizione 28ª Organizzatore FIGC - LNDComitato Regionale Lombardo Luogo Italia Partecipanti 64 Formula 4 gironi all'italiana Cronologia della competizione 1982-1983 1984-1985 Manuale Nella stagione 1983-1984 la Promozione era il sesto livello del calcio italiano (il massimo livello regionale). Qui vi sono le statistiche relative al campionato in Lombardia e nella provincia di Piace...

† Человек прямоходящий Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:ЧелюстноротыеНадкласс:ЧетвероногиеКлада:АмниотыКлада:Синапсиды�...

Children's animated television series Tumble LeafGenreAnimationCartoon seriesChildren's animationEducationalFantasyStop motionCreated byDrew HodgesVoices ofChristopher DownsBrooke WolloffZak McDowellAlex TrugmanCountry of originUnited StatesOriginal languageEnglishNo. of seasons4No. of episodes52 (101 segments)ProductionExecutive producersKelli Bixler, Drew HodgesRunning time24 minutesProduction companiesAmazon StudiosBix Pix Entertainment[1]Original releaseNetworkAmazon VideoReleaseM...

У этого термина существуют и другие значения, см. Горностай (значения). Горностай Научная классификация Домен:ЭукариотыЦарство:ЖивотныеПодцарство:ЭуметазоиБез ранга:Двусторонне-симметричныеБез ранга:ВторичноротыеТип:ХордовыеПодтип:ПозвоночныеИнфратип:Челюстнороты...

Part of a series onFrancoismEagle of Saint John Organizations Movimiento Nacional FET y de las JONS Consejo del Reino Organización Sindical Española Frente de Juventudes Sección Femenina Tribunal de Orden Público Instituto Nacional de Colonización Instituto Nacional de Industria Fuerzas Armadas de España Cortes Españolas Sindicato Español Universitario Servicio Exterior de Falange History Spanish coup of July 1936 Spanish Civil War White Terror Francoist concentration camps Spain dur...

Indigenous ethnic group of eastern North America This article is about the people. For other uses, see Mi'kmaq (disambiguation). Ethnic group Mi'kmaqLnuGrand Council Flag of the Miꞌkmaq Nation.[1][2] Although the flag is meant to be displayed hanging vertically as shown here, it is quite commonly flown horizontally, with the star near the upper hoist.A Miꞌkmaw father and child at Tufts Cove, Nova Scotia, around 1871Total population66,748 registered members (2023)168,480 cl...

Museum in Sydney, Australia Colonial Museum redirects here. For New Zealand's Colonial Museum, see Dominion Museum building. This article is about the museum in Sydney. For the museum in Canberra, see National Museum of Australia. Australian MuseumThe William Street exterior and Crystal Hall entry to the Australian Museum in 2016Location within Sydney central business districtFormer nameColonial Museum;Sydney MuseumEstablished1827; 197 years ago (1827)Location1 William Stree...

Leonés LlionésHablado en España EspañaRegión León León ZamoraZamoraHablantes 25 000 (patrimoniales).[1][2]Puesto No está entre los 100 primeros (Ethnologue, 2013)Familia Indoeuropeo Itálico Grupo Romance Romance Italo-occidental Subgrupo Occidental Ibero-romance Ibero-occidental A...

也南新村华人新村Kampung Baru Jeram也南新村也南新村于马来西亚半岛和马来西亚的位置显示霹雳州的地图也南新村也南新村 (馬來西亞)显示馬來西亞的地图坐标:4°23′18″N 101°09′18″E / 4.38833°N 101.15500°E / 4.38833; 101.15500国家 马来西亚州属 霹靂县金宝县开埠[1]1949年政府 • 地方政府金宝县议会 • 市长凯鲁阿米尔(Khairul Amir) ...
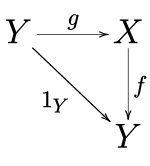
f {\displaystyle f} is a retraction of g {\displaystyle g} . g {\displaystyle g} is a section of f {\displaystyle f} . In category theory, a branch of mathematics, a section is a right inverse of some morphism. Dually, a retraction is a left inverse of some morphism. In other words, if f : X → Y {\displaystyle f:X\to Y} and g : Y → X {\displaystyle g:Y\to X} are morphisms whose composition f ∘ g : Y → Y {\displaystyle f\circ g:Y\to Y} is the identity morphism on Y...
