Feynman sprinkler
|
Read other articles:

Mycobacterium leprae Gambar Mycobacterium leprae dari lesi kulit. Klasifikasi ilmiah Kerajaan: Bacteria Filum: Actinobacteria Ordo: Actinomycetales Subordo: Corynebacterineae Famili: Mycobacteriaceae Genus: Mycobacterium Spesies: M. leprae Nama binomial Mycobacterium lepraeHansen, 1874 Mycobacterium leprae, juga disebut Basillus Hansen, adalah bakteri yang menyebabkan penyakit kusta (penyakit Hansen).[1] Bakteri ini merupakan bakteri intraselular.[2] M. leprae merupakan ...

Målselv kommune Málatvuomi suohkanMunisipalitasView of the mountain Istind on the Bardu-Målselv border Lambang kebesaranTroms di NorwayLetak Målselv di TromsNegara NorwegiaCountyTromsDistrictMidt-TromsAdministrative centreMoenPemerintahan • Mayor (2011)Helene Rognli (H)Luas • Total3.321,72 km2 (128,252 sq mi) • Luas daratan3.206,97 km2 (123,822 sq mi) • Luas perairan114,75 km2 (4,431 sq ...

Piala FA 1974–1975Negara Inggris WalesJuara bertahanLiverpoolJuaraWest Ham United(gelar ke-2)Tempat keduaFulham← 1973–1974 1975–1976 → Piala FA 1974–1975 adalah edisi ke-94 dari penyelenggaraan Piala FA, turnamen tertua dalam sepak bola di Inggris. Edisi ini dimenangkan oleh West Ham United setelah mengalahkan Fulham pada pertandingan final dengan skor 2–0. Final Artikel utama: Final Piala FA 1975 West Ham United v Fulham 3 Mei 1975 West Ham United 2–0 Fulham Laporan...

Voce principale: UEFA Champions League 2020-2021. Finale della UEFA Champions League 2020-2021Lo stadio do Dragão di Porto, teatro della finaleInformazioni generaliSport Calcio CompetizioneChampions League 2020-21 Data29 maggio 2021 CittàPorto ImpiantoStadio do Dragão Spettatori14 110[1] Dettagli dell'incontro Manchester City Chelsea 0 1 Arbitro Antonio Mateu Lahoz[2] MVP N'Golo Kanté Successione ← Finale della UEFA Champions League 2019-2020 F...
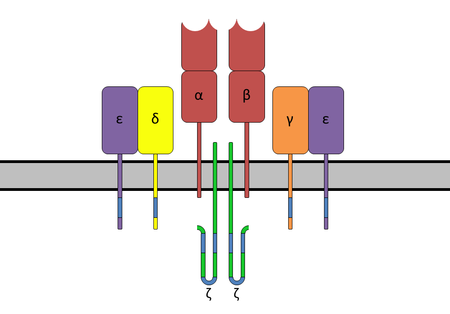
هذه مقالة غير مراجعة. ينبغي أن يزال هذا القالب بعد أن يراجعها محرر؛ إذا لزم الأمر فيجب أن توسم المقالة بقوالب الصيانة المناسبة. يمكن أيضاً تقديم طلب لمراجعة المقالة في الصفحة المخصصة لذلك. (أغسطس 2020) معزر التنشيط القائم على التيروزين (ITAM) هو تسلسل محفوظ لأربعة أحماض أمينية ت�...

Questa voce o sezione sull'argomento stadi di calcio d'Italia non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Gino Salveti Informazioni generaliStato Italia UbicazioneVia Appia Nuova,03043 Cassino (FR) Inizio lavori1963 Inaugurazione1967 Costo300.000.000 £ ProprietarioComune di Cassino ProgettoBruno Magrelli e Paolo Teresi Informazioni tecnichePost...

Paola ColonnaSignora consorte di PiombinoStemma In carica1399 – 1404 Predecessore(nuovo titolo) SuccessoreDonella Fieschi Signora reggente di PiombinoIn carica1404 – 1419(per il figlio Jacopo II Appiano) Signore di PiombinoIn carica27 dicembre 1441 – 30 novembre 1445 PredecessoreJacopo II Appiano SuccessoreCaterina Appiano con il marito Rinaldo Orsini NascitaGenazzano, 1380 MortePiombino, 30 novembre 1445 SepolturaDuomo di Piombino DinastiaColonna PadreAgapito Colonna MadreCaterin...

Part of a series on theAnthropology of kinship Basic concepts Family Lineage Affinity Consanguinity Marriage Incest taboo Endogamy Exogamy Moiety Monogamy Polygyny Polygamy Concubinage Polyandry Bride price Bride service Dowry Parallel / cross cousins Cousin marriage Levirate Sororate Posthumous marriage Joking relationship Clan Cohabitation Fictive / Milk / Nurture kinship Descent Cognatic / Bilateral Matrilateral Lineal Collateral House society Avunculate...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Biografi ini tidak memiliki referensi atau sumber sehingga isinya tidak dapat dipastikan. Bantu memperbaiki artikel ini dengan menambahkan sumber tepercaya. Materi kontroversial atau trivial yang sumbernya tidak memadai atau tidak bisa dipercaya harus segera dihapus.Cari sumber: Anwas – berita · surat kabar · buku · cendekiawan · JSTOR (Pelajari cara dan kapan saatnya untuk menghapus pesan templat ini) Untuk tokoh ini dalam sudut pandang Yahudi, lihat ...

ثنائي كرومات الصوديوم ثنائي كرومات الصوديوم الاسم النظامي (IUPAC) ثنائي كرومات صوديوم المعرفات رقم CAS 7789-12-0 بوب كيم 25408، و129628287 مواصفات الإدخال النصي المبسط للجزيئات [O-][Cr](=O)(=O)O[Cr](=O)(=O)[O-].[Na+].[Na+][1] المعرف الكيميائي الدولي InChI=1S/2Cr.2Na.7O/q;;2*+1;;;;;;2*-1[1] InChIKey:KIEOKOFEPABQKJ-U...

Islamic Development BankSingkatanIsDBTanggal pendirian1975 (1975)TipeBank pembangunanLokasiJeddah, Arab SaudiJumlah anggota 57 negaraTokoh pentingMuhammed Al-Jasser, PresidenJumlah Karyawan 932Situs webwww.isdb.org Islamic Development Bank (Bahasa Arab: البنك الإسلامي للتنمية) adalah sebuah lembaga keuangan pembangunan multilateral yang fokus menyediakan pembiayaan syariah untuk pembangunan infrastruktur. Bank ini berkantor pusat di Jeddah, Arab Saudi.[2] Terd...

Misi sui iuris Kepulauan Cayman (bahasa Latin: Missio sui iuris Insularum Caimanensium) adalah sebuah misi sui iuris Ritus Latin dari Gereja Katolik Roma di Karibia. Misi tersebut melingkupi seluruh dependensi Inggris Kepulauan Cayman, dan terdiri dari lima paroki, yang meliputi Santo Ignatius di George Town, Gereja Kristus Penebus di West Bay dan Gereja Stella Maris di Cayman Brac.[1] Pemimpin Kardinal Adam Joseph Maida (2000-2009) Allen Henry Vigneron (2009-sekarang) Referensi ...

رياضياتصنف فرعي من علوم شكلية، علوم دقيقةجزء من العلوم والتكنولوجيا والهندسة والرياضيات[1] — علوم شكلية[2] — علوم دقيقة[3][4] يمتهنه رياضياتيفروع رياضيات بحتة، رياضيات تطبيقيةالاستعمالات القائمة ... علم[5] — نمذجة رياضية[5] — هندسة — تمويل[6] �...

Family of carnivorous plants Lentibulariaceae Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Clade: Asterids Order: Lamiales Family: LentibulariaceaeRich.[1] Genera GenliseaPinguiculaUtricularia Lentibulariaceae is a family of carnivorous plants containing three genera: Genlisea, the corkscrew plants; Pinguicula, the butterworts; and Utricularia, the bladderworts. The genera Polypompholyx (two species of pink petticoats or fairy apro...

ماركسية لينينيةمعلومات عامةصنف فرعي من ماركسيةلينينية سُمِّي باسم كارل ماركسفلاديمير لينين لديه جزء أو أجزاء مادية جدليةمادية تاريخيةالاقتصاد الماركسيشيوعية علمية تعديل - تعديل مصدري - تعديل ويكي بيانات الماركسية اللينينية هي تيار إيديولوجي شيوعي برز كاتجاه سائد بين ا�...

«Genus» redirige aquí. Para otras acepciones, véase Genus (desambiguación). Dominio Reino Filo odivisión Clase Orden Familia Género Especie Categorías taxonómicas principales En taxonomía, el género es una categoría taxonómica que se ubica entre la familia y la especie; así, un género es un grupo de organismos que a su vez puede dividirse en varias especies (existen algunos géneros que son monoespecíficos, es decir, contienen una sola especie). El término proviene del latín...

Nicu PopescuPopescu di UNGA 2019 Wakil Perdana MenteriPetahanaMulai menjabat 6 Agustus 2021PresidenMaia SanduPerdana MenteriNatalia GavrilițaMenteri Urusan Luar Negeri dan Integrasi EropaPetahanaMulai menjabat 6 Agustus 2021PresidenMaia SanduPerdana MenteriNatalia GavrilițaPendahuluAureliu CiocoiPenggantiPetahanaMasa jabatan11 Juni 2019 – 14 November 2019PresidenIgor DodonPerdana MenteriMaia SanduPendahuluTudor UlianovschiPenggantiAureliu Ciocoi Informasi pribadiLahir25 A...

218th Brigade218th Independent Infantry Brigade (Home)281th Independent Infantry BrigadeFormation sign worn by 218th Independent Infantry Brigade.[1]Active1916–8 April 191821 October 1940-10 November 1942Country United KingdomBranch British ArmyTypeInfantry BrigadeRoleTraining and Home DefenceInsigniaBadge worn when in the Yorkshire County DivisionMilitary unit The 218th Brigade was a Home Service formation of the British Army during the First and the Second World Wars. First W...

What We Talk About When We Talk About Love Edisi IndonesiaPengarangRaymond CarverNegaraAmerika SerikatBahasaInggrisPenerbitKnopfTanggal terbit1981Jenis mediaPrintingHalaman176 What We Talk About When We Talk About Love adalah sebuah koleksi cerita pendek yang terbit tahun 1981 di Amerika Serikat. Penulis What We Talk About When We Talk About Love adalah Raymond Carver. Pemberian judul berasal dari salah satu cerita dalam koleksi ini.[1][2] Referensi ^ Books of The Ti...

