Dentatorubropallidoluysian atrophy
|
Read other articles:

Salto in altoIl salto a 2,09 m di Stefka Kostadinova nel 1987 a Roma, uno dei più longevi record dell'atletica femminileFederazioneWorld Athletics ContattoNo GenereMaschile e femminile Indoor/outdoorOutdoor e indoor Campo di giocoCampo Olimpicodal 1896 (uomini)dal 1928 (donne) Campione mondialeMaschile outdoor: Gianmarco Tamberi (2023)Femminile outdoor: Eleanor Patterson (2022)Maschile indoor: Woo Sang-hyeok (2022)Femminile indoor: Jaroslava Mahučich (2022) Campione olimpicoMaschile: M...

Private BodyguardGenre Drama Remaja BerdasarkanPrivate Bodyguardoleh Caroline PuspojudoDitulis oleh Lintang Pramudya Wardhani Angga Haryono Nuridzka Mutiaradini Seana Zavira SutradaraAsep KusdinarPemeran Sandrinna Michelle Junior Roberts Fattah Syach Harry Vaughan Arya Mohan Lagu pembukaKalimat Cinta oleh BiancadimasLagu penutupKalimat Cinta oleh BiancadimasPenata musikDonny AksonNegara asalIndonesiaBahasa asliBahasa IndonesiaJmlh. musim1Jmlh. episode10ProduksiProduser eksekutif David ...

American public servant (1878–1953) Gabe E. ParkerSuperintendent of the Five Civilized TribesIn office1915–1921Register of the Treasury of the United StatesIn office1913–1914Succeeded byHouston B. Teehee Personal detailsBornGabriel Edward Parker(1878-09-29)September 29, 1878Fort Towson, OklahomaDiedMay 8, 1953(1953-05-08) (aged 74)Oklahoma City, OklahomaEducationHenry Kendall CollegeSignature Gabriel Edward Parker[1] (September 29, 1878 – May 8, 1953) was an ...

Mathematical operation on vector spaces For generalizations of this concept, see Tensor product of modules and Tensor product (disambiguation). In mathematics, the tensor product V ⊗ W {\displaystyle V\otimes W} of two vector spaces V and W (over the same field) is a vector space to which is associated a bilinear map V × W → V ⊗ W {\displaystyle V\times W\rightarrow V\otimes W} that maps a pair ( v , w ) , v ∈ V , w ∈ W {\displaystyle (v,w),\ v...

Short HairAlbum mini karya AOADirilis19 Juni 2014Direkam2014GenreK-pop, electropop, dance-popDurasi24:08LabelFNC EntertainmentProduserBrave BrothersKronologi AOA Miniskirt(2014)Miniskirt2014 Short Hair(2014) Like a Cat(2014)Like a Cat2014 Singel dalam album Short Hair Short HairDirilis: 19 Juni 2014 Joa Yo!Dirilis: 24Juli 2014 Video musikShort Hair di YouTube Short Hair (Hangul: 단발머리; RR: Danbalmeori) adalah album mini pertama grup vokal wanita asal Korea Sel...

Filipino noodle soup La Paz batchoyA bowl of La Paz batchoyAlternative namesBa-chui (Chinese)Batsoy (Tagalog)Bachoy (Spanish)CourseSoupPlace of originPhilippinesRegion or stateLa Paz, Iloilo CityServing temperatureHotMain ingredientsnoodles, pork organs, vegetables, chicken, shrimp, beefVariationsBatchoy Tagalog, Bumbay Media: La Paz batchoy Batchoy, alternatively spelled batsoy, is a Filipino noodle soup of pork offal, crushed pork cracklings, chicken stock, beef loin, and round no...

2023 single by Thirty Seconds to MarsStuckSingle by Thirty Seconds to Marsfrom the album It's the End of the World but It's a Beautiful Day ReleasedMay 8, 2023 (2023-05-08)RecordedThe International Centre for the Advancement of the Arts and Sciences of Sound, Los Angeles, CaliforniaGenreElectropop[1]Length3:02LabelConcordSongwriter(s)Jared LetoProducer(s)Jared LetoShannon LetoThirty Seconds to Mars singles chronology Rescue Me (2018) Stuck (2023) Seasons (2023) Music vi...

WorkSingel oleh Rihanna featuring Drakedari album AntiBahasaBajan CreolePatwa JamaikaInggrisDirilis27 Januari 2016 (2016-01-27)DirekamDesember 2015GenreDancehallreggae-popR&BDurasi3:39LabelRoc NationWestbury RoadPenciptaJahron BrathwaiteMatthew SamuelsAllen RitterRupert Thomas Jr.Aubrey GrahamRobyn FentyMonte MoirProduserBoi-1daKronologi singel Rihanna American Oxygen (2015) Work (2016) Kiss It Better / Needed Me(2016) Kronologi singel Drake Jumpman(2015) Work(2016) Summer ...

Pour les articles homonymes, voir M1. Ligne M1 TSOL Bem 4/6 558 212-7 quittant la station de la Bourdonnette pour Malley. Réseau Métro de Lausanne Terminus Lausanne-Flon - Renens-Gare Communes desservies 4 (Lausanne, Chavannes-près-Renens, Écublens, Renens) Histoire Mise en service 2 juin 1991 Exploitant TL Infrastructure Conduite (système) Manuelle (Block automatique de ligne) Exploitation Matériel utilisé Bem 4/6 Be 4/6 Dépôt d’attache Garage-atelier d'Écublens Points d’arrê...
Post office in Tasmania, Australia North Hobart Post OfficeLocation412-414 Elizabeth Street, North Hobart, Tasmania, AustraliaCoordinates42°52′20″S 147°18′51″E / 42.8723°S 147.3142°E / -42.8723; 147.3142 Commonwealth Heritage ListOfficial nameNorth Hobart Post OfficeTypeListed place (Historic)Designated8 November 2011Reference no.105538 Tasmanian Heritage RegisterPlace ID137[1]StatusPermanently Registered Location of North Hobart Post Office in...

此条目序言章节没有充分总结全文内容要点。 (2019年3月21日)请考虑扩充序言,清晰概述条目所有重點。请在条目的讨论页讨论此问题。 哈萨克斯坦總統哈薩克總統旗現任Қасым-Жомарт Кемелұлы Тоқаев卡瑟姆若马尔特·托卡耶夫自2019年3月20日在任任期7年首任努尔苏丹·纳扎尔巴耶夫设立1990年4月24日(哈薩克蘇維埃社會主義共和國總統) 哈萨克斯坦 哈萨克斯坦政府...

Another Public EnemyPoster untuk Another Public EnemyNama lainHangul공공의 적2 Hanja公共의 敵2 Alih Aksara yang DisempurnakanGonggongui jeok 2McCune–ReischauerKonggongŭi chŏk 2 SutradaraKang Woo-sukProduserKang Woo-sukDitulis olehKim Hee-jaePemeranSol Kyung-guJung Joon-hoPenata musikHan Jae-kwonSinematograferKim Seong-bokPenyuntingKo Im-pyoDistributorCinema ServiceTanggal rilis 27 Januari 2005 (2005-01-27) Durasi148 menitNegaraKorea SelatanBahasaKoreaPendapatankot...
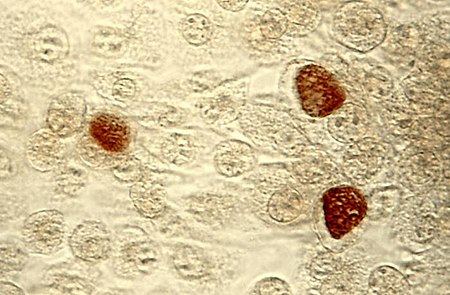
Chlamydia trachomatis C. trachomatis inclusion bodies (brown) in a McCoy cell culture. Klasifikasi ilmiah Kerajaan: Bacteria Filum: Chlamydiae Ordo: Chlamydiales Famili: Chlamydiaceae Genus: Chlamydia Spesies: C. trachomatis Nama binomial Chlamydia trachomatisBusacca, 1935 Chlamydia trachomatis adalah salah satu dari tiga spesies bakteri dalam genus Chlamydia, famili Chlamydiaceae, kelas Chlamydiae, filum Chlamydiae, domain Bacteria. C. trachomatis adalah agen chlamydial pertama yang di...

United States District Court for the Southern District of Texas(S.D. Tex.)LocationHoustonMore locationsBrownsvilleCorpus ChristiGalveston United States Post Office and Courthouse(Galveston)LaredoMcAllenVictoriaAppeals toFifth CircuitEstablishedMarch 11, 1902Judges19Chief JudgeRandy CraneOfficers of the courtU.S. AttorneyAlamdar S. HamdaniU.S. MarshalT. Michael O'Connorwww.txs.uscourts.gov The United States District Court for the Southern District of Texas (in case citatio...

This is a dynamic list and may never be able to satisfy particular standards for completeness. You can help by adding missing items with reliable sources. Lists of battles Before 301 301–1300 1301–1600 1601–1800 1801–1900 1901–2000 2001–current Naval Sieges See also Part of a series onWarOutline History Prehistoric Ancient Post-classical castles Early modern pike and shot napoleonic Late modern industrial fourth-gen Military Organization Command and control Defense ministry Army ...

Township in Essex County, New Jersey, US Township in New JerseyWest Orange, New JerseyTownshipGlenmont, part of the Thomas Edison National Historical Park located in Llewellyn Park FlagSealMotto: Where Invention Lives[1]Interactive map of West OrangeWest OrangeLocation in Essex CountyShow map of Essex County, New JerseyWest OrangeLocation in New JerseyShow map of New JerseyWest OrangeLocation in the United StatesShow map of the United StatesCoordinates: 40°47′09″N 74°15′5...

Este artículo o sección necesita referencias que aparezcan en una publicación acreditada. Busca fuentes: «Tipología lingüística» – noticias · libros · académico · imágenesEste aviso fue puesto el 9 de febrero de 2021. Sufijación y prefijación en las lenguas del mundo. La tipología lingüística se refiere a la clasificación de las lenguas desde el punto de vista de sus similitudes gramaticales. La clasificación tipológica contrasta con la clasificació...

Malcolm DelaneyMalcolm Delaney con l'Olimpia Milano nel 2021Nazionalità Stati Uniti Altezza191 cm Peso86 kg Pallacanestro RuoloPlaymaker Squadra Free agent CarrieraGiovanili Towson Catholic High School2007-2011 Virg. Tech Hokies Squadre di club 2011-2012 Élan Chalon29 (431)2012-2013 Budivelnyk Kiev52 (762)2013-2014 Bayern Monaco33 (341)2014-2016 Lokomotiv Kuban'54 (704)2016-2018 Atlanta Hawks127 (729)2018-2019 Guangdong S. Tigers37 (718)2019-2020 ...

Equivalence between strongly orientable graphs and bridgeless graphs This article is about Robbins' theorem in graph theory. For Robin's theorem in number theory, see divisor function. In graph theory, Robbins' theorem, named after Herbert Robbins (1939), states that the graphs that have strong orientations are exactly the 2-edge-connected graphs. That is, it is possible to choose a direction for each edge of an undirected graph G, turning it into a directed graph that has a path fro...

Railway line in Scotland, UK This article is about the Aberdeen–Edinburgh railway. For other uses of Aberdeen Line, see Aberdeen Line (disambiguation). For other uses of Aberdeen, see Aberdeen (disambiguation). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Edinburgh–Dundee line – news · newspapers · books · ...