Cramér's conjecture
|
Read other articles:

Jalan rayap bandar udara (warna biru) Pesawat F-22 di jalan rayap Pangkalan Udara Elmendorf, Alaska, Amerika Serikat. Landasan gelinding, jalan rayap, atau landas(an) ancang (Inggris: taxiwaycode: en is deprecated ) adalah jalan penghubung antara landasan pacu dengan pelataran pesawat (apron), kandang pesawat (hangar), terminal, atau fasilitas lainnya di sebuah bandar udara. Sebagian besar jalan rayap mempunyai permukaan keras yang merupakan lapisan aspal atau beton, walaupun bandar udara yan...

2013 American filmThe Marine 3: HomefrontMovie poster featuring Mike The Miz MizaninDirected byScott WiperWritten by Scott Wiper Declan O'Brien Produced byMichael LuisiStarring Mike The Miz Mizanin Neal McDonough Michael Eklund Ashley Bell Camille Sullivan Jared Keeso Jeff C. Ballard CinematographyRon StannettEdited byDallas PuettMusic byRobert RevellProductioncompaniesWWE Studios (Homefront Productions, Inc.)Distributed by20th Century Fox Home EntertainmentRelease date March 5, 201...

Voce principale: Associazione Sportiva Dilettantistica Vis Pesaro 1898. Vis Pesaro 1898Stagione 2003-2004Sport calcio Squadra Vis Pesaro Allenatore Mirco Fabbri poi Pieraldo Nemo poi Mirco Fabbri Presidente Gian Luca Bruscoli Serie C115º posto nel girone B. Maggiori presenzeCampionato: Giraldi (33) Miglior marcatoreCampionato: Borneo (11) 2002-2003 2004-2005 Si invita a seguire il modello di voce Questa pagina raccoglie le informazioni riguardanti la Vis Pesaro 1898 nelle competizioni ...
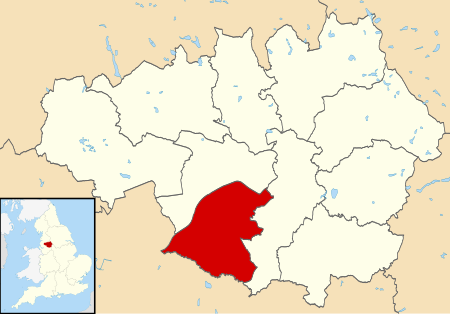
لمعانٍ أخرى، طالع ترافورد (توضيح). ترافورد قصبة حضرية ترافورد تاون هول الشعار الرسمي ل Traffordشعار النبالة الشعار: Hold Fast That Which Is Good [تمسك بما هو جيد] الاسم الرسمي Trafford يظهر ترافورد في داخل مانشستر الكبرى الإحداثيات 53°26′00″N 2°18′00″W / 53.433333333333°N 2.3°W / 53.433333333333; -...

2011 2021 Élections départementales de 2015 en Charente 38 sièges au sein du conseil départemental les 22 et 29 mars 2015 Type d’élection Élections départementales Campagne Du 9 mars 2015 au 21 mars 2015 Du 23 mars 2015 au 28 mars 2015 Corps électoral et résultats Population 353 657 Inscrits au 1er tour 259 630 Votants au 1er tour 130 388 50,22 % Votes exprimés au 1er tour 122 279 Votes blancs au 1er tour 4 932 Votes nuls au 1er tour 3&...

Brandon TransitParentCity of BrandonFoundedBrandon Municipal Rwy. (1913; 111 years ago (1913))Brandon Transit (1955; 69 years ago (1955))Headquarters900 Richmond Avenue E.LocaleBrandon, MBService areaUrban AreaService typebus service, paratransitRoutes10Hubs2Fleet23Annual ridership1,000,000[1]Fuel typeDieselWebsitebrandontransit.ca Brandon Transit is the municipally operated bus service in Brandon; the second largest city in the province of Manitoba...

Voce principale: Associazione Calcio Sampierdarenese. Società Ginnastica SampierdareneseStagione 1923-1924Una formazione della Sampierdarenese, 27 aprile 1924. Sport calcio SquadraGinnastica Sampierdarenese Allenatore Karl Rumbold Presidente Enrico De Amicis Prima Divisione9º posto nel girone A della Lega Nord. Maggiori presenzeCampionato: Cambiaso, Carzino I (22) Miglior marcatoreCampionato: Derchi, Carzino II (4) StadioStadio di Villa Scassi 1922-1923 1924-1925 Si invita a seguire il mod...

For the post-Roman and medieval petty kingdom, see Kingdom of Dyfed. Preserved county of Wales DyfedDyfed shown as a preserved county since 2003Area • 19745765.75 km2 • 19965765.75 km2Ranked 1st Population • 1971316,369 • 1992351,100 (estimate) • 2007375,200 (estimate)[1] Ranked 5th History • OriginKingdom of Dyfed • Created1974 • Abolished1996 • Succeeded byCarma...

ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Раннее христианство Гностическое христианство Вселенские соборы Н...

Dalam nama yang mengikuti kebiasaan penamaan Slavia Timur ini, patronimiknya adalah Platonovna. Tamara KarsavinaTamara Karsavina ((Katalan) 1912)LahirTamara Platonovna Karsavina(1885-03-10)10 Maret 1885Sankt-Peterburg, Kekaisaran RusiaMeninggal26 Mei 1978(1978-05-26) (umur 93)Beaconsfield, Buckinghamshire, InggrisPekerjaanBallet dancerSuami/istriVasili Vasilievich Mukhin (m. 1907; bercerai 1917)Henry James Bruce (...

American politician For his son, the North Dakota state senator, see George B. Sinner. George Sinner29th Governor of North DakotaIn officeJanuary 1, 1985 – December 15, 1992LieutenantRuth MeiersLloyd OmdahlPreceded byAllen I. OlsonSucceeded byEd SchaferMember of the North Dakota SenateIn office1962–1966 Personal detailsBornGeorge Albert Sinner(1928-05-29)May 29, 1928Fargo, North Dakota, U.S.DiedMarch 9, 2018(2018-03-09) (aged 89)Fargo, North Dakota, U.S.Political partyDemocr...

「俄亥俄」重定向至此。关于其他用法,请见「俄亥俄 (消歧义)」。 俄亥俄州 美國联邦州State of Ohio 州旗州徽綽號:七葉果之州地图中高亮部分为俄亥俄州坐标:38°27'N-41°58'N, 80°32'W-84°49'W国家 美國加入聯邦1803年3月1日,在1953年8月7日追溯頒定(第17个加入联邦)首府哥倫布(及最大城市)政府 • 州长(英语:List of Governors of {{{Name}}}]]) •&...

Ferrari F138KategoriFormula SatuKonstruktorFerrariPerancangPat Fry (Direktur Teknis)Nikolas Tombazis (Kepala Desainer) Tiziano Battistini (Kepala Desain Sasis) Simone Resta (Pemimpin Proyek) Nick Collett (Kepala R&D)Giacomo Tortora (Kepala Dinamika Kendaraan)Loic Bigois (Kepala Aerodinamika) Nicolas Hennel (Kepala Ahli Aerodinamika)Luca Marmorini (Mesin dan Elektronik)PendahuluFerrari F2012PenerusFerrari F14 TSpesifikasi teknis,[1][2]SasisCarbon fibre and honeycomb composi...

Major League Baseball award This article is about Major League Baseball's championship trophy. For the award known as the Commissioner's Trophy from 1970 to 1984, see Major League Baseball All-Star Game MVP Award. For a list of Commissioner's Trophy winners, see List of World Series champions. For the similarly named historical award, see Commissioner's Historic Achievement Award. Part of a series on theMajor League Baseball postseason Wild Card Series ALWCS NLWCS Division Series ALDS NLDS L...

密西西比州 哥伦布城市綽號:Possum Town哥伦布位于密西西比州的位置坐标:33°30′06″N 88°24′54″W / 33.501666666667°N 88.415°W / 33.501666666667; -88.415国家 美國州密西西比州县朗兹县始建于1821年政府 • 市长罗伯特·史密斯 (民主党)面积 • 总计22.3 平方英里(57.8 平方公里) • 陸地21.4 平方英里(55.5 平方公里) • ...
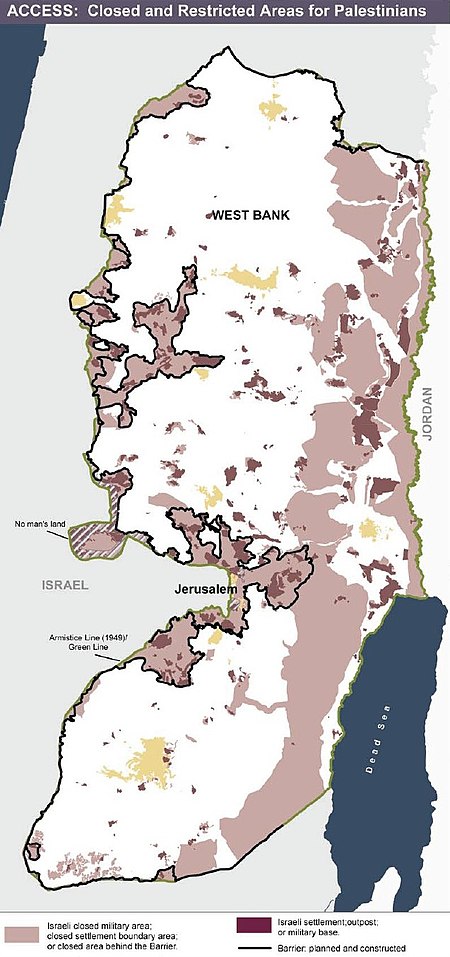
Attacks targeting Palestinians in the West Bank Swedish volunteer Tove Johannson (pictured) suffered a broken cheekbone from a hit in the face with a bottle by an Israeli settler in Hebron on November 18, 2006. She and other European members of the International Solidarity Movement sought to escort Palestinian children home from school.[1][2] Palestinians are the target of violence by Israeli settlers and their supporters, predominantly in the West Bank. In November 2021, Isra...

Germanium tetrachloride Germanium tetrachloride - structural formula Germanium tetrachloride - space-filling model Names IUPAC names Germanium tetrachlorideTetrachlorogermaneTetrachloridogermanium Other names Germanium(IV) chlorideNeutral germanium chloride (1:4) Identifiers CAS Number 10038-98-9 Y 3D model (JSmol) Interactive image ChemSpider 59611 Y ECHA InfoCard 100.030.093 PubChem CID 66226 RTECS number LY5220000 UNII YSV1R803C0 Y CompTox Dashboard (EPA) DTXSID1044350 InCh...

KupidoDewa asmara, cinta erotis, ketertarikan, dan kasih sayangPatung dewa asmara klasik dengan busurnyaSimbolBusur dan PanahKendaraanLumba-lumbaInformasi pribadiPsycheAnakVoluptasOrang tuaMars dan VenusYunaniEros Bagian dari seri tentangCintaRed-outline heart icon Jenis-jenis cinta Afeksi Ikatan Patah hati Cinta yang penuh kasih Cinta konjugal Cinta bahaduri Pacaran Troubadour Jatuh cinta Persahabatan cross-sex romantis Zona pertemanan Keramahan Hubungan antar pribadi Intimasi Limerence Keca...

Railway station in Kostrzyn nad Odrą, Poland KostrzynRailway StationKostrzyn railway stationGeneral informationLocationKostrzyn nad Odrą, Lubusz VoivodeshipPolandCoordinates52°35′29″N 14°38′50″E / 52.5915°N 14.6472°E / 52.5915; 14.6472Operated byPKP Polregio Arriva/Niederbarnimer EisenbahnLine(s)203: Tczew–Kostrzyn railway 273: Wrocław–Szczecin railway 410: Grzmiąca–Kostrzyn railway (closed)Platforms5Other informationFare zoneVBB: 5375[1]H...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Desember 2022. Lisa MillerLISA MILLER, Ph.D.KebangsaanAmericanAlmamaterUniversitas Yale, BA, Magna Cum Laude, Distinction in Psychology Universitas Pennsylvania, M.S., Ph.D. Universitas Columbia, Sekolah Tinggi Dokter & Ahli Bedah, Pasca-doktoralKarier ilmiahBid...



























