Coolie (2004 film)
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Grumman G-72 Kitten Grumman Kitten adalah monoplane kabin sayap rendah (low wing) 1940 Amerika dirancang dan dibangun oleh Grumman. Dua versi dibangun, G-63 Kitten I dengan landing gear tailwheel ditarik, dan G-72 Kitten II dengan landing gear nosewheel ditarik. Referensi Taylor, Michael J. H. (1989). Jane's Encyclopedia of Aviation. London: Studio Editions. The Illustrated Encyclopedia of Aircraft (Part Work 1982-1985). Orbis Publishing. 1985. Pranala luar Aerofiles - Grumman Dia...

Pour les articles homonymes, voir Congrès (homonymie). Dépouillement du vote des adhérents en vue du congrès socialiste de 2008. Le congrès est la plus haute instance de la Section française de l'Internationale ouvrière de 1905 à 1969 puis du Parti socialiste depuis 1969. Le rôle du congrès est de fixer la ligne politique du parti et d'élire sa direction. Congrès historiques Certains congrès ont marqué profondément les socialistes français : Congrès du Globe de 1905, au...

Demographics of the population of Saint Kitts and Nevis include population density, ethnicity, religious affiliations and other aspects. Census population and average annual growth rateYearPop.±% p.a.1871 39,872— 1881 41,100+0.30%1891 44,000+0.68%1901 42,600−0.32%1911 39,200−0.83%1921 34,000−1.41%1946 41,200+0.77%1960 51,100+1.55%1970 44,880−1.29%1981 43,309−0.32%1991 40,613−0.64%2001 46,325+1.32%2011 46,398+0.02%Source:[1][2] Population ...

Infections of the skin This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Skin infection – news · newspapers · books · scholar · JSTOR (September 2019) (Learn how and when to remove this message) A skin infection is an infection of the skin in humans and other animals, that can also affect the associated soft t...

У этого термина существуют и другие значения, см. Петропавловка. СелоПетропавловка 51°00′15″ с. ш. 39°11′55″ в. д.HGЯO Страна Россия Субъект Федерации Воронежская область Муниципальный район Лискинский Сельское поселение Петропавловское История и география Час...

For other similarly pronounced Chinese surnames, see Qiú. QiuQiū surname in traditional Chinese charactersPronunciationQiū (Pinyin)Jau1Khiu / Khu (Pe̍h-ōe-jī)Language(s)Chinese, KoreanOriginLanguage(s)Old ChineseRegion of originChinaOther namesVariant form(s)Qiū (Mandarin)Yau (Cantonese)Kho, Khoe, Khoo, Koo, Khu (Hokkien, Teochew)Khâu (Vietnamese)Koo, Gu, Ku (Korean) Qiu (丘/邱) is an East Asian surname. Pinyin: Qiū in Mandarin Chinese, Yau4 in Cantonese, Chiu1 in Wade-Giles (widel...

Road in Brisbane, Australia Gympie RoadQueenslandGeneral informationTypeRoadLength19 km (12 mi)Route number(s) A3 (Lutwyche to Bald Hills) State Route 58 (Bald Hills to Petrie) Major junctionsSE end Lutwyche Road, Lutwyche, Queensland Gympie Arterial RoadNW end Dayboro Road, Petrie, QueenslandLocation(s)Major suburbsKedron, Chermside, Aspley, Strathpine Gympie Road, Kedron 2008 Gympie Road is a major road in the northern suburbs of Brisbane, Queensland, Australia. The road for...

Fox Business NetworkDiluncurkan15 Oktober 2007PemilikFox Entertainment Group(21st Century Fox)SloganThe Power to Prosper; Opportunity. Pure and Simple.NegaraAmerika SerikatBahasaBahasa InggrisKantor pusatNew York City, New YorkSaluran seindukFox News ChannelSitus webwww.foxbusiness.com Fox Business Network (FBN) adalah sebuah saluran televisi berita dan bisnis kabel dan satelit Amerika Serikat yang dimiliki oleh divisi Fox Entertainment Group yaitu 21st Century Fox. jaringan ini membahas meng...

Ne doit pas être confondu avec Nemanjina. Nemanjina La rue Nemanjina Situation Coordonnées 44° 50′ 22″ nord, 20° 24′ 41″ est Pays Serbie Ville Belgrade Quartier(s) Zemun Début Avijatičarski trg, rues Nikolaja Ostrovskog et Miroslava Tirša Fin Rues Štrosmajerova et Svetosavska Morphologie Type Rue Géolocalisation sur la carte : Serbie modifier La rue Nemanjina (en serbe cyrillique : Немањина) est située à Belgrade, la ca...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (نوفمبر 2019) مفوض النقل الأوروبي أدينا إيوانا فولان [لغات أخرى] عن المنصب المعين رئيس المفوضية الأوروبية...

Il Complesso di San Firenze, Firenze Ferdinando Ruggieri, anche Ruggeri[1] (Firenze, 1691 – Firenze, 27 giugno 1741), è stato un architetto italiano, tra i protagonisti del periodo barocco a Firenze. Indice 1 Biografia 2 Opere letterarie 3 Altre immagini 4 Note 5 Altri progetti 6 Collegamenti esterni Biografia Il suo capolavoro è la facciata del complesso di San Firenze (1715), ma lavorò anche al Palazzo Bastogi, già Capponi, al Palazzo di Gino Capponi per i fratelli Scipione e ...

CervellettoRappresentazione tridimensionale del cervelletto (in rosso) nell'encefalo umano.Disegno di un cervello umano, sono evidenziati il cervelletto e il ponte Anatomia del Gray(EN) Pagina 788 SistemaNevrasse ArteriaArterie vertebrali Venasuperior cerebellar veins e inferior cerebellar veins IdentificatoriMeSHA08.186.211.132.810.428.200 TAA14.1.07.001 FMA67944 ID NeuroLexbirnlex_1489 Modifica dati su Wikidata · Manuale Il cervelletto (in latino cerebellum che significa piccolo cerve...

Questa voce o sezione sull'argomento pittori svedesi non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo le linee guida sull'uso delle fonti. Alexander Roslin, Autoritratto, 1790, Malmö, Konstmuseum Alexander Roslin (Malmö, 15 luglio 1718 – Parigi, 5 luglio 1793) è stato un pittore svedese, noto soprattutto come ritrattista. Indice 1 Biografia 1.1 I primi anni e l'inizio della carriera 1.2...

The following highways are numbered 31C: India National Highway 31C (India) United States Nebraska Spur 31C New York State Route 31C (former) vteList of highways numbered ...0–9 0 1 1A 1B 1D 1X 2 2A 2N 3 3A 3B 3C 3E 3G 4 4A 5 5A 5B 6 6A 6N 7 7A 7B 7C 8 9 9A 9B 9E 9W 10–16 10 10A 10N 11 11A 11B 11C 12 12A 12B 12C 12D 12E 12F 13 13A 14 14A 15 15A 16 16A 17–22 17 17A 17B 17C 17E 17F 17J 18 18A 18B 18C 18D 18E 18F 19 19A 20 20A 20B 20C 20D 21 21A 22 22A 23–31 23 23A 24 24A 25 25A 25B 25C...

Voce principale: Associazione Calcio Savoia 1908. Società Sportiva SavoiaStagione 2002-2003 Sport calcio Squadra Savoia Allenatore Alberto Urban (1ª-14ª) poi Piero Cucchi (15ª-19ª) poi Guglielmo Ricciardi (20ª-34ª) Presidente Dario Pasquariello Serie D6º posto Coppa Italia Serie DPrimo turno Maggiori presenzeCampionato: Bucciarelli, Cerrato, Oriente (29)Totale: Bucciarelli, Cerrato, Oriente (29) Miglior marcatoreCampionato: Sgambati (10)Totale: Sgambati (10) StadioAlfredo Giraud...

Cet article est une ébauche concernant une compétition cycliste. Vous pouvez partager vos connaissances en l’améliorant (comment ?) selon les recommandations des projets correspondants. Championnats d'Afrique de cyclisme sur route Généralités Sport Cyclisme sur route Création 1995 Périodicité Annuelle Pour la dernière compétition voir : Championnats d'Afrique de cyclisme sur route 2023 modifier Les Championnats d'Afrique de cyclisme sur route sont une série d...

Nine Muses(9MUSES) 左から、クムジョ、ヘミ、キョンリ、ソジン(2016年9月)基本情報出身地 韓国ジャンル K-POP、ダンス活動期間 2010年8月12日 - 2019年2月24日レーベル LOENGENIE MUSIC(2015年 - 2019年2月24日)事務所 韓国 スター帝国メンバー ヘミキョンリクムジョソジン旧メンバー ジェギョン、ラナ、ピニ、イセム、ウンジ、セラ、イユエリン、ミンハ、ヒョナ、ソンア Nine Muses...

この記事はドイツ語から大ざっぱに翻訳されたものであり、場合によっては不慣れな翻訳者や機械翻訳によって翻訳されたものかもしれません。 翻訳を改善してくださる方を募集しています。 フロリダ州キー・ラーゴの沖合に設置されたNASAの宇宙飛行士の訓練施設であるAquarius SEALAB Aquariusの内部 海中居住施設(かいちゅうきょじゅうしせつ、英語: Sea habitat)は、�...

The Perfect Mile Cover of paperback, depicting Roger Bannister breaking the 4 minute mile recordAuthorNeal BascombLanguageEnglishGenreNon-fictionPublisherMariner BooksPublication date2004Publication placeUnited StatesPages344ISBN0-618-56209-5OCLC54001404Preceded byHigher: A Historic Race to the Sky and the Making of a City Followed byRed Mutiny: Eleven Fateful Days on the Battleship Potemkin The Perfect Mile: Three Athletes, One Goal, and Less Than Four Minutes to Achieve...
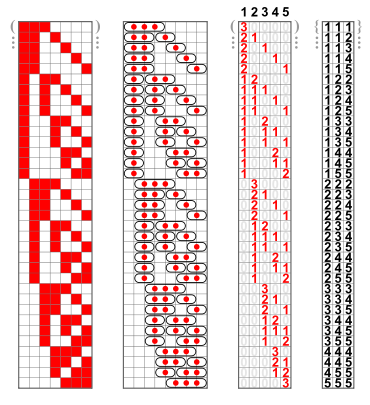
Mathematical set with repetitions allowed This article is about the mathematical concept. For the computer science data structure, see Multiset (abstract data type). In mathematics, a multiset (or bag, or mset) is a modification of the concept of a set that, unlike a set,[1] allows for multiple instances for each of its elements. The number of instances given for each element is called the multiplicity of that element in the multiset. As a consequence, an infinite number of multisets ...
