Chepillo

Chepillo
Corregimiento
Chepillo is located in Panamá Province
Chepillo
Chepillo
Chepillo is located in Panama
Chepillo
Chepillo
Coordinates: 8°57′14.67″N 79°7′43.33″W / 8.9540750°N 79.1287028°W / 8.9540750; -79.1287028
Country Panama
ProvincePanamá
DistrictChepo
Area
[1]
 • Land1 km2 (0.4 sq mi)
Population
 (2010)[1]
 • Total
255
 • Density263.3/km2 (682/sq mi)
 Population density calculated based on land area.
Time zoneUTC−5 (EST)

Chepillo is a corregimiento in Chepo District, Panamá Province, Panama, with a population of 255 as of 2010.[1] Its population as of 1990 was 254; its population as of 2000 was 237.[1]

References

  1. ^ a b c d "Cuadro 11 (Superficie, población y densidad de población en la República...)" Archived 2016-03-04 at the Wayback Machine Table 11 (Area, population, and population density in the Republic...) (.xls). In "Resultados Finales Básicos" [Basic Final Results] (in Spanish). National Institute of Statistics and Census of Panama. Archived from the original on July 10, 2015. Retrieved May 26, 2015.


This article about the geography of Panamá Province is a stub. You can help Wikipedia by expanding it.

Read other articles:

Every Nation for Itself

Every Nation for Itself: Winners and Losers in a G-Zero World PengarangIan BremmerNegaraAmerika SerikatBahasaInggrisGenrePolitik, hubungan internasionalPenerbitPortfolioTanggal terbit1 Mei 2012Jenis mediaSampul kerasHalaman240 hlm. (sampul keras )ISBNISBN 1-5918-4468-1Situs webBook website Every Nation for Itself: Winners and Losers in a G-Zero World adalah buku nonfiksi tahun 2012 karya Ian Bremmer yang menjelaskan berkembangnya kekosongan kekuasaan G-Nol dalam politik internasiona...

 

Karate pada Pekan Olahraga Nasional XIX – Kumite 55 kg putra

Karate padaPekan Olahraga Nasional XIX Kata Putra Putri   Perorangan     Perorangan     Beregu Beregu Kumite Putra Putri   Beregu     Beregu     55 kg 50 kg 60 kg 55 kg 67 kg 61 kg 75 kg 68 kg 84 kg +68 kg +84 kg Karate nomor Kumite 55 kg putra pada Pekan Olahraga Nasional XIX dipertandingkan pada 20 September 2016[1] di Sasana Budaya Ganesha, Institut Teknologi Bandung, Kota Bandung, Jawa Barat. Pertandingan karate menggunakan s...

 

Music of Armenia

History and present of musical culture of Armenia You can help expand this article with text translated from the corresponding article in Armenian. (June 2020) Click [show] for important translation instructions. View a machine-translated version of the Armenian article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than simply copy-past...

The Best of Both Worlds

The Best of Both WorldsSingel oleh Miley Cyrusdari album Hannah MontanaSisi-BIf We Were a MovieDirilis28 Maret 2006 (2006-03-28)Direkam2005GenrePop rockpop remajaDurasi2:54LabelWalt DisneyPenciptaMatthew GerrardRobbie NevilProduserMatthew GerrardKronologi singel Miley Cyrus The Best of Both Worlds (2006) Who Said (2006) The Best of Both Worlds adalah lagu pop-rock yang dibawakan oleh penyanyi dan aktris asal Amerika Serikat Miley Cyrus untuk serial televisi Disney Channel, Hannah Montana...

 

羅德島州

本條目存在以下問題，請協助改善本條目或在討論頁針對議題發表看法。 此條目需要擴充。 (2013年1月1日)请協助改善这篇條目，更進一步的信息可能會在討論頁或扩充请求中找到。请在擴充條目後將此模板移除。 此條目需要补充更多来源。 (2013年1月1日)请协助補充多方面可靠来源以改善这篇条目，无法查证的内容可能會因為异议提出而被移除。致使用者：请搜索一下条目的...

 

中華民國國軍中的中共間諜列表

 本表是動態列表，或許永遠不會完結。歡迎您參考可靠來源來查漏補缺。 潛伏於中華民國國軍中的中共間諜列表收錄根據公開資料來源，曾潛伏於中華民國國軍、被中國共產黨聲稱或承認，或者遭中華民國政府調查審判，為中華人民共和國和中國人民解放軍進行間諜行為的人物。以下列表以現今可查知時間為準，正確的間諜活動或洩漏機密時間可能早於或晚於以下所歸�...

AIPAC

Pro-Israel lobby group in the United States American Israel Public Affairs CommitteeFoundedJanuary 3, 1963; 61 years ago (1963-01-03)[1]Tax ID no. 53-0217164[2]Legal status501(c)(4) organizationHeadquartersWashington, D.C., U.S.[2]Coordinates38°54′02″N 77°00′53″W / 38.9004676°N 77.0146576°W / 38.9004676; -77.0146576President, Board of DirectorsBetsy Berns KornChairman, Board of DirectorsMort FridmanChief Executive O...

 

Lutheran Church - International

Lutheran Church - InternationalClassificationLutheranOrientationEvangelical CatholicPolityEpiscopal/CongregationalFounderPastor Edward TornowOrigin1967 North DakotaCongregations15Members1000Other name(s)International Lutheran Fellowship (1967–2011)Official websitewww.lutheranchurchinternational.org Part of a series onLutheranism in the United States Heritage of Mainline Protestantism and the Confessing Movement Augsburg Lutheran Churches Evangelical Lutheran Church in America Latvian Evange...

 

Oblast Zaporizhzhia

Artikel ini bukan mengenai Zaporizhzhia; Zaporizhzhia, Luhansk; atau Oblast Zaporozhye. Oblast Zaporizhzhia Запоріжжя областьOblastGedung Administrasi Oblast Zaporizhzhia BenderaLambang kebesaranJulukan: Запоріжжя (Zaporizhzhya)Negara UkrainaIbu kotaZaporizhzhiaPemerintahan • GubernurOleksandr Starukh[1] • Ketua Dewan OblastHryhoriy SamardakLuas • Total27.183 km2 (10,495 sq mi)Peringkat9Populasi ...

Meditations (Mal Waldron album)

1972 live album by Mal WaldronMeditationsLive album by Mal WaldronReleased1972Recorded12 July 1972VenueDug, TokyoGenreJazzLength45:05LabelRCA VictorProducerTetsuya ShimodaMal Waldron chronology The Whirling Dervish(1972) Meditations(1972) Up Popped the Devil(1973) Meditations – Live at Dug is a 1972 live album recorded by American jazz pianist Mal Waldron.[1] This is his solo album recorded live in the jazz club Dug in the Shinjuku district of Tokyo. The record was re-releas...

 

House of Medina Sidonia

Spanish noble house originated from the crown of Castile This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these template messages) This article may rely excessively on sources too closely associated with the subject, potentially preventing the article from being verifiable and neutral. Please help improve it by replacing them with more appropriate citations to reliable, independent, third-party sources. (December ...

 

تجمع بدو حبوظة (غيل بن يمين)

' تجمع بدو حبوظة  - قرية -  تقسيم إداري البلد  اليمن المحافظة محافظة حضرموت المديرية مديرية غيل بن يمين العزلة عزلة غيل بن يمين السكان التعداد السكاني 2004 السكان 48   • الذكور 20   • الإناث 28   • عدد الأسر 7   • عدد المساكن 7 معلومات أخرى التوقيت توقيت اليمن (...

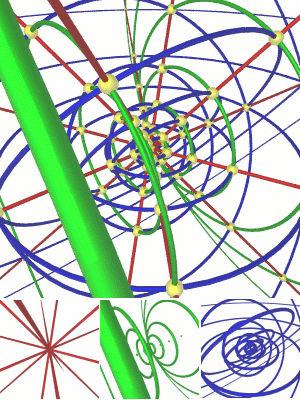
Hypercone

4-dimensional figure This article is about the geometric concept. For the spacecraft re-entry mechanism, see Hypercone (spacecraft). This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Hypercone – news · newspapers · books · scholar · JSTOR (September 2014) (Learn how and when to remove this message) Stereograph...

 

Formentera

Ne doit pas être confondu avec Fuerteventura. Formentera Héraldique Drapeau Vue satellitaire de Formentera. Administration Pays Espagne Communauté autonome Îles Baléares Comarque Formentera District judic. Ibiza Maire Mandat Jaume Ferrer Ribas (GxF) Depuis 2007 Code postal 07871 Démographie Gentilé Formenterer/a, Formenterenc/a Population 11 418 hab. (1 janvier 2022) Densité 137 hab./km2 Géographie Coordonnées 38° 42′ nord, 1° 27′ est Altitu...

 

Abdominoplasty

Surgical procedure to make the abdomen thinner and firmer This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these messages) This article possibly contains original research. Please improve it by verifying the claims made and adding inline citations. Statements consisting only of original research should be removed. (February 2016) (Learn how and when to remove this message) This article needs additional citations f...

Wilhelmina von Bremen

American sprinter Wilhelmina von BremenPersonal informationBornAugust 13, 1909San Francisco, California, United StatesDiedJuly 16, 1976 (aged 66)Alameda, California, United StatesHeight1.82 m (6 ft 0 in)Weight68 kg (150 lb)SportSportSprint runningClubWestern Women's Club, San Francisco Medal record Women's athletics Representing the  United States Olympic Games 1932 Los Angeles 4×100 m relay 1932 Los Angeles 100 meters Wilhelmina Billie von Bremen (August 13, 19...

 

Géométrie dans l'espace

Hyperboloïde de révolution à une nappe En mathématiques, la géométrie dans l'espace consiste à étudier les objets définis dans la géométrie plane dans un espace à trois dimensions et à y ajouter des objets qui ne sont pas contenus dans des plans : surfaces (plans et surfaces courbes) et volumes fermés. Il s'agit donc de géométrie dans un espace à trois dimensions. Géométrie euclidienne dans l'espace On peut adopter, dans l'espace à trois dimensions, les mêmes axiomes...

 

Santo

Disambiguazione – Se stai cercando altri significati, vedi Santo (disambigua). Disambiguazione – Santi rimanda qui. Se stai cercando altri significati, vedi Santi (disambigua). Disambiguazione – Santa rimanda qui. Se stai cercando altri significati, vedi Santa (disambigua). Questa voce o sezione sull'argomento religione non cita le fonti necessarie o quelle presenti sono insufficienti. Puoi migliorare questa voce aggiungendo citazioni da fonti attendibili secondo l...

1000 Ways to Lie

American television special program 1000 Ways to LieGenreDocufictionDocudramaWritten byAliyah SilversteinDirected byThom BeersNarrated byJohn Glover [verification needed]Country of originUnited StatesOriginal languageEnglishNo. of seasons1No. of episodes1ProductionRunning time22 minutesOriginal releaseNetworkSpikeReleaseMarch 3, 2010 (2010-03-03) 1000 Ways to Lie is an American television special and spin-off of the television series 1000 Ways to Die. It recreates intri...

 

Inclusion (mathématiques)

Pour les articles homonymes, voir Inclusion. Ne pas confondre avec la relation d'appartenance Diagramme d'Euler qui montre un ensemble A qui est inclus dans un ensemble B. On dit que A est sous-ensemble de B, ou que B est sur-ensemble de A. En mathématiques, l’inclusion est une relation d'ordre entre ensembles. On dit qu'un ensemble A est inclus dans un ensemble B si tous les éléments de A sont aussi éléments de B. On dit dans ce cas que A est un sous-ensemble ou une partie de B, ou e...