Videmonte
| ||||||||||||||||||||||||||||||||
Read other articles:

American basketball player (born 1996) Jalen BrunsonBrunson with the Knicks in 2023No. 11 – New York KnicksPositionPoint guard / Shooting guardLeagueNBAPersonal informationBorn (1996-08-31) August 31, 1996 (age 27)New Brunswick, New Jersey, U.S.Listed height6 ft 2 in (1.88 m)Listed weight190 lb (86 kg)Career informationHigh schoolStevenson (Lincolnshire, Illinois)CollegeVillanova (2015–2018)NBA draft2018: 2nd round, 33rd overall pickSelected by the Da...
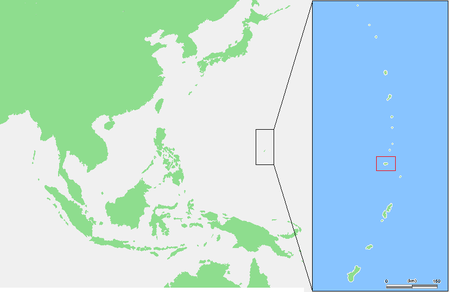
AnatahanGeografiLokasiSamudra PasifikKoordinat16°21′5″N 145°40′43″E / 16.35139°N 145.67861°E / 16.35139; 145.67861KepulauanKepulauan Mariana UtaraLuas33,9 km2Panjang9 kmLebar4 kmTitik tertinggi790 mPemerintahanNegaraAmerika SerikatPersemakmuranKepulauan Mariana UtaraKependudukanPendudukTidak berpenghuni (2010) Erupsi Anatahan pada tahun 2003 Anatahan adalah sebuah pulau di Kepulauan Mariana Utara, Samudra Pasifik. Pulau ini memi...

My Love Story!!Sampul volume manga pertama menampilkan Makoto Sunakawa (kiri), Takeo Gōda (tengah) dan Rinko Yamato (kanan).俺物語!!(Ore Monogatari!!)GenreKomedi romantis[1] MangaPengarangKazune KawaharaIlustratorArukoPenerbitShueishaPenerbit bahasa InggrisNA Viz MediaMajalah Bessatsu Margaret Sister Bessatsu Margaret DemografiShōjoTerbitOktober 2011 – 13 Juli, 2016Volume13 (Daftar volume) Seri animeSutradaraMorio AsakaSkenarioNatsuko TakahashiMusikS.E.N.S.StudioMadhousePel...

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (أبريل 2021) هذه المقالة يتيمة إذ تصل إليها مقالات أخرى قليلة جدًا. فضلًا، ساعد بإضافة وصلة إليها في مقالات متعلقة بها. ...

Siklus enam puluh tahunan干支 (Ganzhi) 01Jiǎzǐ甲子 02Yǐchǒu乙丑 03Bǐngyín丙寅 04Dīngmǎo丁卯 05Wùchén戊辰 06Jǐsì己巳 07Gēngwǔ庚午 08Xīnwèi辛未 09Rénshēn壬申 10Guǐyǒu癸酉 11Jiǎxū甲戌 12Yǐhài乙亥 13Bǐngzǐ丙子 14Dīngchǒu丁丑 15Wùyín戊寅 16Jǐmǎo己卯 17Gēngchén庚辰 18Xīnsì辛巳 19Rénwǔ壬午 20Guǐwèi癸未 21Jiǎshēn甲申 22Yǐyǒu乙酉 23Bǐngxū丙戌 24Dīnghài丁亥 25Wùzǐ戊子 26Jǐchǒu己丑 27Gēngyín庚�...

Хип-хоп Направление популярная музыка Истоки фанкдискоэлектронная музыкадабритм-энд-блюзреггидэнсхоллджаз[1]чтение нараспев[англ.]исполнение поэзииустная поэзияозначиваниедюжины[англ.]гриотыскэтразговорный блюз Время и место возникновения Начало 1970-х, Бронкс, Н...

French mathematician (1907–1979) Michel LoèveBorn(1907-01-22)January 22, 1907Jaffa, Ottoman SyriaDiedFebruary 17, 1979(1979-02-17) (aged 72)Berkeley, California, USNationalityFrench, AmericanAlma materUniversity of ParisKnown forKarhunen–Loève theoremScientific careerFieldsMathematicsInstitutionsUniversity of California, BerkeleyUniversity of Lyon,University of ParisUniversity of LondonDoctoral advisorPaul LévyDoctoral studentsLeo BreimanEmanuel Parzen Michel Loève (Jan...

Panamanian persons (including residents) that are of Chinese origin/descent Ethnic group Ethnic Chinese in Panama巴拿馬華人 Chino-panameñosTotal population135,000 (2003)4% of the Panamanian populationLanguagesSpanish, Hakka, Cantonese, Taishanese, Mandarin, EnglishReligionBuddhism[1] , Christianity[2]Related ethnic groupsAsian Latinos, Overseas Chinese Ethnic Chinese in Panama, also variously referred to as Chinese Panamanians, Panamanian Chinese and Panama Chinese (Span...

Quella sporca ultima metaPoliziotti contro prigionieri, in cima è Paul Crewe (Burt Reynolds) in scenaTitolo originaleThe Longest Yard Lingua originaleinglese Paese di produzioneStati Uniti d'America Anno1974 Durata121 min Rapporto1,85:1 Generecommedia, sportivo RegiaRobert Aldrich SoggettoAlbert S. Ruddy SceneggiaturaTracy Keenan Wynn ProduttoreAlbert S. Ruddy Casa di produzioneParamount Pictures, Albert S. Ruddy Productions, Long Road Productions Distribuzione in italianoParamount Home ...

County in Georgia, United States County in GeorgiaCatoosa CountyCountyCatoosa County Courthouse SealLogoLocation within the U.S. state of GeorgiaGeorgia's location within the U.S.Coordinates: 34°54′N 85°08′W / 34.90°N 85.14°W / 34.90; -85.14Country United StatesState GeorgiaFounded1853; 171 years ago (1853)SeatRinggoldLargest cityFort OglethorpeArea • Total162 sq mi (420 km2) • Land162 sq&#...

Granite carving of Robert Baden-Powell at Baden-Powell House in London, England Statue of Robert Baden-PowellThe statue, situated by the entrance to Baden-Powell House, Queen's Gate, South KensingtonArtistDon PotterYear1960MediumGraniteSubjectRobert Baden-Powell, 1st Baron Baden-PowellDimensions3 m (9.8 ft)LocationLondon, United KingdomCoordinates51°29′44″N 0°10′46″W / 51.4955°N 0.1795°W / 51.4955; -0.1795OwnerThe Scout Association The Statue ...
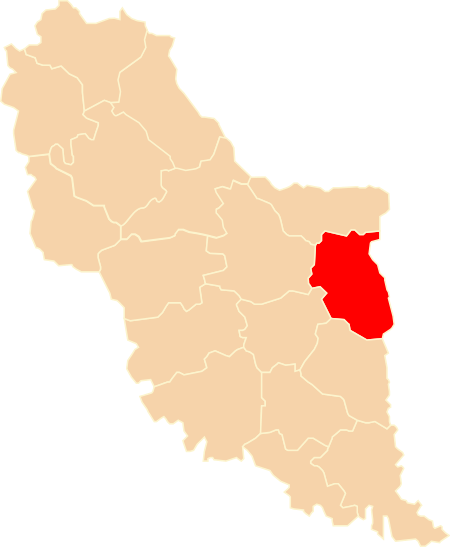
У Вікіпедії є статті про інші значення цього терміна: Скалатський повіт. Скалатський повіт Округ Тернопільський (до 1867) Коронний край Королівство Галичини та Володимирії Країна Австрійська імперія Австро-Угорщина Центр Скалат Створений 1854 Площа 834 км² (після 1867)[1] На...

Capacity of soil to support loads In geotechnical engineering, bearing capacity is the capacity of soil to support the loads applied to the ground. The bearing capacity of soil is the maximum average contact pressure between the foundation and the soil which should not produce shear failure in the soil. Ultimate bearing capacity is the theoretical maximum pressure which can be supported without failure; allowable bearing capacity is the ultimate bearing capacity divided by a factor of safety....

Events at the2019 Asian AthleticsChampionshipsTrack events100 mmenwomen200 mmenwomen400 mmenwomen800 mmenwomen1500 mmenwomen5000 mmenwomen10,000 mmenwomen100 m hurdleswomen110 m hurdlesmen400 m hurdlesmenwomen3000 msteeplechasemenwomen4 × 100 m relaymenwomen4 × 400 m relaymenwomenmixedField eventsHigh jumpmenwomenPole vaultmenwomenLong jumpmenwomenTriple jumpmenwomenShot putmenwomenDiscus throwmenwomenHammer throwmenwomenJavelin throwmenwomenCombined eventsHeptathlonwomenDecathlonmenvte Th...

1968 studio album by Stevie Wonder For Once in My LifeStudio album by Stevie WonderReleasedDecember 6, 1968RecordedMid 1967December 1967–February 1968April–August 1968Venue35:15StudioHitsville U.S.A., Detroit, MichiganGenre Soul[1] jazz[1] pop[2] LabelTamlaProducerHenry Cosby, Don Hunter, Stevie WonderStevie Wonder chronology Eivets Rednow(1968) For Once in My Life(1968) My Cherie Amour(1969) Singles from For Once in My Life Shoo-Be-Doo-Be-Doo-Da-DayRel...
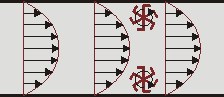
En el cálculo vectorial, el rotacional o rotor es un operador vectorial sobre campos vectoriales definidos en un abierto de R 3 {\displaystyle \mathbb {R} ^{3}} que muestra la tendencia de un campo vectorial a inducir rotación alrededor de un punto. Matemáticamente, esta idea se expresa como el límite de la circulación del campo vectorial, cuando la curva sobre la que se integra se reduce a un punto: U ⋅ rot F = U ⋅ ∇ × F ≡ lim Δ S →...
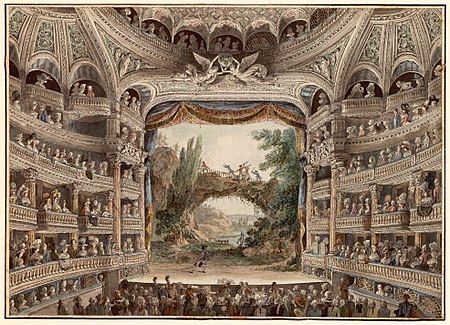
Dieser Artikel oder nachfolgende Abschnitt ist nicht hinreichend mit Belegen (beispielsweise Einzelnachweisen) ausgestattet. Angaben ohne ausreichenden Beleg könnten demnächst entfernt werden. Bitte hilf Wikipedia, indem du die Angaben recherchierst und gute Belege einfügst. Die Schlacht um Hernani (auch: Theaterschlacht, französisch: bataille d’Hernani) war ein Theaterskandal am 25. Februar 1830 in Paris, der die Literatur- und Theatergeschichte prägte. Bei der Uraufführung von Vict...

Vorlage:Infobox hochrangige Straße/Wartung/DE-B Bundesstraße 498 in Deutschland Karte Verlauf der B 498 Alle Koordinaten: OSM | WikiMap Basisdaten Betreiber: Deutschland Bundesrepublik Deutschland Straßenbeginn: Goslar(51° 54′ 53″ N, 10° 27′ 18″ O51.91485138800510.45503616333) Straßenende: Osterode am Harz(51° 43′ 41″ N, 10° 15′ 45″ O51.72807149400910.262517929077) Gesamtlänge: 39 km ...

Further information: Chemistry and Timeline of chemistry Part of a series onChemistryScience of matter Index Outline Glossary History (timeline) Key components Matter Phase Bond Chemical reaction Ion Acid–base reaction Redox Chemical equilibrium Chemical law Branches Analytical chemistry Biochemistry Organic chemistry Inorganic chemistry Physical chemistry Research Chemist (list) List of chemistry awards List of journals List of unsolved problems Chemistry portal Categoryvte The ...

Major railway and metro station in Kyoto, Japan Kyōto Station京都駅Kyoto Station BuildingJapanese nameShinjitai京都駅Kyūjitai京都驛Hiraganaきょうとえき General informationLocationShimogyo-ku, Kyōto CityKyōto PrefectureJapanOperated by JR West JR Central Kintetsu Railway Kyoto Municipal Subway Connections Bus terminalOther informationStation codeK11, B01PassengersFY2015[1]255 million LocationKyōto StationLocation within Kyoto cityShow map of Kyoto cityKyōto Station...


