Polywürfel
|
Read other articles:

Artikel ini perlu diwikifikasi agar memenuhi standar kualitas Wikipedia. Anda dapat memberikan bantuan berupa penambahan pranala dalam, atau dengan merapikan tata letak dari artikel ini. Untuk keterangan lebih lanjut, klik [tampil] di bagian kanan. Mengganti markah HTML dengan markah wiki bila dimungkinkan. Tambahkan pranala wiki. Bila dirasa perlu, buatlah pautan ke artikel wiki lainnya dengan cara menambahkan [[ dan ]] pada kata yang bersangkutan (lihat WP:LINK untuk keterangan lebih lanjut...
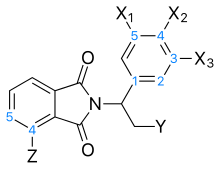
Drug Phosphodiesterase-5 A phosphodiesterase inhibitor is a drug that blocks one or more of the five subtypes of the enzyme phosphodiesterase (PDE), thereby preventing the inactivation of the intracellular second messengers, cyclic adenosine monophosphate (cAMP) and cyclic guanosine monophosphate (cGMP) by the respective PDE subtype(s). The ubiquitous presence of this enzyme means that non-specific inhibitors have a wide range of actions, the actions in the heart, and lungs being some of the ...

This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: Nova Scotia Oilers – news · newspapers · books · scholar · JSTOR (December 2009) (Learn how and when to remove this template message) Ice hockey team in Halifax, Nova ScotiaNova Scotia OilersCityHalifax, Nova ScotiaLeagueAmerican Hockey LeagueOperated1984–1988Home arenaHalifax...

Koordinat: 1°21′03″N 103°37′59″E / 1.35085°N 103.633132°E / 1.35085; 103.633132 Jembatan Malaysia–SingapuraLaluan Kedua Malaysia–SingapuraKoordinat1°21′03″N 103°37′59″E / 1.35085°N 103.633132°E / 1.35085; 103.633132Moda transportasiKendaraan bermotorMelintasiSelat JohorLokalMalaysia Second Link ExpresswaySingapuraAyer Rajah ExpresswayNama resmiMalaysia–Singapore Second LinkPengelolaMalaysiaPLUS Malaysia BerhadProjek...

American singer, songwriter, and pianist (1942–2018) Aretha and Queen of Soul redirect here. For other uses, see Aretha (disambiguation) and Queen of Soul (disambiguation). Aretha FranklinFranklin in 1968BornAretha Louise Franklin(1942-03-25)March 25, 1942Memphis, Tennessee, U.S.DiedAugust 16, 2018(2018-08-16) (aged 76)Detroit, Michigan, U.S.Resting placeWoodlawn Cemetery, DetroitOccupations Singer songwriter pianist civil rights activist record producer Years active1954–2017Spo...

Pour les articles homonymes, voir Paquin. Anna Paquin Anna Paquin à Toronto en septembre 2018. Données clés Nom de naissance Anna Helene Paquin Naissance 24 juillet 1982 (41 ans)Winnipeg, Manitoba (Canada) Nationalité Canadienne Néo-zélandaise Profession ActriceProductrice Films notables La Leçon de pianoDarkness Presque célèbresaga X-Men Séries notables True BloodThe Affair modifier Anna Paquin [ˈænə ˈpækwɪn][1], née le 24 juillet 1982 à Winnipeg, est une actr...

Народная (Национальная) Армиякит. 国民军 Страна Китайская Республика Подчинение до 1926 — самостоятельна, после 1926 — Гоминьдан Тип Вооружённые силы Дислокация Север Китая Участие в Северный поход, Гражданская война в Китае Командиры Известные командиры Фэн Юйсян, Ху ...
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)&#...

Римский Пантеон, при котором была создана академия Папская академия литературы и изящных искусств, полное название — Папская выдающаяся академия изящных искусств и словесности виртуозов при Пантеоне (итал. La Pontificia Insigne Accademia di Belle Arti e Letteratura dei Virtuosi al Pantheon) — старе...

Listing of professional wrestling champions for the TNA World Tag Team Championship Current champions The System (Brian Myers (left) and Eddie Edwards (right)). The TNA World Tag Team Championship is a professional wrestling championship contested for in Total Nonstop Action Wrestling's (TNA) tag team division. After the formation of TNA in June 2002, the company executives signed a contractual agreement with the National Wrestling Alliance (NWA) that allowed them control of the NWA World Hea...

The Middle Dutch Church in New York City near Nassau and Cedar Streets is where hundreds of the enlisted men captured at the Battle of Long Island were imprisoned. The Sugar House next door also became a prison for thousands as the British captured more of Washington's troops from Fort Washington and other engagements during the retreat from New York. The site today is the location of One Chase Manhattan Plaza. (Image from about 1830.)[1] [2]Interior of the British prison shi...

Part of a series on theOlympic water polorecords and statistics Topics Overall statistics men women Champions men women Team appearances men women Player appearances men women Medalists men women Top goalscorers men women Goalkeepers men women Flag bearers and oath takers Venues Teams Men's teams Australia Belgium Brazil Canada Croatia Egypt France Germany Great Britain Greece Hungary Italy Japan Kazakhstan Montenegro Netherlands Romania Russia Serbia Serbia and Montenegro Soviet Union Spain...

Voce principale: Cuoiopelli Cappiano Romaiano. Cuoiopelli Cappiano RomaianoStagione 2008-2009Sport calcio Squadra CuoioCappiano Allenatore Agostino Iacobelli Presidente Michele Videtta e Carlo Battini Lega Pro Seconda Divisione6º posto nel girone B. Il Cuoiopelli Cappiano Romaiano si scioglie e vengono rifondati l'ASD Ponte a Cappiano FC e l'Unione Calcio Cuoiopelli. Maggiori presenzeCampionato: Granito (34) Miglior marcatoreCampionato: Granito (9) 2007-2008 Si invita a seguire il mode...

Éliminatoires de la coupe du monde 1934 Généralités Sport Football Organisateur(s) FIFA Édition 2e Coupe du monde1re pour les éliminatoires Date 1933 - 1934 Participants 32 inscrits32 acceptéspas de qualifié d'office. Épreuves 26 rencontres Navigation Éliminatoires 1938 modifier Les différents tours préliminaires à la Coupe du monde 1934 constituent la première édition des éliminatoires puisqu'en 1930, pour la première Coupe du monde, qui se joue « sur invitation ...

座標: 北緯34度50分46秒 東経138度57分40秒 / 北緯34.846度 東経138.961度 / 34.846; 138.961 八丁池付近の空中写真。1976年撮影。池の西側に見える北北西-南南東に走る線が断層。国土交通省 国土地理院 地図・空中写真閲覧サービスの空中写真を基に作成。 八丁池の位置 八丁池周辺の地形図 八丁池 八丁池(はっちょういけ)は、静岡県伊豆市の天城山の西陵にあ�...

Nuclides predating the Earth's formation (found on Earth) Nuclear physics Nucleus Nucleons p n Nuclear matter Nuclear force Nuclear structure Nuclear reaction Models of the nucleus Liquid drop Nuclear shell model Interacting boson model Ab initio Nuclides' classification Isotopes – equal Z Isobars – equal A Isotones – equal N Isodiaphers – equal N − Z Isomers – equal all the above Mirror nuclei – Z ↔ N Stable Magic Even/odd Halo Borromean Nuclear stability Bindi...

Suède aux Jeux olympiques d'hiver de 2006 Code CIO SWE Comité Comité national olympique suédois Lieu Turin Participation 20e aux Jeux d'hiver Athlètes 106 dans 10 sports Porte-drapeau Anja Pärson (cérémonie d'ouverture) et Anette Norberg (cérémonie de clôture) MédaillesRang : 6e Or7 Arg.2 Bron.5 Total14 Suède aux Jeux olympiques d'hiver Suède aux Jeux olympiques d'hiver de 2002 Suède aux Jeux olympiques d'hiver de 2010 modifier Cet article contient des informations ...

1944 in music By location United Kingdom Norway By genre country jazz By topic List of albums released 1944 in jazzColeman Hawkins/Thelonious Monk - drifting on a reed 1944Decade1940s in jazzMusic1944 in musicStandardsList of 1940s jazz standardsSee also1943 in jazz – 1945 in jazz Overview of the events of 1944 in jazz List of years in jazz … 1934 1935 1936 1937 1938 1939 1940 1941 1942 1943 1944 1945 1946 1947 1948 1949 1950 1951 1952 1953 1954 … Art Archaeology Architecture Litera...

French composer (c. 1628–1677) Robert Cambert (c. 1628–1677) was a French composer principally of opera.[1] His opera Pomone was the first actual opera in French. Biography Under Mazarin Born in Paris c. 1628, he studied music under Chambonnières. His first position was as organist at the church of Saint-Honoré in Paris. In 1655 he married Marie du Moustier. At this time he came under the patronage of Cardinal Mazarin who was instrumental in his appointment as Superintendent of...

No debe confundirse con el país vecino, la República Democrática del Congo. República del CongoRépublique du Congo (francés) Estado miembro de la Unión Africana[1]Bandera Escudo Lema: Unité, travail et progrès(francés: 'Unidad, trabajo y progreso') Himno: La congolaise(francés: «La Congoleña») Capital(y ciudad más poblada) Brazzaville4°16′10″S 15°16′16″E / -4.2694444444444, 15.271111111111 Idioma oficial Francés • Hablados...













