NGC 4607
| |||||||||||||||||||||||||||||||||||||||||||||||||||
Read other articles:

Halaman ini berisi artikel tentang salah satu kota di Jawa Barat (Jabar), Indonesia sejak 1999. Untuk wilayah administratif di Indonesia dalam kurun waktu 1982–1999, lihat Kota Administratif Depok. Untuk tempat lain yang bernama sama, lihat Depok (disambiguasi).Koordinat: 6°22′21″S 106°49′39″E / 6.37250°S 106.82750°E / -6.37250; 106.82750 Kota DepokKotaTranskripsi bahasa daerah • Pegonديڤوك • Aksara Sundaᮓᮨᮕᮧᮊ�...

Species of bat Narrow-winged pipistrelle Conservation status Least Concern (IUCN 3.1)[1] Scientific classification Domain: Eukaryota Kingdom: Animalia Phylum: Chordata Class: Mammalia Order: Chiroptera Family: Vespertilionidae Genus: Pipistrellus Species: P. stenopterus Binomial name Pipistrellus stenopterusDobson, 1875 The narrow-winged pipistrelle (Pipistrellus stenopterus) is a species of vesper bat. It can be found in Brunei Darussalam, Indonesia, and Malaysia. Referenc...

Lauri Pihlap dan Kaido Põldma pada tahun 2007. Soul Militia (dikenal sampai 2002 dengan sebutan 2XL) adalah sebuah grup musik hip hop asal Estonia. Mereka dikenal di mancanegara karena memenangkan Kontes Lagu Eurovision 2001 sebagai penyanyi bekingan untuk Tanel Padar dan Dave Benton, dengan lagu Everybody.[1] Referensi ^ Tanel Padar, Dave Benton & 2XL Everybody (Estonia 2001). Kontes Lagu Eurovision. Diarsipkan dari versi asli tanggal 1 November 2014. Diakses tanggal 19 November...

هنودمعلومات عامةنسبة التسمية الهند التعداد الكليالتعداد قرابة 1.21 مليار[1][2]تعداد الهند عام 2011ق. 1.32 مليار[3]تقديرات عام 2017ق. 30.8 مليون[4]مناطق الوجود المميزةبلد الأصل الهند البلد الهند الهند نيبال 4,000,000[5] الولايات المتحدة 3,982,398[6] الإمار...

Darè abolished municipality in ItalyFrazionecadastral municipality Darè (it) Tempat Negara berdaulatItaliaDaerah otonom dengan status istimewaTrentino-Tirol SelatanProvinsi di ItaliaTrentinoKomune di ItaliaPorte di Rendena NegaraItalia Ibu kotaDarè GeografiLuas wilayah1,16 km² [convert: unit tak dikenal]Ketinggian600 m Berbatasan denganMontagne, Italia Vigo Rendena Villa Rendena Informasi tambahanKode pos38094 (già 38080) Zona waktuUTC+1 UTC+2 Kode telepon0465 Darè adalah komune ya...

Questa voce sugli argomenti allenatori di pallacanestro statunitensi e cestisti statunitensi è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti dei progetti di riferimento 1, 2. Armond Hill Nazionalità Stati Uniti Altezza 193 cm Peso 86 kg Pallacanestro Ruolo Allenatore (ex playmaker) Termine carriera 1984 - giocatore CarrieraGiovanili Bishop Ford High School1972-1976 Princeton TigersSquadre di club 1976-1980 Atlanta...

Nikolaus Harnoncourt Nikolaus Harnoncourt nome completo Johann Nicolaus Graf[1] de la Fontaine und d'Harnoncourt-Unverzagt (Berlino, 6 dicembre 1929 – Sankt Georgen im Attergau, 5 marzo 2016) è stato un direttore d'orchestra, violoncellista, gambista e musicologo austriaco, molto noto per le sue esecuzioni accurate dal punto di vista della pratica esecutiva storica della musica dell'epoca classica e precedente. Indice 1 Biografia 2 CD 3 DVD & BLU-RAY 4 Premi 5 Onorificenze 6 No...

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Januari 2023. SMP KallistaInformasiJenisSekolah SwastaAlamatLokasi, Batam, Kepri, IndonesiaMoto SMP Kallista, merupakan salah satu Sekolah Menengah Pertama swasta yang ada di Batam, Provinsi Kepulauan Riau. Sama dengan SMP pada umumnya di Indonesia masa pendid...

French Catholic priest and philosopher (1782–1854) Felicité de La Mennais (1827); portrait by L. D. Lancôme Part of a series onCatholic philosophy Aquinas, Scotus, and Ockham Ethics Cardinal virtues Just price Just war Principle of Double Effect Casuistry Probabilism Natural law Personalism Social teaching Liberation Theology Christian Humanism Virtue ethics Works of mercy Metaphysics Conceptualism Realism Moderate realism Nominalism Quiddity (essence / nature) Haecceity Quinqu...

Sir Peter Mallore (or Mallory; died c. 1380) was a prominent landowner and local politician in fourteenth-century Northamptonshire, who also served as a judge in Ireland.[1] His career was marked by controversy: he was imprisoned on at least two occasions, the second time for assaulting another judge.[1] The troubles of his later years were due largely to the actions of his son Giles, who was accused of wasting the inheritance of his infant stepson and ward.[2] Fortun...

Founding emperor of the Nguyễn dynasty (r. 1802–04) You can help expand this article with text translated from the corresponding article in Vietnamese. (November 2021) Click [show] for important translation instructions. View a machine-translated version of the Vietnamese article. Machine translation, like DeepL or Google Translate, is a useful starting point for translations, but translators must revise errors as necessary and confirm that the translation is accurate, rather than si...

Voce principale: Unione Calcio Sampdoria. Unione Calcio SampdoriaStagione 1985-1986 Sport calcio Squadra Sampdoria Allenatore Eugenio Bersellini Presidente Paolo Mantovani Serie A12º posto Coppa ItaliaFinale Coppa delle CoppeOttavi di finale Maggiori presenzeCampionato: Bordon (30)Totale: Bordon (46) Miglior marcatoreCampionato: Mancini, Vialli (6)Totale: Mancini (12) StadioLuigi Ferraris Abbonati10106 1984-1985 1986-1987 Si invita a seguire il modello di voce Questa voce raccoglie le ...

For the religious kibbutz, see Kvutzat Yavne. For the town with a similar name, see Gan Yavne. For the yeshiva with a similar name, see Kerem Yavne. For the former Arab village, see Yibna. For the Jamnia in Galilee, see Jamnith. City in IsraelYavne יַבְנֶהCityHebrew transcription(s) • ISO 259Yabne Emblem of YavneYavneShow map of Central IsraelYavneShow map of IsraelCoordinates: 31°53′N 34°44′E / 31.883°N 34.733°E / 31.883; 34.733C...

2019 EP by NU'ESTThe TableDigital and streaming coverEP by NU'ESTReleasedOctober 21, 2019 (2019-10-21)GenreK-popLength19:38LabelPledis EntertainmentProducer Baekho Bumzu NU'EST chronology Happily Ever After(2019) The Table(2019) The Nocturne(2020) Singles from The Table Love MeReleased: October 21, 2019 The Table is the seventh Korean extended play by the South Korean boy band NU'EST, released on October 21, 2019. Background and release On September 30, 2019, Pledis En...

British economist (1906–1983) Sir Roy George Douglas AllenCBEAllen in 1978Born(1906-06-03)3 June 1906Stoke-on-Trent, Staffordshire, EnglandDied29 September 1983(1983-09-29) (aged 77)[1]Southwold, Suffolk, EnglandAlma materUniversity of CambridgeKnown forPartial elasticity of substitutionAwardsGuy Medal (gold, 1978)Scientific careerFieldsStatisticsInstitutionsLondon School of Economics Sir Roy George Douglas Allen, CBE, FBA (3 June 1906 – 29 September 1983) was an En...

Questa voce sull'argomento calciatori italiani è solo un abbozzo. Contribuisci a migliorarla secondo le convenzioni di Wikipedia. Segui i suggerimenti del progetto di riferimento. Giacomo NeriNazionalità Italia Calcio RuoloAla Termine carriera1949 - giocatore1959 - allenatore CarrieraGiovanili 19??-1931 Faenza Squadre di club1 1931-1932 Faenza? (1)1932-1933 Libertas Rimini? (?)1933-1936 Livorno46 (4)1936-1937 Juventus11 (1)1937-1939 Livorno53 (11)193...
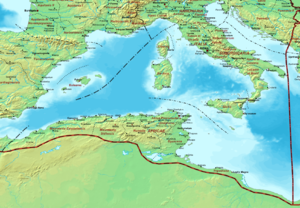
Roman Civil War (432)Part of Fall of the Western Roman EmpireMap of Africa and ItalyDate432LocationItaliaResult Victory of AetiusCommanders and leaders Bonifatius †Sebastianus AetiusStrength 7.000 - 10.000[1] 7.000 - 10.000 The Roman Civil War (432) was a military conflict between the Roman generals Aëtius and Bonifatius during the reign of Emperor Valentinian III. This war took place on Italian soil and was decided in the Battle of Rimini. Despite Aëtius losing the battl...

Lolette PayotLolette Payot nel 1929Nazionalità Svizzera Francia (dal 1937) Tennis Carriera Singolare1 Vittorie/sconfitte Titoli vinti Miglior ranking 4ª (1934)[1] Risultati nei tornei del Grande Slam Australian Open Roland Garros QF (1932, 1934, 1935) Wimbledon QF (1931, 1933, 1934) US Open Doppio1 Vittorie/sconfitte Titoli vinti Miglior ranking Risultati nei tornei del Grande Slam Australian Open Roland Garros SF (1933) Wimbledon ...

Every set is smaller than its power set For other theorems bearing Cantor's name, see Cantor's theorem (disambiguation). The cardinality of the set {x, y, z}, is three, while there are eight elements in its power set (3 < 23 = 8), here ordered by inclusion. This article contains special characters. Without proper rendering support, you may see question marks, boxes, or other symbols. In mathematical set theory, Cantor's theorem is a fundamental result which states that, for any set A {\dis...

لمعانٍ أخرى، طالع مهرة (توضيح). هذه المقالة عن قبيلة المهرة. للمحافظة اليمنية، طالع مهرة (محافظة). مهرةمحافظة المهرة في اليمنمناطق الوجود المميزة اليمن (المهرة، سقطرى)اللغات العربية، المهرية، السقطرية [1]الدين الإسلام (سني)المجموعات العرقية المرتبطةمجم...
