Read other articles:

Species of plant Resin spurge CITES Appendix II (CITES)[1] Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Clade: Rosids Order: Malpighiales Family: Euphorbiaceae Genus: Euphorbia Species: E. resinifera Binomial name Euphorbia resiniferaA.Berger Euphorbia resinifera, the resin spurge, is a species of spurge native to Morocco, where it occurs on the slopes of the Atlas Mountains. The dried latex of the plant was used in ancie...

أفنان فؤاد معلومات شخصية اسم الولادة أفنان فؤاد [1] الميلاد 28 يوليو 1990 (العمر 33 سنة)الرياض، السعودية مواطنة السعودية  الحياة العملية المهنة ممثلة  اللغة الأم العربية  اللغات العربية  سنوات النشاط 2011 - حتى الآن المواقع السينما.كوم صفحتها على موقع السينما تعديل ...

Not to be confused with Fairfield County, Ohio. For the former village in Greene County, see Fairborn, Ohio. City in Ohio, United StatesFairfield, OhioCityFairfield Community Arts Center FlagSealNickname: City of OpportunityLocation in OhioLocation of Fairfield in Butler CountyCoordinates: 39°19′44″N 84°32′34″W / 39.32889°N 84.54278°W / 39.32889; -84.54278CountryUnited StatesStateOhioCountiesButler, HamiltonFounded1787Incorporated1955Reclassified as ci...

Artikel ini tidak memiliki referensi atau sumber tepercaya sehingga isinya tidak bisa dipastikan. Tolong bantu perbaiki artikel ini dengan menambahkan referensi yang layak. Tulisan tanpa sumber dapat dipertanyakan dan dihapus sewaktu-waktu.Cari sumber: 7 Kurcaci – berita · surat kabar · buku · cendekiawan · JSTOR 7 KurcaciLahir1998Bandung, Jawa BaratGenreNu metal, Rap rock, Alternative metalTahun aktif2000 - sekarangLabelIndie Label (2008-sekarang) Mus...

Suatu kain bingata berpola bunga. Bingata adalah jenis tekstil yang diproduksi secara tradisional di Okinawa. Bin berarti warna dan gata berarti stensil kertas yang digunakan untuk menciptakan pola yang diberi warna. Penyebutan nama lain : Ryukyu Bingata.[1] Sejarah Kimono bingata yang dikenakan penari dalam Tari Istana Ryukyu Tidak diketahui dari mana asal mula Bingata. Kemungkinan besar hasil dari pertukaran budaya antara Ryukyu dengan Tiongkok, India dan Indonesia.[1] ...

Final of the 2020–21 EFL Cup Football match2021 EFL Cup FinalManchester City players celebrating their Carabao Cup victoryEvent2020–21 EFL Cup Manchester City Tottenham Hotspur 1 0 Date25 April 2021 (2021-04-25)VenueWembley Stadium, LondonMan of the MatchRiyad Mahrez (Manchester City)[1]RefereePaul Tierney (Lancashire)[2]Attendance7,773[a]← 2020 2022 → The 2021 EFL Cup Final was the final of the 2020–21 EFL Cup. It was played at Wemble...

Sports bar and music venue in Chicago, Illinois This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed.Find sources: The Cubby Bear – news · newspapers · books · scholar · JSTOR (November 2010) (Learn how and when to remove this message) The Cubby BearThe Cubby Bear in 2022Location within ChicagoGeneral informationAddress1059 W....

James TytlerPortrait de James Tytler.BiographieNaissance 17 décembre 1745Fearn, Angus, ÉcosseDécès 11 janvier 1804 (à 58 ans)Salem, Massachusetts, États-UnisNationalité ÉcossaisFormation Théologie, médecineActivités Écrivain, aviateur, chirurgien, pharmacienFratrie Henry William Tytlermodifier - modifier le code - modifier Wikidata James Tytler, né le 17 décembre 1745[1],[2],[3],[4],[n 1] à Fearn, Angus et mort le 11 janvier 1804 à Salem (Massachusetts), est un ministre d...

本條目存在以下問題，請協助改善本條目或在討論頁針對議題發表看法。 此條目需要編修，以確保文法、用詞、语气、格式、標點等使用恰当。 (2015年7月23日)請按照校對指引，幫助编辑這個條目。（幫助、討論） 此條目內容疑欠准确，有待查證。 (2015年7月23日)請在讨论页討論問題所在及加以改善，若此條目仍有爭議及准确度欠佳，會被提出存廢討論。 此條目之中立性有�...

此條目可参照英語維基百科相應條目来扩充。 (2021年5月6日)若您熟悉来源语言和主题，请协助参考外语维基百科扩充条目。请勿直接提交机械翻译，也不要翻译不可靠、低品质内容。依版权协议，译文需在编辑摘要注明来源，或于讨论页顶部标记{{Translated page}}标签。 约翰斯顿环礁Kalama Atoll 美國本土外小島嶼 Johnston Atoll 旗幟颂歌：《星條旗》The Star-Spangled Banner約翰斯頓環礁�...

مدرسة تشهارباغ مدرسه چهارباغ مدرسة تشهارباغ معلومات الموقع الجغرافي المدينة أصفهان البلد  إيران تعديل مصدري - تعديل   مدرسة تشهارباغ هي مدرسة تاريخية تعود إلى السلالة الصفوية، وتقع في أصفهان.[1] معرض الصور مراجع ^ Encyclopaedia of the Iranian Architectural History. Cultural Heritage, Handicrafts and Touri...

Cemplang CemplungGenreGaya hidupNaratorMpok AlpaArio AstungkoroNegara asalIndonesiaBahasa asliBahasa IndonesiaProduksiDurasi30 menit (Sabtu-Minggu)Rumah produksiTrans7DistributorTrans MediaRilis asliJaringanTrans7Format gambarDolby Digital HD 16:9Format audioStereoDolby Digital 5.1RilisSabtu, 27 Juni 2020 –Minggu, 27 Desember 2020Acara terkaitSemua Bisa Masak (di Trans TV) Masak Enak (di NET.) Cemplang Cemplung adalah sebuah program masak-memasak di Trans7 yang menyajikan resep-resep ...

Book by Richard Feynman Not to be confused with The Feynman Lectures on Physics. The Character of Physical Law First editionAuthorRichard FeynmanCountryUnited StatesLanguageEnglishSubjectPhysicsGenreNon-fictionPublished1999 (BBC)Pages173 pp.ISBN0679601279LC ClassQC71 .F44Websitefeynmanlectures.caltech.edu/messenger.html The Character of Physical Law is a series of seven lectures by physicist Richard Feynman concerning the nature of the laws of physics. Feynman delivered the lectures in 1...

A chapter in the Book of Nehemiah Nehemiah 13← chapter 12Esther 1 →Greek Orthodox Icon of Nehemiah, mounted on solid wood.BookBook of NehemiahCategoryKetuvimChristian Bible partOld TestamentOrder in the Christian part16 Nehemiah 13 is the thirteenth (and the final) chapter of the Book of Nehemiah in the Old Testament of the Christian Bible,[1] or the 23rd chapter of the book of Ezra-Nehemiah in the Hebrew Bible, which treats the book of Ezra and the book of Nehemiah as o...

Great Bear WildernessIUCN category Ib (wilderness area)Great Northern Mountain and Stanton Glacier.Location in MontanaLocationFlathead County, Montana, United StatesCoordinates48°11′10″N 113°35′03″W / 48.18611°N 113.58417°W / 48.18611; -113.58417Area286,700 acres (1,160 km2)Established1978Governing bodyU.S. Forest ServiceWebsiteMontana Office of Tourism The Great Bear Wilderness is located in northern Montana, United States, within Flathead Nation...

Over the RainbowPoster promosi untuk Over the RainbowGenreDrama, Musikal, RomansaDitulis olehHong Jin-ah Hong Ah-ramSutradaraHan HeePemeranJi Hyun-wooSeo Ji-hyeHwanheeKim Ok-binNegara asalKorea SelatanBahasa asliKoreaJmlh. episode16ProduksiProduserKim Nam-wonLokasi produksiKorea Selatan Selandia BaruDurasi60 menit pada hari Rabu dan Kamis pukul 21:55 (WSK)Rilis asliJaringanMBC (2006)Rilis26 Juli (2006-07-26) –14 September 2006 (2006-9-14) Korean nameHangul오버 더 레인�...

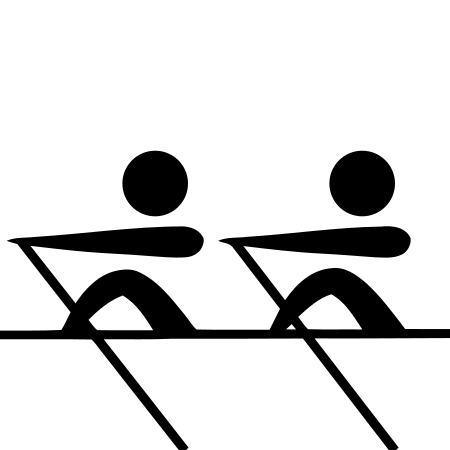
Aviron aux Jeux olympiques d'été de 1956 Généralités Sport Aviron Organisateur(s) CIO Éditions 12e Lieu(x) Melbourne, Australie Date du 23 au 27 novembre 1956 Nations 25 Participants 242 athlètes Épreuves 7 Site(s) Lac Wendouree Navigation Helsinki 1952 Rome 1960 modifier Les épreuves d'aviron lors des Jeux olympiques d'été de 1956 ont eu lieu du 23 au 27 novembre 1956 à Ballarat, au Nord-Ouest de Melbourne, en Australie. Les compétitions rassemblent 242 athlètes, issus de 25 f...

Small hole drilled into a workpiece to guide a larger drill or other tool Drilling pilot holes to hammer nails In construction, a pilot hole is a small hole drilled into a piece of construction material. Its purpose may be: to guide a larger drill to the appropriate location and ease the job of the larger drill, allow the insertion of another hole-making tool, such as a knockout punch, that will produce the final-sized hole, or locate, guide, and provide clearance for a self-threading screw i...

Pour les articles homonymes, voir Un ennemi du peuple. Un ennemi du peuple Page de titre du manuscrit de 1882. Auteur Henrik Ibsen Genre Drame contemporain Nb. d'actes 5 Dates d'écriture 1882 Version originale Titre original En Folkefiende Langue originale Norvégien Pays d'origine Norvège Éditeur original Gyldendalske Boghandel Lieu de parution originale Copenhague Date de parution originale 28 Novembre 1882 Date de création 13 janvier 1883 Lieu de création Théâtre Christiana, Oslo V...

Type of matrix in algebraic graph theory In the mathematical field of algebraic graph theory, the degree matrix of an undirected graph is a diagonal matrix which contains information about the degree of each vertex—that is, the number of edges attached to each vertex.[1] It is used together with the adjacency matrix to construct the Laplacian matrix of a graph: the Laplacian matrix is the difference of the degree matrix and the adjacency matrix.[2] Definition Given a graph G...