Karel Purkyně

Karel Purkyně
Karel Purkyně (portrét od Jana Vilímka z r. 1882)
Karel Purkyně (portrét od Jana Vilímka z r. 1882)
Narození15. března 1834
Breslau
PruskoPrusko Prusko
Úmrtí5. dubna 1868 (ve věku 34 let)
Praha-Nové Město
Rakousko-UherskoRakousko-Uhersko Rakousko-Uhersko
Místo pohřbeníVyšehradský hřbitov[1]
Povolánímalíř
RodičeJan Evangelista Purkyně a Julie Purkyně-Rudolphi
Manžel(ka)Marie Purkyňová
DětiCyrill Purkyně
Jan Purkyně
Rozálie Pokorná
PříbuzníEmanuel Purkyně, Rozálie Purkyňová a Johana Purkyňová (sourozenci)
Karl Asmund Rudolphi (děd)
Cyril Purkyně (vnuk)
PodpisPodpis
Logo Wikimedia Commons multimediální obsah na Commons
Logo Wikizdrojů původní texty na Wikizdrojích
Logo Wikicitátů citáty na Wikicitátech
Seznam děl v databázi Národní knihovny
Některá data mohou pocházet z datové položky.

Příbuzenstvo
syn Cyril Purkyně
syn Jan Purkyně
otec Jan Evangelista Purkyně
bratr Emanuel Purkyně
děd Karl Asmund Rudolphi
strýc Josef Jindřich Purkyně
zeť Rudolf Pokorný
snacha Eliška Purkyňová

Karel Purkyně (15. března 1834 Vratislav, nyní Polsko – 5. dubna 1868 Praha-Nové Město[2]) byl český malíř, kreslíř a výtvarný kritik. Jako portrétista se zařadil mezi přední osobnosti evropského realismu poloviny 19. století.[3]

Život

Narodil se ve Vratislavi jako druhý syn lékaře a přírodovědce Jana Evangelisty Purkyně a jeho manželky Julie, rozené Rudolphi. Matka brzy zemřela[4]. Měl staršího bratra, pozdějšího přírodovědce Emanuela Purkyně. Mládí prožili ve Vratislavi, kde otec působil na univerzitě. Zde také studoval na gymnáziu a krátce se učil malovat u malíře podobizen J.J. Königa.[3] V roce 1850 se rodina vrátila do Prahy a Karel se zapsal na malířskou Akademii. Poprvé se veřejně představil jako malíř v roce 1853 na výstavě Krasoumné jednoty, kam zaslal žánrovou kompozici Děti dívající se na obrázky.[3][5] Během studií navštěvoval známé německé galerie, v nichž studoval renesanční a barokní malířská díla. Navázal mnoho uměleckých kontaktů, přátelství ho pojilo především s Josefem Mánesem a Václavem Levým.[5][6] Studium na Akademii ho však neuspokojovalo, považoval je za suchý akademismus, odmítal kreslit podle sádrových modelů. Proto odešel roku 1854 do Mnichova do učení k malíři J.B.Berdellému.[3][5]. V galerii tam studoval Rembrandtovy, Tizianovy a Rubensovy obrazy, Na Tizianovi obdivoval monumentální pojetí portrétovaných osob a převzal některé technické postupy, jako podmalbu červenohnědou barvou[7].

Na podzim roku 1856 odjel do Paříže, kde se nejprve na otcovo přání zapsal do ateliéru Thomase Coutura. Ani toto studium nedokončil, což ovlivnilo negativně názor rodiny i širší kulturní veřejnosti, která akademickému vzdělání přikládala velký význam. On sám se však raději učil z prací italských a francouzských mistrů. Byl mu blízký realismus Gustava Courbeta, o kterém se později zmiňoval ve svých kritických statích.[3] Dojmy z ročního pobytu v Paříži vyjádřil v několika drobných olejomalbách, např. V údolí nebo Boulogneský lesík.[5]

Koncem roku 1857 se vrátil do Prahy a věnoval se intenzivní práci. Vytvořil řadu mistrovských portrétů a žánrových kompozic, např. podobiznu Betty Reitmeierové, protějškové portréty Emy a Leopolda Křikavových, portrét Barbory Wiesnerové či Podobiznu rodiny řezbáře Vorlíčka. Vedle portrétní tvorby se od roku 1860 více zaměřil na malbu zátiší. Spojení obou těchto oborů je patrné na jednom z jeho stěžejních obrazů Podobizna kováře Jecha.

Hrob Karla Purkyně a Václava Levého na Vyšehradě

Od roku 1862 se Purkyně kromě malování věnoval umělecké kritice, psal do Národních listů, Politika a Květů. Kreslil karikatury pro časopisy Brejle a Humoristické listy. Zapojil se do pražského spolkového života a v roce 1864 se podílel na přípravě slavnosti k třístému výročí narození Williama Shakespeara, kterou organizovala Umělecká beseda. Navrhl kostýmy a scénu pro slavnostní průvod shakespearovských postav. Soubor šesti olejomaleb je ve sbírkách Národní galerie.[6] V roce 1866 přijal zakázku na ilustrování nového knižního vydání Dona Quijota.[5][8]

Karel Purkyně se 7. února 1860 v Praze oženil s Marií Wiedermannovou z Vídně (1841–1914)[9], měli spolu tři děti, Jana, Cyrila a Rosalii (Růženu).[10]. Portrét své manželky vytvořil v roce 1860, své děti zachytil na obraze z roku 1868. Těsně před smrtí namaloval autoportrét vyjadřující jeho vnitřní rozpoložení. Karel Purkyně zemřel předčasně ve věku 34 let a byl pohřben na Vyšehradském hřbitově vedle svého přítele Václava Levého (hrob 2A-28). Vdova Marie Purkyňová ve vysokém věku v Praze dožila v Ústavu choromyslných[11].

Dcera Růžena, provdaná Pokorná, byla malířkou a napsala o svém rodu knihu s titulem Život tří generací, kterou vydala v roce 1944 Umělecká beseda.[8] Syn Jan byl inženýrem v oboru technické hygieny a Cyril se věnoval přírodním vědám, stal se ředitelem Státního geologického ústavu.[6]

Dílo

Je považován za jednoho z nejnadanějších českých realistických malířů, avšak za svého života se uznání nedočkal. Teprve moderní umělci 20. století ocenili Purkyněho jako významného malíře 19. století.[12]

Výtvarné práce

Maloval především portréty (Portrét paní Reitmayerové, Portrét slečny Bubeníčkové, Portrét hospodyně J. E. Purkyně „Máry“, Portrét rodiny J. Szalatnaye, Portrét vlastní manželky, Portrét malého syna, Skupinový portrét tří vlastních dětí, Autoportrét a další), žánrové figurální kompozice a zátiší.

V portrétech navázal na renesanční a barokní malbu, jeho realismus čerpal ze znalosti umění starých mistrů a z usilovného studia skutečnosti. Vytvořil si vlastní styl, přitom každý obraz má své osobité kompoziční řešení. Jeho portréty charakterizuje živost a reálnost figur, mají symbolický obsah a psychologickou hloubku. Usiloval o maximální pravdivost v charakteristice lidské osobnosti a téměř vědeckou metodou řešil problémy kompozice, linií i barevnost. Subjektivní pocity jsou vyjádřeny především v jeho posledních dílech z roku 1868: Podobizna umělcových dětí a Autoportrét. [13]

Ke své portrétní tvorbě připojil od roku 1860 jako samostatný obor malbu zátiší. Mezi jeho díla patří například portrét Podobizna kováře Jecha, zvaný též Politizující kovář. Obraz v sobě slučuje monumentální portrét kováře čtoucího noviny a soubor několika mistrně podaných zátiší, zobrazujících kovářské náčiní i předměty domácí potřeby.[13]

Purkyně nanášel barvu na plátno v tekutých pastách nebo hutnou špachtlí, seříznutou nožem, na povrchu potaženou tenkou lazurou. Základ tvořily tmavé podmalby, na které nanášel v hustých pastách barevné tóny. Plasticity tvarů a charakteru materiálů docílil bělobovými světly a hustším či řidším rozložením barevných past. Ty později spojoval lehkou lazurní malbou. Tmavými těžkými pastami vytvářel plasticitu zobrazovaných tvarů a zároveň strukturoval jejich hmotu, jež byla jedním z nejpodstatnějších znaků jeho tvorby.[13] Tento styl byl negativně posuzován jak kritiky, tak i společnosti, která v něm viděla malíře, který se pouze pokouší o realistickou malbu, ale nemá lehkost v barvách ani v používání světla a je doslova těžkopádný a do salónu se nehodící.[12] Neužíval žádného rafinovaného aranžování předmětů, jeho kompozice jsou prosté a bez efektů, navazují na klidnou atmosféru holandských zátiší.[6] Cit pro barvu a plasticitu prokázal ve svém mistrovském díle Sova sněžná, jehož barevná škála je tvořena z velké části různými tóny bílé, šedé a stříbřité barvy. Malířská interpretace povrchu látek a struktur vyniká i v obrazech Zátiší s pávem, Zátiší s cibulí a koroptvemi a Bažanti.

Kritik a organizátor

Purkyně byl činný v letech 1862 až 1866 také jako umělecký kritik. Snažil se o prosazení pravdy v umění proti lži a konvenci, zabýval se problematikou uměleckého školství a výtvarné výchovy. Byl jedním (spolu se svým otcem) ze zakladatelů spolku Umělecká beseda roku 1863, pracoval na slavnosti k 300 letům Shakespeara v Praze, spoluorganizoval výstavu Vesny v Kutné Hoře roku 1867, psal hodně do Kritické přílohy k Národním listům.[14]

Pozůstalost

Osobní předměty z Purkyňovy domácnosti uchovávala dcera Růžena a darovala je do sbírek oddělení starších českých dějin Národního muzea v Praze.

Galerie

  • Karel Purkyně – Sova sněžná (1862)
    Karel Purkyně – Sova sněžná (1862)
  • Karel Purkyně – Zátiší s pávem (1861)
    Karel Purkyně – Zátiší s pávem (1861)
  • Karel Purkyně - Zátiší se zajícem a rakem 1858
    Karel Purkyně – Zátiší se zajícem a rakem (1858)
  • Karel Purkyně – Umělcovy děti (1868)
    Karel Purkyně – Umělcovy děti (1868)
  • Karel Purkyně – Hospodyně Jana Ev. Purkyně (1858)
    Karel Purkyně – Hospodyně Jana Ev. Purkyně (1858)
  • Portrét kováře Jecha – Politizující kovář (1860)
    Portrét kováře Jecha – Politizující kovář (1860)

Reference

  1. ↑ hrob Karla Purkyně na Vyšehradském hřbitově v Praze. 212.47.2.130 [online]. [cit. 2019-03-16]. Dostupné v archivu pořízeném z originálu dne 2020-03-29. 
  2. ↑ Matriční záznam o úmrtí a pohřbu
  3. ↑ a b c d e POCHE, Emanuel, a kol. Encyklopedie českého výtvarného umění. Praha: Academia, 1975. S. 401–402. 
  4. ↑ http://katalog.ahmp.cz/pragapublica/permalink?xid=397F7E0B953E11E287F174F06DCD07FC&scan=1#scan1
  5. ↑ a b c d e CODR, Milan; MACKOVÁ, Olga. Přemožitelé času sv. 4. Praha: Mezinárodní organizace novinářů, 1988. Kapitola Karel Purkyně, s. 181–185. 
  6. ↑ a b c d 916. schůzka: Nepodařený syn velkého otce. Dvojka [online]. 2013-01-13 [cit. 2019-10-22]. Dostupné online. 
  7. ↑ Volavka, 1968
  8. ↑ a b LOUŽECKÝ, Pavel. Pozitivní noviny › Dobromila Lebrová: Karel Purkyně, malíř a výtvarný kritik. www.pozitivni-noviny.cz [online]. [cit. 2019-10-20]. Dostupné online. 
  9. ↑ VOLAVKA, Vojtěch. Karel Purkyně. Praha: Melantrich, SVU Mánes, 1942. Kapitola Purkyně doma, s. 70. 
  10. ↑ dní archiv, Policejní ředitelství I, konskripce, karton 496, obraz 401, Purkyně, von Karl
  11. ↑ http://katalog.ahmp.cz/pragapublica/permalink?xid=494FBAE5953E11E287F174F06DCD07FC&scan=1#scan1
  12. ↑ a b NASREGION.CZ. Karel Purkyně. Společnost v něm viděla malíře, který se pouze pokouší o realistickou malbu, ale do salónu se nehodí. Náš REGION [online]. 2019-03-15 [cit. 2019-10-22]. Dostupné v archivu pořízeném z originálu dne 2019-10-22. 
  13. ↑ a b c LAB.SNG. Národní galerie v Praze - sbírky. Národní galerie v Praze - sbírky [online]. [cit. 2019-10-22]. Dostupné online. 
  14. ↑ Karel Purkyně, s. 87

Literatura

  • POKORNÁ-PURKYŇOVÁ, Růžena. Život tří generací. 1. vyd. Praha: Výtvarný odbor Umělecké besedy, 1944. 391 s.  - listy a články Karla Purkyně
  • MACKOVÁ, Karel Purkyně 1834-1868, NG Praha 1962
  • VOLAVKA Vojtěch, Karel Purkyně, SNKLU Praha 1962
  • VOLAVKA Vojtěch, České malířství a sochařství 19. století. SPN Praha 1968
  • Nová encyklopedie českého výtvarného umění, Nová encyklopedie českého výtvarného umění, redaktor Anděla Horová. Academia Praha 1998, s. 664-665; ISBN 80-200-0522-6

Externí odkazy

Read other articles:

Inning am Ammersee

Inning a.Ammersee Lambang kebesaranLetak Inning a.Ammersee di Starnberg NegaraJermanNegara bagianBayernWilayahOberbayernKreisStarnbergSubdivisions6 OrtsteilePemerintahan • MayorWerner Röslmair (FBB)Luas • Total24,43 km2 (943 sq mi)Ketinggian553 m (1,814 ft)Populasi (2013-12-31)[1] • Total4.460 • Kepadatan1,8/km2 (4,7/sq mi)Zona waktuWET/WMPET (UTC+1/+2)Kode pos82266Kode area telepon08143Pelat kendaraan...

 

Rob Wielaert

Artikel ini sebatang kara, artinya tidak ada artikel lain yang memiliki pranala balik ke halaman ini.Bantulah menambah pranala ke artikel ini dari artikel yang berhubungan atau coba peralatan pencari pranala.Tag ini diberikan pada Desember 2023. Rob Wielaert Informasi pribadiNama lengkap Rob WielaertTanggal lahir 29 Desember 1978 (umur 45)Tempat lahir Emmeloord, BelandaTinggi 1,78 m (5 ft 10 in)Posisi bermain BekInformasi klubKlub saat ini Roda JC KerkradeNomor 4Karier sen...

 

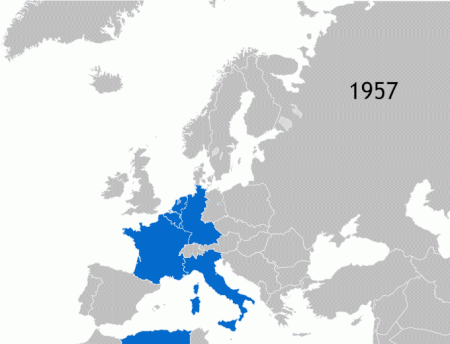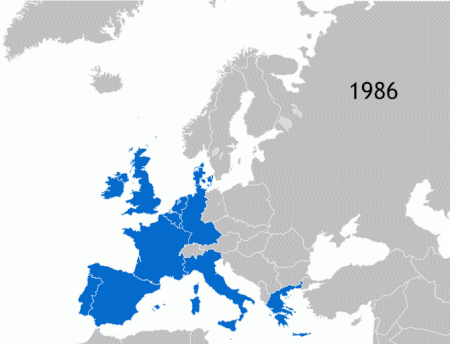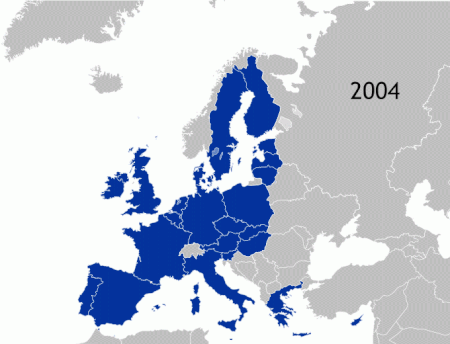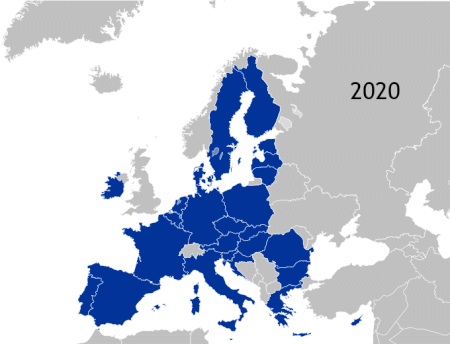
الدول الأعضاء في الاتحاد الأوروبي

دولة عضو في الاتحاد الأوروبينوع التقسيمدولة عضوالدولةالاتحاد الأوروبيالإنشاء1952/1958/1993[أ]العدد27 (اعتباراً من 2022)الأنواع المحتملةجمهورية (21)ملكية (6)السكان446,828,803 (2022)[1]المناطق4,233,255 كـم2 (1,634,469 ميل2)الحكومةديمقراطية تمثيلية برلمانية (21)ديمقراطية تمثيلية شبه رئاس�...

كثافة سكانية

كثافة السكان (الأشخاص لكل كم2) خريطة العالم عام 1994. باعتبار خط الاستواء منصف يمكن ملاحظة أن الغالبية العظمى من سكان الكوكب يعيشون في النصف الشمالي للأرض. الكثافة السكانية (الأشخاص لكل كم2) خريطة العالم عام 1994 توزع الصحاري حول العالم، بالمقارنة مع خرائط التوزع السكاني في الأع...

 

Steve King

Steven Arnold King (lahir 28 Mei 1949) adalah seorang politikus dan mantan pengusaha asal Amerika Serikat. Ia menjabat sebagai anggota DPR dari Iowa sejak 2003. Ia merupakan anggota Partai Republik Pranala luar Wikimedia Commons memiliki media mengenai Steve King (Iowa politician). Wikiquote memiliki koleksi kutipan yang berkaitan dengan: Steve King. Congressman Steve King Diarsipkan 2019-01-16 di Wayback Machine. official U.S. House website Steve King for Congress Diarsipkan 2018-03-06 di W...

 

Первородный грех

Об экономическом термине см. Первородный грех (экономика). ХристианствоБиблия Ветхий Завет Новый Завет Евангелие Десять заповедей Нагорная проповедь Апокрифы Бог, Троица Бог Отец Иисус Христос Святой Дух История христианства Апостолы Хронология христианства Ран�...

Living history

Historical reenactment This article is about the term as it applies to historical reenactors. For Hillary Clinton's autobiography, see Living History (book). A reenactress playing the role of Mary Queen of Scots at a Scottish fair in 2003. Living history is an activity that incorporates historical tools, activities and dress into an interactive presentation that seeks to give observers and participants a sense of stepping back in time. Although it does not necessarily seek to reenact a specif...

 

Symphony No. 9 (Schubert)

Symphony by Franz Schubert Symphony No.9The Greatby Franz SchubertSchubert in 1825KeyC majorCatalogueD 944Composed1824-1826Published1849PublisherBreitkopf & HärtelDuration55 minutesMovements4 The Symphony No. 9 in C major, D 944, known as The Great, is the final symphony completed by Franz Schubert. It was first published by Breitkopf & Härtel in 1849 as Symphonie / C Dur / für großes Orchester[1] and listed as Symphony No. 8 in the New Schubert Edition.[2 ...

 

土库曼斯坦总统

土库曼斯坦总统土库曼斯坦国徽土库曼斯坦总统旗現任谢尔达尔·别尔德穆哈梅多夫自2022年3月19日官邸阿什哈巴德总统府（Oguzkhan Presidential Palace）機關所在地阿什哈巴德任命者直接选举任期7年，可连选连任首任萨帕尔穆拉特·尼亚佐夫设立1991年10月27日 土库曼斯坦土库曼斯坦政府与政治 国家政府 土库曼斯坦宪法 国旗 国徽 国歌 立法機關（英语：National Council of Turkmenistan） ...

Christelijke theosofie

Esoterie Westerse esoterie Esoterie in de klassieke oudheid Esoterische vakgebieden Alchemie Occultisme Magie Astrologie Waarzeggerij Geomantiek Handlijnkunde Numerologie Tarot Esoterische stromingen Antroposofie Christelijke theosofie Gnosis Golden Dawn Hermetisme Iatrosofie Illuminisme Mysticisme Neoplatonisme Neopythagorisme New age New thought Pythagorisme Rozenkruisers Theosofie Vrijmetselarij Esoterische teksten De alchemische bruiloft van Christiaan Rozenkruis De occulta philosophia l...

 

Teocrito

Disambiguazione – Se stai cercando l'omonimo oratore di Chio, vedi Teocrito di Chio. Disambiguazione – Se stai cercando l'omonimo generale bizantino, vedi Teocrito (comes domesticorum). Incisione con busto immaginario di Teocrito Teocrito (in greco antico: Θεόκριτος?, Theókritos; Siracusa, 315 a.C. – 260 a.C. circa) è stato un poeta siceliota, inventore della poesia bucolica. Indice 1 Biografia 2 Opere 2.1 Idilli 2.2 Epigrammi 2.3 Zampogna 3 Poetica e poesia di Teocrito 4 No...

 

Play (theatre)

Dramatic literary form A performance of Macbeth (2018) Literature Oral literature Folklore fable fairy tale folk play folksong heroic epic legend myth proverb Oration Performance audiobook spoken word Saying Major written forms Drama closet drama Poetry lyric narrative Prose Nonsense verse Ergodic Electronic Long prose fiction Anthology Serial Novel/romance Short prose fiction Novella Novelette Short story Drabble Sketch Flash fiction Parable Religious Wisdom Prose genres Fiction Speculative...

Asa Gray

Asa GrayLahir(1810-11-18)18 November 1810Sauquoit, New YorkMeninggal30 Januari 1888(1888-01-30) (umur 77)Cambridge, MassachusettsKebangsaanAmerika SerikatKarier ilmiahBidangBotaniTerinspirasiAmos EatonCharles Darwin Tanda tangan Asa Gray (18 November 1810 – 20 Januari 1888) adalah botanis Amerika paling berpengaruh pada abad ke-19.[1][2] Gray menjadi profesor botani Universitas Harvard selama beberapa dekade. Kehidupan awal dan pendidikan Gray lahir di Sauquoit, New Yo...

 

Polydor Records

PolydorPerusahaan indukUniversal Music GroupDidirikan1924StatusAktifDistributorPolydor(kecuali A.S.)Interscope-Geffen-A&M(A.S.)GenreBeragamAsal negaraJermanLokasiBritania Raya; Amerika SerikatSitus webSitus web resmi Polydor Records Polydor atau Polydor Records Ltd. adalah label rekaman Inggris dan perusahaan yang beroperasi sebagai bagian dari Universal Music Group . Ini memiliki hubungan dekat dengan label Universal's Interscope Geffen A&M Records , yang mendistribusikan rilis Polyd...

 

Mode of transport

Different types of transportation such as air, water, and land transport Part of a series onTransport Modes Armored fighting vehicle Air Cable Human-powered Land Water Animal-powered Personal rapid transit pipeline transport Powered exoskeleton Rapid transit Road Space Supersonic Uncrewed vehicle Vactrain Topics Accessibility Bicycle transportation planning and engineering Cyclability Cycling infrastructure Engineering Free public transport Green transport hierarchy History Outline Public / P...

الدوري الإيطالي 1996–97

يفتقر محتوى هذه المقالة إلى الاستشهاد بمصادر. فضلاً، ساهم في تطوير هذه المقالة من خلال إضافة مصادر موثوق بها. أي معلومات غير موثقة يمكن التشكيك بها وإزالتها. (يوليو 2019) الدوري الإيطالي 1996–97 تفاصيل الموسم الدوري الإيطالي  النسخة 95  البلد إيطاليا  التاريخ بداية:8 سبتم�...

 

إطلاق نار

وضعية مثالية لإطلاق النار حيث توجد أكياس الرمل الحامية للجسد و أيضاً منطقة الزناد تلائم الجندي. إطلاق النار هي حركة أو فعل أو عملية إطلاق قذيفة باستخدام جميع أنواع سلاح من البنادق.[1][2][3] و يمكن تصنيف بعض القذائف مثل الصواريخ و المدفعية كأسلحة إطلاق النار، وكل ش�...

 

Amaranthaceae

Family of flowering plants Amaranthaceae Amaranthus retroflexus Scientific classification Kingdom: Plantae Clade: Tracheophytes Clade: Angiosperms Clade: Eudicots Order: Caryophyllales Family: AmaranthaceaeJuss. Type genus AmaranthusL. Subfamilies Amaranthoideae Betoideae Camphorosmoideae Chenopodioideae Corispermoideae Gomphrenoideae Polycnemoideae Salicornioideae Salsoloideae Suaedoideae Synonyms[1] Chenopodiaceae Vent. Amaranthaceae (/ˌæmərænˈθeɪsiaɪ, -iiː/ AM-ər-an-THAY-...

2009 New South Wales Swifts season

NSW Swifts season New South Wales Swifts2009 seasonHead coachJulie FitzgeraldAsst. coachTracey RobinsonManagerJan TroyCaptainCatherine CoxMain venueSydney Olympic Park Sports CentreSeason resultsWins–losses2–11Regular season9thFinals placingDNQ Team colours New South Wales Swifts seasons← 2008 2010 → The 2009 New South Wales Swifts season saw New South Wales Swifts play in the 2009 ANZ Championship. With a team coached by Julie Fitzgerald and captained by Cather...

 

Tree (graph theory)

Undirected, connected and acyclic graph TreesA labeled tree with 6 vertices and 5 edges.VerticesvEdgesv − 1Chromatic number2 if v > 1Table of graphs and parameters In graph theory, a tree is an undirected graph in which any two vertices are connected by exactly one path, or equivalently a connected acyclic undirected graph.[1] A forest is an undirected graph in which any two vertices are connected by at most one path, or equivalently an acyclic undirected graph, or equi...