Commutador (matemàtiques)
|
Read other articles:

Artikel ini menyajikan daftar kepala pemerintahan Denmark sejak 1699. Jabatan ini dijelaskan sepenuhnya dalam artikel Perdana Menteri Denmark. Monarki Denmark adalah kepala negara. Setiap kepala pemerintahan telah menjadi pemimpin dari satu atau lebih kabinet (untuk daftar lengkap, lihat Kabinet Denmark). Perdana Menteri Denmark saat ini adalah Lars Lokke Rasmussen yang mewakili partai Liberal Venstre . Lars Lokke Rasmussen menjabat pada tanggal 5 April 2009, mengambil alih posisi Anders Fogh...

American politician (born 1954) Cynthia LummisOfficial portrait, 2020United States Senatorfrom WyomingIncumbentAssumed office January 3, 2021Serving with John BarrassoPreceded byMike EnziMember of the U.S. House of Representativesfrom Wyoming's at-large districtIn officeJanuary 3, 2009 – January 3, 2017Preceded byBarbara CubinSucceeded byLiz Cheney27th Treasurer of WyomingIn officeJanuary 4, 1999 – January 9, 2007GovernorJim Geringer Dave Freudent...

PausSilvester IIAwal masa kepausan999Akhir masa kepausan12 Mei 1003PendahuluGregorius VPenerusYohanes XVIIInformasi pribadiNama lahirGerbert d'AurillacLahir±950Auvergne, PrancisWafat12 Mei 1003Roma, ItaliaPaus lainnya yang bernama Silvester Paus Silvester II, nama lahir Gerbert d'Aurillac (±950-12 Mei 1003), adalah Paus Gereja Katolik Roma sejak 999 hingga 12 Mei 1003. Didahului oleh:Gregorius V Paus999 – 1003 Diteruskan oleh:Yohanes XVII lbs Paus Gereja Katolik Daftar paus grafik ma...

James ThiérréeJames Thierrée di pratinjau Korkoro pada 2010.LahirJames Spencer Henry Edmond Marcel Thierrée2 Mei 1974 (umur 49)Lausanne, SwissPendidikanPiccolo Teatro CNSAD Acting International (Paris) Harvard Theater SchoolPekerjaanPementas sirkus, aktor, pengarah teaterTahun aktif1978–sekarangOrang tuaVictoria Chaplin Jean-Baptiste ThierréeKerabatCharlie Chaplin (kakek pihak ibu)Eugene O’Neill (kakek buyut) James Spencer Henry Edmond Marcel Thierrée (lahir 2 Mei 1974) a...

Robert LindstedtRobert Lindstedt di BRD Năstase Țiriac Trophy 2012Kebangsaan SwediaTempat tinggalBåstad, SwediaLahir19 Maret 1977 (umur 47)Sundbyberg, SwediaTinggi190 m (623 ft 4+1⁄2 in)Berat83 kg (183 pon; 13,1 st)Memulai pro1998Tipe pemainTangan kanan (backhand dua tangan)Total hadiah$1,920,253TunggalRekor (M–K)0–3Gelar0Peringkat tertinggiNo. 309 (26 April 2004)GandaRekor (M–K)244–195 (56%)Gelar16Peringkat tertinggiNo. 5 (20 Agustus 2...

Medical condition Auscultogram from normal and abnormal heart sounds Systolic heart murmurs are heart murmurs heard during systole,[1][2][3] i.e. they begin and end between S1 and S2. Many involve stenosis of the semilunar valves or regurgitation of the atrioventricular valves. Types Mid-systolic ejection murmurs are due to blood flow through the semilunar valves. They occur at the start of blood ejection — which starts after S1 — and ends with the cessation of the...

American DJ and record producer DJ ShadowDavis in October 2006Background informationBirth nameJoshua Paul DavisBorn (1972-06-29) June 29, 1972 (age 51)San Jose, California, U.S.OriginDavis, California, U.S.GenresHip hoptrip hopelectronicturntablismexperimentalOccupation(s)DJrecord producerYears active1989–present[citation needed]LabelsMass AppealMo' WaxQuannum ProjectsSolesidesMCAUniversalVerveLiquid AmberFormerly ofUnkleWebsitewww.djshadow.com Musical artist Joshua Paul Davis ...

Unsolicited electronic messages, especially advertisements This article is about unsolicited or undesirable electronic messages. For information specific to email, see Email spam. For other uses, see Spam (disambiguation). An email inbox containing a large amount of spam messages Spamming is the use of messaging systems to send multiple unsolicited messages (spam) to large numbers of recipients for the purpose of commercial advertising, for the purpose of non-commercial proselytizing, for any...

Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article adopte un point de vue régional ou culturel particulier et nécessite une internationalisation (juin 2015). Merci de l'améliorer ou d'en discuter sur sa page de discussion ! Vous pouvez préciser les sections à internationaliser en utilisant {{section à internationaliser}}. Si ce bandeau n'est plus pertinent, retirez-le. Cliquez ici pour en savoir plus. Cet article concernant le droit doit ê...
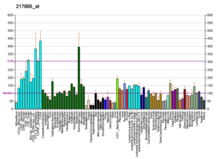
IPO9 المعرفات الأسماء المستعارة IPO9, Imp9, importin 9 معرفات خارجية MGI: MGI:1918944 HomoloGene: 5874 GeneCards: 55705 علم الوجود الجيني الوظيفة الجزيئية • GO:0031493 histone binding• GO:0001948، GO:0016582 ربط بروتيني• nuclear import signal receptor activity المكونات الخلوية • سيتوبلازم• غشاء• نواة• nuclear envelope• عصارة خلوية العمليات ا...

46th edition of the World Snooker Championship at the Crucible in Sheffield 2022 Betfred World Snooker ChampionshipTournament informationDates16 April – 2 May 2022 (2022-04-16 – 2022-05-02)VenueCrucible TheatreCitySheffieldCountryEnglandOrganisationWorld Snooker TourFormatRanking eventTotal prize fund£2,395,000Winner's share£500,000Highest break Neil Robertson (AUS) (147) Graeme Dott (SCO) (147)FinalChampion Ronnie O'Sullivan (...

ASD Castrovillari CalcioCalcio Rossoneri, Lupi del Pollino Segni distintiviUniformi di gara Casa Trasferta Colori sociali Nero, rosso SimboliLupo InnoInno del Castrovillari Dati societariCittàCastrovillari Nazione Italia ConfederazioneUEFA Federazione FIGC CampionatoSerie D Fondazione1921 Rifondazione2003Presidente Nicola Mazzuca Allenatore Davide Boncore StadioStadio Mimmo Rende(2 800 posti) Sito webwww.castrovillaricalcio.it PalmarèsSi invita a seguire il modello di voce L'A.S.D...

Omicidio a ManhattanTitolo originaleExiled: A Law & Order Movie PaeseStati Uniti d'America Anno1998 Formatofilm TV Generepoliziesco, giudiziario Durata120 min Lingua originaleInglese Rapporto16:9 CreditiIdeatoreDick Wolf RegiaJean de Segonzac SoggettoCharles Kipps, Chris Noth SceneggiaturaCharles Kipps Interpreti e personaggi Chris Noth: Mike Logan Dana Eskelson: Frankie Silvera John Fiore: Tony Profaci Paul Guilfoyle: Sammy Kurtz Benjamin Bratt: Rey Curtis Jerry Orbach: Lennie Brisco...

Сельское поселение России (МО 2-го уровня)Новотитаровское сельское поселение Флаг[d] Герб 45°14′09″ с. ш. 38°58′16″ в. д.HGЯO Страна Россия Субъект РФ Краснодарский край Район Динской Включает 4 населённых пункта Адм. центр Новотитаровская Глава сельского пос�...
2020年夏季奥林匹克运动会波兰代表團波兰国旗IOC編碼POLNOC波蘭奧林匹克委員會網站olimpijski.pl(英文)(波兰文)2020年夏季奥林匹克运动会(東京)2021年7月23日至8月8日(受2019冠状病毒病疫情影响推迟,但仍保留原定名称)運動員206參賽項目24个大项旗手开幕式:帕维尔·科热尼奥夫斯基(游泳)和马娅·沃什乔夫斯卡(自行车)[1]闭幕式:卡罗利娜·纳亚(皮划艇)&#...

Mantan MantenPoster promosiSutradaraFarishad LatjubaProduserAnggia KharismaKori AdyaningDitulis olehFarishad LatjubaJenny JusufPemeran Atiqah Hasiholan Arifin Putra Tutie Kirana Tio Pakusadewo Marthino Lio Oxcel Dodit Mulyanto Penata musikBontelSinematograferAmalia T. SPenyuntingAji PradityoPerusahaanproduksiVisinema PicturesJD.ID KaskusDistributorVisinema PicturesTanggal rilis 4 April 2019Negara IndonesiaBahasaBahasa IndonesiaPendapatankotorRp 1,3 miliar Mantan Manten adalah film Indon...

Частина серії проФілософіяLeft to right: Plato, Kant, Nietzsche, Buddha, Confucius, AverroesПлатонКантНіцшеБуддаКонфуційАверроес Філософи Епістемологи Естетики Етики Логіки Метафізики Соціально-політичні філософи Традиції Аналітична Арістотелівська Африканська Близькосхідна іранська Буддій�...

Germanic tribe The approximate positions of some Germanic peoples reported by Graeco-Roman authors in the 1st century. The Hettergouw at the lower Rhine in the Frankish Empire, named after the Hetware. The Chattuarii, also spelled Attoarii, were a Germanic tribe of the Franks.[1] They lived originally north of the Rhine in the area of the modern border between Germany and the Netherlands, but then moved southwards in the 4th century, as a Frankish tribe living on both sides of the Rhi...

Papan tanda dwibahasa di Candi Sewu Papan tanda adalah rancangan dengan tanda dan simbol untuk menyampaikan suatu pesan.[1][2] Papan tanda dapat berupa papan peringatan, papan petunjuk arah, papan reklame, dan papan nama. Bahan-bahannya bisa beraneka ragam, mulai dari kayu, besi, hingga akrilik.[3] Papan tanda melingkupi banyak karya grafis yang dibuat untuk memperlihatkan informasi kepada audiens tertentu. Papan tanda seringkali merujuk pada papan petunjuk arah yang b...

Type of adipose tissue Brown adipose tissueBrown adipose tissue in a woman shown in a FDG PET/CT examDetailsIdentifiersLatintextus adiposus fuscusAcronym(s)BATMeSHD002001THH2.00.03.4.00004 FMA20118Anatomical terminology[edit on Wikidata] Brown adipose tissue (BAT) or brown fat makes up the adipose organ together with white adipose tissue (or white fat).[1] Brown adipose tissue is found in almost all mammals. Classification of brown fat refers to two distinct cell populations with ...

![{\displaystyle x^{y}=x[x,y].\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/af430a356cf1eeb4ab2903c296c6eaf403ff3813)
![{\displaystyle [y,x]=[x,y]^{-1}.\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0d411502ee5a38f8e38da78adec78c1d77a624ec)
![{\displaystyle [x,zy]=[x,y]\cdot [x,z]^{y}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6a9b7c4a764e30d0e2c80b7f31778d427aa3e032)
![{\displaystyle [xz,y]=[x,y]^{z}\cdot [z,y].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/51d505bd20b4dfad61942e780f9019f2342a28df)
![{\displaystyle [x,y^{-1}]=[y,x]^{y^{-1}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/244315ff297e41b5321e6fea0958663f5cc9ad7a)
![{\displaystyle [x^{-1},y]=[y,x]^{x^{-1}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c2831a3e53635f018849a2e168cc77b79a2e6110)
![{\displaystyle [[x,y^{-1}],z]^{y}\cdot [[y,z^{-1}],x]^{z}\cdot [[z,x^{-1}],y]^{x}=1}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5341b9acd3fd04a066959fb2ac90365912e95c6b)
![{\displaystyle [[x,y],z^{x}]\cdot [[z,x],y^{z}]\cdot [[y,z],x^{y}]=1.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e48a3147f321ab3cf38ba0d5c80d3cc961f89f4c)
![{\displaystyle (xy)^{2}=x^{2}y^{2}[y,x][[y,x],y].\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/723f8ca3d7e630d0185a910008178b36b3dff779)
![{\displaystyle (xy)^{n}=x^{n}y^{n}[y,x]^{\binom {n}{2}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/128ae0855aada1e122d118d63c2bfa18a08eb603)
![{\displaystyle [A,A]=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7c816c8f8631ef069ad0b1aee64ed996ad8309ed)
![{\displaystyle [A,B]=-[B,A]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/37b04a066443e96c0b27d4b14f7f5d51c7b2b0c3)
![{\displaystyle [A,[B,C]]+[B,[C,A]]+[C,[A,B]]=0}](https://wikimedia.org/api/rest_v1/media/math/render/svg/046dbc0d4387302c8d321afb443e35bc1890fcac)
![{\displaystyle [A,BC]=[A,B]C+B[A,C]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1c7c96785faa7d6e5ecc7d8ada53830b24338c60)
![{\displaystyle [AB,C]=A[B,C]+[A,C]B}](https://wikimedia.org/api/rest_v1/media/math/render/svg/71ba3177011b65b292d8dc39dd2e3391f29b0a07)
![{\displaystyle [ABC,D]=AB[C,D]+A[B,D]C+[A,D]BC}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b9e755fd1fe3c26c40e007256c6976b9612e5d9b)
![{\displaystyle [AB,CD]=A[B,CD]+[A,CD]B=A[B,C]D+AC[B,D]+[A,C]DB+C[A,D]B}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fda84810a1e01196ebcdb3210f4c937cc6d78b17)
![{\displaystyle [[[A,B],C],D]+[[[B,C],D],A]+[[[C,D],A],B]+[[[D,A],B],C]=[[A,C],[B,D]]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/40f6bd454cad9666854cc31d21b2e7793b55103f)
![{\displaystyle [AB,C]=A\{B,C\}-\{A,C\}B}](https://wikimedia.org/api/rest_v1/media/math/render/svg/fad90f0741f643bc4ee92facd3f9e595e0b27bbb)


![{\displaystyle e^{A}Be^{-A}=B+[A,B]+{\frac {1}{2!}}[A,[A,B]]+{\frac {1}{3!}}[A,[A,[A,B]]]+\cdots \equiv e^{ad(A)}B.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dc4ed88fc8e27782071741ed0c27ee1c71b67ee5)
![{\displaystyle \ln \left(e^{A}e^{B}e^{-A}e^{-B}\right)=[A,B]+{\frac {1}{2!}}[(A+B),[A,B]]+{\frac {1}{3!}}\left([A,[B,[B,A]]]/2+[(A+B),[(A+B),[A,B]]]\right)+\cdots .}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e9c03c9773b1cfd40a8579422fa1205fae91bd36)
![{\displaystyle \ [\omega ,\eta ]_{gr}:=\omega \eta -(-1)^{\deg \omega \deg \eta }\eta \omega .}](https://wikimedia.org/api/rest_v1/media/math/render/svg/142ef2049d3c752c54baaa40729cd72ad586a8dd)
![{\displaystyle \operatorname {ad} _{x}(y)=[x,y].}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f91029e165eca327454ec2f76652cb3678cf46c6)




![{\displaystyle {\rm {ad}}([x,y])=[{\rm {ad}}(x),{\rm {ad}}(y)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4f98000cb464cce715b05d260758e53c19a3ca9c)
